Enhancement effect of frequency chirp on vacuum electron-positron pair production in strong field

Xie Bai-Song; Li Lie-Juan; Melike Mohamedsedik; Wang Li

doi:10.7498/aps.71.20220148

Abstract

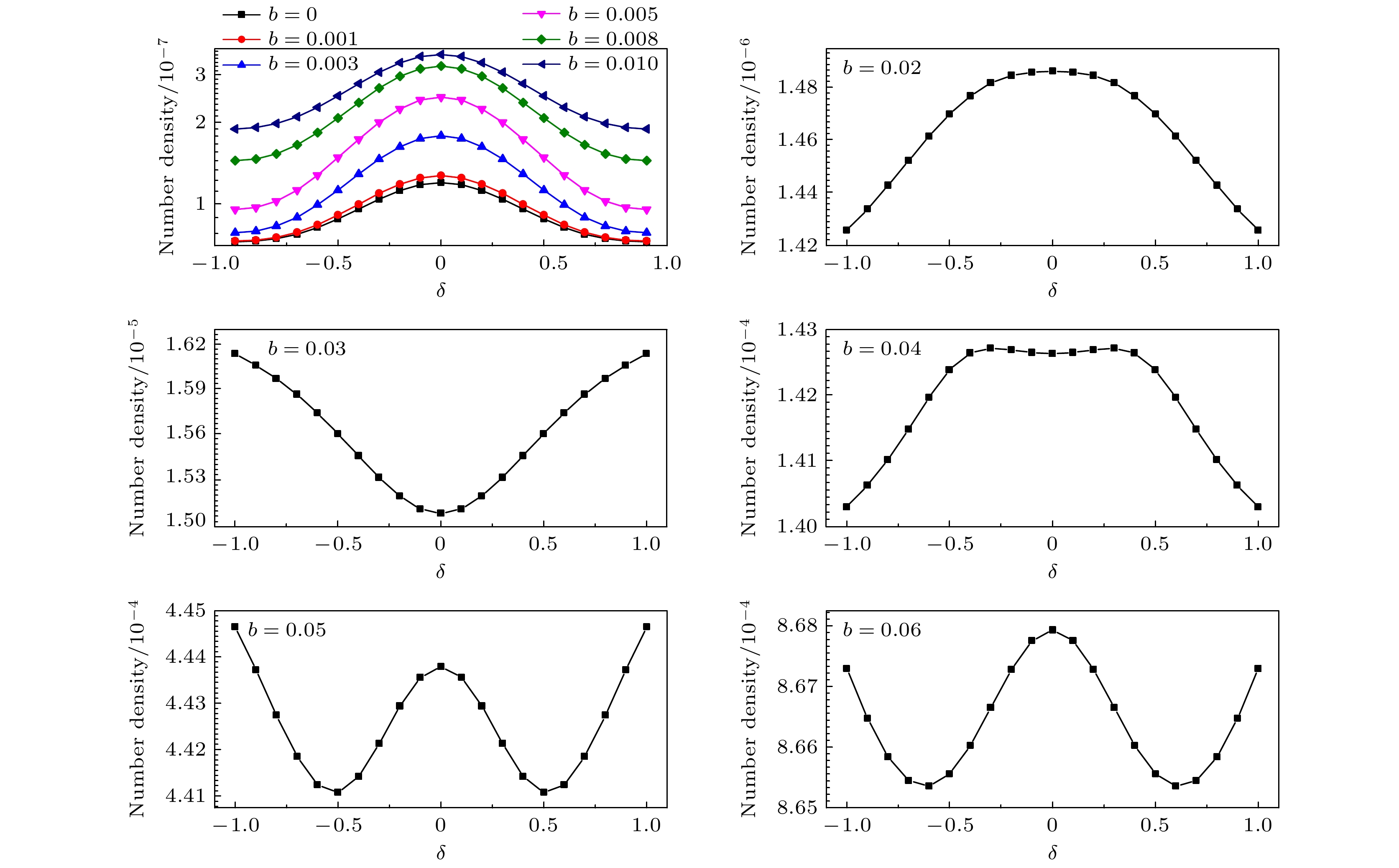
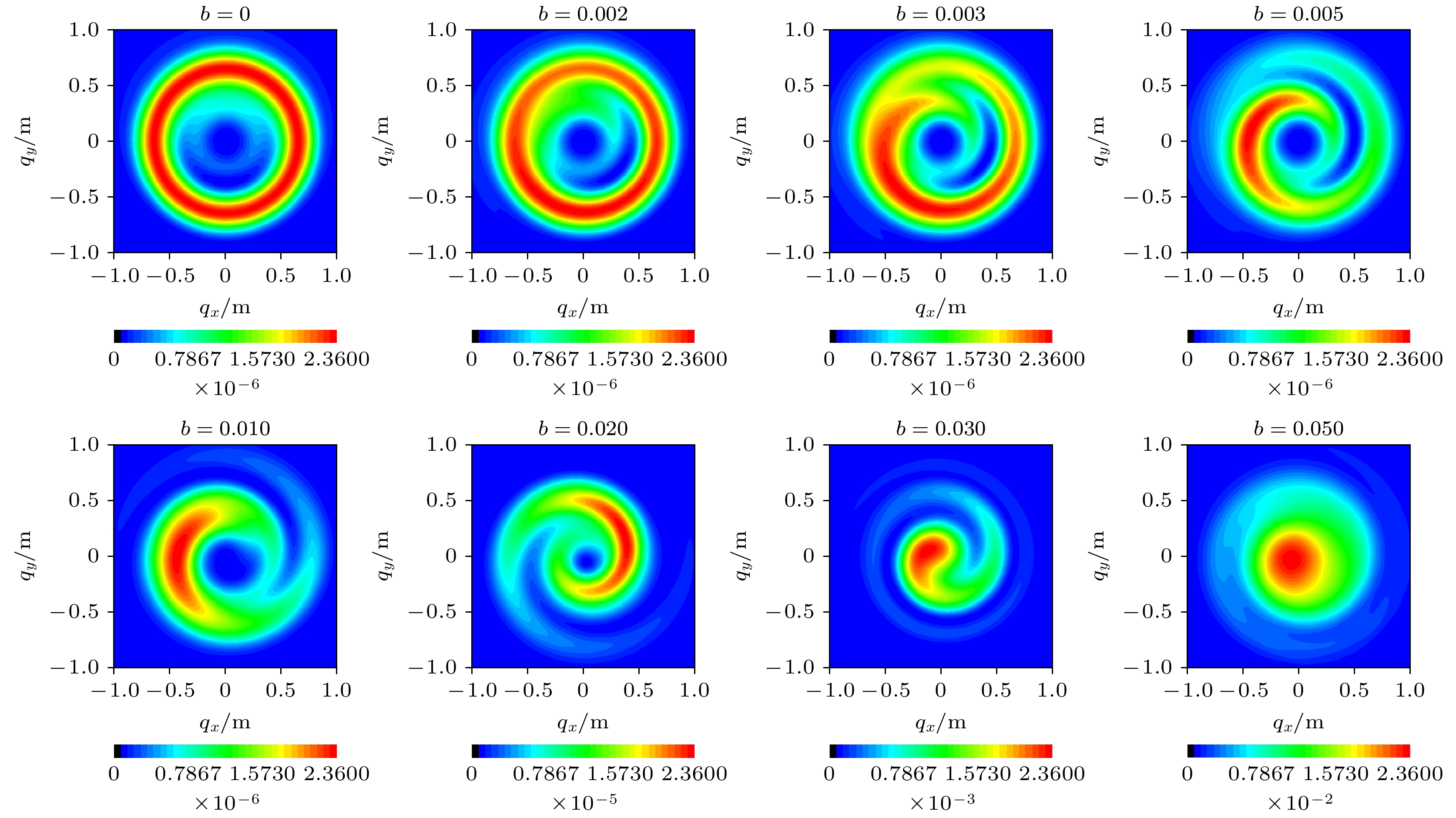
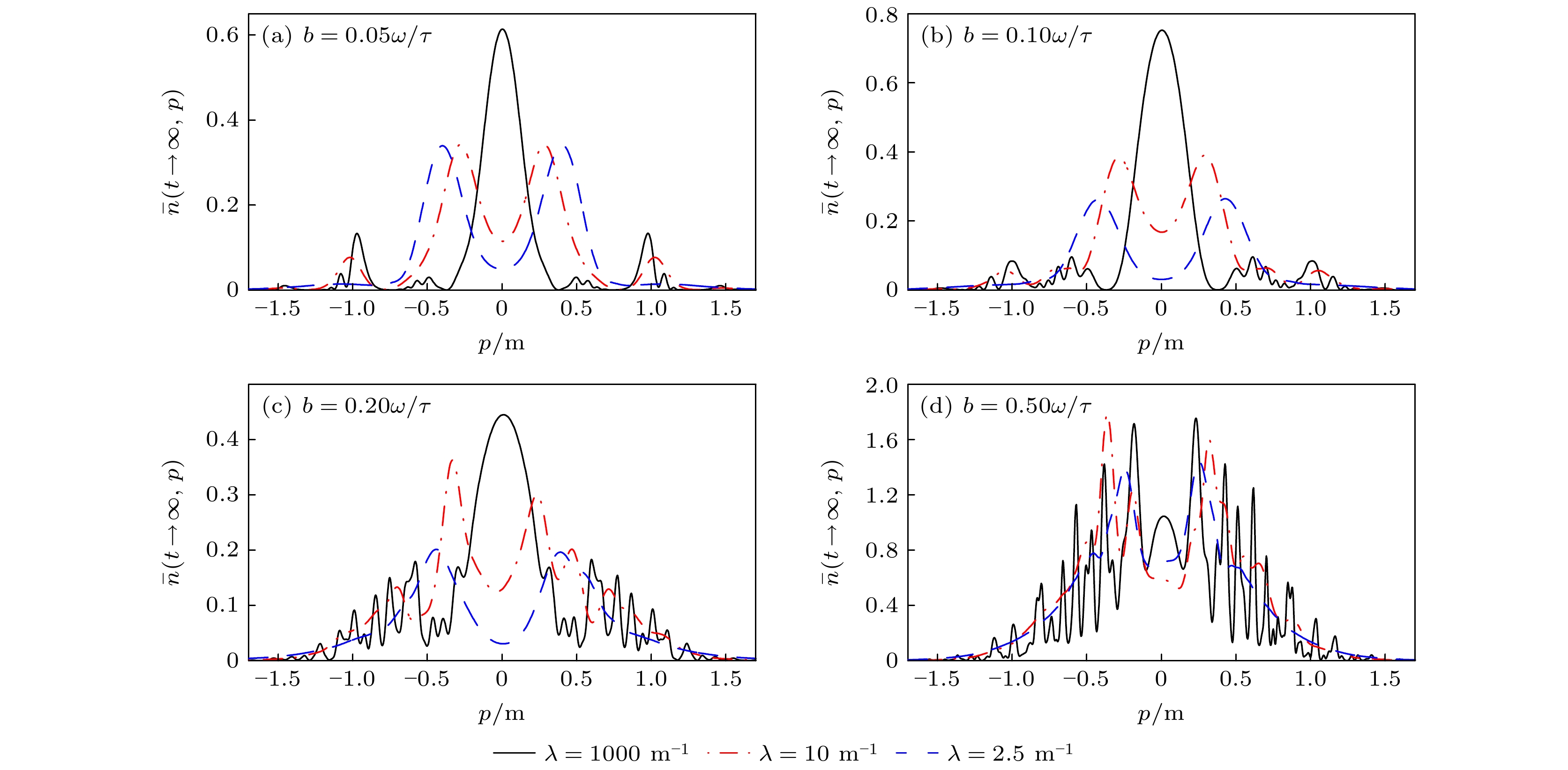
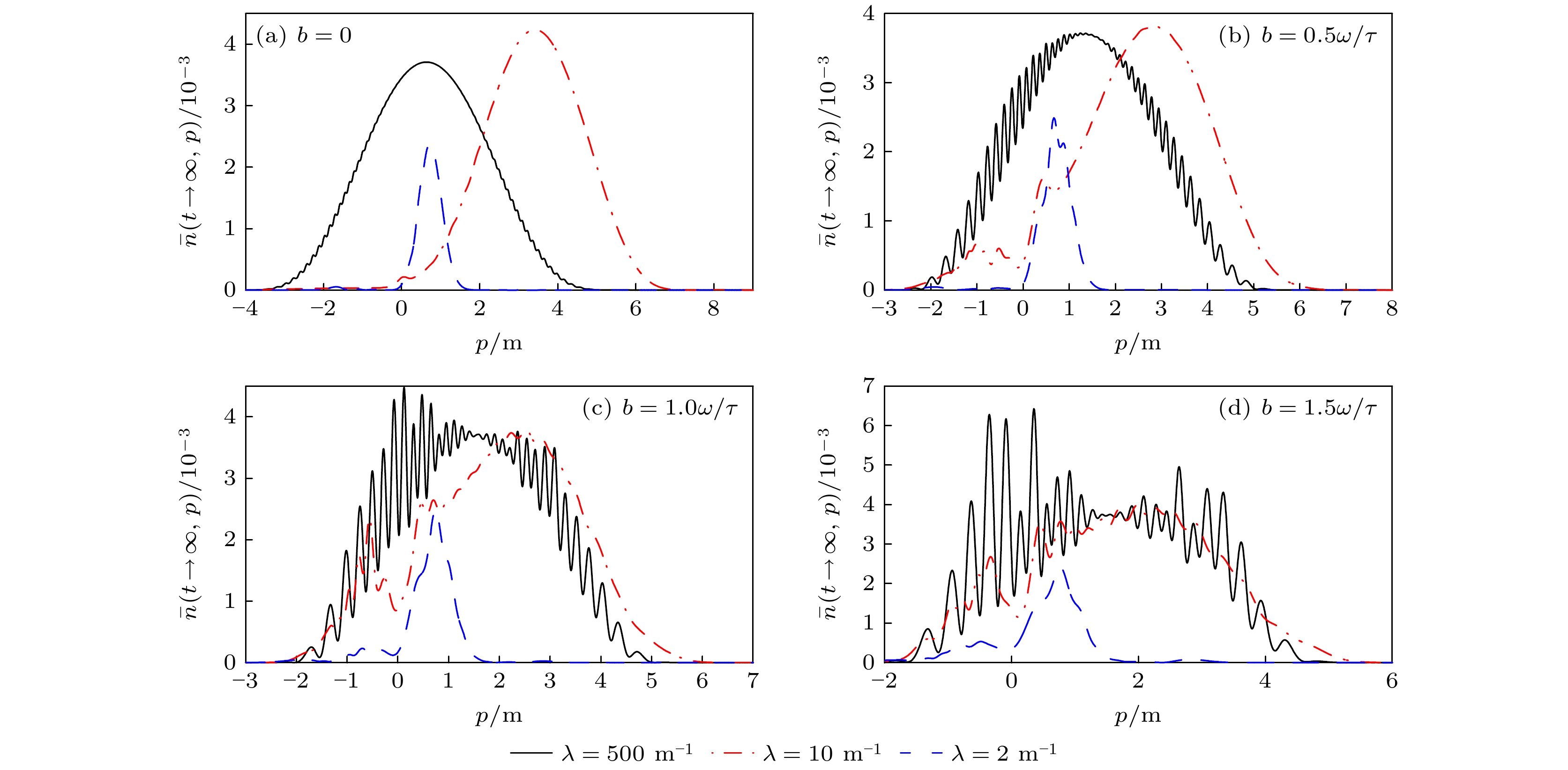
In this review article, we show an important aspect of electron-positron pair production from vacuum under strong background field where the frequency chirping plays a key role in enhancing the pair production. A series of researches on the enhancement effect of frequency chirp on electron-positron pair production in strong field is summarized. Three approaches are introduced, i.e. the Dirac-Heisenberg-Wigner formalism used to treat the spatial inhomogeneous field or/and multidimensional homogeneous time-dependent field, quantum Vlasov equation to cope with the one-dimensional homogeneous time-dependent field, and the computation quantum field theory employed to study the problem with external potential. Some interesting results about the momentum spectrum structure of created particle and the yielding of pair numbers are demonstrated for various different field parameters such as field strength and central frequency, in particular their significant influence on results when the frequency chirping form or/and strength are changed. In general, the number density can be improved by 2-3 orders of magnitude with the strengthening of frequency chirping in comparison with that without chirping for low frequency field, which is attributed to the effect that the dynamically assisted mechanism plays a significant role since the chirping expands the frequency spectrum of field. For high frequency field, however, this effect is suppressed so that the number density is enhanced by about a few times. For spatially inhomogeneous field, field changing on a small scale does not make the number density so high and the frequency chirping can enhance the yield in the order of magnitude, while the field changing on a large scale makes the number density to approach to that of homogeneous field and the chirping increases the yield by a few times. These numerical results can be understood by the Wentzel-Kramer-Brillouin (WKB) approximation and the structure of turning points. Finally the possible applicable prospects of the electron-positron pair production by the frequency chirping are presented briefly.

Keywords:

Authors and contacts

1.
College of Nuclear Science and Technology, Beijing Normal University, Beijing 100875, China
2.
Institute of Radiation Technology, Beijing Academy of Science and Technology, Beijing 100875, China

Corresponding author: Xie Bai-Song, bsxie@bnu.edu.cn ; Wang Li, wangli@brc.ac.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11875007, 11935008)

FullText HTML : translate this paragraph

References

[1]	Dirac P A M 1928 Proc. R. Soc. A 118 351
[2]	Anderson C D 1933 Phys. Rev. 43 491 Google Scholar
[3]	Sauter F 1931 Z. Phys. 69 742 Google Scholar
[4]	Heisenberg W, Euler H 1936 Z. Phys. 98 714 Google Scholar
[5]	Schwinger J 1951 Phys. Rev. 82 664 Google Scholar
[6]	Ritus V I 1985 J. Sov. Laser Res. 6 497 Google Scholar
[7]	Piazza A D 2011 Rev. Mod. Phys. 84 1177
[8]	Gelis F, Tanji N 2016 Prog. Part. Nucl. Phys. 87 1 Google Scholar
[9]	Strickland D, Mourou G 1985 Opt. Commun. 55 447 Google Scholar
[10]	Ringwald A 2001 Phys. Lett. B 510 107 Google Scholar
[11]	Yanovsky V P, Chvykov V, Kalinchenko G, Rousseau P, Krushelnick K 2008 Opt. Express 16 2109 Google Scholar
[12]	Heinzl T, Ilderton A 2009 Eur. Phys. J. D 55 359 Google Scholar
[13]	Marklund M, Lundin J 2009 Eur. Phys. J. D 55 319 Google Scholar
[14]	Dunne G V 2009 Eur. Phys. J. D 55 327 Google Scholar
[15]	Pike O J, Mackenroth F, Hill E G, Rose S J 2014 Nat. Photonics 8 434 Google Scholar
[16]	https://www.eli -beams .eu/. [2022-1-21]
[17]	Schuetzhold R, Gies H, Dunne G 2008 Phys. Rev. Lett. 101 130404 Google Scholar
[18]	Bell A R, Kirk J G 2008 Phys. Rev. Lett. 101 2952
[19]	Dunne G V, Gies H, Schützhold R 2009 Phys. Rev. D 80 111301 Google Scholar
[20]	Piazza A D, E Lötstedt, Milstein A I, Keitel C H 2009 Phys. Rev. Lett. 103 170403 Google Scholar
[21]	Bulanov S S, Mur V D, Narozhny N B, Nees J, Popov V S 2010 Phys. Rev. Lett. 104 220404 Google Scholar
[22]	https://xcels.iapras .ru /news .html[2022-1-21]
[23]	http://www.hibef.eu[2022-1-21]
[24]	Nikishov A I 1969 Sov. Phys. JETP 30 660
[25]	Brezin E, Itzykson C 1970 Phys. Rev. D 2 1191 Google Scholar
[26]	Marinov M S, Popov V S 1977 Fortschr. Phys. 25 373 Google Scholar
[27]	Nikishov A I 1985 J. Sov. Laser Res. 6 619 Google Scholar
[28]	Gies H, Klingmüller K 2005 Phys. Rev. D 72 065001
[29]	Dunne G, Wang Q H, Gies H, Schubert C 2006 Phys. Rev. D 73 065028
[30]	Xie B S, Melike M, Sayipjamal D 2012 Chin. Phys. Lett. 29 021102 Google Scholar
[31]	Schneider C, Schützhold R 2016 J. High Energy Phys. 02 164 Google Scholar
[32]	Kluger Y, Eisenberg J M, Svetitsky B, Cooper F, Mottola E 1991 Phys. Rev. Lett. 67 2427 Google Scholar
[33]	Alkofer R, Hecht M B, Roberts C D, Schmidt S M, Vinnik D V 2001 Phys. Rev. Lett. 87 193902 Google Scholar
[34]	Abdukerim N, Li Z L, Xie B S 2017 Chin. Phys. B 26 020301 Google Scholar
[35]	Krekora P, Cooley K, Su Q, Grobe R 2005 Phys. Rev. Lett. 95 070403 Google Scholar
[36]	Lv Q Z, Liu Y, Li Y J, Grobe R, Su Q 2013 Phys. Rev. Lett. 111 183204 Google Scholar
[37]	Wang L, Wu B B, Xie B S 2019 Phys. Rev. A 100 022127 Google Scholar
[38]	Hebenstreit F, Alkofer R, Gies H 2011 Phys. Rev. Lett. 107 180403 Google Scholar
[39]	Kohlfürst C 2015 Ph. D. Dissertation arXiv: 1512.06082
[40]	Xie B S, Li Z L, Tang S 2017 Matter Radiat. Extremes 2 225 Google Scholar
[41]	Kohlfürst C, Alkofer R 2018 Phys. Rev. D 97 036026 Google Scholar
[42]	Ababekri M, Dulat S, Xie B S, Zhang J 2020 Phys. Lett. B 810 135815 Google Scholar
[43]	Kohlfürst C 2020 Phys. Rev. D 101 096003 Google Scholar
[44]	Hebenstreit F, Alkofer R, Dunne G V, Gies H 2009 Phys. Rev. Lett. 102 150404 Google Scholar
[45]	Dumlu C K 2010 Phys. Rev. D 82 045007 Google Scholar
[46]	Olugh O, Li Z L, Xie B S, Alkofer R 2019 Phys. Rev. D 99 036003 Google Scholar
[47]	Orthaber M, Hebenstreit F, Alkofer R 2011 Phys. Lett. B 698 80 Google Scholar
[48]	Ababekri M, Xie B S, Zhang J 2019 Phys. Rev. D 100 016003 Google Scholar
[49]	Olugh O, Li Z L, Xie B S 2020 Phys. Lett. B 802 135259 Google Scholar
[50]	Gong C, Li Z L, Xie B S, Li Y J 2020 Phys. Rev. D 101 016008 Google Scholar
[51]	Wang K, Hu X H, Dulat S, Xie B S 2021 Chin. Phys. B 30 060204 Google Scholar
[52]	Mohamedsedik M, Li L J, Xie B S 2021 Phys. Rev. D 104 016009 Google Scholar
[53]	Li L J, Mohamedsedik M, Xie B S 2021 Phys. Rev. D 104 036015 Google Scholar
[54]	Li Z L, Lu D, Xie B S 2015 Phys. Rev. D 92 085001 Google Scholar
[55]	Li Z L, Li Y J, Xie B S 2017 Phys. Rev. D 96 076010 Google Scholar
[56]	Li Z L, Xie B S, Li Y J 2019 J. Phys. B:At. Mol. Opt. Phys. 52 025601 Google Scholar
[57]	李子良, 努尔曼古丽·阿卜杜克热木, 谢柏松 2016 物理学进展 36 129 Li Z L, Abdukerim Nuriman, Xie B S 2016 Progress in Phys. 36 129
[58]	谢柏松, 李子良, 唐琐, 刘杰 2017 物理 46 713 Google Scholar Xie B S, Li Z L, Tang S, Liu J 2017 Physics 46 713 Google Scholar
[59]	Keldysh L V 1965 Sov. Phys. JETP 20 1307
[60]	Bialynicki-Birula I, Gornicki P, Rafelski J 1991 Phys. Rev. D 44 1825
[61]	Hebenstreit F 2011 Ph. D. Dissertation arXiv: 1106.5965
[62]	Greiner W 2000 (3 rd Ed. ) (Berlin: Springer)
[63]	Schmidt S M, Blaschke D, Röpke, G, Prozorkevich A V, Smolyansky S A, Toneev V D 1999 Phys. Rev. D. 59 094005 Google Scholar
[64]	Wang L, Li L J, Mohamedsedik M, An R, Li J J, Xie B S, Zhang F S 2021 arXiv: 2109.05399
[65]	Kamiński J Z, Twardy M, Krajewska K 2018 Phys. Rev. D 98 056009 Google Scholar

Cited By

图 1 一色脉冲激光场下正负电子对的数密度与原初频率的依赖关系(取自参考文献[34])

Figure 1. Electron-positron number density vs the original frequency in one-color pulse laser field (from Ref. [34]) .

DownLoad: Full-Size Img PowerPoint

图 2 在不同啁啾参数下产生的粒子数密度与场极化度的依赖关系(取自参考文献[46])

Figure 2. The number density of created particles as a function of the field polarization $ \delta $ for different chirp parameters b (from Ref. [46]).

DownLoad: Full-Size Img PowerPoint

图 3 圆极化时在不同啁啾参数下产生的粒子的动量谱(取自参考文献[46])

Figure 3. Momentum spectra of produced pairs for circular polarization (from Ref. [46]).

DownLoad: Full-Size Img PowerPoint

图 4 在调制幅度$ b=1.0 $下不同啁啾调制频率$ {\omega }_{\mathrm{m}}=0, \mathrm{ }0.07, \mathrm{ }0.1 $时产生的粒子对的动量谱(取自参考文献[50])

Figure 4. Momentum spectra of produced pairs in the field Eq.(39) with $ {\omega }_{\mathrm{m}}=0, \mathrm{ }\mathrm{ }\mathrm{ }\mathrm{ }0.07, \mathrm{ }0.1 $ for (a)–(c), respectively when $ b=1.0 $ is fixed (from Ref. [50]).

DownLoad: Full-Size Img PowerPoint

图 5 高频$ \omega =0.7 $下取不同啁啾参数$ b $时产生的粒子对的约化动量谱(取自参考文献[42])

Figure 5. Reduced momentum spectra of produced pairs in the field Eq.(40) with $ b=0.00078 $ for (a) and $ b=0.0016 $ for (b), respectively, when high frequency $ \omega =0.7 $ is fixed (from Ref. [42]).

DownLoad: Full-Size Img PowerPoint

图 6 $ \omega =0.7 $下粒子对的约化数密度与场的空间尺度$ \lambda $的关系(取自参考文献[42])

Figure 6. Reduced number of produced pairs in the field Eq.(40) with respect to spatial extent $ \lambda $ when $ \omega =0.7 $ (from Ref. [42]).

DownLoad: Full-Size Img PowerPoint

图 7 高频$ \omega =0.7 $下不同啁啾不同空间尺度时产生的粒子对约化动量谱(取自参考文献[52])

Figure 7. Reduced momentum spectra of produced pairs in the field Eq.(41) for high frequency field with $ \omega =0.7 $ (from Ref. [52]) .

DownLoad: Full-Size Img PowerPoint

图 8 $ \omega =0.7 $下粒子对的约化数密度与场的空间尺度$ \lambda $的关系(取自参考文献[52])

Figure 8. Reduced number of produced pairs in the field Eq.(41) with respect to spatial extent $ \lambda $ when $ \omega =0.7 $ (from Ref. [52]).

DownLoad: Full-Size Img PowerPoint

图 9 低频$ \omega =0.1 $下不同啁啾不同空间尺度时产生的粒子对约化动量谱(取自参考文献[52])

Figure 9. Reduced momentum spectra of produced pairs in the field Eq.(41) for low frequency field with $ \omega =0.1 $ (from Ref. [52]).

DownLoad: Full-Size Img PowerPoint

图 10 不同啁啾不同空间尺度时产生的粒子对约化动量谱(取自参考文献[53])

Figure 10. Reduced momentum spectra of produced pairs in the field Eq.(42) for different chirping (from Ref. [53]).

DownLoad: Full-Size Img PowerPoint

图 11 粒子对约化数密度的增强因子与场的空间尺度$ \lambda $的关系(取自参考文献[53])

Figure 11. Enhancement factor of reduced number of produced pairs in the field Eq.(42) with respect to spatial extent $ \lambda $ (from Ref. [53]) .

DownLoad: Full-Size Img PowerPoint

图 12 产生的粒子对的数目与啁啾参数的依赖关系(取自参考文献[64])

Figure 12. Number of produced pairs in the field Eq. (43) with respect to chirping parameter (from Ref. [64]).

DownLoad: Full-Size Img PowerPoint

图 13 电子数目随着基频和啁啾参数变化的等高线图(取自参考文献[64])

Figure 13. The contour plot of the electron number varying with the fundamental frequency and the chirp parameter (from Ref. [64]).

DownLoad: Full-Size Img PowerPoint

图 14 不同的调制频率参数下频率调制的势阱的频谱(取自参考文献[64])

Figure 14. The frequency spectrum of frequency modulated potential well with different modulation parameters (from Ref. [64]).

DownLoad: Full-Size Img PowerPoint

图 15 固定频率(黑色虚线)和啁啾频率(红色实线)下产生电子的能谱(取自参考文献[64])

Figure 15. Energy spectrum of created electrons under the fixed frequency (the black dotted curve) and the chirp frequency (the red solid curve) (from Ref. [64]).

DownLoad: Full-Size Img PowerPoint

图 16 动量为0时的时间复平面上的转变点(取自参考文献[53])

Figure 16. The turning points of complex time-plane when the momentum is zero (from Ref. [53]).

DownLoad: Full-Size Img PowerPoint

表 1 空间尺度$ \lambda =10 $时不同啁啾下(31)式对称啁啾场与(30)式的非对称啁啾场数密度及其比值(参看文献[52])

Table 1. Reduced pair number and ratio of symmetric and asymmetric fields for different chirping when$ \lambda =10 $ (from Ref. [52]) .

b($ \omega /\tau ) $	$ {N}_{\mathrm{s}\mathrm{y}\mathrm{m}} $	$ {N}_{\mathrm{a}\mathrm{s}\mathrm{y}\mathrm{m}} $	$ {N}_{\mathrm{s}\mathrm{y}\mathrm{m}}/{N}_{\mathrm{a}\mathrm{s}\mathrm{y}\mathrm{m}} $
0.05	0.2680	0.1663	1.612
0.10	0.3274	0.1561	2.097
0.20	0.3178	0.1791	1.774
0.50	1.4340	0.7474	1.919

DownLoad: CSV

表 2 优化空间尺度和啁啾参数下单个啁啾场或两个啁啾场的增强因子(参看文献[53])

Table 2. Optimal spatial scales related to the optimal enhancement factor at the chosen optimal chirp parameters (from Ref. [53]).

Different chirping	Chirp parameter ($ {\omega }_{i}/\tau $), ($ i=\mathrm{1, 2} $)	Spatial scale/m^–1	$\displaystyle\frac{N_{\rm 1s+2w} } {N_{\rm 1s}+ N_{\rm 2w}}$
Only $ {b}_{1} $	$ {b}_{1}=0.9 $	$ \lambda =2.4 $	94.756
Only $ {b}_{2} $	$ {b}_{2}=0.3 $	$ \lambda =2.4 $	133.584
$ {b}_{1} $ and $ {b}_{2} $	$ {b}_{1}={b}_{2}=0.3 $	$ \lambda =2.4 $	132.517

DownLoad: CSV

表 3 不同的基频下产生的电子数目的最大值和最小值以及二者之间的比值(参看文献[64])

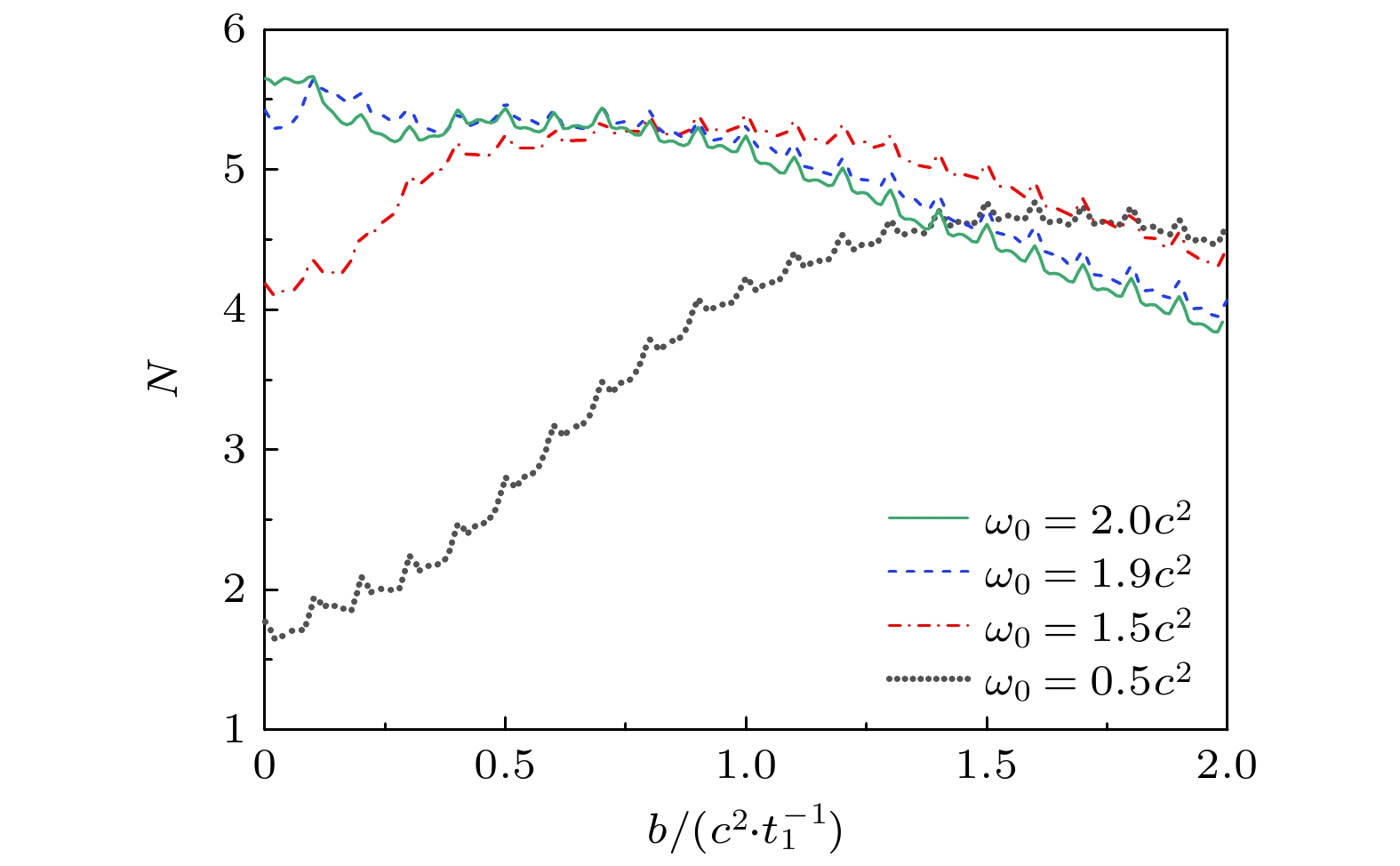
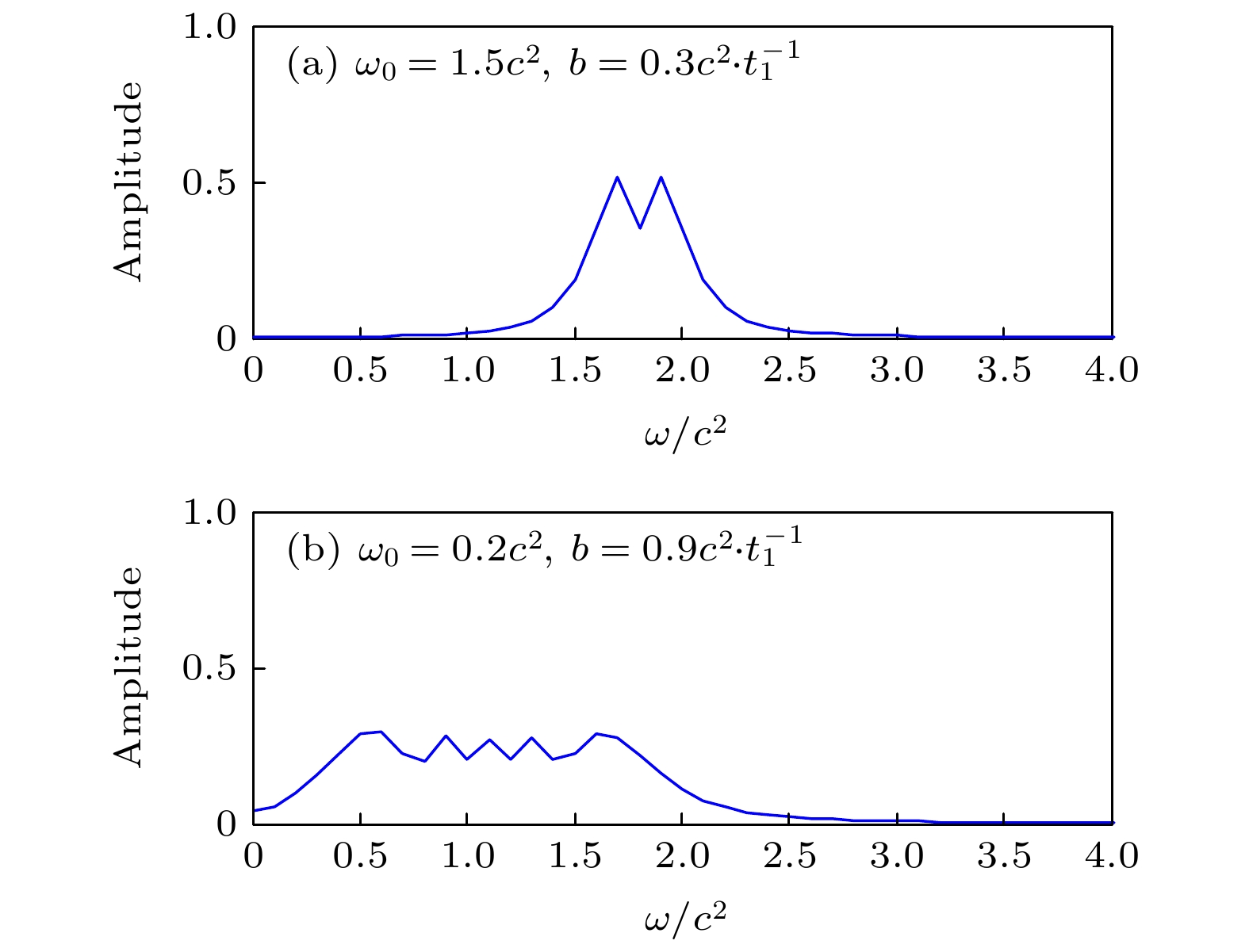
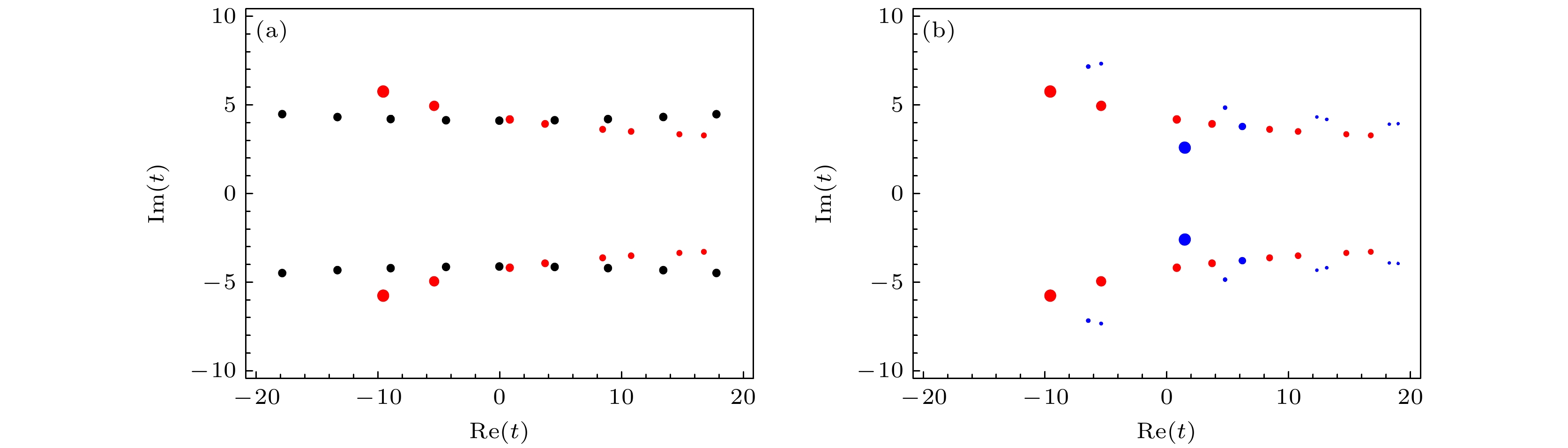
Table 3. The maximum, the minimum number of created electrons and the ratio between them for different fundamental frequencies (from Ref. [64]).

$ {\omega }_{0}/{c}^{2} $	$ {N}_{\mathrm{m}\mathrm{i}\mathrm{n}}(b=0) $	$ {N}_{\mathrm{m}\mathrm{a}\mathrm{x}} $	$ R({N}_{\mathrm{m}\mathrm{a}\mathrm{x}}/{N}_{\mathrm{m}\mathrm{i}\mathrm{n}}) $
0.1	1.87	4.58 ($ b=1.8{c}^{2}/{t}_{1} $)	2.45
0.2	1.85	4.69 ($ b=1.7{c}^{2}/{t}_{1} $)	2.54
0.5	1.77	4.76 ($ b=1.6{c}^{2}/{t}_{1} $)	2.69
1.0	2.30	5.13 ($ b=1.2{c}^{2}/{t}_{1} $)	2.23
1.5	4.18	5.39 ($ b=0.8{c}^{2}/{t}_{1} $)	1.29
1.9	5.42	5.65 ($ b=0.1{c}^{2}/{t}_{1} $)	1.04

DownLoad: CSV

map

[1]	Dirac P A M 1928 Proc. R. Soc. A 118 351
[2]	Anderson C D 1933 Phys. Rev. 43 491 Google Scholar
[3]	Sauter F 1931 Z. Phys. 69 742 Google Scholar
[4]	Heisenberg W, Euler H 1936 Z. Phys. 98 714 Google Scholar
[5]	Schwinger J 1951 Phys. Rev. 82 664 Google Scholar
[6]	Ritus V I 1985 J. Sov. Laser Res. 6 497 Google Scholar
[7]	Piazza A D 2011 Rev. Mod. Phys. 84 1177
[8]	Gelis F, Tanji N 2016 Prog. Part. Nucl. Phys. 87 1 Google Scholar
[9]	Strickland D, Mourou G 1985 Opt. Commun. 55 447 Google Scholar
[10]	Ringwald A 2001 Phys. Lett. B 510 107 Google Scholar
[11]	Yanovsky V P, Chvykov V, Kalinchenko G, Rousseau P, Krushelnick K 2008 Opt. Express 16 2109 Google Scholar
[12]	Heinzl T, Ilderton A 2009 Eur. Phys. J. D 55 359 Google Scholar
[13]	Marklund M, Lundin J 2009 Eur. Phys. J. D 55 319 Google Scholar
[14]	Dunne G V 2009 Eur. Phys. J. D 55 327 Google Scholar
[15]	Pike O J, Mackenroth F, Hill E G, Rose S J 2014 Nat. Photonics 8 434 Google Scholar
[16]	https://www.eli -beams .eu/. [2022-1-21]
[17]	Schuetzhold R, Gies H, Dunne G 2008 Phys. Rev. Lett. 101 130404 Google Scholar
[18]	Bell A R, Kirk J G 2008 Phys. Rev. Lett. 101 2952
[19]	Dunne G V, Gies H, Schützhold R 2009 Phys. Rev. D 80 111301 Google Scholar
[20]	Piazza A D, E Lötstedt, Milstein A I, Keitel C H 2009 Phys. Rev. Lett. 103 170403 Google Scholar
[21]	Bulanov S S, Mur V D, Narozhny N B, Nees J, Popov V S 2010 Phys. Rev. Lett. 104 220404 Google Scholar
[22]	https://xcels.iapras .ru /news .html[2022-1-21]
[23]	http://www.hibef.eu[2022-1-21]
[24]	Nikishov A I 1969 Sov. Phys. JETP 30 660
[25]	Brezin E, Itzykson C 1970 Phys. Rev. D 2 1191 Google Scholar
[26]	Marinov M S, Popov V S 1977 Fortschr. Phys. 25 373 Google Scholar
[27]	Nikishov A I 1985 J. Sov. Laser Res. 6 619 Google Scholar
[28]	Gies H, Klingmüller K 2005 Phys. Rev. D 72 065001
[29]	Dunne G, Wang Q H, Gies H, Schubert C 2006 Phys. Rev. D 73 065028
[30]	Xie B S, Melike M, Sayipjamal D 2012 Chin. Phys. Lett. 29 021102 Google Scholar
[31]	Schneider C, Schützhold R 2016 J. High Energy Phys. 02 164 Google Scholar
[32]	Kluger Y, Eisenberg J M, Svetitsky B, Cooper F, Mottola E 1991 Phys. Rev. Lett. 67 2427 Google Scholar
[33]	Alkofer R, Hecht M B, Roberts C D, Schmidt S M, Vinnik D V 2001 Phys. Rev. Lett. 87 193902 Google Scholar
[34]	Abdukerim N, Li Z L, Xie B S 2017 Chin. Phys. B 26 020301 Google Scholar
[35]	Krekora P, Cooley K, Su Q, Grobe R 2005 Phys. Rev. Lett. 95 070403 Google Scholar
[36]	Lv Q Z, Liu Y, Li Y J, Grobe R, Su Q 2013 Phys. Rev. Lett. 111 183204 Google Scholar
[37]	Wang L, Wu B B, Xie B S 2019 Phys. Rev. A 100 022127 Google Scholar
[38]	Hebenstreit F, Alkofer R, Gies H 2011 Phys. Rev. Lett. 107 180403 Google Scholar
[39]	Kohlfürst C 2015 Ph. D. Dissertation arXiv: 1512.06082
[40]	Xie B S, Li Z L, Tang S 2017 Matter Radiat. Extremes 2 225 Google Scholar
[41]	Kohlfürst C, Alkofer R 2018 Phys. Rev. D 97 036026 Google Scholar
[42]	Ababekri M, Dulat S, Xie B S, Zhang J 2020 Phys. Lett. B 810 135815 Google Scholar
[43]	Kohlfürst C 2020 Phys. Rev. D 101 096003 Google Scholar
[44]	Hebenstreit F, Alkofer R, Dunne G V, Gies H 2009 Phys. Rev. Lett. 102 150404 Google Scholar
[45]	Dumlu C K 2010 Phys. Rev. D 82 045007 Google Scholar
[46]	Olugh O, Li Z L, Xie B S, Alkofer R 2019 Phys. Rev. D 99 036003 Google Scholar
[47]	Orthaber M, Hebenstreit F, Alkofer R 2011 Phys. Lett. B 698 80 Google Scholar
[48]	Ababekri M, Xie B S, Zhang J 2019 Phys. Rev. D 100 016003 Google Scholar
[49]	Olugh O, Li Z L, Xie B S 2020 Phys. Lett. B 802 135259 Google Scholar
[50]	Gong C, Li Z L, Xie B S, Li Y J 2020 Phys. Rev. D 101 016008 Google Scholar
[51]	Wang K, Hu X H, Dulat S, Xie B S 2021 Chin. Phys. B 30 060204 Google Scholar
[52]	Mohamedsedik M, Li L J, Xie B S 2021 Phys. Rev. D 104 016009 Google Scholar
[53]	Li L J, Mohamedsedik M, Xie B S 2021 Phys. Rev. D 104 036015 Google Scholar
[54]	Li Z L, Lu D, Xie B S 2015 Phys. Rev. D 92 085001 Google Scholar
[55]	Li Z L, Li Y J, Xie B S 2017 Phys. Rev. D 96 076010 Google Scholar
[56]	Li Z L, Xie B S, Li Y J 2019 J. Phys. B:At. Mol. Opt. Phys. 52 025601 Google Scholar
[57]	李子良, 努尔曼古丽·阿卜杜克热木, 谢柏松 2016 物理学进展 36 129 Li Z L, Abdukerim Nuriman, Xie B S 2016 Progress in Phys. 36 129
[58]	谢柏松, 李子良, 唐琐, 刘杰 2017 物理 46 713 Google Scholar Xie B S, Li Z L, Tang S, Liu J 2017 Physics 46 713 Google Scholar
[59]	Keldysh L V 1965 Sov. Phys. JETP 20 1307
[60]	Bialynicki-Birula I, Gornicki P, Rafelski J 1991 Phys. Rev. D 44 1825
[61]	Hebenstreit F 2011 Ph. D. Dissertation arXiv: 1106.5965
[62]	Greiner W 2000 (3 rd Ed. ) (Berlin: Springer)
[63]	Schmidt S M, Blaschke D, Röpke, G, Prozorkevich A V, Smolyansky S A, Toneev V D 1999 Phys. Rev. D. 59 094005 Google Scholar
[64]	Wang L, Li L J, Mohamedsedik M, An R, Li J J, Xie B S, Zhang F S 2021 arXiv: 2109.05399
[65]	Kamiński J Z, Twardy M, Krajewska K 2018 Phys. Rev. D 98 056009 Google Scholar

[1]	Liu Qing-Kang, Zhang Xu, Cai Hong-Bo, Zhang En-Hao, Gao Yan-Qi, Zhu Shao-Ping. Suppression of stimulated Raman scattering kinetic bursts by intensity-modulated broadband laser. Acta Physica Sinica, 2024, 73(5): 055202. doi: 10.7498/aps.73.20231679
[2]	Li Chuan-Ke, Lin Nan-Sheng, Zhou Xian-Xian, Jiang Miao, Li Ying-Jun. Theoretical study of double oscillating fields induced electron-positron pairs creation process. Acta Physica Sinica, 2024, 73(4): 044201. doi: 10.7498/aps.73.20230432
[3]	Zhou Li-Na, Hu Han-Qing, Liu Yue-Qiang, Duan Ping, Chen Long, Zhang Han-Yu. Modelling study of fluid and kinetic responses of plasmas to resonant magnetic perturbation. Acta Physica Sinica, 2023, 72(7): 075202. doi: 10.7498/aps.72.20222196
[4]	Mou Jia-Lian, Lü Jun-Guang, Sun Xi-Lei, Lan Xiao-Fei, Huang Yong-Sheng. Time of flight detector for charged particle identification based on circular electron-positron collider. Acta Physica Sinica, 2023, 72(12): 122901. doi: 10.7498/aps.72.20222271
[5]	Ye Quan-Xing, He Guang-Zhao, Wang Qian. Bottominium-like states in e⁺e^– annihilation. Acta Physica Sinica, 2023, 72(20): 201401. doi: 10.7498/aps.72.20230908
[6]	Luo Hui-Yi, Jiang Miao, Xu Miao-Hua, Li Ying-Jun. Electron-position pair creation under combined oscillation fields with different frequencies. Acta Physica Sinica, 2023, 72(2): 021201. doi: 10.7498/aps.72.20221660
[7]	Sun Ting, Wang Yu, Guo Ren-Tong, Lu Zhi-Wei, Li Jian-Xing. Review on laser-driven high-energy polarized electron and positron beams and γ-rays. Acta Physica Sinica, 2021, 70(8): 087901. doi: 10.7498/aps.70.20210009
[8]	Jiang Miao, Zheng Xiao-Ran, Lin Nan-Sheng, Li Ying-Jun. Multi-photon transition effects under different external field widths in electron-positron-pair creation process. Acta Physica Sinica, 2021, 70(23): 231202. doi: 10.7498/aps.70.20202101
[9]	Zhu Xing-Long, Wang Wei-Min, Yu Tong-Pu, He Feng, Chen Min, Weng Su-Ming, Chen Li-Ming, Li Yu-Tong, Sheng Zheng-Ming, Zhang Jie. Research progress of ultrabright γ-ray radiation and electron-positron pair production driven by extremely intense laser fields. Acta Physica Sinica, 2021, 70(8): 085202. doi: 10.7498/aps.70.20202224
[10]	Li Ang, Yu Jin-Qing, Chen Yu-Qing, Yan Xue-Qing. Numerical method of electron-positron pairs generation in photon-photon collider. Acta Physica Sinica, 2020, 69(1): 019501. doi: 10.7498/aps.69.20190729
[11]	Wu Guang-Zhi, Wang Qiang, Zhou Cang-Tao, Fu Li-Bin. Positron wave interference and Klein tunnel during the production of pairs in the double-well potential. Acta Physica Sinica, 2017, 66(7): 070301. doi: 10.7498/aps.66.070301
[12]	Lin Cheng, Zhang Hua-Tang, Sheng Zhi-Hao, Yu Xian-Huan, Liu Peng, Xu Jing-Wen, Song Xiao-Hong, Hu Shi-Lin, Chen Jing, Yang Wei-Feng. Strong field photoelectron holography studied by a generalized quantum-trajectory Monte Carlo method. Acta Physica Sinica, 2016, 65(22): 223207. doi: 10.7498/aps.65.223207
[13]	Xin Guo-Guo, Zhao Qing, Liu Jie. Maximum correlation at the transition to the saturation regime of nonsequential double ionization. Acta Physica Sinica, 2012, 61(13): 133201. doi: 10.7498/aps.61.133201
[14]	Ye Di-Fa, Liu Jie, Xin Guo-Guo, Zhao Qing. The role of multi-return induced collision-ionization in atomic nonsequential double ionization. Acta Physica Sinica, 2011, 60(9): 093204. doi: 10.7498/aps.60.093204
[15]	Zeng Si-Liang, Zou Shi-Yang, Wang Jian-Guo, Yan Jun. Numerical solution of the three-dimensional time-dependent Schr?dinger equation and its application. Acta Physica Sinica, 2009, 58(12): 8180-8187. doi: 10.7498/aps.58.8180
[16]	Zhang Hong-Ying, Chen De-Ying, Lu Zhen-Zhong, Fan Rong-Wei, Xia Yuan-Qin. Numerical calculation of laser-induced collisional energy transfer in Ba-Sr system. Acta Physica Sinica, 2008, 57(12): 7600-7605. doi: 10.7498/aps.57.7600
[17]	Jiang Yang, Yu Jin-Long, Hu Hao, Zhang Ai-Xu, Zhang Li-Tai, Wang Wen-Rui, Yang En-Ze. The improvement of fiber parametric amplifier pulse source via modulation of signal light. Acta Physica Sinica, 2008, 57(5): 2994-3000. doi: 10.7498/aps.57.2994
[18]	Zheng Hong-Jun, Liu Shan-Liang, Li Xin, Xu Jing-Ping. Effect of initial frequency chirp on the linear propagation characteristics of the hyperbolic secant optical pulse. Acta Physica Sinica, 2007, 56(4): 2286-2292. doi: 10.7498/aps.56.2286
[19]	ZHENG LI-PING, QIU XI-JUN. THE INFLUENCE OF THE INTENSITY AND THE FREQUENCY ON THE ENHANCED IONIZATION BEHA VIOR OF MULTIATOMIC MOLECULAR IONS IN THE INTENSE LASER FIELDS. Acta Physica Sinica, 2000, 49(10): 1965-1968. doi: 10.7498/aps.49.1965
[20]	. . Acta Physica Sinica, 1975, 24(2): 145-150. doi: 10.7498/aps.24.145

Catalog

Vol. 71, Issue 13 - 2022-07-05

Metrics

Abstract views: 4845
PDF Downloads: 103
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板