-
This study investigates the combination of two not completely overlapping oscillating fields. The aim is to analyze the effect of the separation distance between the fields on the production of electron-positron pairs in a vacuum.The process was simulated using Computational Quantum Field Theory (CQFT) methods and the split-operator technique, based on the space-time dependent Dirac equation. The primary focus was on analyzing the impact of separation distance and frequency combinations on the pair production rate and energy spectrum.The research found that partially overlapping subcritical oscillating fields can still effectively generate electron-positron pairs within a small separation distance. The variation in separation distance in the overlapping direction significantly affects the pair production rate. For two oscillating fields with a fixed sum of frequencies, the separation distance has a notable impact on the production rate, with different frequency combinations showing varying degrees of dependency.Further analysis of the energy spectrum revealed that the number and position of spectral peaks are differently affected by the separation distance. Models with smaller frequency differences exhibited more concentrated energy distributions, generally presenting a single-peak structure. In contrast, models with larger frequency differences showed more dispersed energy distributions, typically presenting a dual-peak structure. As the separation distance increases, the energy spectrum structure varies with different frequency combinations, especially for larger separation distances. In cases with larger frequency differences, the high-energy peak decreases rapidly with increasing separation distance, resulting in a lower proportion of high-energy electrons, whereas the cases with smaller frequency differences exhibit less change. This phenomenon was further analyzed using particle energy transition probability distribution diagrams.By observing the particle energy transition probability distribution diagrams, we gained preliminary insights into the differences in various frequency combinations with changes in separation distance, explaining the variations in energy spectrum structure from the perspective of multiphoton transitions.Additionally, a more detailed analysis of these diagrams based on the law of energy conservation allowed us to extract the trends in particle production corresponding to various multiphoton transition effects.It was found that for the same frequency combination, the trends of second and third-order effects with varying separation distances differ, with higher-order effects decreasing more rapidly.By analyzing the changes in multiphoton transition probabilities for the combined fields with separation distance, as well as the changes in the individual fields' multiphoton transition probabilities, we concluded that when the separation distance is small, the combined fields with larger frequency differences have an advantage in electron-positron pair production. However, when the separation distance is large, the combined fields with smaller frequency differences begin to play a major role due to their inherent multiphoton effects, demonstrating better stability. For different cases under the combined influence of two fields, we conducted a more in-depth analysis of the differences between various orders within the same frequency combination and the differences between the same order transitions under different frequency combinations. By proposing hypotheses and performing computational verification, it was found that the trend of normalized-overlapping photon numbers with varying separation distances under the same conditions is consistent with the trend of corresponding particle production numbers, providing a more convenient method for examining the trends in particle production with separation distance.This study not only enriches our understanding of vacuum electron-positron pair generation in strong fields but also provides theoretical guidance and reference for designing experimental setups for pair production.
-
图 1 组合场分开不同间距D时的场强图象, 分别为 (a)$ D = 2/c $, (b)$ D = 4/c $, (c)$ D = 6/c $, (d)$ D = 12/c $, 两场场宽均为$ W = 2/c $
Figure 1. The field strength diagrams for combined fields separated by different distances D are shown, with (a) $ D = 2/c $, (b) $ D = 4/c $, (c) $ D = 6/c $, and (d) $ D = 12/c $. The width of both fields is $ W = 2/c$.
图 2 (a)产率$ {k} $随$ {D} $的变化趋势, 频率差渐变的过程中, $ {D} $从$ 0 $到$ 10/{c} $的下降趋势. (b)归一化产率$ {k/k_{max}} $随间距$ {D} $的变化趋势
Figure 2. (a) The trend of the electron pair production rate $ {k} $ as the frequency difference gradually changes, showing a decreasing trend of $ {D} $ from $ 0 $ to $ 10/{c} $. (b) The trend of normalized electron pair production rate $ {k/k_{max}} $ with respect to the change in distance $ {D} $.
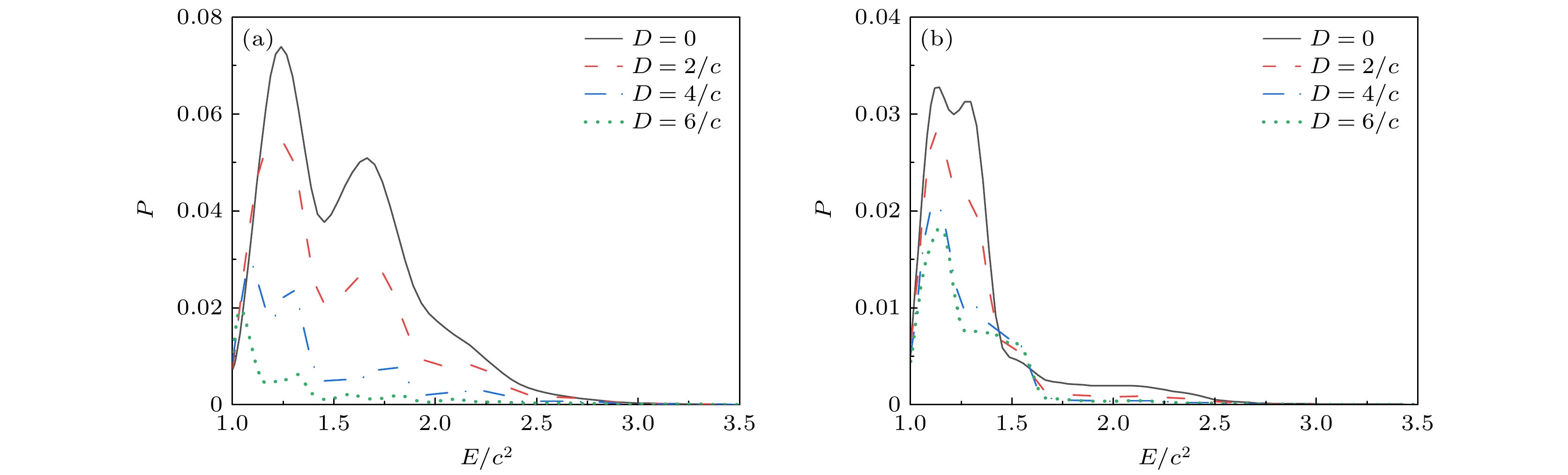
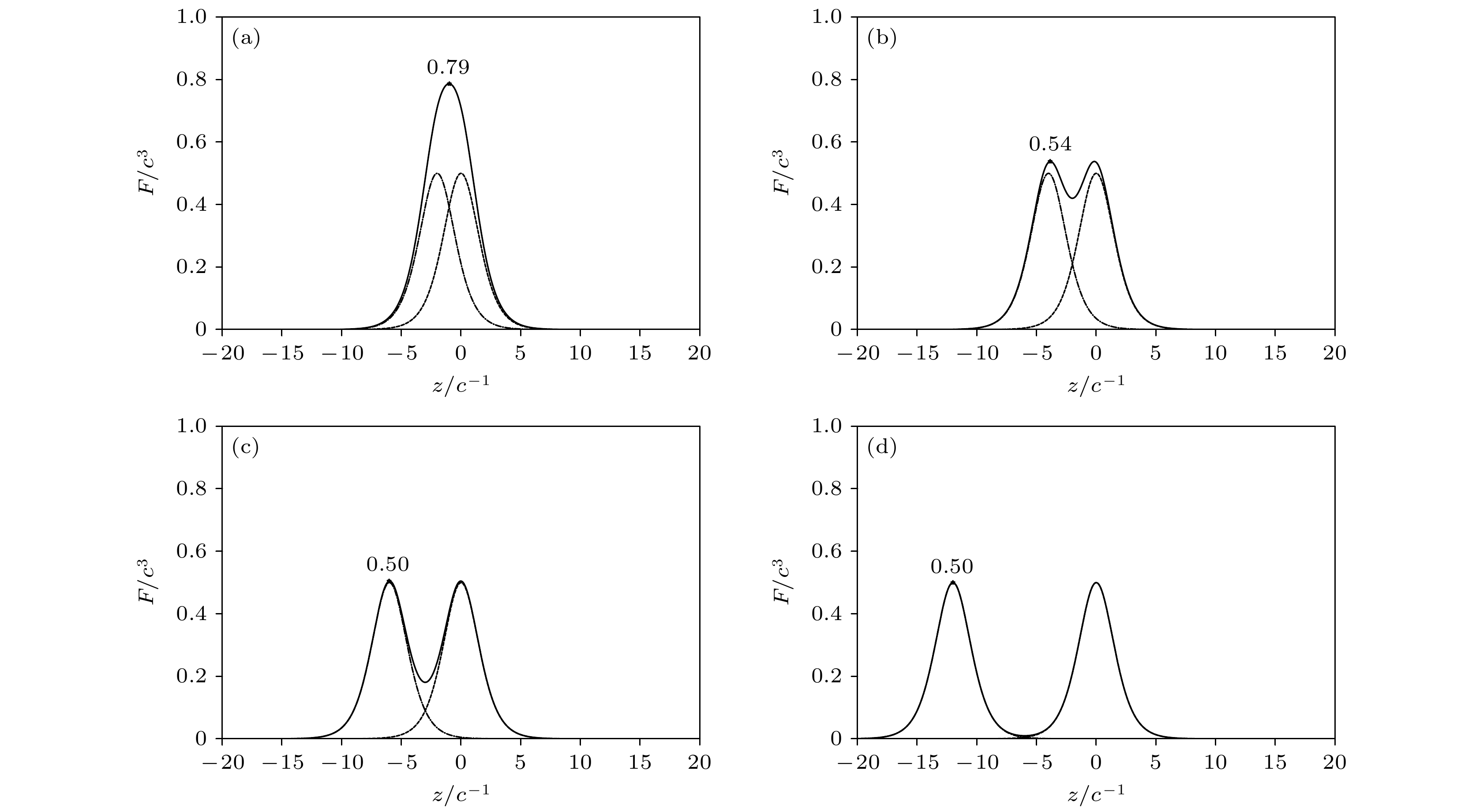
图 3 $ {\rm{(a)}}\;\omega_1 = 0.5{c}^2, \omega_2 = 1.9{c}^2 $时不同$ {D} $对应的产生粒子能量分布概率. $ ({\mathrm{b}})\;\omega_1 = 1.1{c}^2, \omega_2 = 1.3{c}^2 $时不同$ {D} $对应的产生粒子的能量分布概率. 演化时间均为$ {t} = 0.003 \ {a.u.} $势高$ {{\cal{V}}_1={\cal{V}}_2 = 2.0 c^2} $, 场宽$ {W = 2/c} $
Figure 3. $ {\rm{(a)}} $ Energy spectrum for different $ {D} $ values when $ \omega_1 = 0.5{c}^2, \omega_2 = 1.9{c}^2 $. $ {({\mathrm{b}})} $ Energy spectrum for different $ {D} $ values when $ \omega_1 = 1.1{c}^2, \omega_2 = 1.3{c}^2 $.Evolution time is $ {t} = 0.003 \ {a.u.} $ with potential heights $ {{\cal{V}}_1={\cal{V}}_2 = 2.0 c^2} $ and field width $ {W = 2/c} $
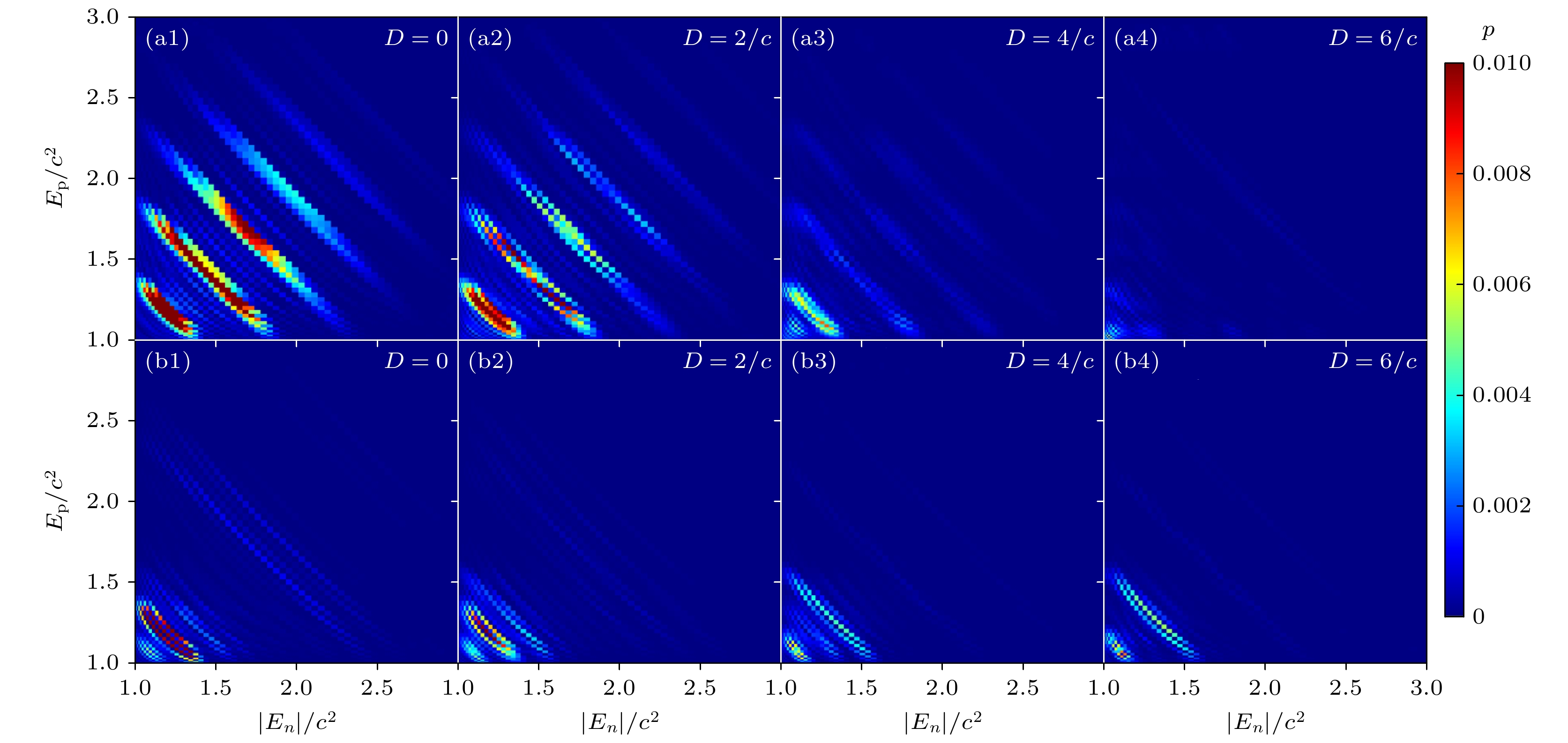
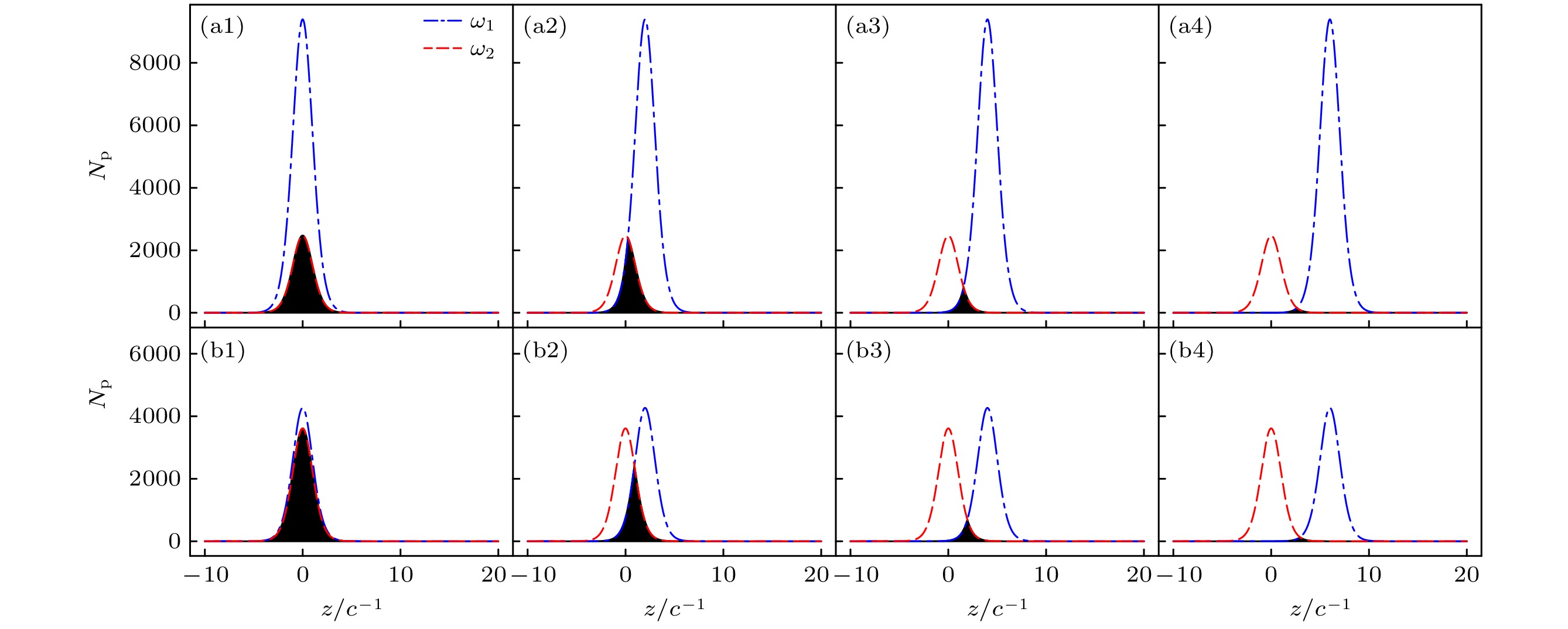
图 4 两种组合场参数下的粒子跃迁能量概率分布: (a) $ \omega_1 = 0.5\, c^2 $和$ \omega_2 = 1.9\, c^2 $, (b) $ \omega_1 = 1.1\, c^2 $和$ \omega_2 = 1.3\, c^2 $, 其中子图$ {\rm{(a_1)}}\rightarrow {\rm{(a_4)}} $和$ {\rm{(b_1)}}\rightarrow {\rm{(b_4)}} $分别对应空间参数D从$ 0 $到$ 6/c $的变化. 场宽为$ W_1 = W_2 = 2/c $, 势场高度为$ {\cal{V}}_1 = {\cal{V}}_2 = 2.0\, c^2 $
Figure 4. Particle transition energy probability distribution for combined fields with (a) $ \omega_1 = 0.5\, c^2 $ and $ \omega_2 = 1.9\, c^2 $, and (b) $ \omega_1 = 1.1\, c^2 $ and $ \omega_2 = 1.3\, c^2 $. The subfigures $ {\rm{(a_1)}}\rightarrow {\rm{(a_4)}} $ and $ {\rm{(b_1)}}\rightarrow {\rm{(b_4)}} $ correspond to the spatial parameter D varying from $ 0 $ to $ 6/c $, respectively. The field widths are $ W_1 = W_2 = 2/c $, and the potential heights are $ {\cal{V}}_1 = {\cal{V}}_2 = 2.0\, c^2 $.
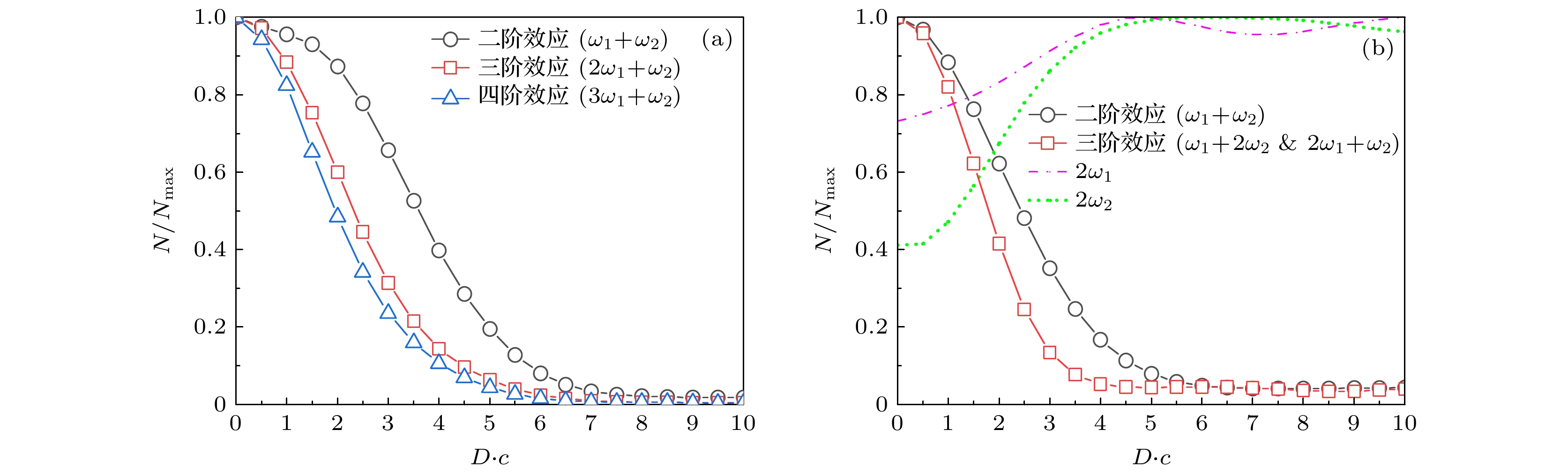
图 5 (a) $ \omega_1 = 0.5{c}^2, \omega_2 = 1.9{c}^2 $, (b) $ \omega_1 = 1.1{c}^2, \omega_2 = 1.3{c}^2 $条件下各阶多光子跃迁效应对应的归一化粒子数对比, 纵轴为归一化粒子数, 演化时长$ {(t = 0.003 \ [a.u.] )} $不同的线条样式代表不同的跃迁阶级
Figure 5. (a) $ \omega_1 = 0.5{c}^2, \omega_2 = 1.9{c}^2 $, (b) $ \omega_1 = 1.1{c}^2, \omega_2 = 1.3{c}^2 $, under these conditions, the normalized particle numbers corresponding to various order multiphoton transition effects are compared. The vertical axis represents the normalized particle numbers, and different line styles represent different transition orders, with an evolution time of $ {(t = 0.003 \ [a.u.] )} $.
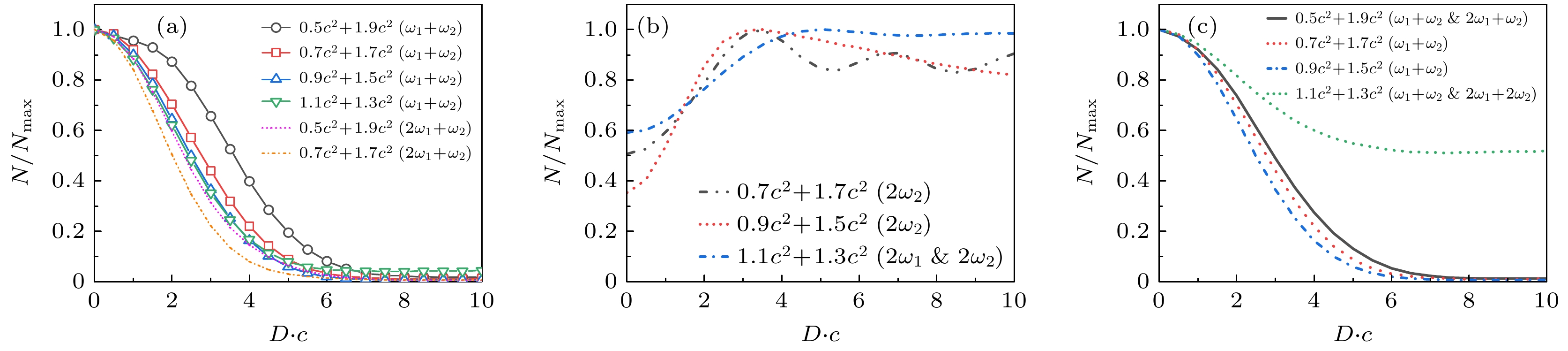
图 6 不同频率组合的低阶跃迁效应随间距变化趋势对比. (a)组合场二阶以及部分三阶跃迁效应粒子数; (b)独立场的二阶以及部分三阶跃迁效应粒子数; (c)各阶主要成分相加的粒子数比较, 且进行了归一化处理.
Figure 6. Comparison of trends in low-order transition effects with varying separation distances for different frequency combinations. (a) Particle numbers for second-order and partial third-order transition effects of combined fields; (b) Particle numbers for second-order and partial third-order transition effects of independent fields; (c) Comparison of particle numbers for the main components of each order, with normalization applied.
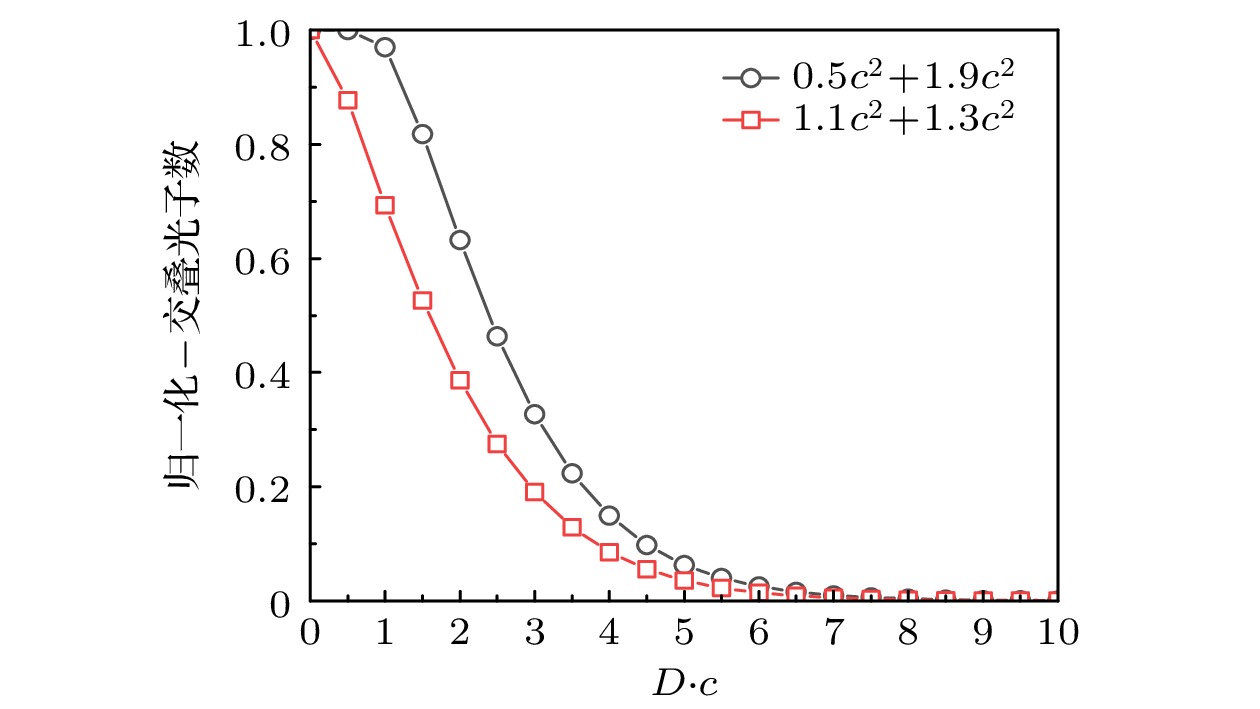
图 7 频率组合$ {\rm{(a)}} $ $ 0.5{c}^2+1.9{c}^2 $和$ {\rm{(b)}} $ $ 1.1{c}^2+1.3{c}^2 $下的交叠光子数随间距$ {D} $的变化. 子图的角标(1, 2, 3, 4)分别对应间距$ {D} $的值为$ {0} $, $ {2/c} $, $ {4/c} $, $ {6/c} $. 场宽$ W = 2/c $, 势高$ {\cal{V}} = 2 c^2 $
Figure 7. Variation of overlapping photon numbers with distance $ {D} $ under frequency combinations $ {\rm{(a)}} $ $ 0.5{c}^2+1.9{c}^2 $ and $ {\rm{(b)}} $ $ 1.1{c}^2+1.3{c}^2 $. The subscripts of the subplots (1, 2, 3, 4) correspond to distance $ {D} $ values of $ {0} $, $ {2/c} $, $ {4/c} $, and $ {6/c} $ respectively. Field width $ W = 2/c $, potential height $ {\cal{V}} = 2 c^2 $.
图 8 频率组合分别为$ 0.5{c}^2+1.9{c}^2 $与$ 1.1{c}^2+1.3{c}^2 $时的归一化-交叠光子数随间距$ {D} $变化趋势
Figure 8. The trend of the normalized overlapping photon number with the change of distance $ {D} $ when the frequency combinations are respectively $ 0.5{c}^2+1.9{c}^2 $ and $ 1.1{c}^2+1.3{c}^2 $.
-
[1] Schwinger, J. 1951 Phys. Rev. 82 664
Google Scholar
[2] Kohlfürst, C., Ahmadiniaz, N., Oertel, J., Schützhold, R. 2022 Phys. Rev. Lett. 129 241801
Google Scholar
[3] Marinov, M. S., Popov, V. S. 1977 Fortschr. Phys. 25 373
Google Scholar
[4] Brezin, E., Itzykson, C. 1970 Phys. Rev. D 2 1191
Google Scholar
[5] Popov, V. S. 1971 Zh. Eksp. Teor. Fiz. 61 1334
[6] Zhu, X. L., Wang, W. M., Yu, T. P., He, F., Chen, M., Weng, S. M., Chen, L. M., Li, Y. T., Sheng, Z. M., Zhang, J. 2021 Acta Phys. Sinica 70 085202
Google Scholar
[7] Shen, B. F., Ji, L. L., Zhang, X. M., Bu, Z. G., Xu, J. C. 2021 Acta Phys. Sinica 70 084101
Google Scholar
[8] Kluger, Y., Eisenberg, J. M., Svetitsky, B., Cooper, F., Mottola, E. 1991 Phys. Rev. Lett. 67 2427
Google Scholar
[9] Alkofer, R., Hecht, M. B., Roberts, C. D., Schmidt, S. M., Vinnik, D. V. 2001 Phys. Rev. Lett. 87 193902
Google Scholar
[10] Schmidt, S., Blaschke, D., Röpke, G., Prozorkevich, A. V., Smolyansky, S. A., Toneev, V. D. 1999 Phys. Rev. D 59 094005
Google Scholar
[11] Blinne, A., Strobel, E. 2016 Phys. Rev. D 93 025014
Google Scholar
[12] Kohlfürst, C., Alkofer, R. 2018 Phys. Rev. D 97 036026
Google Scholar
[13] Gies, H., Klingmüller, K. 2005 Phys. Rev. D 72 065001
Google Scholar
[14] Ilderton, A., Torgrimsson, G., Wårdh, J. 2015 Phys. Rev. D 92 065001
Google Scholar
[15] Dumlu, C. K., Dunne, G. V. 2011 Phys. Rev. D 83 065028
Google Scholar
[16] Dumlu, C. K. 2010 Phys. Rev. D 82 045007
Google Scholar
[17] Krekora, P., Cooley, K., Su, Q., Grobe, R. 2005 Phys. Rev. Lett. 95 070403
Google Scholar
[18] Xie, B. S., Li, Z. L., Tang, S., Liu, J. 2017 Physics 46 713
[19] Lv, Q. Z., Liu, Y., Li, Y. J., Grobe, R., Su, Q. 2013 Phys. Rev. Lett. 111 183204
Google Scholar
[20] Bulanov, S. S., Mur, V. D., Narozhny, N. B., Nees, J., Popov, V. S. 2010 Phys. Rev. Lett. 104 220404
Google Scholar
[21] Di Piazza, A., Müller, C., Hatsagortsyan, K. Z., Keitel, C. H. 2012 Rev. Mod. Phys. 84 1177
Google Scholar
[22] Luo, H. Y., Jiang, M., Xu, M. H., Li, Y. J. 2023 Acta Phys. Sinica 72 021201
Google Scholar
[23] Braun, J. W., Su, Q., Grobe, R. 1999 Phys. Rev. A 59 604
Google Scholar
[24] Wagner, R. E., Ware, M. R., Shields, B. T., Su, Q., Grobe, R. 2011 Phys. Rev. Lett. 106 023601
Google Scholar
[25] Wang, L., Wu, B., Xie, B. S. 2019 Phys. Rev. A 100 022127
Google Scholar
[26] Cheng, T., Su, Q., Grobe, R. 2010 Contemp. Phys. 51 315
Google Scholar
DownLoad:
Metrics
- Abstract views: 339
- PDF Downloads: 2
- Cited By: 0
