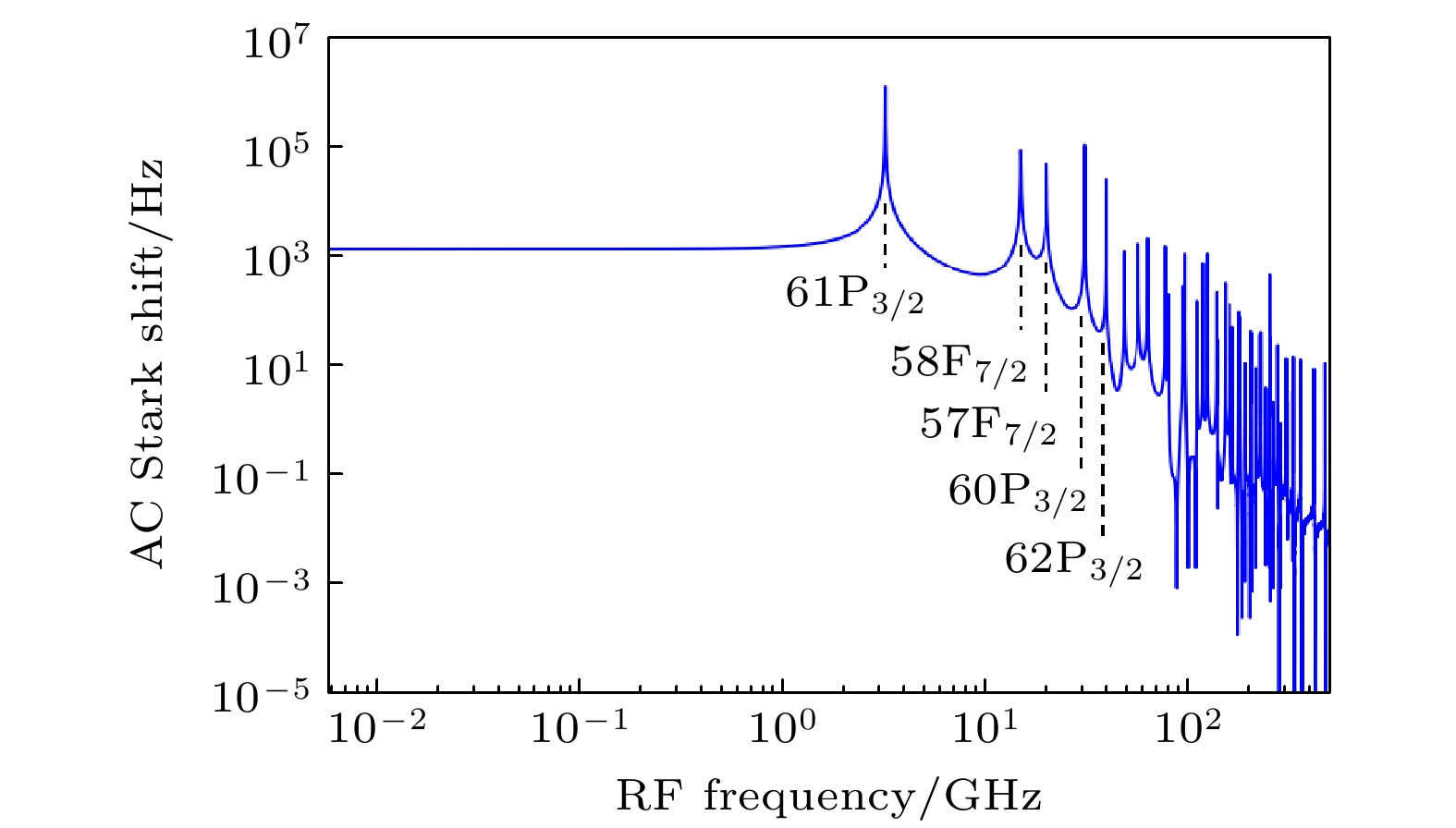
-
Rydberg atoms hold special attraction in electric applications due to their large transition electric dipole moments and huge polarization, which leads to a strong response of atom to electric fields. In radio-frequency (RF) fields, the Rydberg levels are AC Stark shift and splitting, which can realize the study of high-sensitivity electric field sensor of Rydberg atoms. In this work, we use the simpler Shirley’s time-independent Floquet Hamiltonian model to calculate the AC Stark energy spectrum of Cs Rydberg atoms. This model can reduce the basic Hamiltonian into such a Hamiltonian that includes only those Rydberg states that have direct dipole-allowed transitions with the target state, thereby significantly improving the speed of computation. The accuracy of the calculation is proved by fitting with the calculated frequency shift of DC Stark energy levels in the weak fields, and the polarizability of 60D5/2 and 70D5/2 Rydberg atomic states are obtained by fitting with the measured ion spectra of DC Stark Cs ultra-cold Rydberg atoms in magneto-optical trap. In addition, we calculate the AC Stark shift of Cs Rydberg atom
$ \left| {60{{\text{D}}_{5/2}},{m_j} = 1/2} \right\rangle $ state in electric fields with different frequencies with ε = 100 mV/m. Rydberg atoms provide a structured spectrum of sensitivity to electric fields due to strong resonant interaction and off-resonant interaction with many dipole-allowed transitions to nearby Rydberg states. This kind of the frequency response structure is of significance to a broadband sensor. And we calculate the sensitivity and the scaling of the signal-to-noise ratio (SNR), β, varying with detuning from the$ \left| {60{{\text{D}}_{5/2}}} \right\rangle \to \left| {61{{\text{P}}_{3/2}}} \right\rangle $ transition. The value of β allows one to use the result for any Rydberg state sensor to determine the SNR for any Ε in a 1 s measurement. Therefrom, Rydberg sensor can preferentially detect many RF frequencies spreading across its carrier spectral range without modification while effectively rejecting large portions where the atom response is significantly weaker, and the signal depends primarily on the detuning of the RF field to the nearest resonance which does not convey the RF frequency directly.-
Keywords:
- AC Stark effect /
- Rydberg sensor /
- Floquet method
[1] Fabre C, Gross M, Raimond J M, Haroche S 1983 J. Phys. B 16 L671
Google Scholar
[2] Hansen W 1983 J. Phys. B. 16 933
Google Scholar
[3] Feng Z G, Zhang H, Che J L, Zhang L J, Li C Y, Zhao J M, Jia S T 2011 Phys. Rev. A 83 042711
Google Scholar
[4] Fabre C, Haroche S 1975 Opt. Commun. 15 254
Google Scholar
[5] Shirley J H 1965 Phys. Rev. 138 B979
Google Scholar
[6] Meyer D H, Castillo Z A, Cox K C, Kunz P D 2020 J. Phys. B 53 034001
Google Scholar
[7] Jing M Y, Hu Y, Ma J, Zhang H, Zhang L J, Xiao L T, Jia S T 2020 Nat. Phys. 16 911
Google Scholar
[8] Yang W G, Jing M Y, Zhang H, Zhang L J, Xiao L T, Jia S T 2023 Phys. Rev. Appl. 19 064021
Google Scholar
[9] 张临杰, 景明勇, 张好 2022 山西大学学报 45 712
Google Scholar
Zhang L J, Jing M Y, Zhang H 2022 J. Shanxi Univ. 45 712
Google Scholar
[10] Fan H, Kumar S, Sedlacek J, Kübler H, Karimkashi S, Shaffer J P 2015 J. Phys. B 48 202001
Google Scholar
[11] Jiao Y C, Han X X, Yang Z W, Li J K, Raithel G, Zhao J M, Jia S T 2016 Phys. Rev. A 94 023832
Google Scholar
[12] Jiao Y C, Hao L P, Han X X, Bai S Y, Raithel G, Zhao J M, Jia S T 2017 Phys. Rev. Appl. 8 014028
Google Scholar
[13] Liao K Y, Tu H T, Yang S Z, Chen C J, Liu X H, Liang J, Zhang X D, Yan H, Zhu S L 2020 Phys. Rev. A 101 053432
Google Scholar
[14] Jia F D, Liu X B, Mei J, Yu Y H, Zhang H Y, Lin Z Q, Dong H Y, Zhang J, Xie F, Zhong Z P 2021 Phys. Rev. A 103 063113
Google Scholar
[15] Liu X B, Jia F D, Zhang H Y, Mei J, Yu Y H, Liang W C, Zhang J, Xie F, Zhong Z P 2021 AIP Adv. 11 085127
Google Scholar
[16] Hu J L, Jiao Y C, He Y H, Zhang H, Zhang L J, Zhao J M, Jia S T 2023 EPJ Quantum Tech. 10 51
Google Scholar
[17] Sedlacek J A, Schwettmann A, Kübler H, Löw R, Pfau T, Shaffer J P 2012 Nat. Phys. 8 819
Google Scholar
[18] Sedlacek J A, Schwettmann A, Kübler H, Shaffer J P 2013 Phys. Rev. Lett. 111 063001
Google Scholar
[19] Simons M T, Haddab A H, Gordon J A, Holloway C L 2019 Appl. Phys. Lett. 114 114101
Google Scholar
[20] Liu Z K, Zhang L H, Liu B, Zhang Z Y, Guo G C, Ding D S, Shi B S 2022 Nat. Commun. 13 1997
Google Scholar
[21] Zhou F, Jia F D, Liu X B, Yu Y H, Mei J, Zhang J, Xie F, Zhong Z P 2023 J. Phys. B 56 025501
Google Scholar
[22] Cui Y, Jia F D, Hao J H, Wang Y H, Zhou F, Liu X B, Yu Y H, Mei J, Bai J H, Bao Y Y, Hu D, Wang Y, Liu Y, Zhang J, Xie F, Zhong Z P 2023 Phys. Rev. A 107 043102
Google Scholar
[23] Li X H, Cui Y, Hao J H, Zhou F, Wang Y X, Jia F D, Zhang J, Xie F, Zhong Z P 2023 Opt. Express 31 38165
Google Scholar
[24] 杨凯, 安强, 姚佳伟, 毛瑞棋, 林沂, 刘燚, 付云起 2022 光学学报 42 1528002
Google Scholar
Yang K, An Q, Yao J W, Mao R Q, Lin Y, Liu Y, Fu Y Q 2022 Acta Opt. Sin. 42 1528002
Google Scholar
[25] Anderson D A, Schwarzkopf A, Miller S A, Thaicharoen N, Raithel G, Gordon J A, Holloway C L 2014 Phys. Rev. A 90 043419
Google Scholar
[26] Anderson D A, Miller S A, Raithel G, Gordon J A, Butler M L, Holloway C L 2016 Phys. Rev. Appl. 5 034003
Google Scholar
[27] Khadjavi A, Lurio A, Happer W 1968 Phys. Rev. 167 128
Google Scholar
[28] Zimmerman M L, Littman M G, Kash M M, Kleppner D 1979 Phys. Rev. A 20 2251
Google Scholar
[29] Bason M G, Tanasittikosol M, Sargsyan A, Mohapatra A K, Sarkisyan D, Potvliege R M, Adams C S 2010 New J. Phys. 12 065015
Google Scholar
[30] Jau Y Y, Carter T 2020 Phys. Rev. Appl. 13 054034
Google Scholar
[31] Mohapatra A K, Bason M G, Butscher B, Weatherill K J, Adams C S 2008 Nat. Phys. 4 890
Google Scholar
[32] Wade C G, Šibalić N, Melo N R, Kondo J M, Adams C S, Weatherill K J 2017 Nat. Photon. 11 40
Google Scholar
-
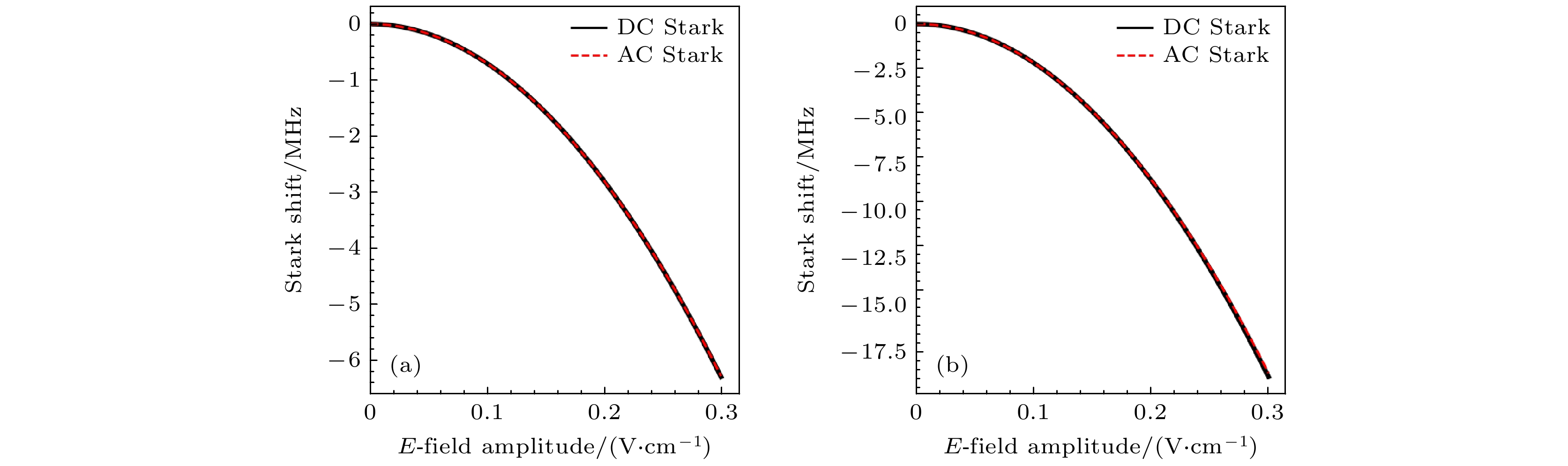
图 1 理论计算 Cs 原子(a) $ \left| {60{{\text{D}}_{5/2}}, {m_j} = 5/2} \right\rangle $和(b) $ \left| {70{{\text{D}}_{5/2}}, {m_j} = 5/2} \right\rangle $态的Stark能谱, 红色虚线为AC Stark能谱, 黑色实线为校准后的DC Stark能谱
Figure 1. Calculated Stark spectra for Cs Rydberg atom of (a) $ \left| {60{{\text{D}}_{5/2}}, {m_j} = 5/2} \right\rangle $ and (b) $ \left| {70{{\text{D}}_{5/2}}, {m_j} = 5/2} \right\rangle $ state. The red dotted line is the AC Stark spectrum, and the black solid line is the Stark map in a DC electric field on a field axis scaled such that the rms fields.
图 2 实验测的x方向70D5/2里德伯原子 Stark 谱, 黑、红和绿色点线分别为理论计算的mj = 1/2, 3/2和5/2的Stark 谱
Figure 2. Measurements of the Stark spectrum for 70D5/2 Rydberg atom in the x-direction, and the black, red, and green dotted lines show the calculation Stark spectra of mj = 1/2, 3/2, and 5/2, respectively.
图 3 Cs里德伯原子60D5/2态在 ε = 100 mV/m不同频率射频场中的AC Stark频移, 其中临近的共振态用黑色虚线标定
Figure 3. AC Stark shifts of the 60D5/2 state in RF field with ε = 100 mV/m. The adjacent resonant state is labeled with a black dashed line.
图 4 在低频DC场中, 测量时间t = 1 s, 原子数N = 103 (104, 105, 106)的$ \left| {60{{\text{D}}_{5/2}}, {m_j} = 1/2} \right\rangle $Cs里德伯原子的最小可检测场与射频频率的关系
Figure 4. In a quasi-DC field, the minimum detectable field in a 1 s measurement vs. RF frequency using a $ \left| {60{{\text{D}}_{5/2}}, {m_j} = 1/2} \right\rangle $ target state with N = 103 (104, 105, 106) Cs Rydberg atoms.
图 5 测量时间为1 s的最小可检测场和信噪比缩放因子β (红色实线)与$ \left| {60{{\text{D}}_{5/2}}} \right\rangle \to \left| {61{{\text{P}}_{3/2}}} \right\rangle $射频共振失谐量的关系, 其中三角形、正方形和五边形分别对应β = 1, 1—2和 2
Figure 5. The minimum detectable field in a 1 s measurement and the scaling of the SNR (red solid), β, versus detuning of RF transition from the $ \left| {60{{\text{D}}_{5/2}}} \right\rangle \to $$ \left| {61{{\text{P}}_{3/2}}} \right\rangle $. The triangle, square, and pentagon symbols match correspond to β = 1, 2 or somewhere in between, respectively.
表 1 实验测量极化率与计算60D5/2和70D5/2态极化率的比较
Table 1. Comparisons of the measurement and calculation of the polarizability for 60D5/2 and 70D5/2 Rydberg atom.
状态 极化率实验值/
(MHz·cm2/V2)极化率理论值/
(MHz·cm2/V2)$ 60{{\text{D}}_{5/2, 1/2}} $ –5007.11 –4984.80 $ 60{{\text{D}}_{5/2, 3/2}} $ –3620.61 –3633.78 $ 60{{\text{D}}_{5/2, 5/2}} $ 281.19 280.93 $ 70{{\text{D}}_{5/2, 1/2}} $ –15455.87 –15432.29 $ 70{{\text{D}}_{5/2, 3/2}} $ –11160.68 –11171.34 $ 70{{\text{D}}_{5/2, 5/2}} $ 835.18 833.77 
DownLoad: CSV
-
[1] Fabre C, Gross M, Raimond J M, Haroche S 1983 J. Phys. B 16 L671
Google Scholar
[2] Hansen W 1983 J. Phys. B. 16 933
Google Scholar
[3] Feng Z G, Zhang H, Che J L, Zhang L J, Li C Y, Zhao J M, Jia S T 2011 Phys. Rev. A 83 042711
Google Scholar
[4] Fabre C, Haroche S 1975 Opt. Commun. 15 254
Google Scholar
[5] Shirley J H 1965 Phys. Rev. 138 B979
Google Scholar
[6] Meyer D H, Castillo Z A, Cox K C, Kunz P D 2020 J. Phys. B 53 034001
Google Scholar
[7] Jing M Y, Hu Y, Ma J, Zhang H, Zhang L J, Xiao L T, Jia S T 2020 Nat. Phys. 16 911
Google Scholar
[8] Yang W G, Jing M Y, Zhang H, Zhang L J, Xiao L T, Jia S T 2023 Phys. Rev. Appl. 19 064021
Google Scholar
[9] 张临杰, 景明勇, 张好 2022 山西大学学报 45 712
Google Scholar
Zhang L J, Jing M Y, Zhang H 2022 J. Shanxi Univ. 45 712
Google Scholar
[10] Fan H, Kumar S, Sedlacek J, Kübler H, Karimkashi S, Shaffer J P 2015 J. Phys. B 48 202001
Google Scholar
[11] Jiao Y C, Han X X, Yang Z W, Li J K, Raithel G, Zhao J M, Jia S T 2016 Phys. Rev. A 94 023832
Google Scholar
[12] Jiao Y C, Hao L P, Han X X, Bai S Y, Raithel G, Zhao J M, Jia S T 2017 Phys. Rev. Appl. 8 014028
Google Scholar
[13] Liao K Y, Tu H T, Yang S Z, Chen C J, Liu X H, Liang J, Zhang X D, Yan H, Zhu S L 2020 Phys. Rev. A 101 053432
Google Scholar
[14] Jia F D, Liu X B, Mei J, Yu Y H, Zhang H Y, Lin Z Q, Dong H Y, Zhang J, Xie F, Zhong Z P 2021 Phys. Rev. A 103 063113
Google Scholar
[15] Liu X B, Jia F D, Zhang H Y, Mei J, Yu Y H, Liang W C, Zhang J, Xie F, Zhong Z P 2021 AIP Adv. 11 085127
Google Scholar
[16] Hu J L, Jiao Y C, He Y H, Zhang H, Zhang L J, Zhao J M, Jia S T 2023 EPJ Quantum Tech. 10 51
Google Scholar
[17] Sedlacek J A, Schwettmann A, Kübler H, Löw R, Pfau T, Shaffer J P 2012 Nat. Phys. 8 819
Google Scholar
[18] Sedlacek J A, Schwettmann A, Kübler H, Shaffer J P 2013 Phys. Rev. Lett. 111 063001
Google Scholar
[19] Simons M T, Haddab A H, Gordon J A, Holloway C L 2019 Appl. Phys. Lett. 114 114101
Google Scholar
[20] Liu Z K, Zhang L H, Liu B, Zhang Z Y, Guo G C, Ding D S, Shi B S 2022 Nat. Commun. 13 1997
Google Scholar
[21] Zhou F, Jia F D, Liu X B, Yu Y H, Mei J, Zhang J, Xie F, Zhong Z P 2023 J. Phys. B 56 025501
Google Scholar
[22] Cui Y, Jia F D, Hao J H, Wang Y H, Zhou F, Liu X B, Yu Y H, Mei J, Bai J H, Bao Y Y, Hu D, Wang Y, Liu Y, Zhang J, Xie F, Zhong Z P 2023 Phys. Rev. A 107 043102
Google Scholar
[23] Li X H, Cui Y, Hao J H, Zhou F, Wang Y X, Jia F D, Zhang J, Xie F, Zhong Z P 2023 Opt. Express 31 38165
Google Scholar
[24] 杨凯, 安强, 姚佳伟, 毛瑞棋, 林沂, 刘燚, 付云起 2022 光学学报 42 1528002
Google Scholar
Yang K, An Q, Yao J W, Mao R Q, Lin Y, Liu Y, Fu Y Q 2022 Acta Opt. Sin. 42 1528002
Google Scholar
[25] Anderson D A, Schwarzkopf A, Miller S A, Thaicharoen N, Raithel G, Gordon J A, Holloway C L 2014 Phys. Rev. A 90 043419
Google Scholar
[26] Anderson D A, Miller S A, Raithel G, Gordon J A, Butler M L, Holloway C L 2016 Phys. Rev. Appl. 5 034003
Google Scholar
[27] Khadjavi A, Lurio A, Happer W 1968 Phys. Rev. 167 128
Google Scholar
[28] Zimmerman M L, Littman M G, Kash M M, Kleppner D 1979 Phys. Rev. A 20 2251
Google Scholar
[29] Bason M G, Tanasittikosol M, Sargsyan A, Mohapatra A K, Sarkisyan D, Potvliege R M, Adams C S 2010 New J. Phys. 12 065015
Google Scholar
[30] Jau Y Y, Carter T 2020 Phys. Rev. Appl. 13 054034
Google Scholar
[31] Mohapatra A K, Bason M G, Butscher B, Weatherill K J, Adams C S 2008 Nat. Phys. 4 890
Google Scholar
[32] Wade C G, Šibalić N, Melo N R, Kondo J M, Adams C S, Weatherill K J 2017 Nat. Photon. 11 40
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2206
- PDF Downloads: 130
- Cited By: 0
