-
In ultracold atomic experiments, evaporative cooling is usually achieved by using Feshbach resonance magnetic fields on the order of Gauss to hundreds of Gausses. The frequency of resonant transition induced by the optical field or radiofrequency is directly affected by the stability of the quantum axis. For example, the phase between two linearly independent vectors of a qubit is affected by the magnetic field noise. Based on the Feshbach resonance technique, magnetic field regulation has become a basic tool to control the interaction between atoms. Narrow Feshbach resonance shows unique advantages in high-temperature superconducting, superfluidity, neutron star state simulation, etc. However, since its resonance width and Fermi energy can be compared with each other, the scattering characteristics are greatly disturbed by the magnetic field. Therefore, a stable and uniform magnetic field is a prerequisite for studying the narrow Feshbach resonances. In experiment, Helmholtz coils are usually used to provide the magnetic field for cold atomic gas, and the magnetic field noise is generally determined by the coil current noise and other magnetic field noises of the environment. However, there are relatively few researches of the high-precision control of large magnetic fields above hundreds of Gausses. With a larger coil current required, the coil current noise contributes more to the magnetic field noise, thus high-precision control of large magnetic fields is still challenging. In this paper, a magnetic field locking system is used to realize a
$2.27 \times 10^{-6} $ level locking of the Feshbach magnetic field. A feedback locking system is used to achieve the stability by shunting the magnetic field coil current noise. Compared with the non-locked magnetic field, the low-frequency current noise is suppressed by more than 45 dB. To assess the stability of the actual magnetic field at the atoms, the Rabi oscillation is measured, the coherence time increases nearly 9.6 times, which effectively improves the stability of the ultracold atomic system. Furthermore, we measure the atom number fluctuation at the Gaussian inflection point of the loss spectrum under different Raman pulse widths to evaluate the noise of the magnetic field. Roman pulse duration up to a 24 μs is used to increase the sensitivity of atom number fluctuation in loss spectrum relative to magnetic field noise, of which the root mean square (RMS) noise is suppressed from 20.66 mGs to 1.2 mGs, a 16-fold reduction of the noise is obtained. Such a magnetic field locking system can provide an accurate and stable background magnetic field for ultracold atomic gases, which is of great significance for extending quantum storage time, precisely controlling atomic scattering, and simulating of condensed matter and other ultracold quantum gas in experiment.-
Keywords:
- magnetic field locking /
- Feshbach resonance /
- ultracold quantum gas
[1] Xu Z, Wu Y, Tian L, Chen L, Zhang Z, Yan Z, Li S, Wang H, Xie C, Peng K 2013 Phys. Rev. Lett. 111 240503
Google Scholar
[2] Duan L M, Lukin M D, Cirac J I, Zoller P 2001 Nature 414 413
Google Scholar
[3] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[4] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
Google Scholar
[5] 杨荣国, 张超霞, 李妮, 张静, 郜江瑞 2019 68 094205
Google Scholar
Yang R G, Zhang C X, Li N, Zhang J, Gao J R 2019 Acta Phys. Sin. 68 094205
Google Scholar
[6] Jing J, Zhang J, Yan Y, Zhao F, Xie C, Peng K 2003 Phys. Rev. Lett. 90 167903
Google Scholar
[7] Riedl S, Lettner M, Vo C, Baur S, Rempe G, Durr S 2012 Phys. Rev. A 85 022318
Google Scholar
[8] Szwer D J, Webster S C, Steane A M, Lucas D M 2011 J. Phys. B: At. Mol. Opt. Phys. 44 025501
Google Scholar
[9] De Lange G, Wang Z H, Ristè D, Dobrovitski V V, Hanson R 2010 Science 330 60
Google Scholar
[10] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[11] Bruun G M 2004 Phys. Rev. A. 70 053602
Google Scholar
[12] Hazlett E L, Zhang Y, Stites R W, O’Hara K M 2012 Phys. Rev. Lett. 108 045304
Google Scholar
[13] Jagannathan A, Arunkumar N, Joseph J A, Thomas J E 2016 Phys. Rev. Lett. 116 075301
Google Scholar
[14] Deng S J, Shi Z Y, Diao P P, Yu Q L, Zhai H, Qi R, Wu H B 2016 Science 353 371
Google Scholar
[15] Deng S J, Diao P P, Yu Q L, Wu H B 2015 Chin. Phys. Lett. 32 053401
Google Scholar
[16] Ruster T, Schmiegelow C T, Kaufmann H, Warschburger C, Schmidt-Kaler F, Poschinger U G 2016 Appl. Phys. B 122 254
[17] Brandl M F, van Mourik M W, Postler L, Nolf A, Lakhmanskiy K, Paiva R R, Moller S, Daniilidis N, Haffner H, Kaushal V, Ruster T, Warschburger C, Kaufmann H, Poschinger U G, Schmidt-Kaler F, Schindler P, Monz T, Blatt R 2016 Rev. Sci. Instrum. 87 113103
Google Scholar
[18] Lamporesi G, Donadello S, Serafini S, Ferrari G 2013 Rev. Sci. Instrum. 84 063102
Google Scholar
[19] Farolfi A, Trypogeorgos D, Colzi G, Fava E, Lamporesi G, Ferrari G 2019 Rev. Sci. Instrum. 90 115114
Google Scholar
[20] Kornack T W, Smullin S J, Lee S K, Romalis M V 2007 Appl. Phys. Lett. 90 223501
Google Scholar
[21] Tayler M C D, Theis T, Sjolander T F, Blanchard J W, Kentner A, Pustelny S, Pines A, Budker D 2017 Rev. Sci. Instrum. 88 091101
Google Scholar
[22] Burt E A, Ekstrom C R 2002 Rev. Sci. Instrum. 73 2699
Google Scholar
[23] Kubelka-Lange A, Herrmann S, Grosse J, Lammerzahl C, Rasel E M, Braxmaier C 2016 Rev. Sci. Instrum. 87 063101
Google Scholar
[24] Shilmi K 2013 Ph.D. Dissertation (Israel: Weizmann Institute Of Science)
[25] Dedman C J, Dall R G, Byron L J, Truscott A G 2007 Rev. Sci. Instrum. 78 024703
Google Scholar
[26] Merkel B, Thirumalai K, Tarlton J E, Schafer V M, Ballance C J, Harty T P, Lucas D M 2019 Rev. Sci. Instrum. 90 044702
Google Scholar
[27] Tarlton J E 2018 Ph.D. Dissertation (Ann Arbor: Imperial College London )
[28] Xu X T, Wang Z Y, Jiao R H, Yi C R, Sun W, Chen S 2019 Rev. Sci. Instrum. 90 054708
Google Scholar
[29] Yang Y M, Xie H T, Ji W C, Wang Y F, Zhang W Y, Chen S, Jiang X 2019 Rev. Sci. Instrum. 90 014701
Google Scholar
[30] Wang J, She S, Zhang S 2002 Rev. Sci. Instrum. 73 2175
Google Scholar
[31] Botti L, Buffa R, Bertoldi A, Bassi D, Ricci L 2006 Rev. Sci. Instrum. 77 035103
Google Scholar
[32] Zhang X, Chen Y, Wu Z, Wang J, Fan J, Deng S, Wu H 2021 Science 373 1359
Google Scholar
[33] 俞千里, 邓书金, 刁鹏鹏, 李芳, 尉石, 武海斌 2017 量子光学学报 23 254
Yu Q L, Deng S J, Diao P P, Li F, Yu s, Wu H B 2017 J. Quantum Opt. 23 254
[34] Li R, Wu Y L, Rui Y, Li B, Jiang Y Y, Ma L S, Wu H B 2020 Phys. Rev. Lett. 124 063002
Google Scholar
[35] Claudon J, Zazunov A, Hekking F W J, Buisson O 2008 Phys. Rev. B 78 184503
Google Scholar
-
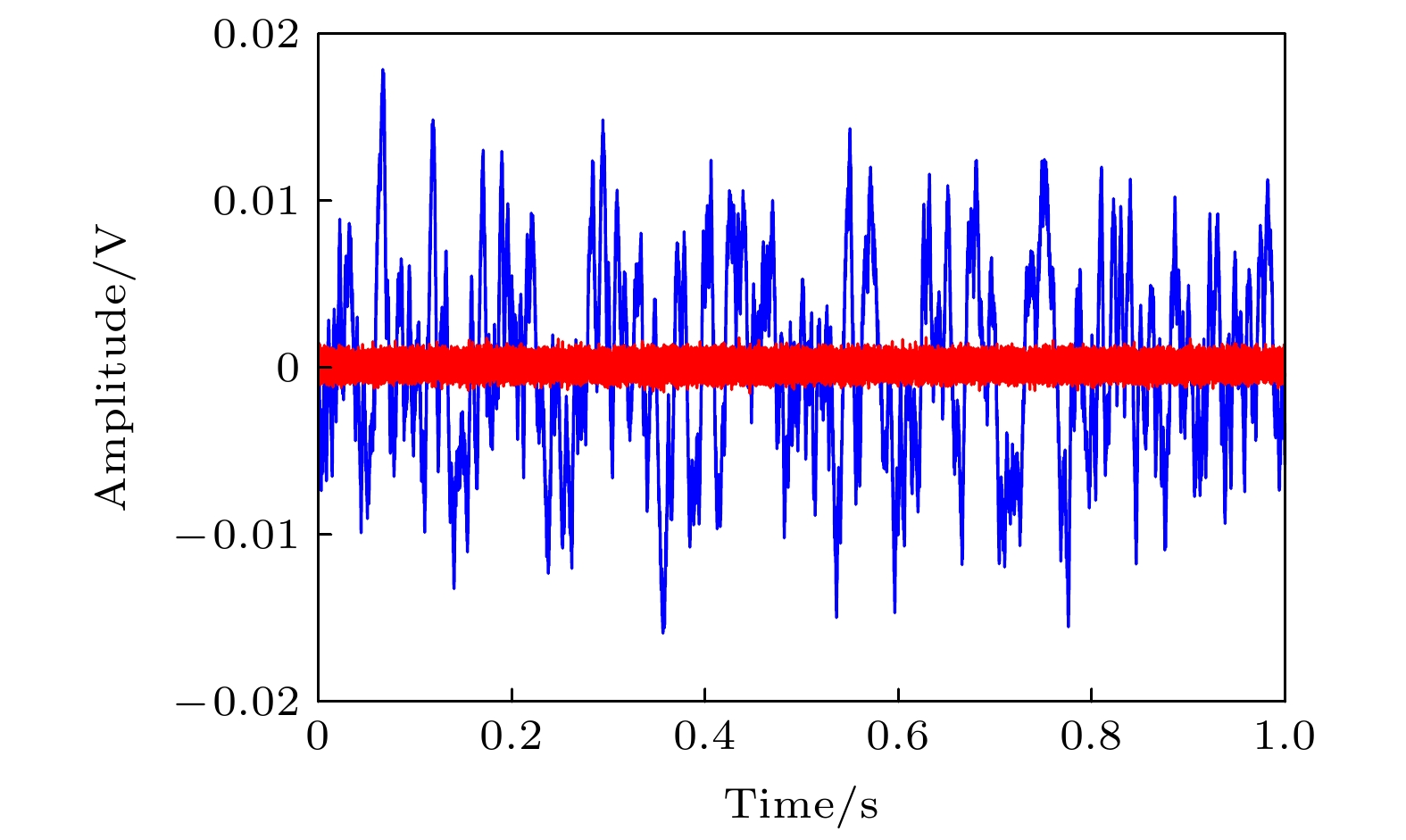
图 1 (a)磁场锁定系统. 图中电源输出为恒流模式, 线圈与分流支路并联, 电流传感器测量通过线圈的电流, 并通过锁定电路控制
$I_{\rm{noise}}$ 的大小. (b)信号处理流程图. 线圈电流$I_{\rm{coils}}$ 通过电流探测器($\times {1}/{2000}$ )、10 Ω采样电阻($\times\;10$ )后, 再经过与粗调、细调参考电压作差放大($\times \;200$ ), 获得误差信号$V_{\rm{error}}$ , 对误差信号进行滤波、补偿处理后, 再积分并选择合适的反馈带宽, 最终获得控制电压$V_{\rm{noise}}$ Figure 1. (a) Magnetic locking system. The power supply runs in constant-current mode. The coil is in parallel with the
$I_{\rm{noise}}$ . The current sensor measures the current passing through the coil and controls the$I_{\rm{noise}}$ through the magnetic field stabilization circuit. (b) Block diagram of the locking circuit. After the coil current$I_{\rm{coils}}$ passes through the current detector with a conversion ratio of 1∶2000, the signal is reduced by 2000 times, and then amplified by 10 times through the 10 Ω sampling resistor, and then amplified by 200 times after the difference with the reference voltage for coarse adjustment and fine adjustment. After filtering and compensating the error signal, the gain is obtained through the integrating circuit and the bandwidth is defined to obtain the control voltage.
图 2 锁定前后误差信号波形图. 红色为有磁场锁定时的误差信号, 蓝色为没有磁场锁定时的误差信号
Figure 2. Error signal waveform. Red is the error signal measured when the magnetic field is stable, and blue is the error signal obtained when the magnetic field is locked
图 3 误差信号的功率谱密度. 红色为锁定后误差信号的功率谱密度, 蓝线为锁定前误差信号的功率谱密度, 黑线为锁定系统的底噪
Figure 3. The power spectral density (PSD) of the error signal. The red line is the PSD of the error signal after locking, the blue line is the PSD of the error signal before locking, and the black line is the measured noise floor of the system.
图 4 (a)磁场锁定前和(b)磁场锁定后, 6Li原子
$|2\rangle$ 和$|5\rangle$ 态之间的拉比振荡. 蓝色点为测量值, 红色曲线为拟合曲线Figure 4. (a) Rabi oscillation between 6Li ground state
$|2\rangle$ and$|5\rangle$ : (a) Without magnetic lock; (b) with magnetic lock. Blue line: measured results; red line: fitted results
图 5 锁定前后的原子损失谱 (a) Raman光π脉冲2.5 μs, 没有磁场锁定时的拉曼跃迁谱; (b) Raman光π脉冲2.5 μs, 磁场锁定时的原子损失谱; (c) Raman光π脉冲24 μs, 磁场锁定时的拉曼跃迁谱. 蓝色的点是测量值, 红线为拟合曲线
Figure 5. The atom loss spectrum. The atom loss spectrum with 2.5 μs Raman π pulse duration when the magnetic field is (a) unlocked and (b) locked; (c) the atom loss spectrum with 24 μs Raman π pulse duration when the magnetic field is locked. The blue dots are the measured data, and the red line is the fitted curve.
图 6 原子损失谱的高斯拐点处原子数的抖动 (a) Raman光π脉冲为2.5
$\text{µ}{\rm{s}}$ 时, 红色(蓝色)数据点为磁场锁定(未锁定)时原子的抖动; (b) Raman光π脉冲为24$\text{µ}{\rm{s}}$ 时, 绿色数据点为磁场锁定时原子的抖动. 图(b)与图(a)相比, 原子数对失谐有不同的敏感度系数, 提高了16.5倍Figure 6. Relative atom number fluctuation when two-photon detuning is at half width of the atom loss spectrum: (a) When Raman π pulse duration is 2.5
$\text{µ}{\rm{s}}$ , red (blue) dots are the measured data when the magnetic field is locked (unlocked); (b) when Raman π pulse duration is 24$\text{µ}{\rm{s}}$ , green dots are the measured data when the magnetic field is locked -
[1] Xu Z, Wu Y, Tian L, Chen L, Zhang Z, Yan Z, Li S, Wang H, Xie C, Peng K 2013 Phys. Rev. Lett. 111 240503
Google Scholar
[2] Duan L M, Lukin M D, Cirac J I, Zoller P 2001 Nature 414 413
Google Scholar
[3] Feynman R P 1982 Int. J. Theor. Phys. 21 467
Google Scholar
[4] Georgescu I M, Ashhab S, Nori F 2014 Rev. Mod. Phys. 86 153
Google Scholar
[5] 杨荣国, 张超霞, 李妮, 张静, 郜江瑞 2019 68 094205
Google Scholar
Yang R G, Zhang C X, Li N, Zhang J, Gao J R 2019 Acta Phys. Sin. 68 094205
Google Scholar
[6] Jing J, Zhang J, Yan Y, Zhao F, Xie C, Peng K 2003 Phys. Rev. Lett. 90 167903
Google Scholar
[7] Riedl S, Lettner M, Vo C, Baur S, Rempe G, Durr S 2012 Phys. Rev. A 85 022318
Google Scholar
[8] Szwer D J, Webster S C, Steane A M, Lucas D M 2011 J. Phys. B: At. Mol. Opt. Phys. 44 025501
Google Scholar
[9] De Lange G, Wang Z H, Ristè D, Dobrovitski V V, Hanson R 2010 Science 330 60
Google Scholar
[10] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[11] Bruun G M 2004 Phys. Rev. A. 70 053602
Google Scholar
[12] Hazlett E L, Zhang Y, Stites R W, O’Hara K M 2012 Phys. Rev. Lett. 108 045304
Google Scholar
[13] Jagannathan A, Arunkumar N, Joseph J A, Thomas J E 2016 Phys. Rev. Lett. 116 075301
Google Scholar
[14] Deng S J, Shi Z Y, Diao P P, Yu Q L, Zhai H, Qi R, Wu H B 2016 Science 353 371
Google Scholar
[15] Deng S J, Diao P P, Yu Q L, Wu H B 2015 Chin. Phys. Lett. 32 053401
Google Scholar
[16] Ruster T, Schmiegelow C T, Kaufmann H, Warschburger C, Schmidt-Kaler F, Poschinger U G 2016 Appl. Phys. B 122 254
[17] Brandl M F, van Mourik M W, Postler L, Nolf A, Lakhmanskiy K, Paiva R R, Moller S, Daniilidis N, Haffner H, Kaushal V, Ruster T, Warschburger C, Kaufmann H, Poschinger U G, Schmidt-Kaler F, Schindler P, Monz T, Blatt R 2016 Rev. Sci. Instrum. 87 113103
Google Scholar
[18] Lamporesi G, Donadello S, Serafini S, Ferrari G 2013 Rev. Sci. Instrum. 84 063102
Google Scholar
[19] Farolfi A, Trypogeorgos D, Colzi G, Fava E, Lamporesi G, Ferrari G 2019 Rev. Sci. Instrum. 90 115114
Google Scholar
[20] Kornack T W, Smullin S J, Lee S K, Romalis M V 2007 Appl. Phys. Lett. 90 223501
Google Scholar
[21] Tayler M C D, Theis T, Sjolander T F, Blanchard J W, Kentner A, Pustelny S, Pines A, Budker D 2017 Rev. Sci. Instrum. 88 091101
Google Scholar
[22] Burt E A, Ekstrom C R 2002 Rev. Sci. Instrum. 73 2699
Google Scholar
[23] Kubelka-Lange A, Herrmann S, Grosse J, Lammerzahl C, Rasel E M, Braxmaier C 2016 Rev. Sci. Instrum. 87 063101
Google Scholar
[24] Shilmi K 2013 Ph.D. Dissertation (Israel: Weizmann Institute Of Science)
[25] Dedman C J, Dall R G, Byron L J, Truscott A G 2007 Rev. Sci. Instrum. 78 024703
Google Scholar
[26] Merkel B, Thirumalai K, Tarlton J E, Schafer V M, Ballance C J, Harty T P, Lucas D M 2019 Rev. Sci. Instrum. 90 044702
Google Scholar
[27] Tarlton J E 2018 Ph.D. Dissertation (Ann Arbor: Imperial College London )
[28] Xu X T, Wang Z Y, Jiao R H, Yi C R, Sun W, Chen S 2019 Rev. Sci. Instrum. 90 054708
Google Scholar
[29] Yang Y M, Xie H T, Ji W C, Wang Y F, Zhang W Y, Chen S, Jiang X 2019 Rev. Sci. Instrum. 90 014701
Google Scholar
[30] Wang J, She S, Zhang S 2002 Rev. Sci. Instrum. 73 2175
Google Scholar
[31] Botti L, Buffa R, Bertoldi A, Bassi D, Ricci L 2006 Rev. Sci. Instrum. 77 035103
Google Scholar
[32] Zhang X, Chen Y, Wu Z, Wang J, Fan J, Deng S, Wu H 2021 Science 373 1359
Google Scholar
[33] 俞千里, 邓书金, 刁鹏鹏, 李芳, 尉石, 武海斌 2017 量子光学学报 23 254
Yu Q L, Deng S J, Diao P P, Li F, Yu s, Wu H B 2017 J. Quantum Opt. 23 254
[34] Li R, Wu Y L, Rui Y, Li B, Jiang Y Y, Ma L S, Wu H B 2020 Phys. Rev. Lett. 124 063002
Google Scholar
[35] Claudon J, Zazunov A, Hekking F W J, Buisson O 2008 Phys. Rev. B 78 184503
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4487
- PDF Downloads: 172
- Cited By: 0
