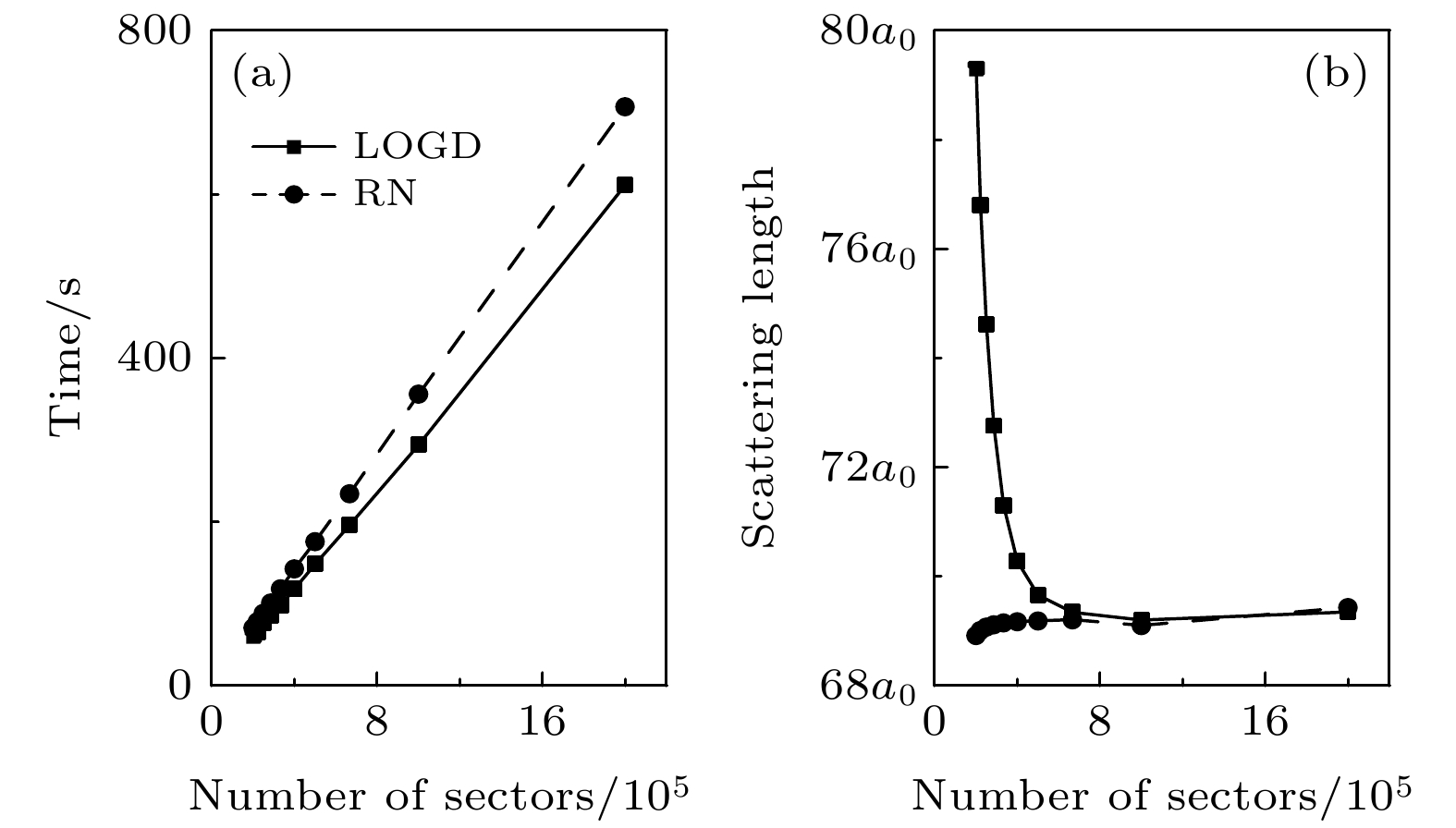
-
The renormalized Numerov algorithm is applied to solving time-independent Schrödinger equation relating to atom-atom collisions at ultralow temperature. The proprieties of Feshbach resonance in 39K-133Cs collisions are investigated as an example. The results show that the renormalized Numerov method can give excellent results for ultracold colliding process. In contrast to improved log derivative method, the renormalized Numerov method displays a certain weakness in computational efficiency under the same condition. However, it is much stable in a wide range of grid step size. Hence a new propagating method is proposed by combining renormalized Numerov and logarithmic derivative method which can save computational time with a better accuracy. This algorithm can be used to solve close-coupling Schrödinger equation at arbitrary temperature for two-body collisions.
[1] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[2] Vogels J M, Tsai C C, Freeland R S, Kokkelmans S J J M F, Verhaar B J, Heinzen D J 1997 Phys. Rev. A 56 R1067
Google Scholar
[3] Pellegrini P, Gacesa M, Côté R 2008 Phys. Rev. Lett. 101 053201
Google Scholar
[4] Giorgini S, Pitaevskii L P, Stringari S 2008 Rev. Mod. Phys. 80 1215
Google Scholar
[5] Gao B 1998 Phys. Rev. A 58 1728
Google Scholar
[6] Tiecke T G, Goosen M R, Walraven J T M, Kokkelmans S J J M F 2010 Phys. Rev. A 82 042712
Google Scholar
[7] Li Z, Madison K W 2009 Phys. Rev. A 79 042711
Google Scholar
[8] Johnson B R 1973 J. Comput. Phys. 13 445
Google Scholar
[9] Stechel E B, Walker R B, Light J C 1978 J. Chem. Phys. 69 3518
Google Scholar
[10] 李磐, 时雷, 毛庆和 2013 62 154205
Google Scholar
Li P, Shi L, Mao Q H 2013 Acta Phys. Sin. 62 154205
Google Scholar
[11] Dunn D, Grieves B 1989 J. Phys. A: Math. Gen. 22 L1093
Google Scholar
[12] Du M L 1993 Comput. Phys. Commun. 76 39
Google Scholar
[13] Johnson B R 1977 J. Chem. Phys. 67 4086
Google Scholar
[14] Colavecchia F D, Mrugala F, Parker G A, Pack R T 2003 J. Chem. Phys. 118 10387
Google Scholar
[15] Karman T, Janssen L M C, Sprenkels R, Groenenboom G C 2014 J. Chem. Phys. 141 064102
Google Scholar
[16] Blandon J, Park G A, Madrid C 2016 J. Phys. Chem. A 120 785
Google Scholar
[17] DeMille D 2002 Phys. Rev. Lett. 88 067901
Google Scholar
[18] Borsalino D, Vexiau R, Aymar M, Luc-Koening E, Dulieu O, Bouloufa-Maafa N 2016 J. Phys. B 49 055301
Google Scholar
[19] Gonzales-Sanchez L, Tacconi M, Bodo E, Gianturco F A 2008 Eur. Phys. J. D 49 85
Google Scholar
[20] Burke V M, Nobel C J 1995 Comput. Phys. Commun. 85 471
Google Scholar
[21] Gao B, Tiesinga E, Williams C J, Julienne P S 2005 Phys. Rev. A 72 042719
Google Scholar
[22] Patel H J, Blackley C L, Cornish S L, Hutson J M 2014 Phys. Rev. A 90 032716
Google Scholar
[23] Köppinger M P, Mccarron D J, Jenkin D L, Molony P K, Cho H W, Cornish S L, Ruth Le Sueur C, Blackley C L, Hutson J M 2014 Phys. Rev. A 89 033604
Google Scholar
[24] Ferber R, Nikolayeva O, Tamanis M, Knöckel H, Tiemann E 2013 Phys. Rev. A 88 012516
Google Scholar
[25] Gröbner M, Weinmann P, Kirilov E, Nägerl H C, Julienne P S, Ruth Le Sueur C, Hutson J M 2017 Phys. Rev. A 95 022715
Google Scholar
[26] D’Errico C, Zaccanti M, Fattori M, Roati G, Inguscio M, Modugno G, Simoni A 2007 New J. Phys. 9 223
Google Scholar
[27] Tiecke T G, Goosen M R, Ludewig A, Gensemer S D, Kraft S, Kokkelmans S J J M F, Walraven J T M 2010 Phys. Rev. Lett. 104 053202
Google Scholar
[28] Manolopoulos D E 1986 J. Chem. Phys. 85 6425
Google Scholar
-
图 1 39K-133Cs体系基单重态和三重态相互作用势
Figure 1. Ground electronic potential curves of singlet and triplet states in 39K-133Cs.
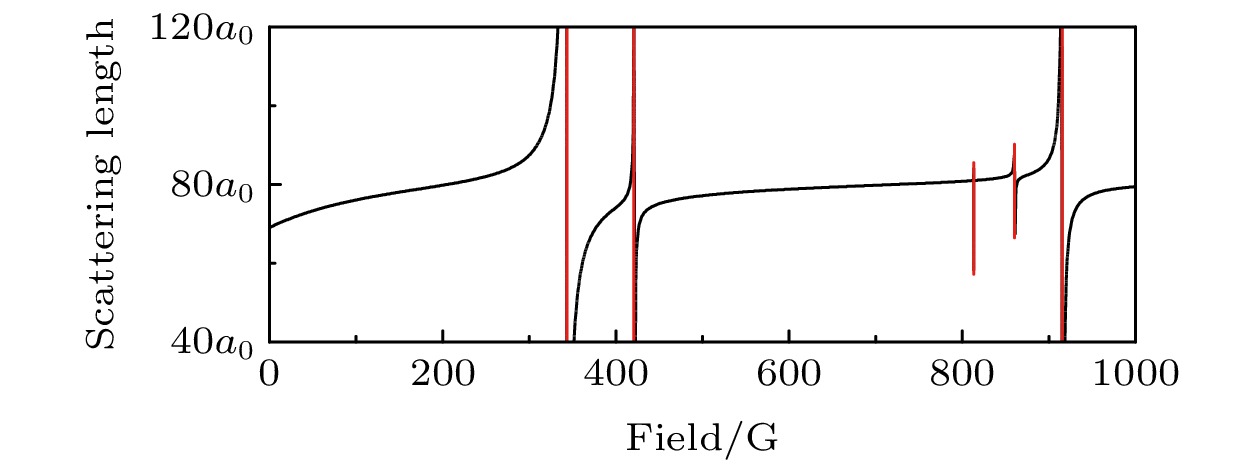
图 2 在入射通道
$ \left| {{f_{\text{K}}} = 1, \;{m_{f{\text{K}}}} = 1} \right\rangle + \left| {{f_{{\text{Cs}}}} = 3, \;{m_{f{\text{Cs}}}} = 3} \right\rangle $ 计算的磁场0—1000 G范围内的s波散射长度, 红线标记录5个共振位置Figure 2. Calculated s-wave scattering length in the incoming channel
$ \left| {{f_{\text{K}}} = 1, \;{m_{f{\text{K}}}} = 1} \right\rangle + \left| {{f_{{\text{Cs}}}} = 3, \;{m_{f{\text{Cs}}}} = 3} \right\rangle $ with field range 0–1000 G. The five resonant positions are labeled with red lines.
图 3 (a)采用两种方法计算100个磁场强度下散射长度的计算时间随格点数的变化; (b)在磁场为1 G时散射长度随格点数的变化
Figure 3. (a) Computational time of scattering length at 100 magnetic fields as a function of number of sectors by using two methods; (b) the scattering lengths as a function of number of sectors at 1 G.
表 1 两种计算方法得到的共振位置、共振宽度和背景散射长度参量
Table 1. Calculated resonant positions, widths and background scattering lengths in the incoming channel
$ \left| {{f_{\text{K}}} = 1, \;{m_{f{\text{K}}}} = 1} \right\rangle + \left| {{f_{{\text{Cs}}}} = 3, \;{m_{f{\text{Cs}}}} = 3} \right\rangle $ by both methods.RN LOGD B0 /G ΔB/G abg B0/G ΔB/G abg $ \begin{gathered} \left| {{f_{\text{K}}} = 1, \;{m_{f{\text{K}}}} = 1} \right\rangle + \\ \left| {{f_{{\text{Cs}}}} = 3, \;{m_{f{\text{Cs}}}} = 3} \right\rangle \\ \end{gathered} $ 341.89 4.8 79.1a0 341.90 4.8 79.0a0 421.35 0.4 74.7a0 421.36 0.4 74.7a0 831.14 4 × 10–4 80.9a0 831.14 3 × 10–4 81.0a0 860.50 0.05 82.1a0 860.52 0.05 82.0 a0 915.57 1.2 80.2a0 915.56 1.2 80.1a0 
DownLoad: CSV
表 2 采用短程RN和长程变步长LOGD相结合的传播方法得到的共振位置总误差, 这里两种方法的接合点在R = 20a0. 第一列ΔR表示在RN方法中使用的固定格点步长, 第二列NRN表示RN方法在短程传播的总步数, 第三列NLOGD表示在变步长LOGD方法在长程传播的总步数, 第四列
$ B_0^{\rm error} $ 表示使用两者结合方法产生的所有共振位置误差的绝对值总和Table 2. Total errors of resonant positions by using the method combining RN method in short range with LOGD with variable step size in long range, where the connected point is located at 20a0. The first column,
ΔR, represents the fixed step size in RN method. The second and third columns, NRN and NLOGD, denote the steps propagated in RN and LOGD methods, respectively. The last column is the sum of errors for all resonant positions. ΔR NRN NLOGD $ B_0^{\rm error} $/G 0.004a0 4251 1990 0.02 0.006a0 2834 1990 0.11 0.008a0 2126 1990 0.57 0.010a0 1701 1990 2.31
DownLoad: CSV
-
[1] Chin C, Grimm R, Julienne P, Tiesinga E 2010 Rev. Mod. Phys. 82 1225
Google Scholar
[2] Vogels J M, Tsai C C, Freeland R S, Kokkelmans S J J M F, Verhaar B J, Heinzen D J 1997 Phys. Rev. A 56 R1067
Google Scholar
[3] Pellegrini P, Gacesa M, Côté R 2008 Phys. Rev. Lett. 101 053201
Google Scholar
[4] Giorgini S, Pitaevskii L P, Stringari S 2008 Rev. Mod. Phys. 80 1215
Google Scholar
[5] Gao B 1998 Phys. Rev. A 58 1728
Google Scholar
[6] Tiecke T G, Goosen M R, Walraven J T M, Kokkelmans S J J M F 2010 Phys. Rev. A 82 042712
Google Scholar
[7] Li Z, Madison K W 2009 Phys. Rev. A 79 042711
Google Scholar
[8] Johnson B R 1973 J. Comput. Phys. 13 445
Google Scholar
[9] Stechel E B, Walker R B, Light J C 1978 J. Chem. Phys. 69 3518
Google Scholar
[10] 李磐, 时雷, 毛庆和 2013 62 154205
Google Scholar
Li P, Shi L, Mao Q H 2013 Acta Phys. Sin. 62 154205
Google Scholar
[11] Dunn D, Grieves B 1989 J. Phys. A: Math. Gen. 22 L1093
Google Scholar
[12] Du M L 1993 Comput. Phys. Commun. 76 39
Google Scholar
[13] Johnson B R 1977 J. Chem. Phys. 67 4086
Google Scholar
[14] Colavecchia F D, Mrugala F, Parker G A, Pack R T 2003 J. Chem. Phys. 118 10387
Google Scholar
[15] Karman T, Janssen L M C, Sprenkels R, Groenenboom G C 2014 J. Chem. Phys. 141 064102
Google Scholar
[16] Blandon J, Park G A, Madrid C 2016 J. Phys. Chem. A 120 785
Google Scholar
[17] DeMille D 2002 Phys. Rev. Lett. 88 067901
Google Scholar
[18] Borsalino D, Vexiau R, Aymar M, Luc-Koening E, Dulieu O, Bouloufa-Maafa N 2016 J. Phys. B 49 055301
Google Scholar
[19] Gonzales-Sanchez L, Tacconi M, Bodo E, Gianturco F A 2008 Eur. Phys. J. D 49 85
Google Scholar
[20] Burke V M, Nobel C J 1995 Comput. Phys. Commun. 85 471
Google Scholar
[21] Gao B, Tiesinga E, Williams C J, Julienne P S 2005 Phys. Rev. A 72 042719
Google Scholar
[22] Patel H J, Blackley C L, Cornish S L, Hutson J M 2014 Phys. Rev. A 90 032716
Google Scholar
[23] Köppinger M P, Mccarron D J, Jenkin D L, Molony P K, Cho H W, Cornish S L, Ruth Le Sueur C, Blackley C L, Hutson J M 2014 Phys. Rev. A 89 033604
Google Scholar
[24] Ferber R, Nikolayeva O, Tamanis M, Knöckel H, Tiemann E 2013 Phys. Rev. A 88 012516
Google Scholar
[25] Gröbner M, Weinmann P, Kirilov E, Nägerl H C, Julienne P S, Ruth Le Sueur C, Hutson J M 2017 Phys. Rev. A 95 022715
Google Scholar
[26] D’Errico C, Zaccanti M, Fattori M, Roati G, Inguscio M, Modugno G, Simoni A 2007 New J. Phys. 9 223
Google Scholar
[27] Tiecke T G, Goosen M R, Ludewig A, Gensemer S D, Kraft S, Kokkelmans S J J M F, Walraven J T M 2010 Phys. Rev. Lett. 104 053202
Google Scholar
[28] Manolopoulos D E 1986 J. Chem. Phys. 85 6425
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3754
- PDF Downloads: 68
- Cited By: 0
