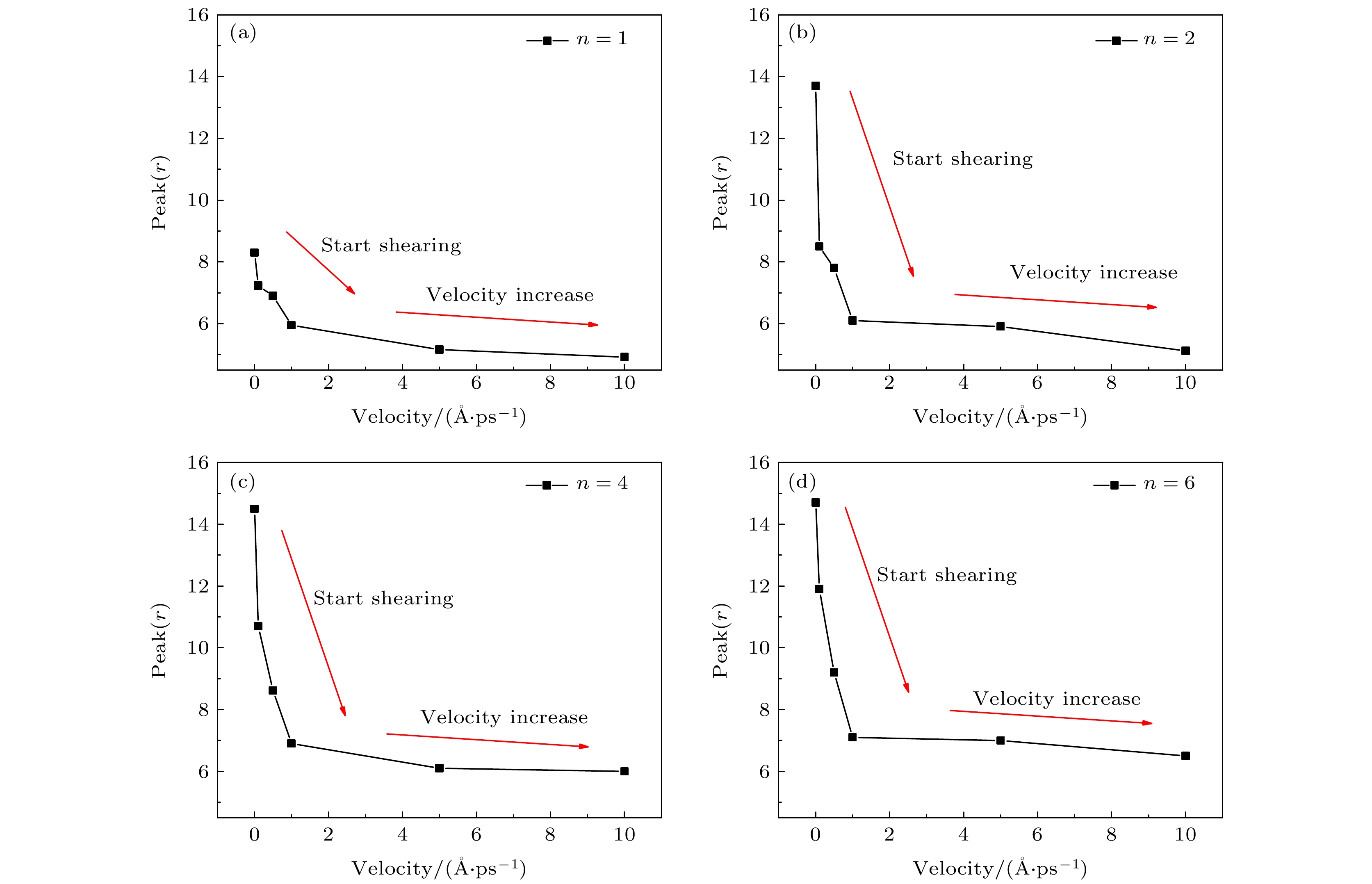
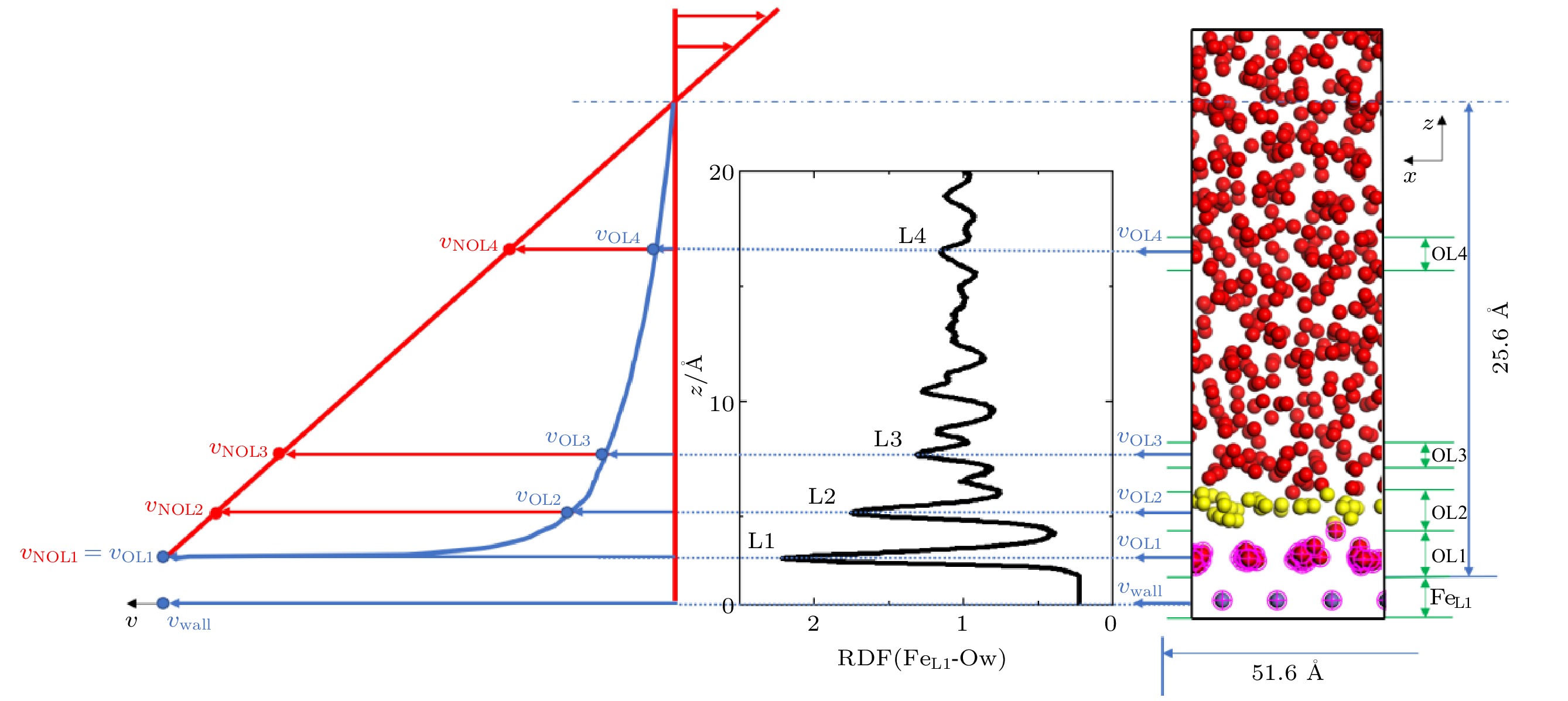
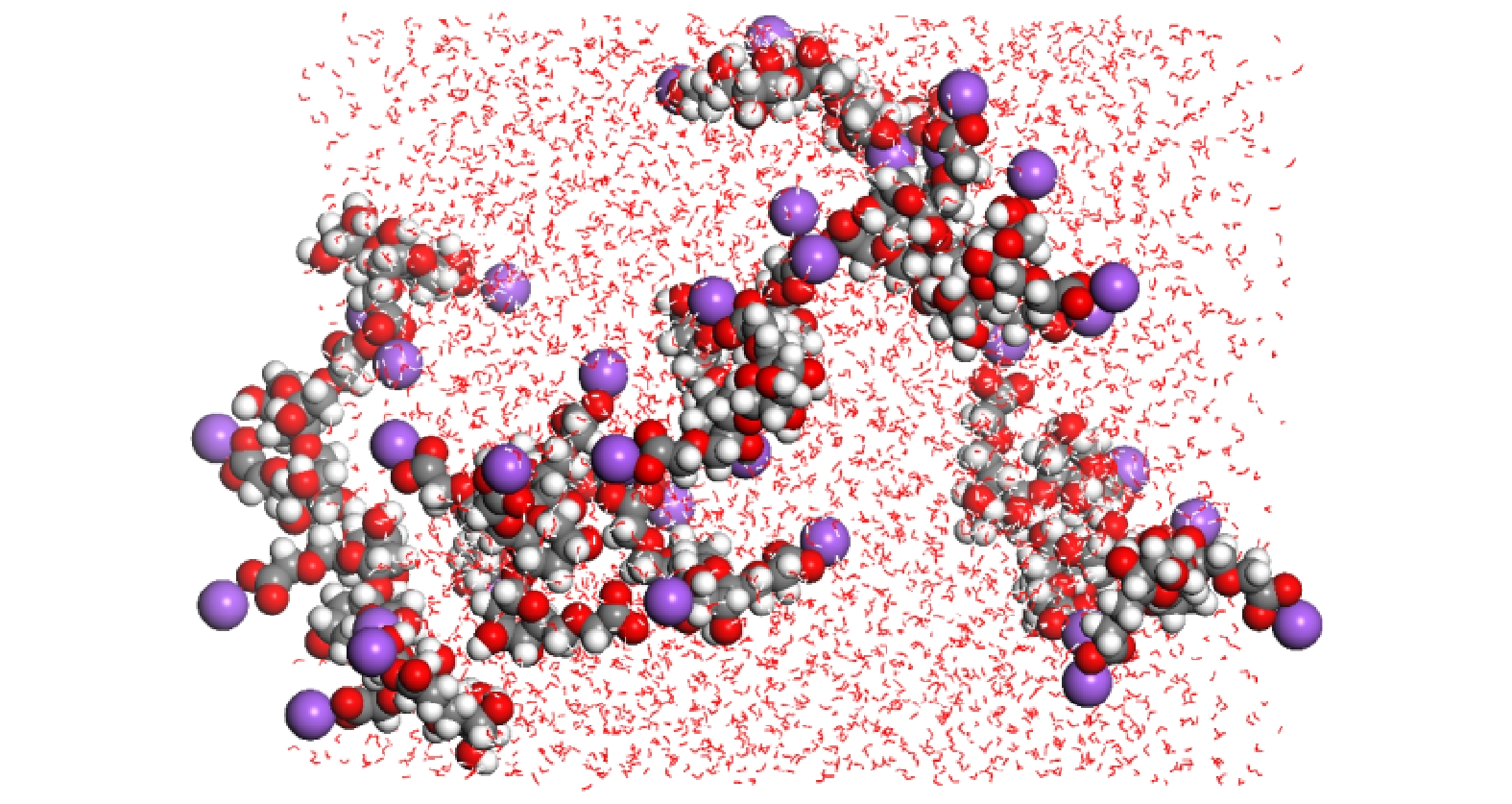
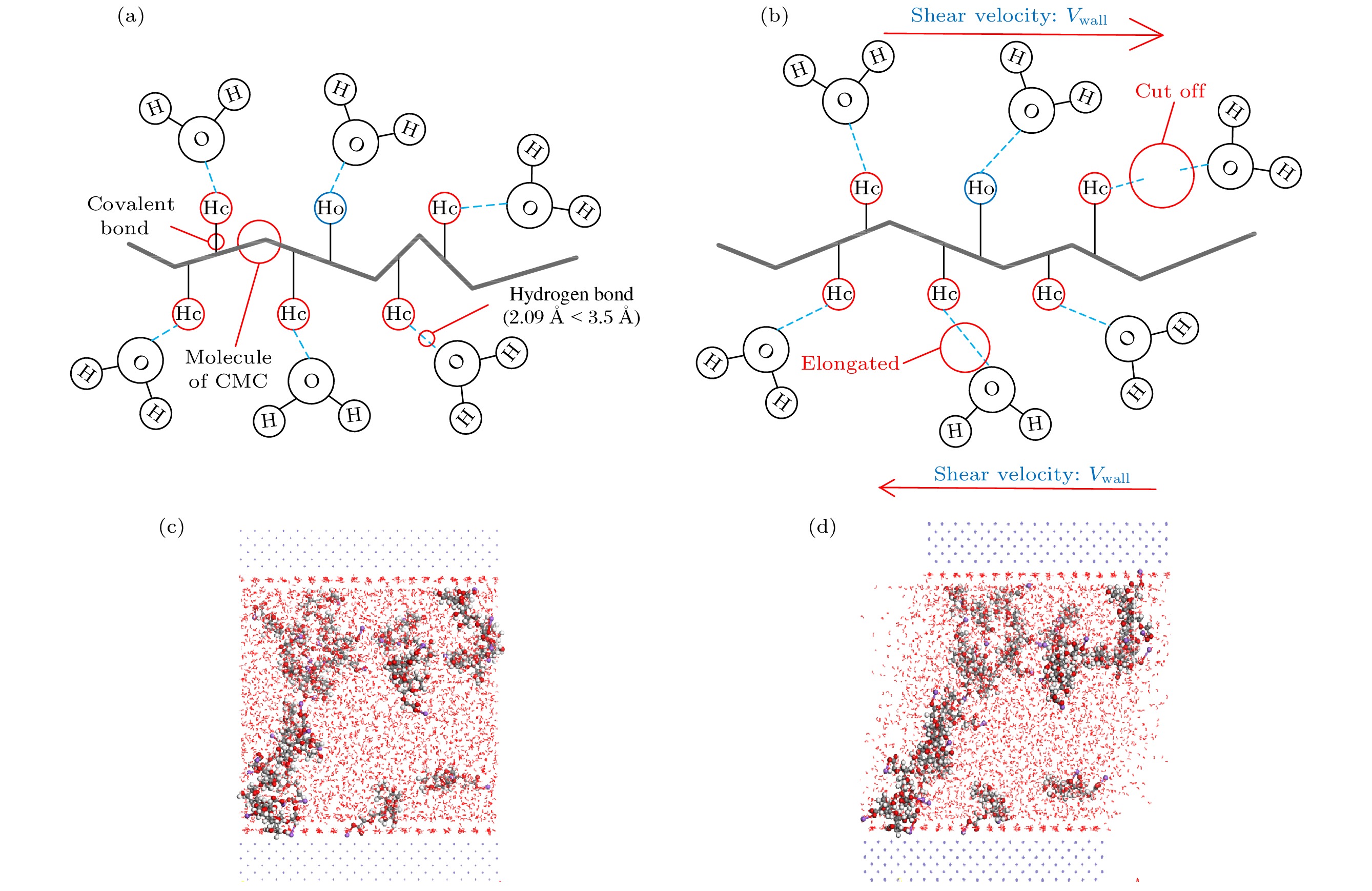
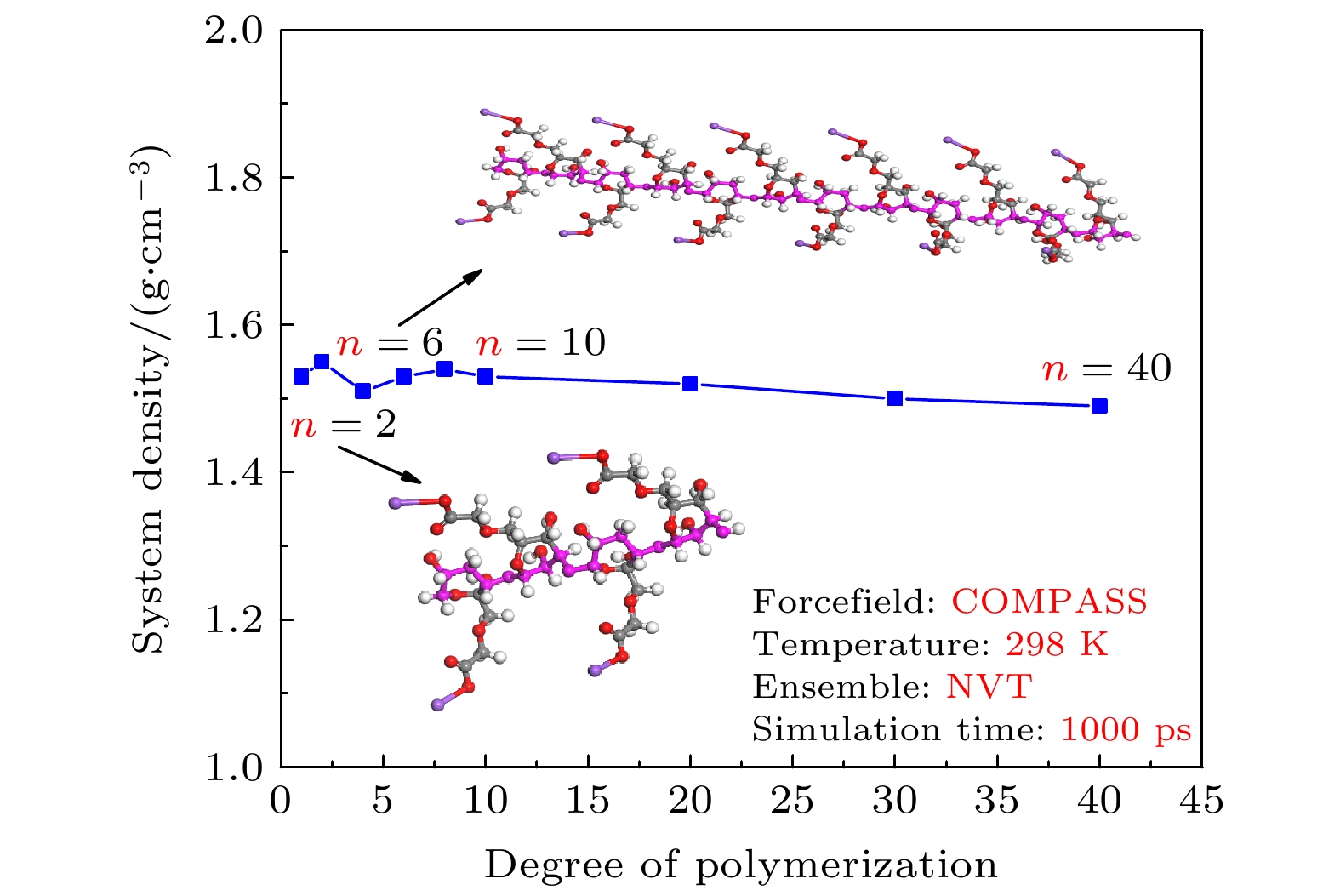
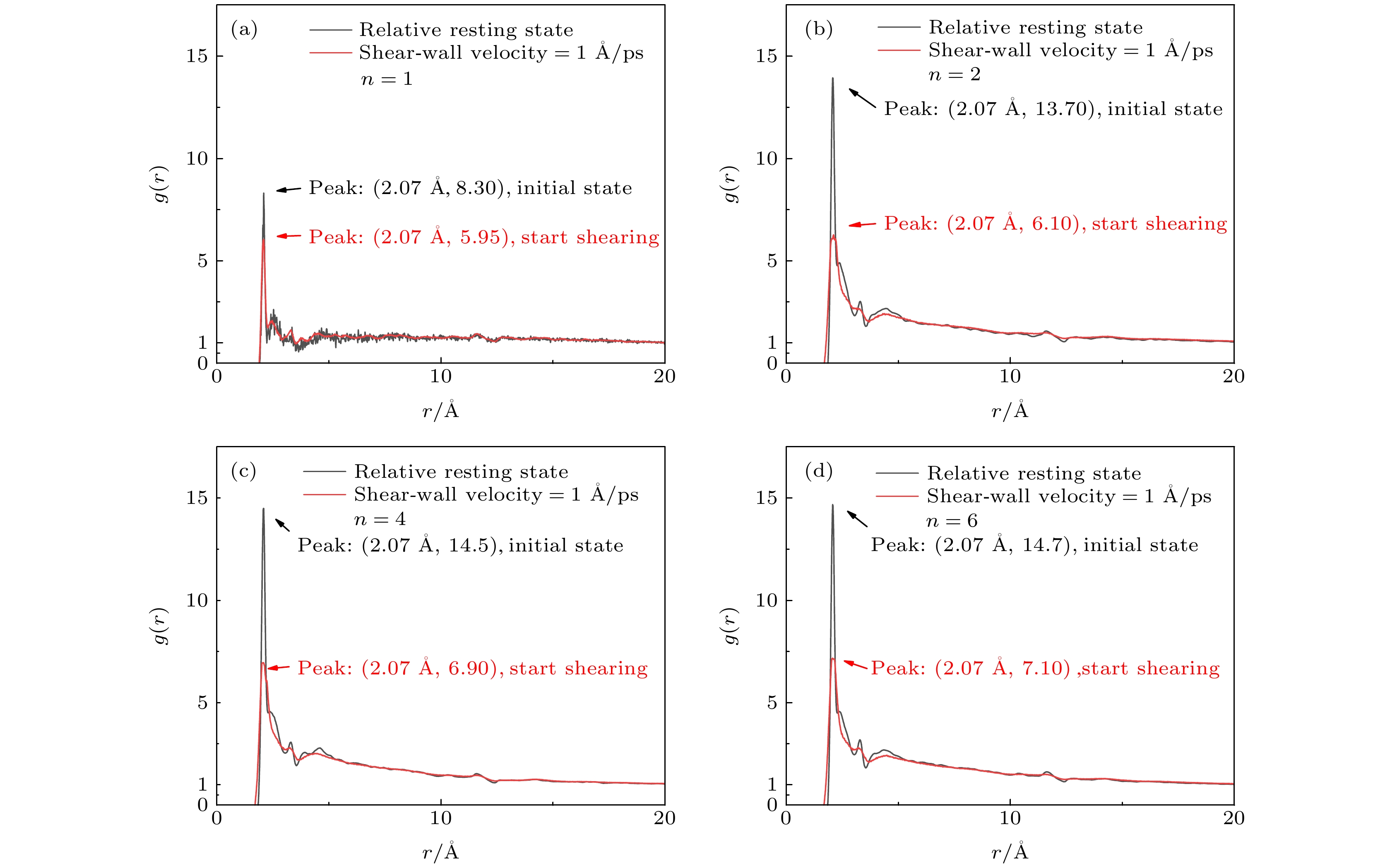
-
Non-Newtonian fluids are widely used in microfluidic systems and biomedical fields. In this paper, based on molecular dynamics simulation, taking the system composed of sodium carboxymethyl cellulose molecules and water molecules as the research object, the configuration evolutions of sodium carboxymethyl cellulose solution are simulated under different shear rates. Change of the solution viscosity is characterized by mean square displacement of sodium carboxymethyl cellulose molecules and the relative velocity between water layer and shear boundary. The effect of hydrogen bonding on the viscosity of the solution is analyzed emphatically. The results show that water molecules and solute molecules attract each other to form a more compact hydrogen bond network, which increases the viscosity of the solution; the peak value of the radial distribution function between the hydrogen atoms attached to carbon and the water oxygen atoms decreases when shear action is applied to the solution, and the hydrogen bond between the two atoms is weakened; the mobility of solute molecules increases and the blocking effect of water molecules on the movement of solute molecules weakens under the shear action; at the same time, the shorter the distance to the shear boundary, the closer to the shear velocity the velocity of water molecules is, and with the increase of distance, the velocity of water molecular layer decreases greatly. These results are macroscopically understood as the viscosity of the system decreasing. As the shear rate increases, the shear thinning of the sodium carboxymethyl cellulose solution becomes more significant.
-
Keywords:
- non-Newtonian fluids /
- molecular dynamics /
- shear thinning /
- hydrogen bonding
[1] Nair R, Choudhury A R 2020 Int. J. Biol. Macromol. 159 922
Google Scholar
[2] Ponalagusamy R 2018 Appl. Math. Comput. 337 545
Google Scholar
[3] 杨金姝 2014 农产品加工(学刊) 22 76
Google Scholar
Yang J S 2014 J. Process. Agric. Prod. 22 76
Google Scholar
[4] 吴淑茗, 柯萍萍, 黄俊祥, 陈梦霞, 许心怡, 王玮靖 2018 化学工程与装备 10 246
Google Scholar
Wu S M, Ke P P, Huang J X, Chen M X, Xu X Y, Wang W J 2018 J. Chem. Eng. Equip. 10 246
Google Scholar
[5] Jordi L, Josep S, Joan L 2007 Colloids Surf. A 301 8
Google Scholar
[6] Laporte M, Montillet A, Belkadi A, et al. 2020 Chem. Eng. Process. 148 107787
Google Scholar
[7] Khan M, Salahuddin T, Malik M Y, Khan F 2020 Physica A 547 123440
Google Scholar
[8] Roberts T G, Cox S J 2020 J. Non-Newton. Fluids Mech. 280 104278
Google Scholar
[9] Wu W W, Sun S L, Wang Z Z, Ding S 2019 Mech. Mater. 139 103187
Google Scholar
[10] Dong B, Zhang Y, Zhou X, Chen C, Li W 2019 Ther. Sci. Eng. Progr. 10 309
Google Scholar
[11] Afrouzi H, Ahmadian M, Moshfegh A, Toghraie D, Javadzadegan 2019 Physica A 535 122486
Google Scholar
[12] Blanco-Díaz E G, Castrejón-González E O, Alvarado J F, et al. 2017 J. Mol. Liq. 242 265
Google Scholar
[13] Esmaeili A, Haseli M 2017 Carbohydr. Polym. 173 645
Google Scholar
[14] Zhao Y, Xu Z M, Wang B B, He J J 2019 Int. J. Heat Mass Transfer 141 457
Google Scholar
[15] Reshma G, Reshmi C R, Shantikumar V N, Deepthy M 2020 Carbohydr. Polym. 248 116763
Google Scholar
[16] Sridevi S, Sutha S, Kavitha L, Gopi D 2020 Mater. Chem. Phys. 254 123455
Google Scholar
[17] Wang B, Cavallo D, Chen J B 2020 Polym. J. 210 123000
Google Scholar
[18] Castillo-Tejas J, Castrejón-González O, Carro S, et al. 2016 Colloids Surf. A 491 37
Google Scholar
[19] 张烨, 张冉, 常青, 李桦 2019 68 124702
Google Scholar
Zhang Y, Zhang R, Chang Q, Li H 2019 Acta Phys. Sin. 68 124702
Google Scholar
[20] Graham R S 2019 J. Rheol. 63 203
Google Scholar
[21] 洪迪昆, 刘亮, 郭欣 2015 中国电机工程学报 35 6099
Google Scholar
Hong D K, Liu L, Guo X 2015 Proc. CSEE 35 6099
Google Scholar
[22] 张跃 2007 计算材料学基础 (北京: 北京航空航天大学出版社) 第121页
Zhang Y 2007 Foundations of Computational Materials Science (Beijing: Beihang University Press) p121 (in Chinese)
[23] Kumar R, Schmidt J R, Skinner J L 2007 Chem. Phys. 126 204107
Google Scholar
-
图 1 CMC分子结构示意图
Figure 1. Molecular structure diagram of CMC.
图 2 CMC水溶液模型
Figure 2. Model of CMC aqueous solution.
图 3 剪切模拟模型 (a) 剪切运动前仿真系统示意图; (b) 剪切运动后仿真系统示意图; (c) 剪切运动前的仿真系统; (d) 剪切运动后的仿真系统
Figure 3. Shear simulation model: (a) System before shearing; (b) system after shearing; (c) simulating configuration before shearing; (d) simulating configuration after shearing.
图 4 分子能量最小化和分子结构示意图 (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6
Figure 4. Molecular energy minimization and molecular structure diagram: (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6.
图 5 CMC分子聚合度对体系密度的影响
Figure 5. Influence of CMC molecular polymerization degree on system density.
图 6 水分子和小范围CMC水溶液模型 (a) n = 0; (b) n = 1; (c) n = 2; (d) n = 4; (e) n = 6
Figure 6. Models of H2O molecule and small range CMC aqueous solution: (a) n = 0; (b) n = 1; (c) n = 2; (d) n = 4; (e) n = 6.
图 7 水分子数量对CMC溶液和纯水体系体积的影响
Figure 7. Influence of the number of water molecules on the volume of CMC solution and pure water system.
图 8 Hc-Ow径向分布函数
Figure 8. Hc-Ow radial distribution function.
图 9 开始剪切后Hc-Ow的径向分布函数与相对静止状态的对比 (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6
Figure 9. Comparison between the radial distribution of Hc-Ow and the relative static state after shearing: (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6.
图 10 Hc-Ow径向分布函数峰值随剪切速度的变化 (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6
Figure 10. Variation of the peak value of Hc-Ow radial distribution function with shear rate: (a) n = 1; (b) n = 2; (c) n = 4; (d) n = 6
图 11 剪切状态下溶液中分子的受力状态示意图
Figure 11. Stress state diagram of molecules in solution under shear state.
图 12 不同剪切速度下CMC分子的RMSDα曲线 (a) n = 1; (b) n = 6
Figure 12. RMSDα curves of CMC molecules at different shear rates: (a) n = 1; (b) n = 6.
图 13 水分子层的速度分布图及FeL1-Ow径向分布函数
Figure 13. Velocity distribution diagram of water molecular layer and FeL1-Ow radial distribution function.
-
[1] Nair R, Choudhury A R 2020 Int. J. Biol. Macromol. 159 922
Google Scholar
[2] Ponalagusamy R 2018 Appl. Math. Comput. 337 545
Google Scholar
[3] 杨金姝 2014 农产品加工(学刊) 22 76
Google Scholar
Yang J S 2014 J. Process. Agric. Prod. 22 76
Google Scholar
[4] 吴淑茗, 柯萍萍, 黄俊祥, 陈梦霞, 许心怡, 王玮靖 2018 化学工程与装备 10 246
Google Scholar
Wu S M, Ke P P, Huang J X, Chen M X, Xu X Y, Wang W J 2018 J. Chem. Eng. Equip. 10 246
Google Scholar
[5] Jordi L, Josep S, Joan L 2007 Colloids Surf. A 301 8
Google Scholar
[6] Laporte M, Montillet A, Belkadi A, et al. 2020 Chem. Eng. Process. 148 107787
Google Scholar
[7] Khan M, Salahuddin T, Malik M Y, Khan F 2020 Physica A 547 123440
Google Scholar
[8] Roberts T G, Cox S J 2020 J. Non-Newton. Fluids Mech. 280 104278
Google Scholar
[9] Wu W W, Sun S L, Wang Z Z, Ding S 2019 Mech. Mater. 139 103187
Google Scholar
[10] Dong B, Zhang Y, Zhou X, Chen C, Li W 2019 Ther. Sci. Eng. Progr. 10 309
Google Scholar
[11] Afrouzi H, Ahmadian M, Moshfegh A, Toghraie D, Javadzadegan 2019 Physica A 535 122486
Google Scholar
[12] Blanco-Díaz E G, Castrejón-González E O, Alvarado J F, et al. 2017 J. Mol. Liq. 242 265
Google Scholar
[13] Esmaeili A, Haseli M 2017 Carbohydr. Polym. 173 645
Google Scholar
[14] Zhao Y, Xu Z M, Wang B B, He J J 2019 Int. J. Heat Mass Transfer 141 457
Google Scholar
[15] Reshma G, Reshmi C R, Shantikumar V N, Deepthy M 2020 Carbohydr. Polym. 248 116763
Google Scholar
[16] Sridevi S, Sutha S, Kavitha L, Gopi D 2020 Mater. Chem. Phys. 254 123455
Google Scholar
[17] Wang B, Cavallo D, Chen J B 2020 Polym. J. 210 123000
Google Scholar
[18] Castillo-Tejas J, Castrejón-González O, Carro S, et al. 2016 Colloids Surf. A 491 37
Google Scholar
[19] 张烨, 张冉, 常青, 李桦 2019 68 124702
Google Scholar
Zhang Y, Zhang R, Chang Q, Li H 2019 Acta Phys. Sin. 68 124702
Google Scholar
[20] Graham R S 2019 J. Rheol. 63 203
Google Scholar
[21] 洪迪昆, 刘亮, 郭欣 2015 中国电机工程学报 35 6099
Google Scholar
Hong D K, Liu L, Guo X 2015 Proc. CSEE 35 6099
Google Scholar
[22] 张跃 2007 计算材料学基础 (北京: 北京航空航天大学出版社) 第121页
Zhang Y 2007 Foundations of Computational Materials Science (Beijing: Beihang University Press) p121 (in Chinese)
[23] Kumar R, Schmidt J R, Skinner J L 2007 Chem. Phys. 126 204107
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 10158
- PDF Downloads: 179
- Cited By: 0
