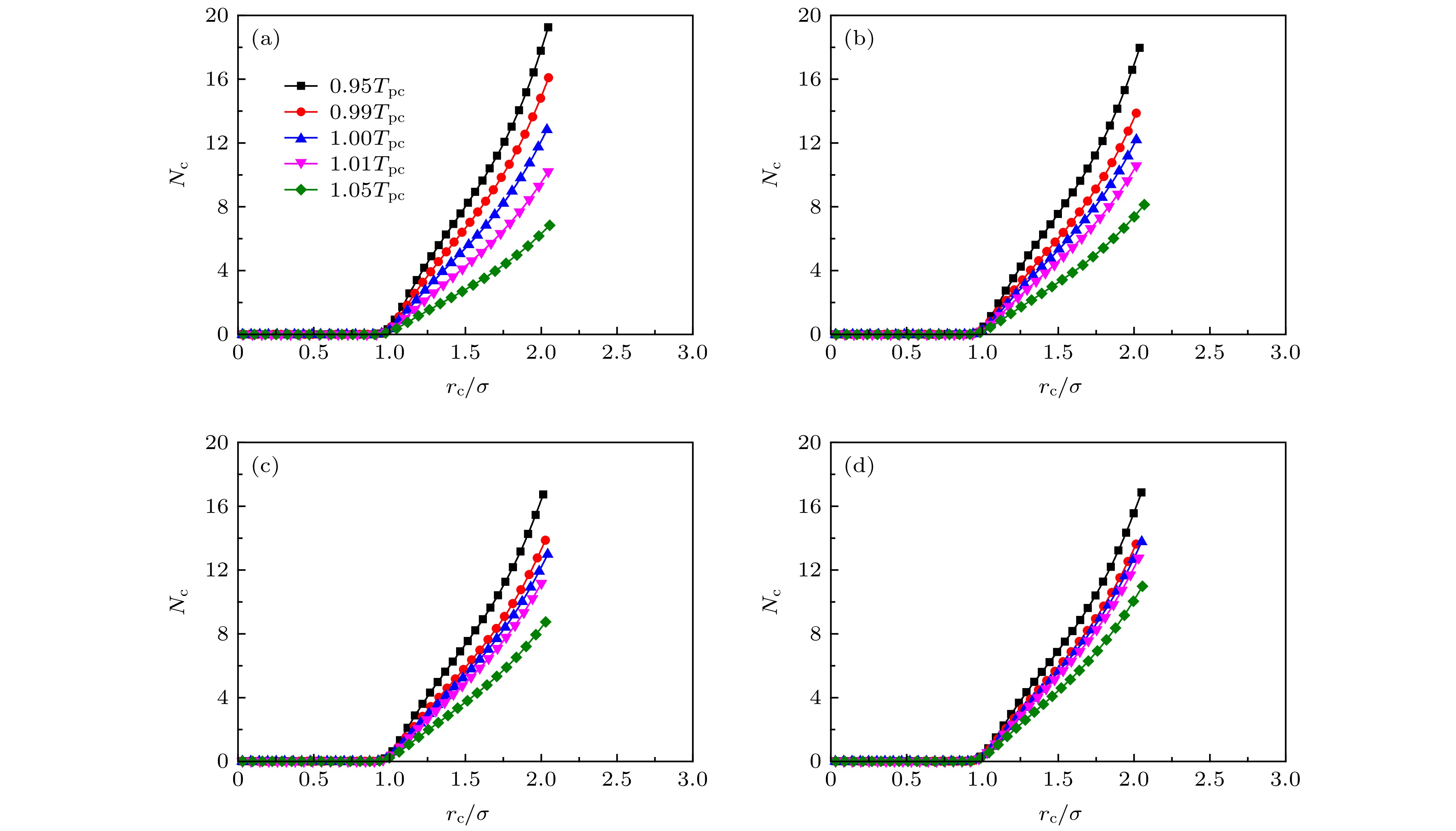
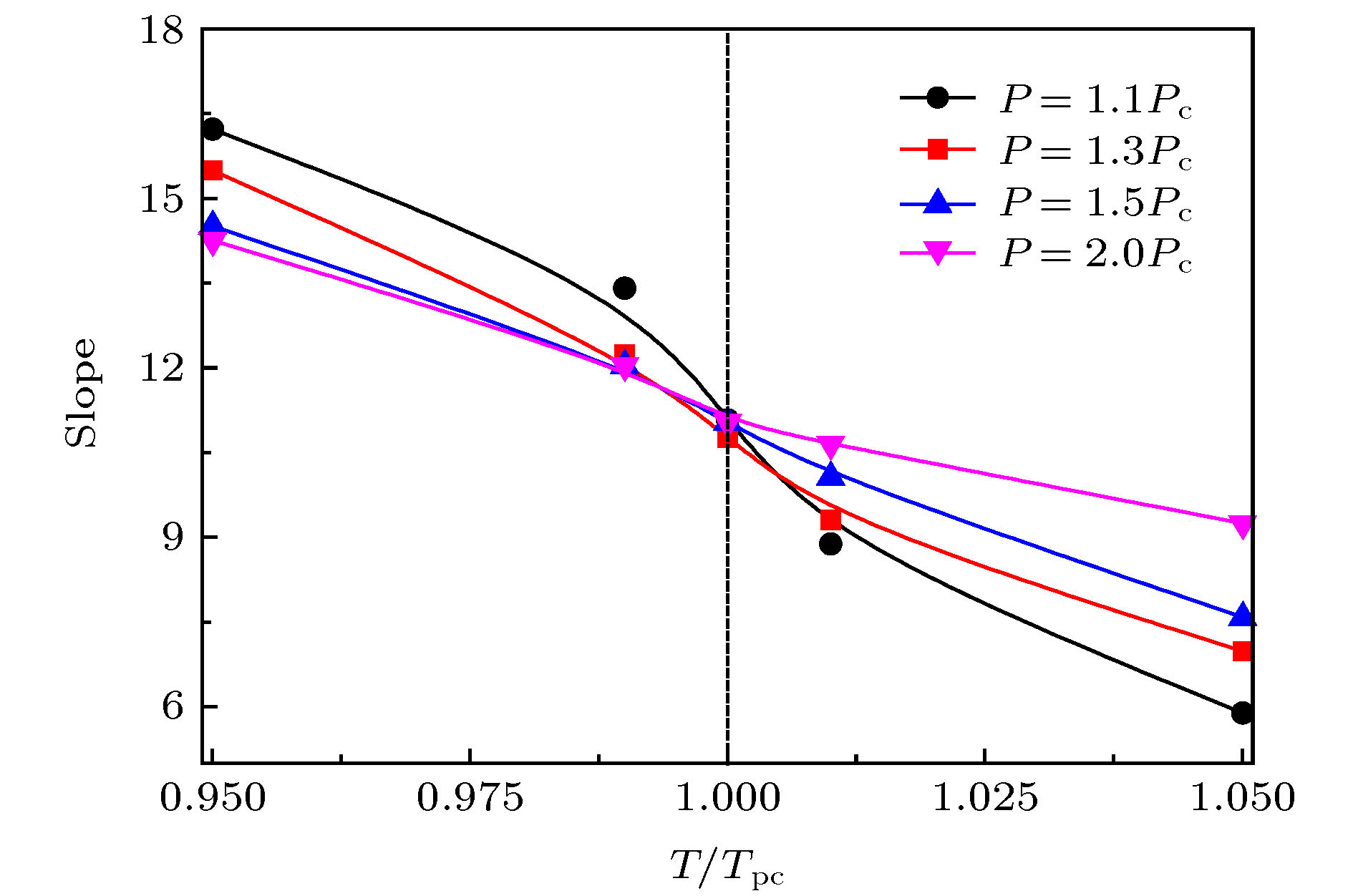
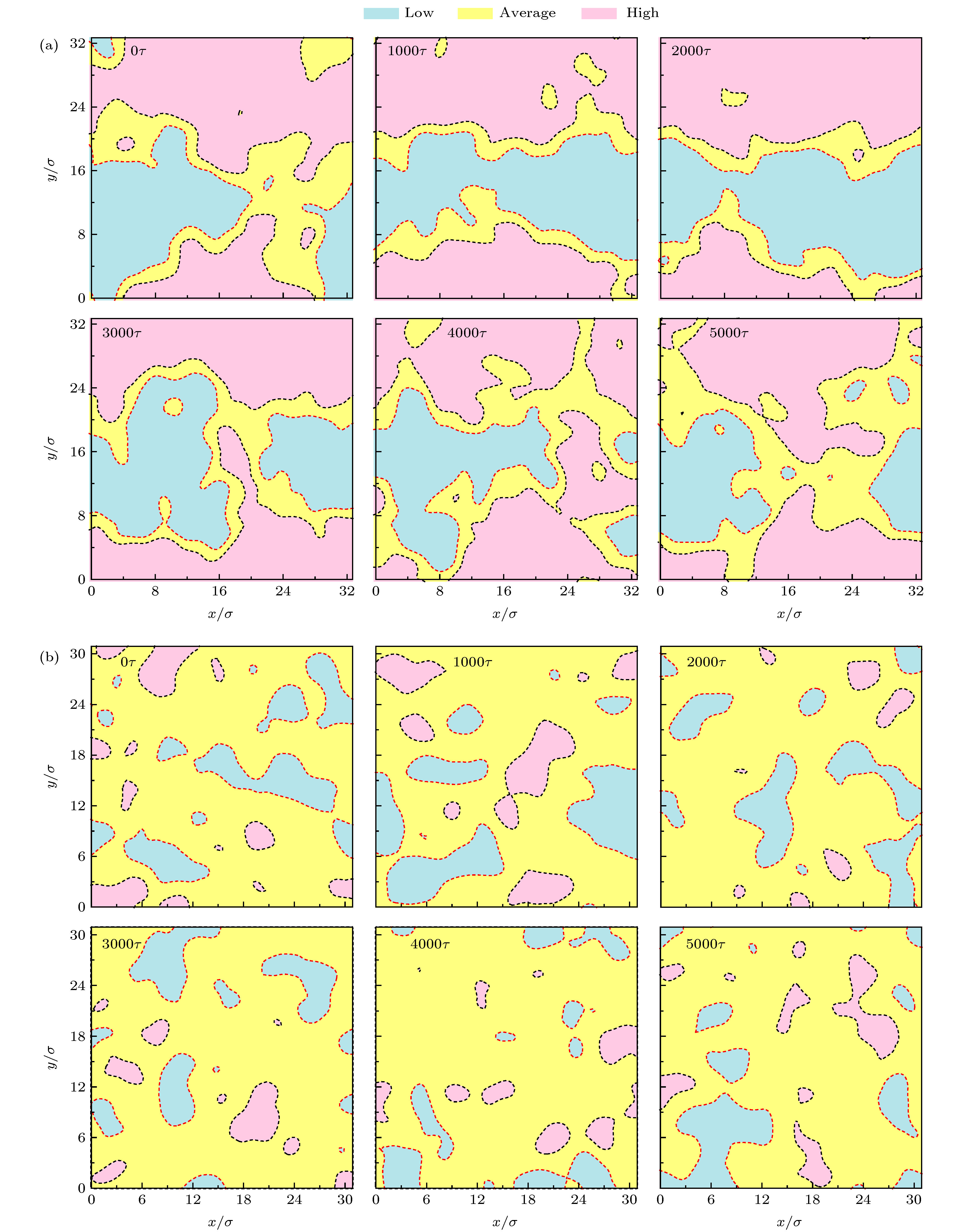
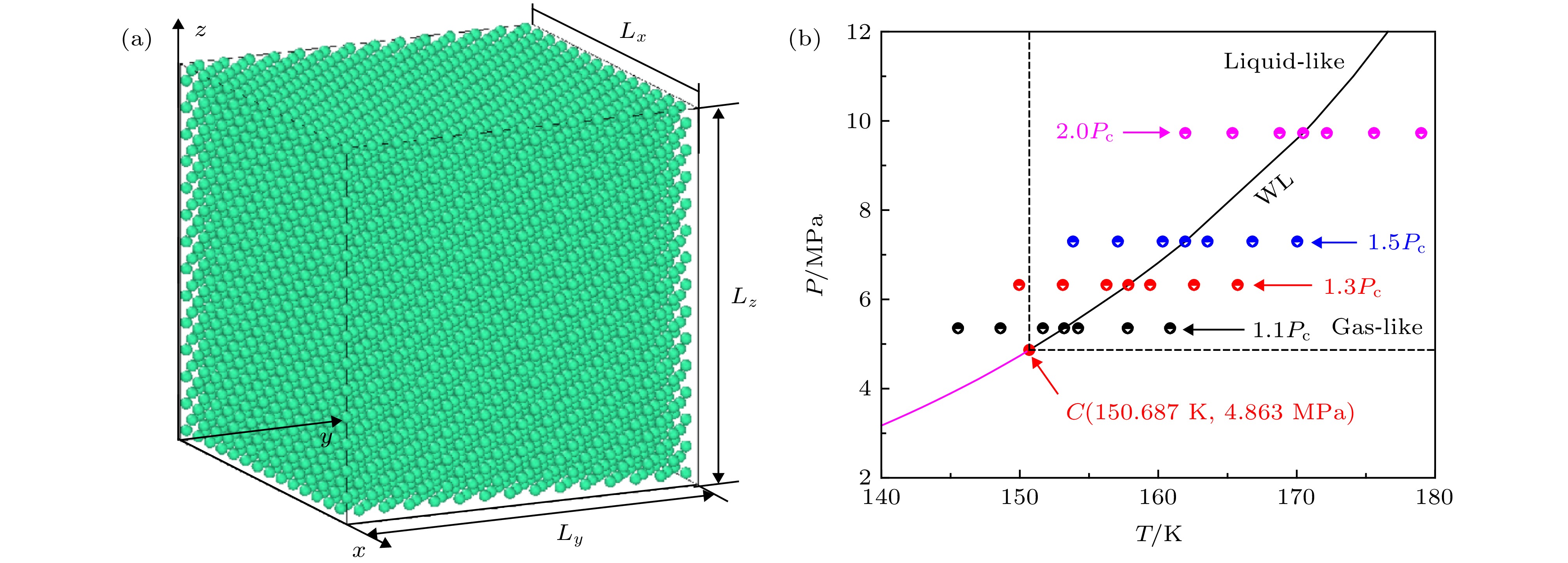
-
Supercritical fluids (SCF) have been widely utilized in the industrial processes, such as extraction, cleaning, drying, foaming and power generation driven by primary energy. Therefore, SCF have attracted more and more attention in recent years. At supercritical state, liquid, and gas phase are not clearly distinguished, but the thermal-physical properties of fluid show an interesting characteristic, especially near the pseudo-critical temperature. Thus, it is of great significant to study the structure and density time series evolution of SCF.Due to high pressure and temperature for SCF, it can be challenging to collect experimental data of SCF. However, the advantage of molecular dynamics simulation in convenience, safty and cost over experiments. Therefore, in this paper,molecular dynamics simulation was performed to investigate the fluid structure and density series fluctuation curves at supercritical state, and the influence of parameters varitation including pressure and temperature onstructural characteristics was analyzed. In the simulation system, more than 104 atoms and simple Lennard-Jones(LJ) supercritical fluids were contained. The radial distribution function(RDF), coordination number(CN), density time series curve and permutation entropy of fluids at different pressures and temperatures were calculated. At specified pressure, the position of the first peak value of RDF gradually moves to the right with the increase of temperature, and the trend weakens with the increase of pressure. CN shows a downward trend with the increase of pressure and the CN difference at different temperatures gradually decreases. Simultaneously, the CN distribution area becomes narrow with the increase of pressure. The high/low density region calibrated by CN is stable, concentrated and large area distribution at low pressure, and the average density region is small, with the increase of pressure, the area of high/low density region is only a size of a few molecular and fluctuates sharply with time, and the area of average region is constantly expanding. At relatively low pressure, the density time series curve shows the characteristic that both the fluctuation range and quasi-period are large at pseudo-critical temperature. Simultaneously, the permutation entropy obtained from the time series curve shows three cases: (i) at low pressure (P = 1.1Pc), the minimum permutation entropy is obtained under the temperature that is lower than pseudo-critical temperature, and the system has higher orderliness; (ii) at moderate pressure (P = 1.3Pc and 1.5Pc), the state points corresponding to minimum permutation entropy is consistent with that corresponding to the maximum of isothermal compression coefficient and (iii) at high pressure (P = 2.0Pc), the permutation entropy curve fluctuates slightly and remains basically on the horizontal line. The results provide reliable support for revealing the characteristics of SCF from the microscale, and also provide useful inspiration for the practical application of SCF.
-
Keywords:
- supercritical fluids /
- molecular dynamics /
- structure characteristics /
- permutation entropy
[1] Pena-Pereira F, Tobiszewski M 2017 Elsevier 155
[2] Carlès P 2010 J. Supercrit. Fluids 53 2
Google Scholar
[3] Raju M, BanutiD T, MaP C, Ihme M 2017 Sci. Rep.-UK 7 3027
Google Scholar
[4] Artemenko S, Krijgsman P, Mazur V 2017 J. Mol. Liq. 238 122
Google Scholar
[5] Brazhkin V V, Fomin Y D, Lyapin A G, Lyapin V V, Ryzhov V N, TsiokE N, Trachenko Kostya 2013 Phys. Rev. Lett. 111 145901
Google Scholar
[6] Fomin Y D, Ryzhov V N, Tsiok E N, Brazhkin V V 2015 Sci.Rep.-UK 5 14234
Google Scholar
[7] Banuti D T, Raju M, Ihme M 2017 Cent. Turbul. Res. Annu. Res. Briefs 165
[8] Banuti D T 2015 J.Supercrit.Fluids. 98 12
Google Scholar
[9] Raman A S, Li H, Chiew Y C 2018 J. Chem. Phys. 148 014502
Google Scholar
[10] Nichele J, Abreu C R A, Alves L S B, Jr I B 2018 J.Supercrit.Fluids. 135 225
Google Scholar
[11] Nichele J, de Oliveira A B, Alves L S B, Borges I 2017 J. Mol.Liq. 237 65
Google Scholar
[12] Egorov S A 2002 Chem. Phys. Lett. 354 140
Google Scholar
[13] Skarmoutsos I, Samios J 2007 J. Chem. Phys. 126 044503
Google Scholar
[14] Skarmoutsos I, Samios J 2006 J. Phys. Chem. B 110 21931
Google Scholar
[15] Yoshii N, Okazaki S 1998 Fluid PhaseEquilib. 144 225
[16] Yoshii N, Okazaki S 1997 J. Chem. Phys. 107 2020
Google Scholar
[17] Metatla N, Lafond F, Jay-Gerin J P, Soldera A 2016 Rsc. Adv. 6 30484
Google Scholar
[18] Maddox M W, Goodyear G, Tucker S C 2000 J. Phys. Chem. B 104 6248
Google Scholar
[19] Yamane A, Shimojo F, Hoshino K 2006 J. Phys. Soc.Jpn. 75 124602
Google Scholar
[20] Nishikawa K, Arai A A, Morita T 2004 J.Supercrit.Fluids. 30 249
Google Scholar
[21] Nishikawa K, Ochiai H, Saitow K, Morita T 2003 Chem. Phys. 286 421
Google Scholar
[22] Cabaço M I, Besnard M, Tassaing T, Danten Y 2004 Pure Appl. Chem. 76 141
Google Scholar
[23] Arai A A, Morita T, Nishikawa K 2007 Fluid Phase Equilibria. 252 114
Google Scholar
[24] Ghosh K, Krishnamurthy C V 2018 Phys. Rev. E 97 012131
[25] 陈正隆, 徐为人, 汤立达 2007 分子模拟的理论与实践 (北京: 化学工业出版社) 第110−112页
Chen Z L, Xu W R, Tang L D 2007 (Beijing: Chemical Industry Press) pp110−112 (in Chinese)
[26] Bolmatov D, Brazhkin V V, Fomin Y D, Ryzhov V N, Trachenko K 2013 J. Chem. Phys. 139 234501
Google Scholar
[27] 吴方棣, 郑辉东, 刘俊劭, 郑细鸣 2014 辽宁石油化工大学学报 34 8
Google Scholar
Wu F K, Zheng H D, Liu J X, Zheng X M 2014 Journal of Liaoning Shihua University 34 8
Google Scholar
[28] Skarmoutsos I, Guardia E, Samios J 2017 J.Supercrit.Fluids. 130 156
Google Scholar
[29] 计伟荣 1993 浙江工业大学学报 59 1
Ji W R 1993 Journal of Zhejiang University of Technology 59 1
[30] Martinez H L, Ravi R, Tucker S C 1996 J. Chem. Phys. 104 1067
Google Scholar
[31] 于渌, 郝柏林, 陈晓松 2016 边缘奇迹:相变和临界现象 (北京: 科学出版社) 第81−93页
Yu L, Hao B L, Chen X S 2016 (Beijing: Science Press) pp81−93 (in Chinese)
[32] March N H, Tosi M P 2002 (Singapore: World Scientific) pp75−80
[33] Bandt C, Pompe B 2002 Phys. Rev. Lett. 88 174102
Google Scholar
-
图 1 (a) 物理模型图; (b) 模拟状态点在相图中的分布
Figure 1. (a) Physical model of system; (b) simulation points on phase diagram with Widom line, liquid-like and gas-like region.
图 2 (a) 定压比热容(cp)变化曲线; (b) 等温压缩系数(kT)变化曲线
Figure 2. (a) The curve of cp under different pressures; (b) the curve of kT under different pressures.
图 3 径向分布函数 (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc
Figure 3. Radial distribution function: (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc.
图 4 配位数 (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc
Figure 4. Coordination number: (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc.
图 5 配位数曲线斜率
Figure 5. The slope of coordination number curve.
图 6 流体在xy平面内高/低密度区分布随时间的演化 (a) P = 1.1Pc, T = Tpc; (b) P = 2.0Pc, T = Tpc
Figure 6. Liqud atoms evolution over the xy plane with different pressure: (a) P = 1.1Pc, T = Tpc; (b) P = 2.0Pc, T = Tpc.
图 7 不同压力下, 拟临界点温度下高/低密度区占比 (a) 高密度区占比; (b) 低密度区占比
Figure 7. The ratio of high/low density region at pseudo-critical point temperature under different pressure: (a) The ratio of high density region; (b) the ratio of low density region.
图 8 静态结构因子 (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc
Figure 8. Static structure fator: (a) P = 1.1Pc; (b) P = 1.3Pc; (c) P = 1.5Pc; (d) P = 2.0Pc.
图 9 (a) 密度时间序列曲线 ( T = Tpc); (b) 排列熵
Figure 9. (a) Time series of density for T = Tpc; (b) permutation entropy.
-
[1] Pena-Pereira F, Tobiszewski M 2017 Elsevier 155
[2] Carlès P 2010 J. Supercrit. Fluids 53 2
Google Scholar
[3] Raju M, BanutiD T, MaP C, Ihme M 2017 Sci. Rep.-UK 7 3027
Google Scholar
[4] Artemenko S, Krijgsman P, Mazur V 2017 J. Mol. Liq. 238 122
Google Scholar
[5] Brazhkin V V, Fomin Y D, Lyapin A G, Lyapin V V, Ryzhov V N, TsiokE N, Trachenko Kostya 2013 Phys. Rev. Lett. 111 145901
Google Scholar
[6] Fomin Y D, Ryzhov V N, Tsiok E N, Brazhkin V V 2015 Sci.Rep.-UK 5 14234
Google Scholar
[7] Banuti D T, Raju M, Ihme M 2017 Cent. Turbul. Res. Annu. Res. Briefs 165
[8] Banuti D T 2015 J.Supercrit.Fluids. 98 12
Google Scholar
[9] Raman A S, Li H, Chiew Y C 2018 J. Chem. Phys. 148 014502
Google Scholar
[10] Nichele J, Abreu C R A, Alves L S B, Jr I B 2018 J.Supercrit.Fluids. 135 225
Google Scholar
[11] Nichele J, de Oliveira A B, Alves L S B, Borges I 2017 J. Mol.Liq. 237 65
Google Scholar
[12] Egorov S A 2002 Chem. Phys. Lett. 354 140
Google Scholar
[13] Skarmoutsos I, Samios J 2007 J. Chem. Phys. 126 044503
Google Scholar
[14] Skarmoutsos I, Samios J 2006 J. Phys. Chem. B 110 21931
Google Scholar
[15] Yoshii N, Okazaki S 1998 Fluid PhaseEquilib. 144 225
[16] Yoshii N, Okazaki S 1997 J. Chem. Phys. 107 2020
Google Scholar
[17] Metatla N, Lafond F, Jay-Gerin J P, Soldera A 2016 Rsc. Adv. 6 30484
Google Scholar
[18] Maddox M W, Goodyear G, Tucker S C 2000 J. Phys. Chem. B 104 6248
Google Scholar
[19] Yamane A, Shimojo F, Hoshino K 2006 J. Phys. Soc.Jpn. 75 124602
Google Scholar
[20] Nishikawa K, Arai A A, Morita T 2004 J.Supercrit.Fluids. 30 249
Google Scholar
[21] Nishikawa K, Ochiai H, Saitow K, Morita T 2003 Chem. Phys. 286 421
Google Scholar
[22] Cabaço M I, Besnard M, Tassaing T, Danten Y 2004 Pure Appl. Chem. 76 141
Google Scholar
[23] Arai A A, Morita T, Nishikawa K 2007 Fluid Phase Equilibria. 252 114
Google Scholar
[24] Ghosh K, Krishnamurthy C V 2018 Phys. Rev. E 97 012131
[25] 陈正隆, 徐为人, 汤立达 2007 分子模拟的理论与实践 (北京: 化学工业出版社) 第110−112页
Chen Z L, Xu W R, Tang L D 2007 (Beijing: Chemical Industry Press) pp110−112 (in Chinese)
[26] Bolmatov D, Brazhkin V V, Fomin Y D, Ryzhov V N, Trachenko K 2013 J. Chem. Phys. 139 234501
Google Scholar
[27] 吴方棣, 郑辉东, 刘俊劭, 郑细鸣 2014 辽宁石油化工大学学报 34 8
Google Scholar
Wu F K, Zheng H D, Liu J X, Zheng X M 2014 Journal of Liaoning Shihua University 34 8
Google Scholar
[28] Skarmoutsos I, Guardia E, Samios J 2017 J.Supercrit.Fluids. 130 156
Google Scholar
[29] 计伟荣 1993 浙江工业大学学报 59 1
Ji W R 1993 Journal of Zhejiang University of Technology 59 1
[30] Martinez H L, Ravi R, Tucker S C 1996 J. Chem. Phys. 104 1067
Google Scholar
[31] 于渌, 郝柏林, 陈晓松 2016 边缘奇迹:相变和临界现象 (北京: 科学出版社) 第81−93页
Yu L, Hao B L, Chen X S 2016 (Beijing: Science Press) pp81−93 (in Chinese)
[32] March N H, Tosi M P 2002 (Singapore: World Scientific) pp75−80
[33] Bandt C, Pompe B 2002 Phys. Rev. Lett. 88 174102
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 10133
- PDF Downloads: 177
- Cited By: 0
