Transmission time and spin polarization for electron in magnetically confined semiconducotr nanostructure modulated by spin-orbit coupling

Wen Li; Lu Mao-Wang; Chen Jia-Li; Chen Sai-Yan; Cao Xue-Li; Zhang An-Qi

doi:10.7498/aps.73.20240285

Abstract

Because digital information in semiconductor spintronics is encoded, stored, processed, and transferred by electron spins instead of its charge, the operation of a spintronic device requires that electrons in semiconductors be spin polarized. But spin states of electrons in traditional semiconductor materials are usually degenerate, therefore, conventional semiconductors cannot be directly used to design spintronic devices. Thus, how to spin polarized electrons in ordinary semiconductors (also called spin injection) including its effective manipulation, has become an important direction of research. In physics, either Zeeman effect between electron spins and external magnetic fields or spin-orbit coupling of electron spins and its spatial momentums can be employed to achieve electron-spin polarization. According to these physical mechanisms, some effective schemes have been developed successfully, such as spin filtering, temporally separating electron-spins, and spatial separations of electron spins. Utilizing the combination of theoretical analysis and numerical calculation, transmission time is investigated by considering both Zeeman effect as well as Rashba and Dresselhaus spin-orbit couplings for electron in magnetically confined semiconductor nanostructure, which is constructed on the GaAs/Al_xGa_1–xAs heterostructure. Schrödinger equation of an electron is numerically solved by matrix diagonalization and improved transfer-matrix method. Adopting H.G. Winful’s theory, dwell time of electron is calculated and spin polarization ratio is given. Due to Zeeman effect and spin-orbit coupling, dwell time of electron is obviously associated with the spins, which is used to separate electron-spins in time dimension and to realize spin polarization of electrons in semiconductors. Because the semiconductor GaAs has a small effective g-factor, which is about 4 times larger than that induced by Zeeman effect, electron-spin polarization originates mainly from spin-orbit coupling including Rashba and Dresselhaus types. Dwell time of electron and its spin polarization can be efficaciously modified by interfacial confining electric-field or strain engineering, because the effective potential of electron is related to spin-orbit coupling’s strength. These interesting findings not only have some references for spin injection into semiconductors, but also provide a controllable temporal electron-spin splitter for semiconductor spintronics device applications.

Keywords:

semiconductor spintronics /
magnetically confined semiconductor nanostructure /
spin-orbit coupling /
dwell time

Authors and contacts

College of Physics and Electronic Information Engineering, Guilin University of Technology, Guilin 541004, China

Corresponding author: Lu Mao-Wang, maowanglu@glut.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11864009, 62164005) and the Guangxi Natural Science Foundation of China (Grant No. 2021JJB110053).

FullText HTML : translate this paragraph

References

[1]	Wolf S A, Awschalom D D, Buhrman R A, Daughton J M, von Molnár S, Roukes M L, Chtchelkanova A Y, Treger D M 2001 Science 294 1488 Google Scholar
[2]	Žutíc I, Fabiam J, Sarma S Das 2004 Rev. Mod. Phys. 76 323 Google Scholar
[3]	Gong C, Zhang X 2019 Science 363 706 Google Scholar
[4]	Soumyanrayanan A, Reyren N, Fert A, Panagopoulos C 2016 Nature 539 509 Google Scholar
[5]	蒋龙兴, 李庆超, 张旭, 李京峰, 张静, 陈祖信, 曾敏, 吴昊 2024 73 017505 Google Scholar Jiang L X, Li Q C, Zhang X, Li J F, Zhang J, Chen Z X, Zeng M, Wu H 2024 Acta Phys. Sin. 73 017505 Google Scholar
[6]	Gurram M, Omar S, Wees B J V 2017 Nat. Commun. 8 248 Google Scholar
[7]	Zhu W K, Lin H L, Yan F G, Hu C, Wang Z A, Zhao L X, Deng Y C, Kudrynskyi Z R, Zhou T, Kovalyuk Z D, Zheng Y H, Patanè A, Žutić I, Li S S, Zheng H Z, Wang K Y 2021 Adv. Mater. 33 2104658 Google Scholar
[8]	Zhu W K, Xie S H, Lin H L, Zhang G J, Wu H, Hu T G, Wang Z A, Zhang X M, Xu J H, Wang Y J, Zheng Y H, Yan F G, Zhang J, Zhao L X, Patanè A, Zhang J, Chang H X, Wang K Y 2022 Chin. Phys. Lett. 39 128501 Google Scholar
[9]	Zhu W K, Zhu J M, Zhou T, Zhang X P, Lin H L, Cui Q R, Yan F G, Wang Z A, Deng Y C, Yang H X, Zhao L X, Žutić I, Belashchenko K D, Wang K Y 2023 Nat. Commun. 14 5371 Google Scholar
[10]	Kitchen D, Richardella A, Tang J M, Flatté M E, Yazdani A 2006 Nature 442 436 Google Scholar
[11]	贺亚萍, 陈明霞, 潘杰锋, 李冬, 林港钧, 黄新红 2023 72 028503 Google Scholar He Y P, Chen M X, Pan J F, Li D, Lin G J, Huang X H 2023 Acta Phys. Sin. 72 028503 Google Scholar
[12]	李春雷, 郑军, 王小明, 徐燕 2023 72 227201 Google Scholar Li C L, Zheng J, Wang X M, Xu Y 2023 Acta Phys. Sin. 72 227201 Google Scholar
[13]	Liu X H, Zhang G L, Kong Y H, Li A H, Fu X 2014 Appl. Surf. Sci. 313 545 Google Scholar
[14]	Wang L, Guo Y 2006 Phys. Rev. B 73 205311 Google Scholar
[15]	Das S, Ghosh S, Kumar R, Bag A, Biswas D 2017 IEEE Trans. Electron Devices 64 4650 Google Scholar
[16]	Kong Y H, Liu X H, Li A H, Gong Y J 2019 Vacuum 159 410 Google Scholar
[17]	Nogaret A 2010 J. Phys. Condens. Matter 22 253201 Google Scholar
[18]	Hauge E H, Støvneng J A 1989 Rev. Mod. Phys. 61 917 Google Scholar
[19]	王瑞琴, 宫箭, 武建英, 陈军 2013 62 087303 Google Scholar Wang R Q, Gong J, Wu J Y, Chen J 2013 Acta Phys. Sin. 62 087303 Google Scholar
[20]	Winful H G 2003 Phys. Rev. Lett. 91 260401 Google Scholar
[21]	Zhai F, Guo Y, Gu B L 2002 Eur. Phys. J. B 29 147 Google Scholar
[22]	Xu H Z, Liu P J, Zhang Y F 2003 Phys. Status Solidi B 240 169 Google Scholar
[23]	Chen S Y, Zhang G L, Cao X L, Peng F F 2021 J. Comput. Electron. 20 785 Google Scholar
[24]	Zhang G L, Lu M W, Chen S Y, Peng F F, Meng J S 2021 IEEE Trans. Magn. 57 1400305
[25]	Guo Q M, Lu M W, Huang X H, Yang S Q, Qin Y J 2021 Vacuum 186 110059 Google Scholar
[26]	Xie S S, Lu M W, Huang X H, Wen L, Chen J L 2023 Phys. Lett. A 480 128976 Google Scholar
[27]	Guo Q M, Lu M W, Yang S Q, Qin Y J, Xie S S 2022 Braz. J. Phys. 52 74 Google Scholar
[28]	Lu M W, Chen S Y, Cao X L , Huang X H 2021 IEEE Trans. Electron Devices 68 860
[29]	Capasso F, Mohammed K, Cho A Y, Hull R, Hutchinson A L 1985 Appl. Phys. Lett. 47 420 Google Scholar
[30]	Lu M W, Chen S Y, Cao X L, Huang X H 2020 Results Phys. 19 103375 Google Scholar
[31]	Chen S Y, Cao X L, Huang X H, Lu M W 2023 Eur. Phys. J. Plus 138 111 Google Scholar
[32]	Xie S S, Lu M W, Huang X H, Wen L, Chen J L 2023 Results Phys. 51 106605 Google Scholar
[33]	Guo Q M, Chen S Y, Cao X L, Yang S Q 2021 Semicond. Sci. Technol. 36 055013 Google Scholar
[34]	Guo Q M, Lu M W, Yang S Q, Qin Y J, Xie S S 2022 J. Nanoelectron. Optoe. 16 1554
[35]	Chen S Y, Lu M W, Cao X L 2022 Chin. Phys. B 31 017201 Google Scholar
[36]	Lu M W, Chen S Y, Zhang G L, Huang X H 2018 IEEE Trans. Electron Devices 65 3045 Google Scholar
[37]	Rashba E I, Efros A L 2003 Phys. Rev. Lett. 91 126405 Google Scholar
[38]	Schliemann J, Loss D 2003 Phys. Rev. B 68 165311 Google Scholar
[39]	Bindel J R, Pezzotta M, Ulrich J, Liebmamm M, sheman E Y, Morgenstern M 2016 Nat. Phys. 12 920 Google Scholar
[40]	Intronati G A, Tamborenea P I, Weinmann D A, Jarabert R A 2012 Phys. Rev. Lett. 108 016601 Google Scholar
[41]	Xie S S, Lu M W, Chen S Y, Qin Y J, Wen L, Chen J L 2023 Commun. Theor. Phys. 75 015703 Google Scholar
[42]	von Bergmann K, Heinze S, Bode M, Vedmedenko E Y, Bihlmayer G, Blügel S, Wiesendanger R 2006 Phys. Rev. Lett. 96 167203 Google Scholar
[43]	Lu M W, Chen S Y, Zhang G L 2017 IEEE Trans. Electron Devices 64 1825 Google Scholar
[44]	You J Q, Zhang L D, Ghosh P K 1995 Phys. Rev. B 52 17243 Google Scholar
[45]	Lu M W, Chen S Y, Zhang G L, Huang X H 2018 J. Phys. Condens. Matter 30 145302 Google Scholar
[46]	Lu K Y, He Z Y, Zu M M, Guo S Y 2022 IEEE Electron Device Lett. 43 1645 Google Scholar
[47]	Lu K Y, He Z Y, Zu M M, Guo S Y, Lu M W 2023 IEEE Electron Device Lett. 44 1424 Google Scholar
[48]	Rusetsky V S, Golyashov V A, Eremeev S V, Kusdov D A, Rusinov I P, Shamirzaev T S, Mironov A V, Demin A Yu, Tereshchenko O E 2022 Phys. Rev. Lett. 129 166802 Google Scholar

Cited By

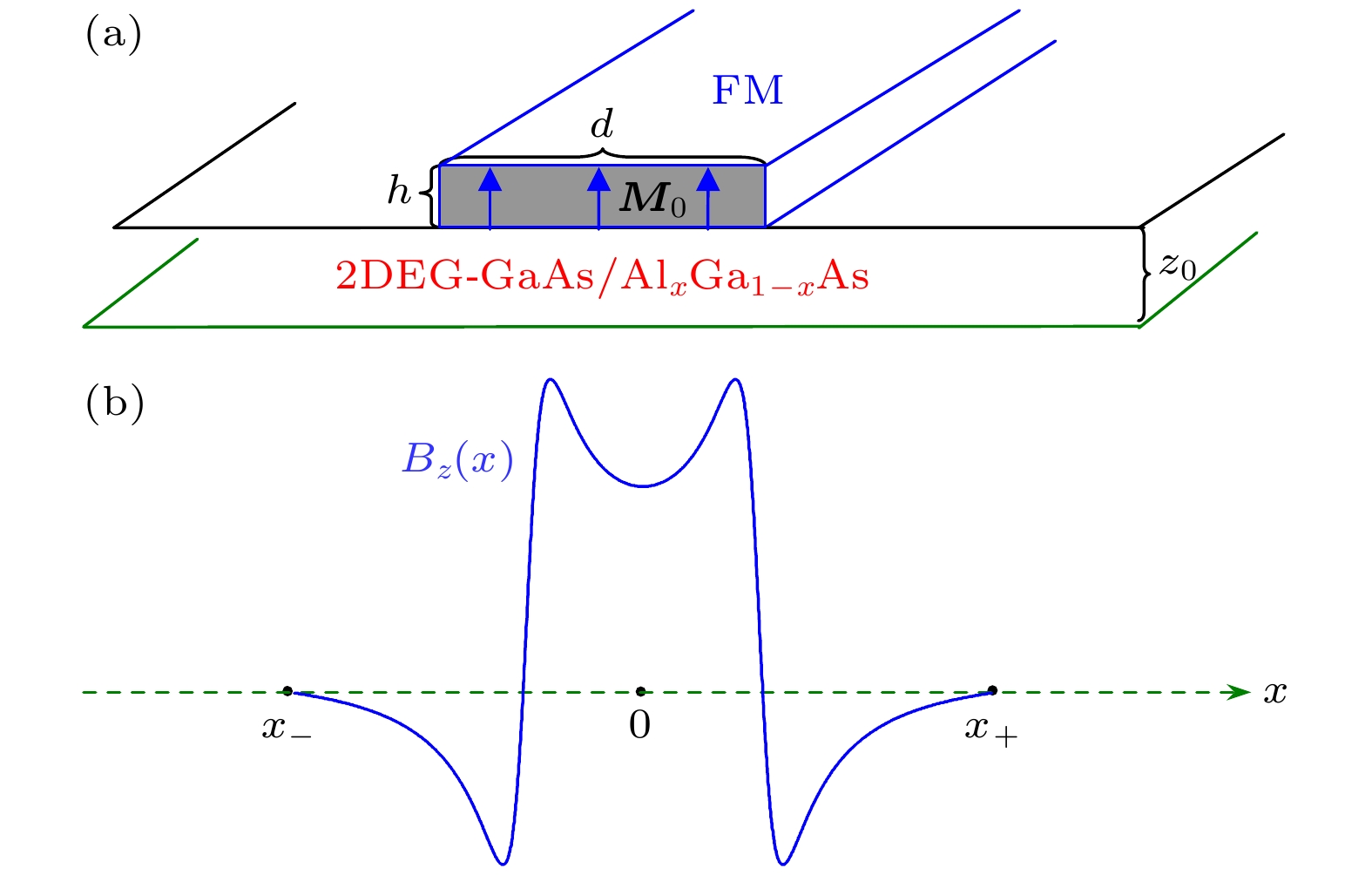
图 1 真实MCSN结构(a)及其模型(b), 图中垂直磁化的铁磁(FM)条带沉积在GaAs/Al_xGa_1–xAs异质结的表面, d、h和$ {M_0} $分别是FM条带的宽度、厚度和磁化强度, z₀表示FM条带与2DEG之间的距离, 结构的两端分别位于x_–和x₊处, B_z(x)是FM条带在2DEG平面内产生的磁场

Figure 1. The MCSN device(a)and its model(b), where a vertically magnetized ferromagnetic (FM) stripe is patterned in the surface of GaAs/Al_xGa_1–xAs heterostructure, d, h, $ {\vec M_0} $, x_- and x₊ are width, thickness, magnetization, left position and right end, respectively, z₀ represents the distance between FM stripe and 2DEG, and B_z(x) is magnetic field induced by FM stripe within 2DEG.

DownLoad: Full-Size Img PowerPoint

图 2 仅考虑塞曼效应时, 自旋向上电子和自旋向下电子在真实MCSN结构中的居留时间及其自旋极化(在插图中)随着入射能的变化, 图中电子的横向波矢取为k_y = 0(垂直入射)

Figure 2. When only Zeeman effect is involved, dwell time changes with incident energy for spin-up (up-triangle curve) and spin-down (down-triangle curve) electrons in the MCSN, respectively, where spin polarization ratio defined by dwell time is presented in the inset and transverse wave vector of electron is chosen as k_y = 0 (i.e., the normal incidence).

DownLoad: Full-Size Img PowerPoint

图 3 只考虑Rashba型SOC耦合　(a) 自旋向上和自旋向下电子在真实MCSN结构中的居留时间及其自旋极化(在插图中)随着入射能量的变化, 其中Rashba-SOC耦合的强度为$\eta_{\mathrm{R}} $ = 0.2; (b)当入射能量分别为E = 3.0, 5.0, 7.0时, 自旋极化随着Rashba-SOC耦合强度的变化; 图中电子的横向波矢取为k_y = 0

Figure 3. Only Rashba-SOC effect is considered: (a) Dwell time and spin polarization ratio (in the inset) vary with incident energy for spin-up and spin-down electrons in the MCSN, where Rashba-SOC strength is chosen as $\eta_{\mathrm{R}} $ = 0.2; (b) spin polarization ratio changes with Rashba-SOC strength for incident energy E = 3.0, 5.0, 7.0; and transverse wave vector is set to be k_y = 0.

DownLoad: Full-Size Img PowerPoint

图 4 仅计及Dresselhaus型SOC效应　(a) 自旋向上和自旋向下电子在真实MCSN结构的居留时间及其自旋极化(在插图中)随着入射能量的变化, 其中Dresselhaus-SOC耦合的强度为$\eta_{\mathrm{D}} $ = 0.2; (b)当入射能量分别为E = 3.0, 5.0, 7.0时, 自旋极化随着Dresselhaus-SOC强度的变化; 图中电子的横向波矢取为k_y = 0

Figure 4. Only Dresselhaus-SOC effect is considered: (a) Dwell time and spin polarization ratio (in the inset) vary with incident energy for spin-up and spin-down electrons in the MCSN, where Dresselhaus-SOC strength is chosen as $\eta_{\mathrm{D}} $ = 0.2; (b) spin polarization ratio changes with Dresselhaus-SOC strength for incident energy E = 3.0, 5.0, 7.0; and transverse wave vector is set to be k_y = 0.

DownLoad: Full-Size Img PowerPoint

map

[1]	Wolf S A, Awschalom D D, Buhrman R A, Daughton J M, von Molnár S, Roukes M L, Chtchelkanova A Y, Treger D M 2001 Science 294 1488 Google Scholar
[2]	Žutíc I, Fabiam J, Sarma S Das 2004 Rev. Mod. Phys. 76 323 Google Scholar
[3]	Gong C, Zhang X 2019 Science 363 706 Google Scholar
[4]	Soumyanrayanan A, Reyren N, Fert A, Panagopoulos C 2016 Nature 539 509 Google Scholar
[5]	蒋龙兴, 李庆超, 张旭, 李京峰, 张静, 陈祖信, 曾敏, 吴昊 2024 73 017505 Google Scholar Jiang L X, Li Q C, Zhang X, Li J F, Zhang J, Chen Z X, Zeng M, Wu H 2024 Acta Phys. Sin. 73 017505 Google Scholar
[6]	Gurram M, Omar S, Wees B J V 2017 Nat. Commun. 8 248 Google Scholar
[7]	Zhu W K, Lin H L, Yan F G, Hu C, Wang Z A, Zhao L X, Deng Y C, Kudrynskyi Z R, Zhou T, Kovalyuk Z D, Zheng Y H, Patanè A, Žutić I, Li S S, Zheng H Z, Wang K Y 2021 Adv. Mater. 33 2104658 Google Scholar
[8]	Zhu W K, Xie S H, Lin H L, Zhang G J, Wu H, Hu T G, Wang Z A, Zhang X M, Xu J H, Wang Y J, Zheng Y H, Yan F G, Zhang J, Zhao L X, Patanè A, Zhang J, Chang H X, Wang K Y 2022 Chin. Phys. Lett. 39 128501 Google Scholar
[9]	Zhu W K, Zhu J M, Zhou T, Zhang X P, Lin H L, Cui Q R, Yan F G, Wang Z A, Deng Y C, Yang H X, Zhao L X, Žutić I, Belashchenko K D, Wang K Y 2023 Nat. Commun. 14 5371 Google Scholar
[10]	Kitchen D, Richardella A, Tang J M, Flatté M E, Yazdani A 2006 Nature 442 436 Google Scholar
[11]	贺亚萍, 陈明霞, 潘杰锋, 李冬, 林港钧, 黄新红 2023 72 028503 Google Scholar He Y P, Chen M X, Pan J F, Li D, Lin G J, Huang X H 2023 Acta Phys. Sin. 72 028503 Google Scholar
[12]	李春雷, 郑军, 王小明, 徐燕 2023 72 227201 Google Scholar Li C L, Zheng J, Wang X M, Xu Y 2023 Acta Phys. Sin. 72 227201 Google Scholar
[13]	Liu X H, Zhang G L, Kong Y H, Li A H, Fu X 2014 Appl. Surf. Sci. 313 545 Google Scholar
[14]	Wang L, Guo Y 2006 Phys. Rev. B 73 205311 Google Scholar
[15]	Das S, Ghosh S, Kumar R, Bag A, Biswas D 2017 IEEE Trans. Electron Devices 64 4650 Google Scholar
[16]	Kong Y H, Liu X H, Li A H, Gong Y J 2019 Vacuum 159 410 Google Scholar
[17]	Nogaret A 2010 J. Phys. Condens. Matter 22 253201 Google Scholar
[18]	Hauge E H, Støvneng J A 1989 Rev. Mod. Phys. 61 917 Google Scholar
[19]	王瑞琴, 宫箭, 武建英, 陈军 2013 62 087303 Google Scholar Wang R Q, Gong J, Wu J Y, Chen J 2013 Acta Phys. Sin. 62 087303 Google Scholar
[20]	Winful H G 2003 Phys. Rev. Lett. 91 260401 Google Scholar
[21]	Zhai F, Guo Y, Gu B L 2002 Eur. Phys. J. B 29 147 Google Scholar
[22]	Xu H Z, Liu P J, Zhang Y F 2003 Phys. Status Solidi B 240 169 Google Scholar
[23]	Chen S Y, Zhang G L, Cao X L, Peng F F 2021 J. Comput. Electron. 20 785 Google Scholar
[24]	Zhang G L, Lu M W, Chen S Y, Peng F F, Meng J S 2021 IEEE Trans. Magn. 57 1400305
[25]	Guo Q M, Lu M W, Huang X H, Yang S Q, Qin Y J 2021 Vacuum 186 110059 Google Scholar
[26]	Xie S S, Lu M W, Huang X H, Wen L, Chen J L 2023 Phys. Lett. A 480 128976 Google Scholar
[27]	Guo Q M, Lu M W, Yang S Q, Qin Y J, Xie S S 2022 Braz. J. Phys. 52 74 Google Scholar
[28]	Lu M W, Chen S Y, Cao X L , Huang X H 2021 IEEE Trans. Electron Devices 68 860
[29]	Capasso F, Mohammed K, Cho A Y, Hull R, Hutchinson A L 1985 Appl. Phys. Lett. 47 420 Google Scholar
[30]	Lu M W, Chen S Y, Cao X L, Huang X H 2020 Results Phys. 19 103375 Google Scholar
[31]	Chen S Y, Cao X L, Huang X H, Lu M W 2023 Eur. Phys. J. Plus 138 111 Google Scholar
[32]	Xie S S, Lu M W, Huang X H, Wen L, Chen J L 2023 Results Phys. 51 106605 Google Scholar
[33]	Guo Q M, Chen S Y, Cao X L, Yang S Q 2021 Semicond. Sci. Technol. 36 055013 Google Scholar
[34]	Guo Q M, Lu M W, Yang S Q, Qin Y J, Xie S S 2022 J. Nanoelectron. Optoe. 16 1554
[35]	Chen S Y, Lu M W, Cao X L 2022 Chin. Phys. B 31 017201 Google Scholar
[36]	Lu M W, Chen S Y, Zhang G L, Huang X H 2018 IEEE Trans. Electron Devices 65 3045 Google Scholar
[37]	Rashba E I, Efros A L 2003 Phys. Rev. Lett. 91 126405 Google Scholar
[38]	Schliemann J, Loss D 2003 Phys. Rev. B 68 165311 Google Scholar
[39]	Bindel J R, Pezzotta M, Ulrich J, Liebmamm M, sheman E Y, Morgenstern M 2016 Nat. Phys. 12 920 Google Scholar
[40]	Intronati G A, Tamborenea P I, Weinmann D A, Jarabert R A 2012 Phys. Rev. Lett. 108 016601 Google Scholar
[41]	Xie S S, Lu M W, Chen S Y, Qin Y J, Wen L, Chen J L 2023 Commun. Theor. Phys. 75 015703 Google Scholar
[42]	von Bergmann K, Heinze S, Bode M, Vedmedenko E Y, Bihlmayer G, Blügel S, Wiesendanger R 2006 Phys. Rev. Lett. 96 167203 Google Scholar
[43]	Lu M W, Chen S Y, Zhang G L 2017 IEEE Trans. Electron Devices 64 1825 Google Scholar
[44]	You J Q, Zhang L D, Ghosh P K 1995 Phys. Rev. B 52 17243 Google Scholar
[45]	Lu M W, Chen S Y, Zhang G L, Huang X H 2018 J. Phys. Condens. Matter 30 145302 Google Scholar
[46]	Lu K Y, He Z Y, Zu M M, Guo S Y 2022 IEEE Electron Device Lett. 43 1645 Google Scholar
[47]	Lu K Y, He Z Y, Zu M M, Guo S Y, Lu M W 2023 IEEE Electron Device Lett. 44 1424 Google Scholar
[48]	Rusetsky V S, Golyashov V A, Eremeev S V, Kusdov D A, Rusinov I P, Shamirzaev T S, Mironov A V, Demin A Yu, Tereshchenko O E 2022 Phys. Rev. Lett. 129 166802 Google Scholar

[1]	Wang Huan, He Xia-Yao, Li Shuai, Liu Bo. Quench dynamics of a spin-orbital coupled Bose-Einstein condensate with nonlinear interactions. Acta Physica Sinica, 2023, 72(10): 100309. doi: 10.7498/aps.72.20222401
[2]	Li Xin-Yue, Qi Juan-Juan, Zhao Dun, Liu Wu-Ming. Soliton solutions of the spin-orbit coupled binary Bose-Einstein condensate system. Acta Physica Sinica, 2023, 72(10): 106701. doi: 10.7498/aps.72.20222319
[3]	Gao Jian-Hua, Huang Xu-Guang, Liang Zuo-Tang, Wang Qun, Wang Xin-Nian. Spin-orbital coupling in strong interaction and global spin polarization. Acta Physica Sinica, 2023, 72(7): 072501. doi: 10.7498/aps.72.20230102
[4]	Yuan Jia-Wang, Chen Li, Zhang Yun-Bo. Adiabatic elimination theory of multi-level system in spin-orbit coupled Bose-Einstein condensate. Acta Physica Sinica, 2023, 72(21): 216701. doi: 10.7498/aps.72.20231052
[5]	He Ya-Ping, Chen Ming-Xia, Pan Jie-Feng, Li Dong, Lin Gang-Jun, Huang Xin-Hong. Electron-spin polarization effect in Rashba spin-orbit coupling modulated single-layered semiconductor nanostructure. Acta Physica Sinica, 2023, 72(2): 028503. doi: 10.7498/aps.72.20221381
[6]	Ma Yun-E, Qiao Xin, Gao Rui, Liang Jun-Cheng, Zhang Ai-Xia, Xue Ju-Kui. Tunneling dynamics of tunable spin-orbit coupled Bose-Einstein condensates. Acta Physica Sinica, 2022, 71(21): 210302. doi: 10.7498/aps.71.20220697
[7]	Zhou Yong-Xiang, Xue Xun. Electron vortices in spin-orbit coupling system. Acta Physica Sinica, 2022, 71(21): 210301. doi: 10.7498/aps.71.20220751
[8]	Gao Feng, Zhang Hong, Zhang Chang-Zhe, Zhao Wen-Li, Meng Qing-Tian. Accurate theoretical study of potential energy curves, spectroscopic parameters, vibrational energy levels and spin-orbit coupling interaction on SiH⁺(X¹Σ⁺) ion. Acta Physica Sinica, 2021, 70(15): 153301. doi: 10.7498/aps.70.20210450
[9]	Li Ji, Liu Bin, Bai Jing, Wang Huan-Yu, He Tian-Chen. Ground state of spin-orbit coupled rotating ferromagnetic Bose-Einstein condensate in toroidal trap. Acta Physica Sinica, 2020, 69(14): 140301. doi: 10.7498/aps.69.20200372
[10]	Liang Shi-Heng, Lu Yuan, Han Xiu-Feng. Research progress of spin light emitting diode. Acta Physica Sinica, 2020, 69(20): 208501. doi: 10.7498/aps.69.20200866
[11]	Wen Lin, Liang Yi, Zhou Jing, Yu Peng, Xia Lei, Niu Lian-Bin, Zhang Xiao-Fei. Effects of linear Zeeman splitting on the dynamics of bright solitons in spin-orbit coupled Bose-Einstein condensates. Acta Physica Sinica, 2019, 68(8): 080301. doi: 10.7498/aps.68.20182013
[12]	Li Ji, Liu Wu-Ming. Ground state of spin-orbit coupled rotating two-component Bose-Einstein condensate in gradient magnetic field. Acta Physica Sinica, 2018, 67(11): 110302. doi: 10.7498/aps.67.20180539
[13]	He Li, Yu Zeng-Qiang. Landau critical velocity of spin-orbit-coupled Bose-Einstein condensate across quantum phase transition. Acta Physica Sinica, 2017, 66(22): 220301. doi: 10.7498/aps.66.220301
[14]	Huang Zhen, Zeng Wen, Gu Yi, Liu Li, Zhou Lu, Zhang Wei-Ping. Double reflection of spin-orbit-coupled cold atoms. Acta Physica Sinica, 2016, 65(16): 164201. doi: 10.7498/aps.65.164201
[15]	He Li, Yu Zeng-Qiang. Dynamic structure factors and sum rules in two-component quantum gases with spin-orbit coupling. Acta Physica Sinica, 2016, 65(13): 131101. doi: 10.7498/aps.65.131101
[16]	Zeng Shao-Long, Li Ling, Xie Zheng-Wei. Tunneling times in double spin-filter junctions. Acta Physica Sinica, 2016, 65(22): 227302. doi: 10.7498/aps.65.227302
[17]	Li Zhi, Cao Hui. Klein tunneling in spin-orbit coupled Bose-Einstein condensate scattered by cusp barrier. Acta Physica Sinica, 2014, 63(11): 110306. doi: 10.7498/aps.63.110306
[18]	Li Zhi, Wang Jian-Zhong. Barrier scattering properties in spin-orbit coupled Bose-Einstein condensate. Acta Physica Sinica, 2013, 62(10): 100306. doi: 10.7498/aps.62.100306
[19]	Li Rui, Yan Bing, Zhao Shu-Tao, Guo Qing-Qun, Lian Ke-Yan, Tian Chuan-Jin, Pan Shou-Fu. Spin-orbit ab initio calculation of photodissociation of methyl iodide. Acta Physica Sinica, 2008, 57(7): 4130-4133. doi: 10.7498/aps.57.4130
[20]	Fu Mei-Huan, Ren Zhong-Zhou. Four kinds of raising and lowering operators of three-dimensional isotropic harmonic oscillators with spin-orbit coupling. Acta Physica Sinica, 2004, 53(5): 1280-1283. doi: 10.7498/aps.53.1280

Catalog

Vol. 73, Issue 11 - 2024-06-05

Metrics

Abstract views: 1474
PDF Downloads: 84
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板