Soliton solutions of the spin-orbit coupled binary Bose-Einstein condensate system

Li Xin-Yue; Qi Juan-Juan; Zhao Dun; Liu Wu-Ming

doi:10.7498/aps.72.20222319

Abstract

In a quantum system with spin, spin-orbit coupling is manifested by linking the spin angular momentum of a particle with its orbital angular momentum, which leads to many exotic phenomena. The experimental realization of synthetic spin-orbit coupling effects in ultra-cold atomic systems provides an entirely new platform for exploring quantum simulations. In a spinor Bose-Einstein condensate, the spin-orbit coupling can change the properties of the system significantly, which offers an excellent opportunity to investigate the influence of spin-orbit coupling on the quantum state at the macroscopic level. As typical states of macroscopic quantum effects, solitons in spin-orbit coupled Bose-Einstein condensates can be manipulated by spin-orbit coupling directly, which makes the study on spin-orbit coupled Bose-Einstein condensates become one of the hottest topics in the research of ultracold atomic physics in recent years. This paper investigates exact vector soliton solutions of the Gross-Pitaevskii equation for the one-dimensional spin-orbit coupled binary Bose-Einstein condensates, which has four parameters $\mu$, $\delta$, $\alpha$ and $\beta$, where $\mu$ denotes the strength of the spin-orbit coupling, $\delta$ is the detuning parameter, $\alpha$ and $\beta$ are the parameters of the self- and cross-interaction, respectively. For the case $\beta=\alpha$, by a direct ansatz, two kinds of stripe solitons, namely, the oscillating dark-dark solitons are obtained; meanwhile, a transformation is presented such that from the solutions of the integrable Manakov system, one can get soliton solutions for the spin-orbit coupled Gross-Pitaevskii equation. For the case $\beta=3\alpha$, a bright-W type soliton for $\alpha>0$ and a kink-antikink type soliton for $\alpha<0$ are presented. It is found that the relation between $\mu$ and $\delta$ can affect the states of the solitons. Based on these solutions, the corresponding dynamics and the impact of the spin-orbit coupling effects on the quantum magnetization and spin-polarized domains are discussed. Our results show that spin-orbit coupling can result in rich kinds of soliton states in the two-component Bose gases, including the stripe solitons as well as the classical non-stripe solitons, and various kinds of multi-solitons. Furthermore, spin-orbit coupling has a remarkable influence on the behaviors of quantum magnetization. In the experiments of Bose-Einstein condensates, there have been many different methods to observe the soliton states of the population distribution, the magnetic solitons, and the spin domains, so our results provide some possible options for the related experiments.

Keywords:

Authors and contacts

1.
School of Mathematics and Statistics, Lanzhou University, Lanzhou 730000, China
2.
Institute of Physics, Chinese Academy of Sciences, Beijing 100190, China

Corresponding author: Zhao Dun, zhaod@lzu.edu.cn ; Liu Wu-Ming, wmliu@iphy.ac.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12075102, 61835013, 12234012), the National Key R&D Program of China (Grant Nos. 2021YFA1400900, 2021YFA0718300, 2021YFA1402100), and the Space Application System of China Manned Space Program

FullText HTML : translate this paragraph

References

[1]	Pitaevskii L, Stringari S 2016 Bose-Einstein Condensation and Superfluidity (Oxford: Oxford University Press)
[2]	Proukakis N P, Snoke D W, Littlewood P B 2017 Universal Themes of Bose-Einstein Condensation (Cambridge: Cambridge University Press)
[3]	Zhai H 2020 Ultracold Atomic Physics (Cambridge: Cambridge University Press)
[4]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[5]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191 Google Scholar
[6]	Liu W M, Kengne E 2019 Schrödinger Equations in Nonlinear Systems (Singapore: Springer Nature Singapore Pte Ltd)
[7]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83 Google Scholar
[8]	Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301 Google Scholar
[9]	Wang P, Yu Z Q, Fu Z, Miao J, Huang L, Chai S, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[10]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[11]	Zhai H 2015 Rep. Prog. Phys. 78 026001 Google Scholar
[12]	Zhang Y P, Mossman M E, Busch T, Engels P, Zhang C W 2016 Front. Phys. 11 118103 Google Scholar
[13]	李吉, 刘伍明 2018 67 110302 Google Scholar Li J, Liu W M 2018 Acta Phys. Sin. 67 110302 Google Scholar
[14]	Zhang S C, Jo G B 2019 J. Phys. Chem. Solids 128 75 Google Scholar
[15]	王力, 刘静思, 李吉, 周晓林, 陈向荣, 刘超飞, 刘伍明 2020 69 010303 Google Scholar Wang L, Liu J S, Li J, Zhou X L, Chen X R, Liu C F, Liu W M 2020 Acta Phys. Sin. 69 010303 Google Scholar
[16]	施婷婷, 汪六九, 王璟琨, 张威 2020 69 016701 Google Scholar Shi T T, Wang L J, Wang J K, Zhang W 2020 Acta Phys. Sin. 69 016701 Google Scholar
[17]	Achilleos V, Frantzeskakis D J, Kevrekidis P G, Pelinovsky D E 2013 Phys. Rev. Lett. 110 264101 Google Scholar
[18]	Achilleos V, Stockhofe J, Frantzeskakis D J, Kevrekidis P G, Schmelcher P 2013 EPL 103 20002 Google Scholar
[19]	Lobanov V E, Kartashov Y V, Konotop V V 2014 Phys. Rev. Lett. 112 180403 Google Scholar
[20]	Li Y Y, Luo Z H, Liu Y, Chen Z P, Huang C Q, Fu S H, Tan H S, Malomed B A 2017 New. J. Phys. 19 113043 Google Scholar
[21]	Kartashov Y V, Konotop V V 2017 Phys. Rev. Lett. 118 190401 Google Scholar
[22]	Sakaguchi H, Malomed B A 2018 Phys. Rev. A 97 013607 Google Scholar
[23]	Wang C, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[24]	Bhuvaneswari S, Nithyanandan K, Muruganandam P 2018 J. Phys. Commun. 2 025008 Google Scholar
[25]	Xu X Q, Han J H 2011 Phys. Rev. Lett. 107 200401 Google Scholar
[26]	Radić J, Sedrakyan T A, Spielman I B, Galitski V 2011 Phys. Rev. A 84 063604 Google Scholar
[27]	Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F Ç, Jamison A O, Ketterle W 2017 Nature 543 91 Google Scholar
[28]	Léonard J, Morales A, Zupancic P, Esslinger T, Donner T 2017 Nature 543 87 Google Scholar
[29]	Khaykovich L, Schreck F, Ferrari G, Bourdel T, Cubizolles J, Carr L D, Castin Y, Salomo C 2002 Science 296 1290 Google Scholar
[30]	Nguyen J H V, Luo D, Hulet R G 2017 Science 356 422 Google Scholar
[31]	Kengne E, Liu W M, Malomed B A 2021 Phys. Rep. 899 1 Google Scholar
[32]	Xu Y, Zhang Y P, Wu B 2013 Phys. Rev. A 87 013614 Google Scholar
[33]	Kartashov Y V, Konotop V V, Abdullaev F Kh 2013 Phys. Rev. Lett. 111 060402 Google Scholar
[34]	Sakaguchi H, Li B, Malomed B A 2014 Phys. Rev. E 89 032920 Google Scholar
[35]	Cao S, Shan C J, Zhang D W, Qin X Z, Xu J 2015 J. Opt. Soc. Am. B 32 201 Google Scholar
[36]	Qu C L, Pitaevskii L P, Stringari S 2016 Phys. Rev. Lett. 116 160402 Google Scholar
[37]	Chiquillo E 2018 Phys. Rev. A 97 013614 Google Scholar
[38]	Farolfi A, Trypogeorgos D, Mordini C, Lamporesi G, Ferrari G 2020 Phys. Rev. Lett. 125 030401 Google Scholar
[39]	Chai X, Lao D, Fujimoto K, Raman C 2021 Phys. Rev. Res. 3 L012003 Google Scholar
[40]	Liu Y K, Yang S J 2014 EPL 108 30004 Google Scholar
[41]	Gautam S, Adhikari S K 2021 Braz. J. Phys. 51 298 Google Scholar
[42]	He J T, Fang P P, Lin J 2022 Chin. Phys. Lett. 39 020301 Google Scholar
[43]	Yang Y, Gao P, Wu Z, Zhao L C, Yang Z Y 2021 Annals of Physics 431 168562 Google Scholar
[44]	Yang Y, Gao P, Zhao L C, Yang Z Y 2022 Front. Phys. 17 32503 Google Scholar
[45]	De S, Campbell D L, Price R M, Putra A, Anderson B M, Spielman I B 2014 Phys. Rev. A 89 033631 Google Scholar
[46]	Sakaguchi H, Malomed B A 2019 Symmetry 11 388 Google Scholar
[47]	Zakharov V E, Schulman E I 1982 Phys. D 4 270 Google Scholar
[48]	Manakov S V 1973 Zh. Eksp. Teor. Fiz. 65 1392
[49]	Yang J K 2010 Nonlinear Waves in Integrable and Nonintegrable Systems (Philadelphia: SIAM) pp79–118
[50]	Guo B L, Ling L M 2011 Chin. Phys. Lett. 28 110202 Google Scholar
[51]	Qin Y H, Zhao L C, Ling L 2019 Phys. Rev. E 100 022212 Google Scholar
[52]	Qin Y H, Wu Y, Zhao L C, Yang Z Y 2020 Chin. Phys. B 29 020303 Google Scholar
[53]	Tian H Y, Tian B, Yuan Y Q, Zhang C R 2021 Phys. Scr. 96 045213 Google Scholar
[54]	Radhakrishnan R, Lakshmanan M 1995 J. Phys. A: Math. Gen. 28 2683 Google Scholar
[55]	Lan Y, Zhao L C, Luo X W 2019 Commun. Nonlinear Sci. Numer. Simul. 70 334 Google Scholar
[56]	Ohta Y, Wang D S, Yang J K 2011 Stud. Appl. Math. 127 345 Google Scholar
[57]	Yan C T 1996 Phys. Lett. A 224 77 Google Scholar
[58]	Punya A, Lambrecht W R L 2012 Phys. Rev. B 85 195147 Google Scholar
[59]	Sheng X L, Wang Z J, Yu R, Weng H M, Fang Z, Dai X 2014 Phys. Rev. B 90 245308 Google Scholar
[60]	Łepkowski S P, Bardyszewski W 2018 Sci. Rep. 8 15403 Google Scholar

Cited By

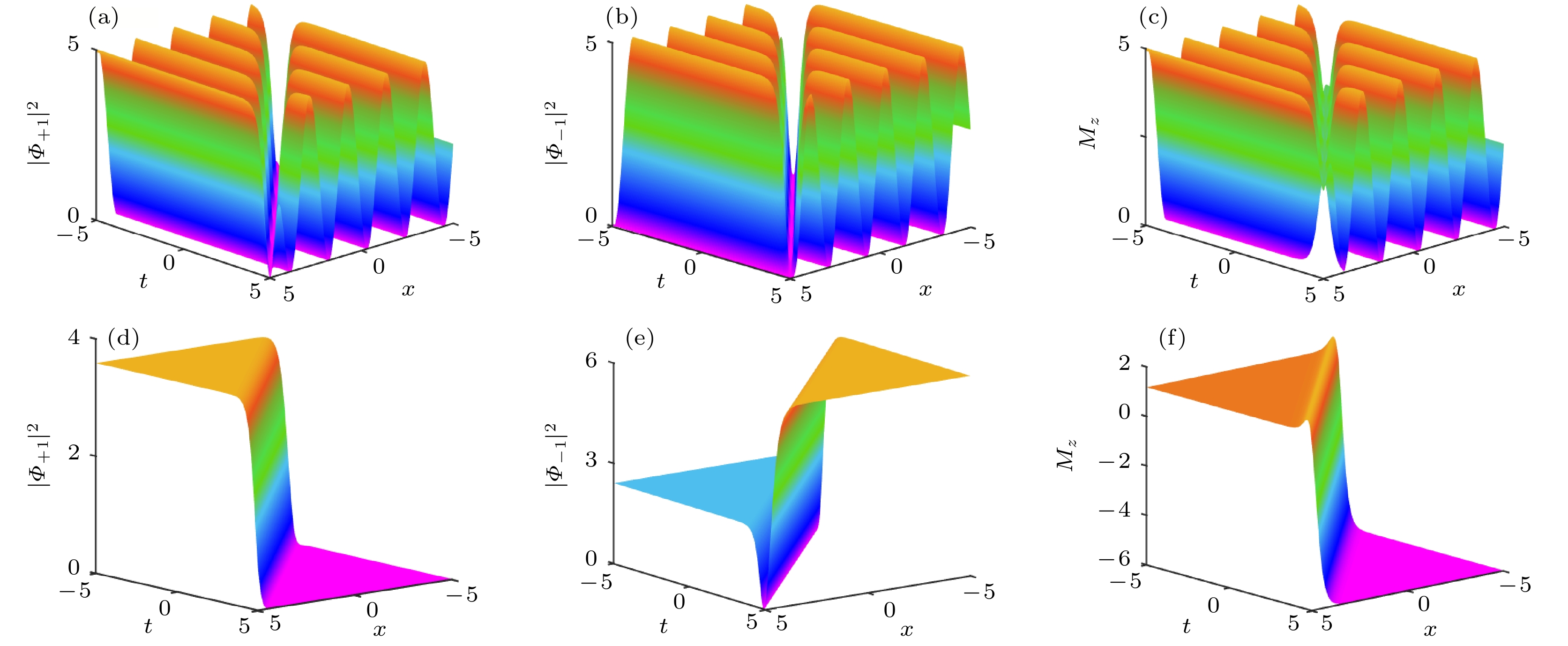
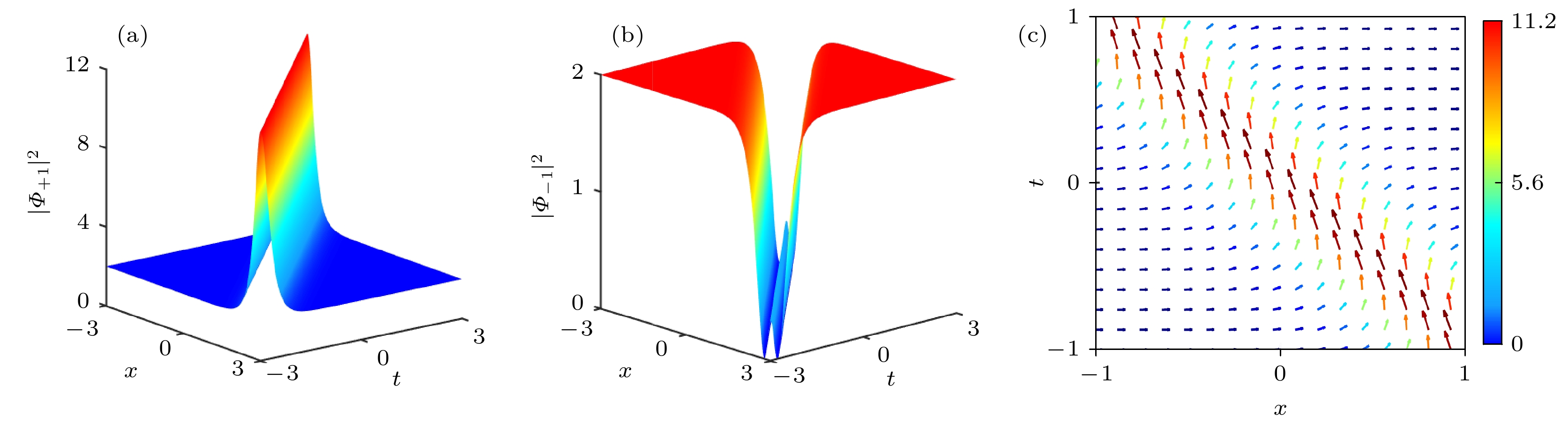
图 1 (5)式对应的SOC调制的条纹暗孤子. 暗孤子的均匀背景被SOC调制为周期背景, 暗孤子中心与周期背景产生能量交换, 形成了周期背景中的呼吸子传播. 参数取值为$k= 1$, $\delta = 0$, $\mu = 3$, $\omega = 2$, $\alpha = -1$

Figure 1. Stripe dark soliton given by Eq. (5). The uniform background of the dark soliton is modulated by the SOC into the periodic background, and the energy exchange between the center of the dark soliton and the periodic background results in a breather like soliton propagating in the periodic background. The parameters are chosen as $k = 1$, $\delta = 0$, $\mu = 3$, $\omega = 2$, $\alpha = -1$

DownLoad: Full-Size Img PowerPoint

图 2 (5)式对应的不同参数下的赝自旋演化　(a) $\mu=3/2$, $\delta=0$, $\omega=-4$; (b) $\mu=1/2$, $\delta=-1$, $\omega=-4$; (c) $\delta=1$, $\mu=1$, $\omega=-4$. 其他参数为$k=1$, $\alpha=-1$

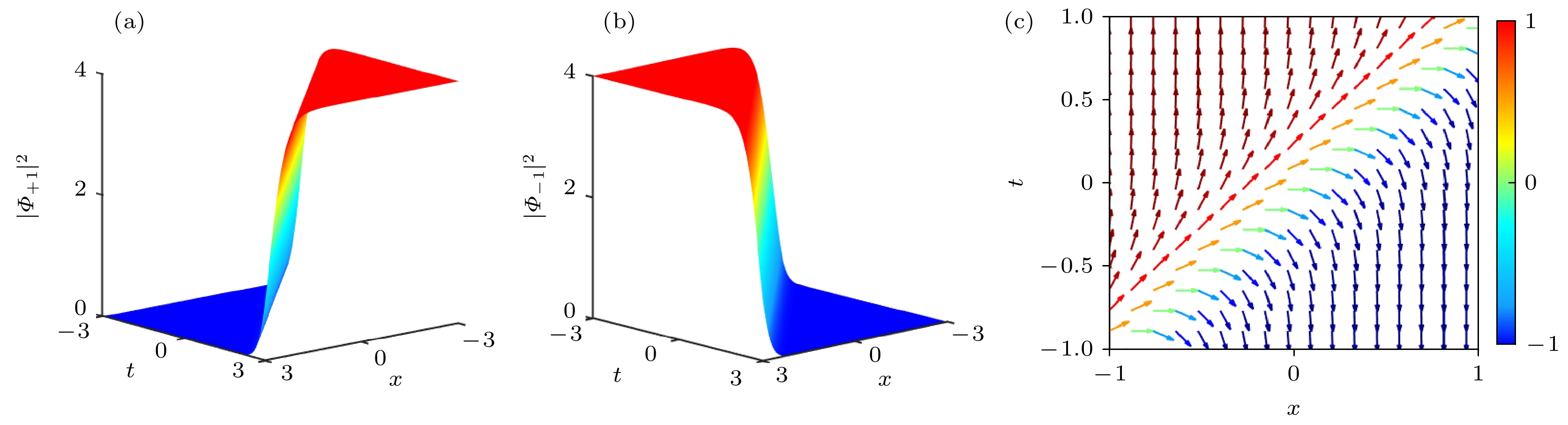
Figure 2. Evolution of pseudo-spin corresponding to Eq. (5) with different parameters: (a) $\mu=3/2$, $\delta=0$, $\omega=-4$; (b) $\mu=1/2$, $\delta=-1$, $\omega=-4$; (c) $\delta=1$, $\mu=1$, $\omega=-4$. Other parameters are taken as $k=1$, $\alpha=-1$

DownLoad: Full-Size Img PowerPoint

图 3 (7)式对应的不同参数下的密度分布和磁化分布　(a)—(c) $\mu = 3/2$, $\delta = 0$; (d)—(f) $\mu=1$, $\delta=-2$. 其他参数为$G_1 = 2$, $G_2 = 1$, $k = 1$, $\alpha = -1$

Figure 3. Distributions of the density and magnetization corresponding to Eq. (7) with different parameters: (a)–(c)$\mu = 3/2$, $\delta = 0$; (d)–(f)$\mu=1$, $\delta=-2$. Other parameters are taken as $G_1 = 2$, $G_2 = 1$, $k = 1$, $\alpha = -1$

DownLoad: Full-Size Img PowerPoint

图 4 (7)式对应的不同参数下的赝自旋演化　(a) $\mu=3/2$, $\delta=0$; (b) $\mu=1$, $\delta=-2$; (c) $\delta=1$, $\mu=1$. 其他参数为$G_1=2$, $G_2=1$, $k=1$, $\alpha=-1$

Figure 4. Evolution of pseudo-spin corresponding to Eq. (7) with the different parameters: (a) $\mu=3/2$, $\delta=0$; (b) $\mu=1$, $\delta=-2$; (c) $\delta=1$, $\mu=1$. Other parameters are taken as $G_1=2$, $G_2=1$, $k=1$, $\alpha=-1$

DownLoad: Full-Size Img PowerPoint

图 5 (13)式对应的密度分布和赝自旋演化. 参数取值为$\alpha = 1$, $\mu = 1$, $\delta = 0$, $G = 2$

Figure 5. Density distribution and the evolution of pseudo-spin corresponding to (13). The parameters are taken as $\alpha = 1$, $\mu = 1$, $\delta = 0$, $G = 2$

DownLoad: Full-Size Img PowerPoint

图 6 (14)式对应的密度分布和赝自旋演化. 参数取值为$\alpha =-1$, $\mu =-1$, $\delta = 0$, $G = 1$

Figure 6. Density distribution and the evolution of pseudo-spin corresponding to (14). The parameters are taken as $\alpha =-1$, $\mu =-1$, $\delta = 0$, $G = 1$

DownLoad: Full-Size Img PowerPoint

map

[1]	Pitaevskii L, Stringari S 2016 Bose-Einstein Condensation and Superfluidity (Oxford: Oxford University Press)
[2]	Proukakis N P, Snoke D W, Littlewood P B 2017 Universal Themes of Bose-Einstein Condensation (Cambridge: Cambridge University Press)
[3]	Zhai H 2020 Ultracold Atomic Physics (Cambridge: Cambridge University Press)
[4]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 Google Scholar
[5]	Stamper-Kurn D M, Ueda M 2013 Rev. Mod. Phys. 85 1191 Google Scholar
[6]	Liu W M, Kengne E 2019 Schrödinger Equations in Nonlinear Systems (Singapore: Springer Nature Singapore Pte Ltd)
[7]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83 Google Scholar
[8]	Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301 Google Scholar
[9]	Wang P, Yu Z Q, Fu Z, Miao J, Huang L, Chai S, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301 Google Scholar
[10]	Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302 Google Scholar
[11]	Zhai H 2015 Rep. Prog. Phys. 78 026001 Google Scholar
[12]	Zhang Y P, Mossman M E, Busch T, Engels P, Zhang C W 2016 Front. Phys. 11 118103 Google Scholar
[13]	李吉, 刘伍明 2018 67 110302 Google Scholar Li J, Liu W M 2018 Acta Phys. Sin. 67 110302 Google Scholar
[14]	Zhang S C, Jo G B 2019 J. Phys. Chem. Solids 128 75 Google Scholar
[15]	王力, 刘静思, 李吉, 周晓林, 陈向荣, 刘超飞, 刘伍明 2020 69 010303 Google Scholar Wang L, Liu J S, Li J, Zhou X L, Chen X R, Liu C F, Liu W M 2020 Acta Phys. Sin. 69 010303 Google Scholar
[16]	施婷婷, 汪六九, 王璟琨, 张威 2020 69 016701 Google Scholar Shi T T, Wang L J, Wang J K, Zhang W 2020 Acta Phys. Sin. 69 016701 Google Scholar
[17]	Achilleos V, Frantzeskakis D J, Kevrekidis P G, Pelinovsky D E 2013 Phys. Rev. Lett. 110 264101 Google Scholar
[18]	Achilleos V, Stockhofe J, Frantzeskakis D J, Kevrekidis P G, Schmelcher P 2013 EPL 103 20002 Google Scholar
[19]	Lobanov V E, Kartashov Y V, Konotop V V 2014 Phys. Rev. Lett. 112 180403 Google Scholar
[20]	Li Y Y, Luo Z H, Liu Y, Chen Z P, Huang C Q, Fu S H, Tan H S, Malomed B A 2017 New. J. Phys. 19 113043 Google Scholar
[21]	Kartashov Y V, Konotop V V 2017 Phys. Rev. Lett. 118 190401 Google Scholar
[22]	Sakaguchi H, Malomed B A 2018 Phys. Rev. A 97 013607 Google Scholar
[23]	Wang C, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 Google Scholar
[24]	Bhuvaneswari S, Nithyanandan K, Muruganandam P 2018 J. Phys. Commun. 2 025008 Google Scholar
[25]	Xu X Q, Han J H 2011 Phys. Rev. Lett. 107 200401 Google Scholar
[26]	Radić J, Sedrakyan T A, Spielman I B, Galitski V 2011 Phys. Rev. A 84 063604 Google Scholar
[27]	Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F Ç, Jamison A O, Ketterle W 2017 Nature 543 91 Google Scholar
[28]	Léonard J, Morales A, Zupancic P, Esslinger T, Donner T 2017 Nature 543 87 Google Scholar
[29]	Khaykovich L, Schreck F, Ferrari G, Bourdel T, Cubizolles J, Carr L D, Castin Y, Salomo C 2002 Science 296 1290 Google Scholar
[30]	Nguyen J H V, Luo D, Hulet R G 2017 Science 356 422 Google Scholar
[31]	Kengne E, Liu W M, Malomed B A 2021 Phys. Rep. 899 1 Google Scholar
[32]	Xu Y, Zhang Y P, Wu B 2013 Phys. Rev. A 87 013614 Google Scholar
[33]	Kartashov Y V, Konotop V V, Abdullaev F Kh 2013 Phys. Rev. Lett. 111 060402 Google Scholar
[34]	Sakaguchi H, Li B, Malomed B A 2014 Phys. Rev. E 89 032920 Google Scholar
[35]	Cao S, Shan C J, Zhang D W, Qin X Z, Xu J 2015 J. Opt. Soc. Am. B 32 201 Google Scholar
[36]	Qu C L, Pitaevskii L P, Stringari S 2016 Phys. Rev. Lett. 116 160402 Google Scholar
[37]	Chiquillo E 2018 Phys. Rev. A 97 013614 Google Scholar
[38]	Farolfi A, Trypogeorgos D, Mordini C, Lamporesi G, Ferrari G 2020 Phys. Rev. Lett. 125 030401 Google Scholar
[39]	Chai X, Lao D, Fujimoto K, Raman C 2021 Phys. Rev. Res. 3 L012003 Google Scholar
[40]	Liu Y K, Yang S J 2014 EPL 108 30004 Google Scholar
[41]	Gautam S, Adhikari S K 2021 Braz. J. Phys. 51 298 Google Scholar
[42]	He J T, Fang P P, Lin J 2022 Chin. Phys. Lett. 39 020301 Google Scholar
[43]	Yang Y, Gao P, Wu Z, Zhao L C, Yang Z Y 2021 Annals of Physics 431 168562 Google Scholar
[44]	Yang Y, Gao P, Zhao L C, Yang Z Y 2022 Front. Phys. 17 32503 Google Scholar
[45]	De S, Campbell D L, Price R M, Putra A, Anderson B M, Spielman I B 2014 Phys. Rev. A 89 033631 Google Scholar
[46]	Sakaguchi H, Malomed B A 2019 Symmetry 11 388 Google Scholar
[47]	Zakharov V E, Schulman E I 1982 Phys. D 4 270 Google Scholar
[48]	Manakov S V 1973 Zh. Eksp. Teor. Fiz. 65 1392
[49]	Yang J K 2010 Nonlinear Waves in Integrable and Nonintegrable Systems (Philadelphia: SIAM) pp79–118
[50]	Guo B L, Ling L M 2011 Chin. Phys. Lett. 28 110202 Google Scholar
[51]	Qin Y H, Zhao L C, Ling L 2019 Phys. Rev. E 100 022212 Google Scholar
[52]	Qin Y H, Wu Y, Zhao L C, Yang Z Y 2020 Chin. Phys. B 29 020303 Google Scholar
[53]	Tian H Y, Tian B, Yuan Y Q, Zhang C R 2021 Phys. Scr. 96 045213 Google Scholar
[54]	Radhakrishnan R, Lakshmanan M 1995 J. Phys. A: Math. Gen. 28 2683 Google Scholar
[55]	Lan Y, Zhao L C, Luo X W 2019 Commun. Nonlinear Sci. Numer. Simul. 70 334 Google Scholar
[56]	Ohta Y, Wang D S, Yang J K 2011 Stud. Appl. Math. 127 345 Google Scholar
[57]	Yan C T 1996 Phys. Lett. A 224 77 Google Scholar
[58]	Punya A, Lambrecht W R L 2012 Phys. Rev. B 85 195147 Google Scholar
[59]	Sheng X L, Wang Z J, Yu R, Weng H M, Fang Z, Dai X 2014 Phys. Rev. B 90 245308 Google Scholar
[60]	Łepkowski S P, Bardyszewski W 2018 Sci. Rep. 8 15403 Google Scholar

[1]	Gao Jian-Hua, Huang Xu-Guang, Liang Zuo-Tang, Wang Qun, Wang Xin-Nian. Spin-orbital coupling in strong interaction and global spin polarization. Acta Physica Sinica, 2023, 72(7): 072501. doi: 10.7498/aps.72.20230102
[2]	Qiu Xu, Wang Lin-Xue, Chen Guang-Ping, Hu Ai-Yuan, Wen Lin. Dynamics of spin-tensor-momentum coupled Bose-Einstein condensates. Acta Physica Sinica, 2023, 72(18): 180304. doi: 10.7498/aps.72.20231076
[3]	Wang Huan, He Xia-Yao, Li Shuai, Liu Bo. Quench dynamics of a spin-orbital coupled Bose-Einstein condensate with nonlinear interactions. Acta Physica Sinica, 2023, 72(10): 100309. doi: 10.7498/aps.72.20222401
[4]	Yuan Jia-Wang, Chen Li, Zhang Yun-Bo. Adiabatic elimination theory of multi-level system in spin-orbit coupled Bose-Einstein condensate. Acta Physica Sinica, 2023, 72(21): 216701. doi: 10.7498/aps.72.20231052
[5]	Wang Qing-Qing, Zhou Yu-Shan, Wang Jing, Fan Xiao-Bei, Shao Kai-Hua, Zhao Yue-Xing, Song Yan, Shi Yu-Ren. Surface gap solitons and their stabilities in quasi-1D Bose-Einstein condensate with three-body interactions. Acta Physica Sinica, 2023, 72(10): 100308. doi: 10.7498/aps.72.20222195
[6]	Jiao Chen, Jian Yue, Zhang Ai-Xia, Xue Ju-Kui. Excitation spectrum of tunable spin-orbit coupled Bose-Einstein condensates and its effective regulation. Acta Physica Sinica, 2023, 72(6): 060302. doi: 10.7498/aps.72.20222306
[7]	Ma Yun-E, Qiao Xin, Gao Rui, Liang Jun-Cheng, Zhang Ai-Xia, Xue Ju-Kui. Tunneling dynamics of tunable spin-orbit coupled Bose-Einstein condensates. Acta Physica Sinica, 2022, 71(21): 210302. doi: 10.7498/aps.71.20220697
[8]	Zhang Ai-Xia, Jiang Yan-Fang, Xue Ju-Kui. Nonlinear energy band structure of spin-orbit coupled Bose-Einstein condensates in optical lattice. Acta Physica Sinica, 2021, 70(20): 200302. doi: 10.7498/aps.70.20210705
[9]	Li Ji, Liu Bin, Bai Jing, Wang Huan-Yu, He Tian-Chen. Ground state of spin-orbit coupled rotating ferromagnetic Bose-Einstein condensate in toroidal trap. Acta Physica Sinica, 2020, 69(14): 140301. doi: 10.7498/aps.69.20200372
[10]	Wen Lin, Liang Yi, Zhou Jing, Yu Peng, Xia Lei, Niu Lian-Bin, Zhang Xiao-Fei. Effects of linear Zeeman splitting on the dynamics of bright solitons in spin-orbit coupled Bose-Einstein condensates. Acta Physica Sinica, 2019, 68(8): 080301. doi: 10.7498/aps.68.20182013
[11]	Li Ji, Liu Wu-Ming. Ground state of spin-orbit coupled rotating two-component Bose-Einstein condensate in gradient magnetic field. Acta Physica Sinica, 2018, 67(11): 110302. doi: 10.7498/aps.67.20180539
[12]	He Li, Yu Zeng-Qiang. Landau critical velocity of spin-orbit-coupled Bose-Einstein condensate across quantum phase transition. Acta Physica Sinica, 2017, 66(22): 220301. doi: 10.7498/aps.66.220301
[13]	Chen Guang-Ping. Ground state of a rotating spin-orbit-coupled Bose-Einstein condensate in a harmonic plus quartic potential. Acta Physica Sinica, 2015, 64(3): 030302. doi: 10.7498/aps.64.030302
[14]	Li Zhi, Cao Hui. Klein tunneling in spin-orbit coupled Bose-Einstein condensate scattered by cusp barrier. Acta Physica Sinica, 2014, 63(11): 110306. doi: 10.7498/aps.63.110306
[15]	Li Zhi, Wang Jian-Zhong. Barrier scattering properties in spin-orbit coupled Bose-Einstein condensate. Acta Physica Sinica, 2013, 62(10): 100306. doi: 10.7498/aps.62.100306
[16]	Liu Chao-Fei, Wan Wen-Juan, Zhang Gan-Yuan. Vortex pattern in spin-orbit coupled spin-1 Bose-Einstein condensate of 23Na. Acta Physica Sinica, 2013, 62(20): 200306. doi: 10.7498/aps.62.200306
[17]	Zhang Bo, Wang Deng-Long, She Yan-Chao, Zhang Wei-Xi. Soliton dynamical behavior of the condensates trapped in a square-well potential. Acta Physica Sinica, 2013, 62(11): 110501. doi: 10.7498/aps.62.110501
[18]	Zong Feng-De, Yang Yang, Zhang Jie-Fang. Evolution and controlled manipulation of a Bose-Einstein condensate chirped soliton in external potentials. Acta Physica Sinica, 2009, 58(6): 3670-3678. doi: 10.7498/aps.58.3670
[19]	Zong Feng-De, Zhang Jie-Fang. N-soliton interactions of Bose-Einstein condensates in external potentials. Acta Physica Sinica, 2008, 57(5): 2658-2668. doi: 10.7498/aps.57.2658
[20]	Zhao Xing-Dong, Xie Zheng-Wei, Zhang Wei-Ping. Nonlinear spin waves in a Bose condensed atomic chain. Acta Physica Sinica, 2007, 56(11): 6358-6366. doi: 10.7498/aps.56.6358

Catalog

Vol. 72, Issue 10 - 2023-05-20

Metrics

Abstract views: 4196
PDF Downloads: 165
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板