-
Two-dimensional (2D) borophene has attracted widespread research interest in condensed matter physics and materials science because of its rich physical and chemical properties. However, the mechanical properties and deformation mechanisms of borophene under different loadings are still unclear and not thoroughly investigated. In this work, the tensile, shear, and nanoindentation failure processes of borophene are simulated via molecular dynamics method to obtain the key mechanical parameters of borophene. The mechanical response and deformation mechanism of borophene under different loads are analyzed from the change of B—B bond length with the strain/indentation depth. The results show that the tensile mechanical properties of borophene exhibit significant anisotropic characteristics, with the Young’s modulus and strength along the armchair direction being much higher than those along the zigzag direction. However, the anisotropy of the shear mechanical properties of borophene is not significant. This phenomenon can be attributed to the different contributions of the strong B—B σ bonds and weak multi-center bonds in borophene when they are stretched in different directions. It is also found that borophene exhibits different mechanical responses under spherical indentation and cylindrical indentation. The force at failure of the borophene under spherical indentation is much lower than the value under cylindrical one, and the intrinsic mechanical parameters of borophene under spherical indentation cannot be estimated accurately because of the anisotropic characteristics of borophene. However, under cylindrical indentation, borophene exhibits similar anisotropic characteristics to those under tension, and the mechanical parameters such as Young’s modulus can be measured accurately, which are consistent with those obtained under tension. In addition, the effects of the borophene indentation model and spherical/cylindrical indenter size, the loading rate and temperature on the mechanical parameters of borophene are also studied systematically. The results indicate that the Young’s modulus of borophene from spherical indentation is highly estimated when a/R < 15 but not sensitive when a/R > 15, while the results from cylindrical indentation are hardly affected by the values of L/R and W/L. The Young’s modulus of borophene slightly decreases with temperature increasing, while the loading rate has almost no influence on the value of Young’s modulus of borophene. These findings are expected to provide important guidelines for realizing the practical applications of borophene based micro/nano electromechanical systems.
-
Keywords:
- borophene /
- mechanical properties /
- deformation mechanisms /
- molecular dynamics
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] Bianco E, Butler S, Jiang S, Restrepo O D, Windl W, Goldberger J E 2013 ACS nano 7 4414
Google Scholar
[3] Vogt P, De Padova P, Quaresima C, Avila J, Frantzeskakis E, Asensio M C, Resta A, Ealet B, Le Lay G 2012 Phys. Rev. Lett. 108 155501
Google Scholar
[4] Bertolazzi S, Brivio J, Kis A 2011 ACS Nano 5 9703
Google Scholar
[5] Liu H, Neal A T, Zhu Z, Luo Z, Xu X, Tománek D, Ye P D 2014 ACS nano 8 4033
Google Scholar
[6] Feng B J, Zhang J, Zhong Q, Li W B, Li S, Li H, Cheng P, Meng S, Chen L, Wu K H 2016 Nat. Chem. 8 564
[7] Lau K C, Pati R, Pandey R, Pineda A C 2006 Chem. Phys. Lett. 418 549
Google Scholar
[8] Kunstmann J, Quandt A 2006 Phys. Rev. B 74 035413
Google Scholar
[9] Tang H, Ismail-Beigi S 2007 Phys. Rev. Lett. 99 115501
Google Scholar
[10] Yang X B, Ding Y, Ni J 2008 Phys. Rev. B 77 041402(R
[11] Mannix A J, Zhou X F, Kiraly B, Wood J D, Alducin D, Myers B D, Liu X, Fisher B L, Santiago U, Guest J R, Yacaman M J, Ponce A, Oganov A R, Hersam M C, Guisinger N P 2015 Science 350 1513
Google Scholar
[12] Feng B J, Zhang J, Zhong Q, Li W B, Li S, Li H, Cheng P, Meng S, Chen L, Wu K H 2016 Nat. Chem. 8 563
Google Scholar
[13] 李文辉, 陈岚, 吴克辉 2022 71 108104
Li W H, Chen L, Wu K H 2022 Acta Phys. Sin. 71 108104
[14] Zhang Z H, Yang Y, Penev E S, Yakobson B I 2017 Adv. Funct. Mater. 27 1605059
Google Scholar
[15] Kong L J, Liu L R, Chen L, Zhong Q, Cheng P, Li H, Zhang Z H, Wu K H 2019 Nanoscale 11 15605
Google Scholar
[16] Zhong H X, Huang K X, Yu G D, Yuan S J 2018 Phys. Rev. B 98 054104
Google Scholar
[17] Peng B, Zhang H, Shao H, Xu Y, Zhang R, Zhu H 2016 J. Mater. Chem. C 4 3592
Google Scholar
[18] Mortazavi B, Rahaman O, Dianat A, Rabczuk T 2016 Phys. Chem. Chem. Phys. 18 27405
Google Scholar
[19] Wang Z, Lu T Y, Wang H Q, Feng Y P, Zheng J C 2016 Phys. Chem. Chem. Phys. 18 31424
Google Scholar
[20] Wang H F, Li Q F, Gao Y, Miao F, Zhou X F, Wan X G 2016 New J. Phys. 18 073016
Google Scholar
[21] Faghihnasiri M, Jafari H, Ramazani A, Shabani M, Estalaki S M, Larson R G 2019 J. Appl. Phys. 125 145107
Google Scholar
[22] Xiao R C, Shao D F, Lu W J, Lv H Y, Li J Y, Sun Y P 2016 Appl. Phys. Lett. 109 122604
[23] Giannopoulos G I 2017 Comp. Mater. Sci. 129 304
Google Scholar
[24] Zhou Y P, Jiang J W 2017 Sci. Rep. 7 45516
Google Scholar
[25] Pham V T, Fang T H 2020 Thin Solid Films 709 138197
Google Scholar
[26] Pham V T, Fang T H 2021 Sci. Rep. 11 12123
Google Scholar
[27] Sha Z D, Pei Q X, Zhou K, Dong Z L, Zhang Y W 2018 Extreme Mech. Lett. 19 39
Google Scholar
[28] Ayodhya D, Veerabhadram G 2020 Flatchem 19 100150
Google Scholar
[29] Mannix A J, Zhang Z, Guisinger N P, Yakobson B I, Hersam M C 2018 Nat. Nanotechnol. 13 444
Google Scholar
[30] Kaneti Y V, Benu D P, Xu X, Yuliarto B, Yamauchi Y, Golberg D 2022 Chem. Rev. 122 1000
Google Scholar
[31] Rubab A, Baig N, Sher M, Sohail M 2020 Chem. Eng. J. 401 126109
Google Scholar
[32] Duo Y H, Xie Z J, Wang L D, Abbasi N M, Yang T Q, Li Z H, Hu G X, Zhang H 2021 Coordin. Chem. Rev. 427 213549
Google Scholar
[33] Hoover W G 1985 Phys. Rev. A Gen. Phys. 31 1695
Google Scholar
[34] Swope W C, Andersen H C, Berens P H, Wilson K R 1982 J. Chem. Phys. 76 637
Google Scholar
[35] Erhart P, Albe K 2005 Phys. Rev. B 71 035211
Google Scholar
[36] Vodenitcharova T, Zhang L C 2004 Phys. Rev. B 69 115410
Google Scholar
[37] Tran T B T, Fang T H, Nguyen V T, Pham V T 2021 Comp. Mater. Sci. 197 110624
Google Scholar
[38] Subramaniyan A K, Sun C T 2008 Int. J. Solids Struct. 45 4340
Google Scholar
[39] 赵亚溥2014 纳米与介观力学(北京: 科学出版社) 第14页
Zhao Y P 2014 Nano and Mesoscopic Mechanics (Beijing: Science Press) p14
[40] Min K, Aluru N R 2011 Appl. Phys. Lett. 98 013113
Google Scholar
[41] Lee C, Wei X, Kysar J W, Hone J 2008 Science 321 385
Google Scholar
[42] Zhou L X, Wang Y G, Cao G X 2013 J. Phys. Condens. Matter 25 475303
Google Scholar
[43] Wang V, Geng W T 2017 J. Phys. Chem. C 121 10224
Google Scholar
[44] Peng B, Zhang H, Shao H Z, Ning Z Y, Xu Y F, Ni G, Lu H L, Zhang D W, Zhu H Y 2017 Mater. Res. Lett. 5 399
Google Scholar
[45] Cao G X, Gao H J 2019 Prog. Mater. Sci. 103 558
Google Scholar
[46] 周立新, 曹国鑫 2018 力学进展 48 201804
Zhou L X, Cao G X 2018 Adv. Mech. 48 201804
[47] Bui T X, Fang T H, Lee C I 2021 Nanotechnology 32 165704
Google Scholar
[48] Han T W, Li R, Zhang X Y, Scarpa F 2023 Mech. Mater. 180 104628
Google Scholar
[49] Tan X J, Wu J, Zhang K W, Peng X Y, Sun L Z, Zhong J X 2013 Appl. Phys. Lett. 102 071908
Google Scholar
[50] Xiang L, Ma S Y, Wang F, Zhang K W 2015 J. Phys. D. Appl. Phys. 48 395305
Google Scholar
-
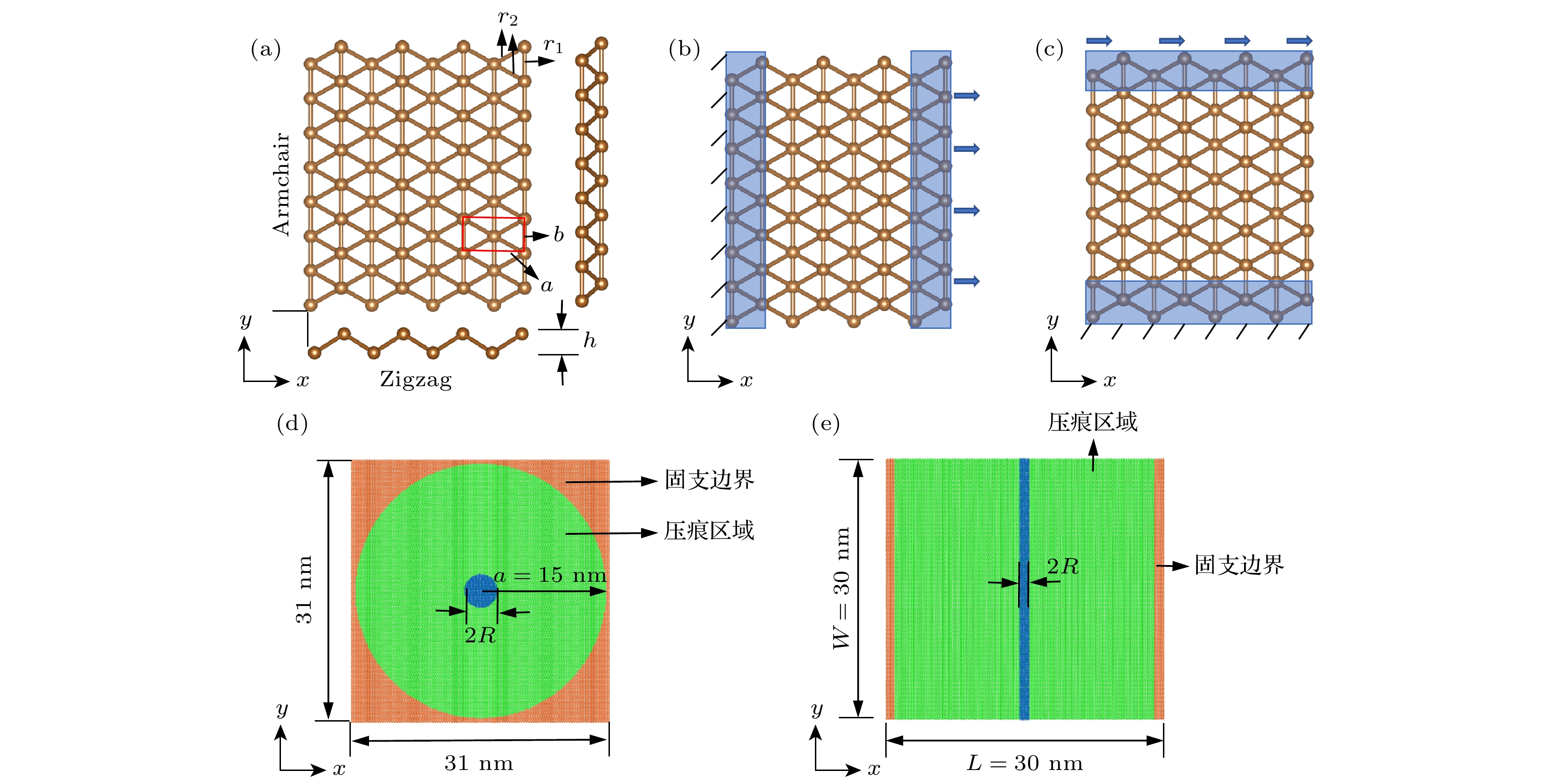
图 1 硼烯原子结构示意图以及分子动力学模拟模型 (a) 硼烯俯视图和侧视图; (b) 拉伸模型; (c) 剪切模型; (d) 球形压头纳米压痕模型; (e) 圆柱形压头纳米压痕模型
Figure 1. Schematics of simulation models and atomic structures for borophene: (a) Top view and side view of borophene; (b) stretching model of borophene; (c) shearing model of borophene; (d) spherical indentation model of borophene; (e) cylindrical indentation model of borophene.
图 2 硼烯在拉伸和剪切载荷下的力学响应规律 (a)拉伸应力-应变曲线; (b)剪切应力-剪切应变曲线; (c)强度和(d)弹性模量随温度的变化规律; (e) 强度和(f)弹性模量随应变率的变化规律
Figure 2. Mechanicsl response of borophene under tensile and shear load: (a) Tensile stress-strain curves of borophene; (b) shear stress-strain curves of borophene; temperature-dependence of the (c) tensile/shear stress and (d) tensile Young’s modulus/shear modulus for borophene; (e) tensile/shear stress and (f) tensile Young’s modulus/shear modulus of borophene as a function of strain rate.
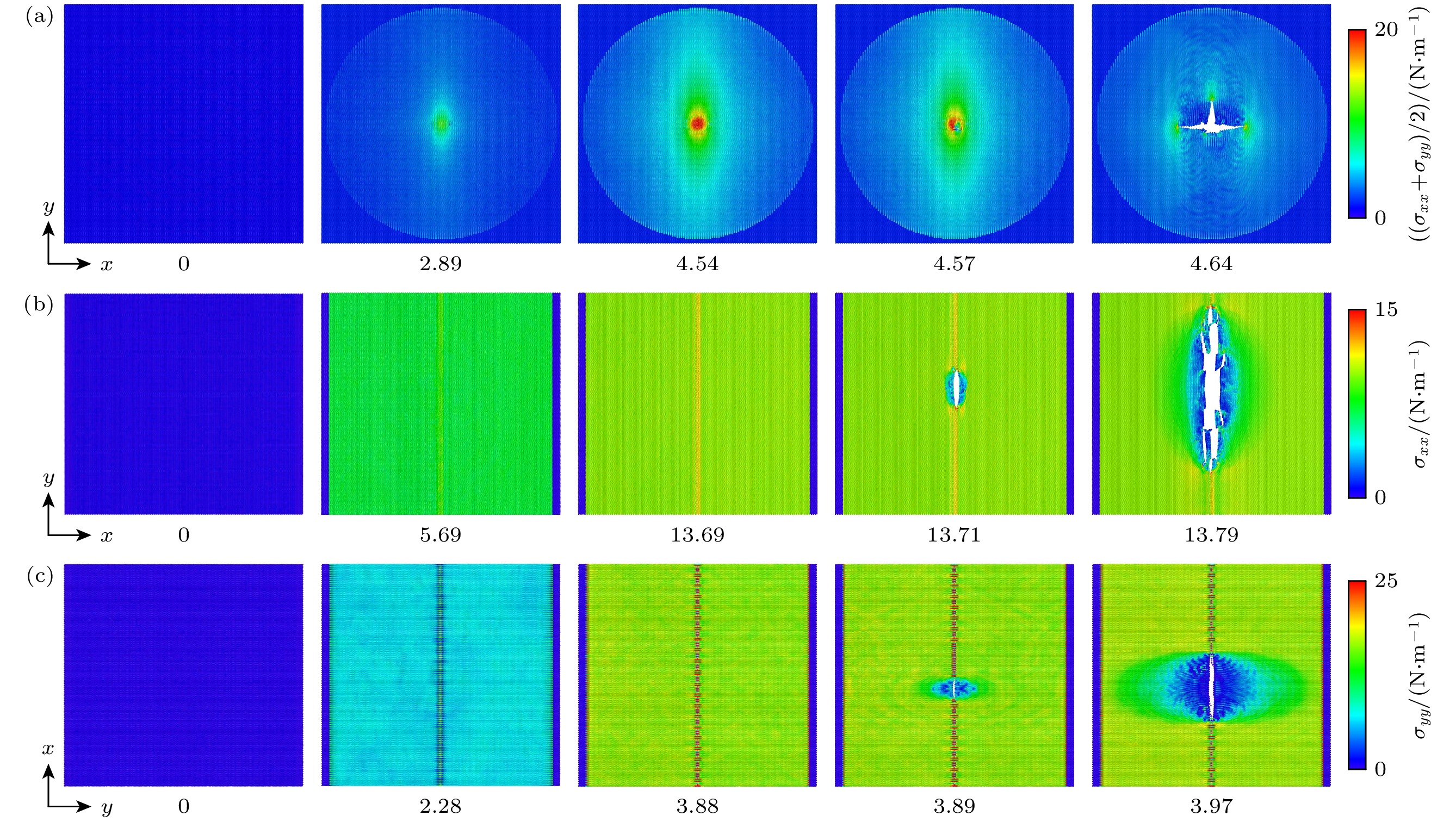
图 3 硼烯在拉伸和剪切载荷作用下的变形过程和应力分布图 (a) 沿锯齿方向拉伸; (b)沿扶手椅方向拉伸; (c)沿锯齿方向剪切; (d)沿扶手椅方向剪切
Figure 3. Deformation process and stress distribution of borophene under tensile and shear loads: (a) Tension along zigzag direction; (b) tension along armchair direction; (c) shear along zigzag direction; (d) shear along armchair direction.
图 4 硼烯在不同载荷作用下的键长随应变/压入深度的变化规律 (a) 沿锯齿方向拉伸; (b)沿扶手椅方向拉伸; (c) 沿锯齿方向剪切; (d) 球形压头压痕实验; (e) 圆柱形压头沿扶手椅方向压痕; (f) 圆柱形压头沿锯齿方向压痕
Figure 4. (a) Change of bond length of borophene with strain/indentation depth under different loads: (a) Tension along zigzag direction; (b) tension along armchair direction; (c) shear along zigzag direction; (d) indentation under spherical indenter; (e) indentation under cylindrical indenter along armchair direction; (f) indentation under cylindrical indenter along zigzag direction.
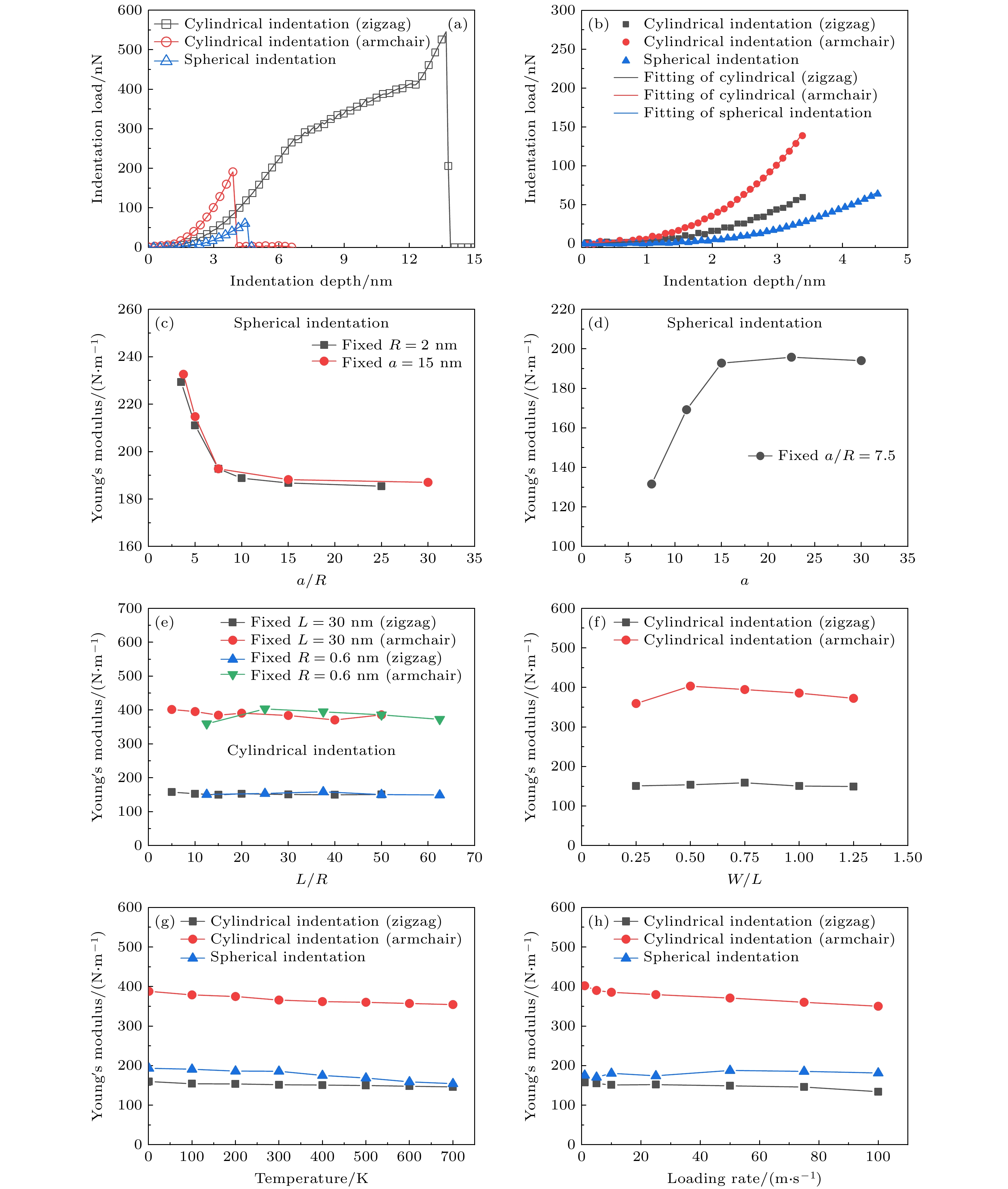
图 5 硼烯的纳米压痕结果以及不同压痕参数的影响 (a)硼烯在不同压头下的载荷-位移曲线; (b)利用(3)式和(5)式分别对初始阶段的拟合曲线; (c) 杨氏模量与a/R的关系曲线; (d) 杨氏模量与a的关系曲线; (e) 杨氏模量与L/R的关系曲线; (f) 杨氏模量与W/L的关系曲线; (g) 杨氏模量与温度的关系曲线; (h)杨氏模量与加载速率的关系曲线
Figure 5. Nanoindentation results of borophene and the influence of various indentation parameters: (a) Force-indentation depth curves for borophene under spherical and cylindrical indentation; (b) the fitting of the initial stage of the curves by Eq. (3) for spherical and Eq. (5) for cylindrical indentation; (c) Young’s modulus vs. a/R under spherical indentation; (d) Young’s modulus vs. a under spherical indentation with fixed a/R; (e) Young’s modulus vs. L/R under cylindrical indentation; (f) Young’s modulus vs. W/L under cylindrical indentation; (g) Young’s modulus vs. temperature; (h) Young’s modulus vs. loading rate.
图 6 硼烯在不同压痕深度下的面内应力分布图 (a) 球形压头; (b) 圆柱形压头沿扶手椅方向压痕; (c) 圆柱形压头沿锯齿方向压痕
Figure 6. In-plane stress distribution of borophene under: (a) Spherical indentation; (b) cylindrical indentation along armchair direction; (c) cylindrical indentation along zigzag direction at various indentation depths.
表 1 硼烯的拉伸和剪切力学性能参数对比一览表
Table 1. Comparison of tensile and shear mechanical parameters of borophene with previous MD and DFT calculations.
研究方法 $ {\varepsilon }_{x} $ $ {\varepsilon }_{y} $ $ {\sigma }_{x}/ $(N·m–1) $ {\sigma }_{y}/ $(N·m–1) $ {Y}_{x} $/(N·m–1) $ {Y}_{y} $/(N·m–1) $ G $/(N·m–1) MD(1 K)本文 0.15 0.08 15.53 24.07 158 379 76/77 MD(300 K)本文 0.10 0.05 12.03 15.18 149 355 68/71 DFT[11] 170 397 94 DFT[14] 163 399 MD[24] 1 K 0.15 0.10 12 24 163 394 MD[24] 300 K 0.06 0.05 7 14 144 345 MD[27] 300 K 0.102 0.087 11.2 26.8 150.8 357.9 DFT[20] 0.15 0.08 12.98 20.26 166 389 DFT[16] 158 397 86.5 DFT[44] 154 382 76.4 DFT[18] 0.145 0.105 14 22.8 163 382 DFT[43] 162 377 84 
DownLoad: CSV
表 2 硼烯在球形压头和圆柱形压头作用下的压痕力学参数
Table 2. Indentation mechanical parameters of borophene under spherical and cylindrical indenters.
压头类型 $ {\delta }_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $/nm $ {P}_{{\mathrm{m}}{\mathrm{a}}{\mathrm{x}}} $/nN $ \sigma $/(N·m–1) Y/(N·m–1) 球形压头 4.54 64 15.64 193 圆柱形压头(平行于扶手椅方向) 13.69 546 12.65 160 圆柱形压头(平行于锯齿方向) 3.88 191 25.34 388
DownLoad: CSV
-
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] Bianco E, Butler S, Jiang S, Restrepo O D, Windl W, Goldberger J E 2013 ACS nano 7 4414
Google Scholar
[3] Vogt P, De Padova P, Quaresima C, Avila J, Frantzeskakis E, Asensio M C, Resta A, Ealet B, Le Lay G 2012 Phys. Rev. Lett. 108 155501
Google Scholar
[4] Bertolazzi S, Brivio J, Kis A 2011 ACS Nano 5 9703
Google Scholar
[5] Liu H, Neal A T, Zhu Z, Luo Z, Xu X, Tománek D, Ye P D 2014 ACS nano 8 4033
Google Scholar
[6] Feng B J, Zhang J, Zhong Q, Li W B, Li S, Li H, Cheng P, Meng S, Chen L, Wu K H 2016 Nat. Chem. 8 564
[7] Lau K C, Pati R, Pandey R, Pineda A C 2006 Chem. Phys. Lett. 418 549
Google Scholar
[8] Kunstmann J, Quandt A 2006 Phys. Rev. B 74 035413
Google Scholar
[9] Tang H, Ismail-Beigi S 2007 Phys. Rev. Lett. 99 115501
Google Scholar
[10] Yang X B, Ding Y, Ni J 2008 Phys. Rev. B 77 041402(R
[11] Mannix A J, Zhou X F, Kiraly B, Wood J D, Alducin D, Myers B D, Liu X, Fisher B L, Santiago U, Guest J R, Yacaman M J, Ponce A, Oganov A R, Hersam M C, Guisinger N P 2015 Science 350 1513
Google Scholar
[12] Feng B J, Zhang J, Zhong Q, Li W B, Li S, Li H, Cheng P, Meng S, Chen L, Wu K H 2016 Nat. Chem. 8 563
Google Scholar
[13] 李文辉, 陈岚, 吴克辉 2022 71 108104
Li W H, Chen L, Wu K H 2022 Acta Phys. Sin. 71 108104
[14] Zhang Z H, Yang Y, Penev E S, Yakobson B I 2017 Adv. Funct. Mater. 27 1605059
Google Scholar
[15] Kong L J, Liu L R, Chen L, Zhong Q, Cheng P, Li H, Zhang Z H, Wu K H 2019 Nanoscale 11 15605
Google Scholar
[16] Zhong H X, Huang K X, Yu G D, Yuan S J 2018 Phys. Rev. B 98 054104
Google Scholar
[17] Peng B, Zhang H, Shao H, Xu Y, Zhang R, Zhu H 2016 J. Mater. Chem. C 4 3592
Google Scholar
[18] Mortazavi B, Rahaman O, Dianat A, Rabczuk T 2016 Phys. Chem. Chem. Phys. 18 27405
Google Scholar
[19] Wang Z, Lu T Y, Wang H Q, Feng Y P, Zheng J C 2016 Phys. Chem. Chem. Phys. 18 31424
Google Scholar
[20] Wang H F, Li Q F, Gao Y, Miao F, Zhou X F, Wan X G 2016 New J. Phys. 18 073016
Google Scholar
[21] Faghihnasiri M, Jafari H, Ramazani A, Shabani M, Estalaki S M, Larson R G 2019 J. Appl. Phys. 125 145107
Google Scholar
[22] Xiao R C, Shao D F, Lu W J, Lv H Y, Li J Y, Sun Y P 2016 Appl. Phys. Lett. 109 122604
[23] Giannopoulos G I 2017 Comp. Mater. Sci. 129 304
Google Scholar
[24] Zhou Y P, Jiang J W 2017 Sci. Rep. 7 45516
Google Scholar
[25] Pham V T, Fang T H 2020 Thin Solid Films 709 138197
Google Scholar
[26] Pham V T, Fang T H 2021 Sci. Rep. 11 12123
Google Scholar
[27] Sha Z D, Pei Q X, Zhou K, Dong Z L, Zhang Y W 2018 Extreme Mech. Lett. 19 39
Google Scholar
[28] Ayodhya D, Veerabhadram G 2020 Flatchem 19 100150
Google Scholar
[29] Mannix A J, Zhang Z, Guisinger N P, Yakobson B I, Hersam M C 2018 Nat. Nanotechnol. 13 444
Google Scholar
[30] Kaneti Y V, Benu D P, Xu X, Yuliarto B, Yamauchi Y, Golberg D 2022 Chem. Rev. 122 1000
Google Scholar
[31] Rubab A, Baig N, Sher M, Sohail M 2020 Chem. Eng. J. 401 126109
Google Scholar
[32] Duo Y H, Xie Z J, Wang L D, Abbasi N M, Yang T Q, Li Z H, Hu G X, Zhang H 2021 Coordin. Chem. Rev. 427 213549
Google Scholar
[33] Hoover W G 1985 Phys. Rev. A Gen. Phys. 31 1695
Google Scholar
[34] Swope W C, Andersen H C, Berens P H, Wilson K R 1982 J. Chem. Phys. 76 637
Google Scholar
[35] Erhart P, Albe K 2005 Phys. Rev. B 71 035211
Google Scholar
[36] Vodenitcharova T, Zhang L C 2004 Phys. Rev. B 69 115410
Google Scholar
[37] Tran T B T, Fang T H, Nguyen V T, Pham V T 2021 Comp. Mater. Sci. 197 110624
Google Scholar
[38] Subramaniyan A K, Sun C T 2008 Int. J. Solids Struct. 45 4340
Google Scholar
[39] 赵亚溥2014 纳米与介观力学(北京: 科学出版社) 第14页
Zhao Y P 2014 Nano and Mesoscopic Mechanics (Beijing: Science Press) p14
[40] Min K, Aluru N R 2011 Appl. Phys. Lett. 98 013113
Google Scholar
[41] Lee C, Wei X, Kysar J W, Hone J 2008 Science 321 385
Google Scholar
[42] Zhou L X, Wang Y G, Cao G X 2013 J. Phys. Condens. Matter 25 475303
Google Scholar
[43] Wang V, Geng W T 2017 J. Phys. Chem. C 121 10224
Google Scholar
[44] Peng B, Zhang H, Shao H Z, Ning Z Y, Xu Y F, Ni G, Lu H L, Zhang D W, Zhu H Y 2017 Mater. Res. Lett. 5 399
Google Scholar
[45] Cao G X, Gao H J 2019 Prog. Mater. Sci. 103 558
Google Scholar
[46] 周立新, 曹国鑫 2018 力学进展 48 201804
Zhou L X, Cao G X 2018 Adv. Mech. 48 201804
[47] Bui T X, Fang T H, Lee C I 2021 Nanotechnology 32 165704
Google Scholar
[48] Han T W, Li R, Zhang X Y, Scarpa F 2023 Mech. Mater. 180 104628
Google Scholar
[49] Tan X J, Wu J, Zhang K W, Peng X Y, Sun L Z, Zhong J X 2013 Appl. Phys. Lett. 102 071908
Google Scholar
[50] Xiang L, Ma S Y, Wang F, Zhang K W 2015 J. Phys. D. Appl. Phys. 48 395305
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1600
- PDF Downloads: 43
- Cited By: 0
