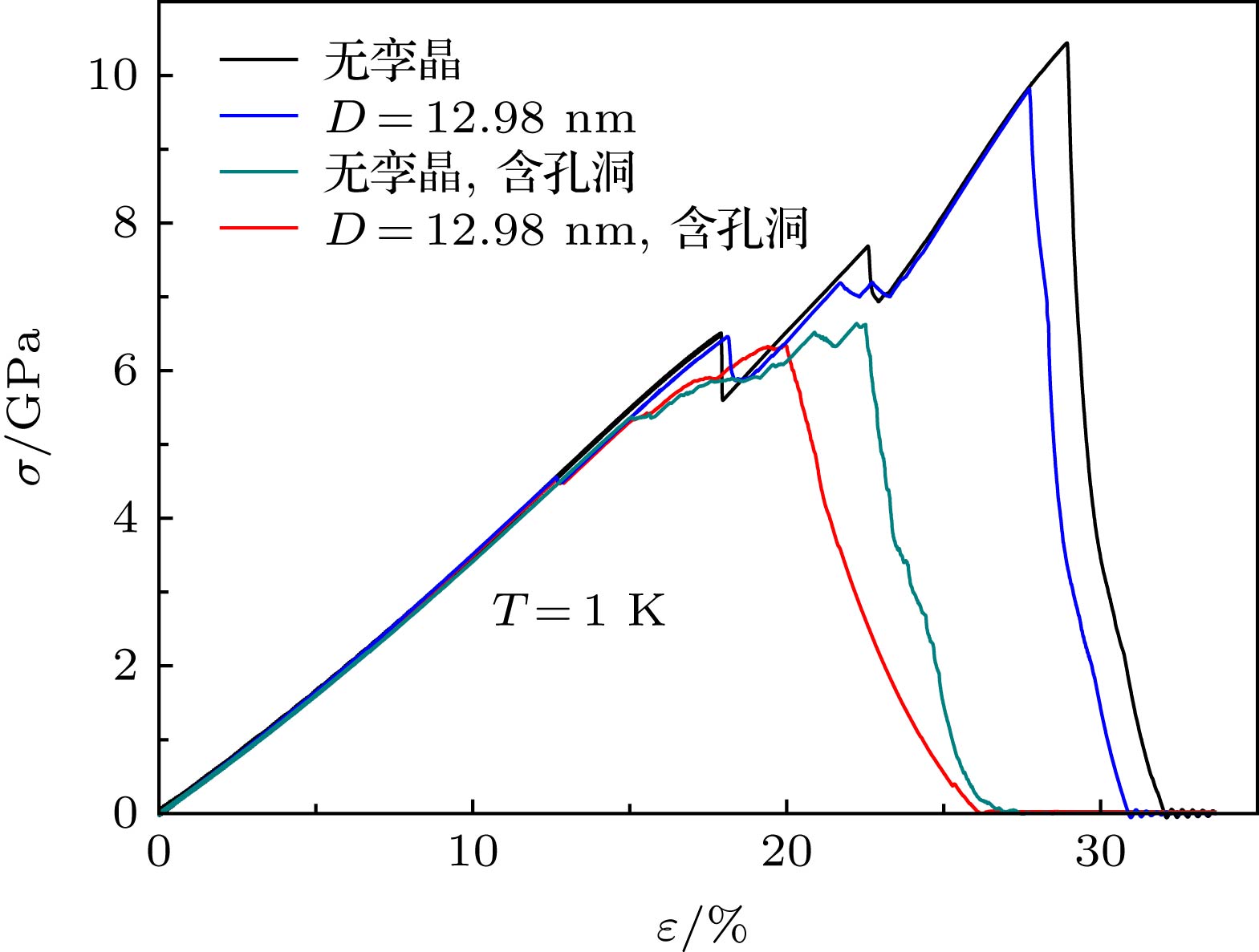
-
孪晶界是单层过渡金属二硫族化物材料中的一种重要结构缺陷. 本文通过分子动力学模拟结合Stillinger-Weber势函数研究单轴拉伸过程中孪晶界对单层MoS2力学行为的影响. 结果表明: 1)孪晶界能够诱发裂纹在孪晶界附近形核, 并促使裂纹沿界面扩展, 从而降低晶体的断裂应变; 2)温度的升高能够加剧孪晶界附近的裂纹形核过程, 从而进一步减弱单层MoS2的断裂强度和断裂应变; 3)孔洞能够造成应力集中, 从而进一步触发断裂过程, 但孪晶界能够阻碍孔洞应力场的扩散, 从而延缓单层MoS2材料的断裂过程; 4)孪晶片层间距对单层MoS2断裂应变具有重要影响, 特别是对于含孔洞的单层MoS2材料, 材料断裂应变能够随着片层间距的减小而显著提高.Grain boundary (GB) plays a key role in determining the electrical and mechanical properties of mono-layer transition metal dichalcogenide (TMDC), however it is still a challenge to uncover the GB-mediated TMDC material experimentally. In this paper, the effect of twin boundary on the tensile behaviors of mono-layer MoS2 is investigated by using the molecular dynamics simulation combined with the Stillinger-Weber potential. Mono-layer MoS2 model under the varied size and temperature condition is adopted. Stress calculation is performed by using Virial theorem. The results are obtained as follows. 1) Twin boundary promotes the brittle fracture of an undefected mono-layer MoS2 sheet by inducing the nucleation of the crack near boundaries, thus the fracture strength and strain are weakened. 2) Increasing the ambient temperature from 1 K to 600 K, the crack nucleation process near the twin boundary is intensely accelerated, and the fracture strength and strain are further declined. 3) Twin lamellar spacing also plays an important role in the tensile process of mono-layer MoS2, and the specimen with dense twin boundary, especially with void, shows higher fracture strain. 4) Stress analysis at an atomic level outlines the stress concentration caused by voids and the shielding effect of twin boundary. Because of the interactions between voids and twin boundary, the fracture strength and strain of a voided mono-layer MoS2 sheet can be greatly improved.
-
Keywords:
- mono-layer MoS2 /
- twin boundary /
- mechanical properties /
- molecular dynamics
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] Geim A K, Novoselov K S 2007 Nat. Mater. 6 183
Google Scholar
[3] Radisavljevic B, Radenovic A, Brivio J, Giacometti V, Kis A 2011 Nat. Nanotech. 6 147
Google Scholar
[4] Wang Q H, Kalantar-Zadeh K, Kis A, Coleman J N, Strano M S 2012 Nat. Nanotech. 7 699
Google Scholar
[5] Yin X B, Ye Z L, Chenet D A, Ye Y, Brien K O, Hone J C, Zhang X 2014 Science 344 488
Google Scholar
[6] 魏争, 王琴琴, 郭玉拓, 李佳蔚, 时东霞, 张广宇 2018 67 128103
Google Scholar
Wei Z, Wang Q Q, Guo Y T, Li J W, Shi D X, Zhang G Y 2018 Acta Phys. Sin. 67 128103
Google Scholar
[7] 吴木生, 徐波, 刘刚, 欧阳楚英 2012 61 227102
Google Scholar
Wu M S, Xu B, Liu G, Ouyang C Y 2012 Acta Phys. Sin. 61 227102
Google Scholar
[8] Tao P, Guo H, Yang T, Zhang Z 2014 J. Appl. Phys. 115 054305
Google Scholar
[9] Dang K Q, Spearot D E 2014 J. Appl. Phys. 116 013508
Google Scholar
[10] Casillas G, Santiago U, Barron H, Alducin D, Ponce A, José-Yacamán M 2015 J. Phys. Chem. C 119 710
Google Scholar
[11] 李明林, 万亚玲, 胡建玥, 王卫东 2016 65 176201
Google Scholar
Li M L, Wan Y L, Hu J Y, Wang W D 2016 Acta Phys. Sin. 65 176201
Google Scholar
[12] Wang W D, Li L L, Yang C G, Soler-Crespo R A, Meng Z X, Li M L, Zhang X, Keten S, Espinosa H D 2017 Nanotechnology 28 164005
Google Scholar
[13] Wu J Y, Cao P Q, Zhang Z S, Ning F L, Zheng S S, He J Y, Zhang Z L 2018 Nano Lett. 18 1543
Google Scholar
[14] Zhang R, Koutsos V, Cheung R 2016 Appl. Phys. Lett. 108 042104
Google Scholar
[15] Hao S, Yang B, Gao Y 2017 Appl. Phys. Lett. 110 153105
Google Scholar
[16] Yang Y, Li X, Wen M, Hacopian E, Chen W, Gong Y, Zhang J, Li B, Zhou W, Ajayan P M, Chen Q, Zhu T, Lou J 2017 Adv. Mater. 29 1604201
Google Scholar
[17] Yun W S, Han S W, Hong S C, Kim I G, Lee J D 2012 Phys. Rev. B 85 033305
Google Scholar
[18] Wang X, Tabarraei A, Spearot D E 2015 Nanotechnology 26 175703
Google Scholar
[19] Zhou W, Zou X, Najmaei S, Liu Z, Shi Y, Kong J, Lou J, Ajayan P M, Yakobson B I, Idrobo J C 2013 Nano Lett. 13 2615
Google Scholar
[20] Lin Z, Carvalho B R, Kahn E, Lü R, Rao R, Terrones H, Pimenta M A, Terrones M 2016 2D Mater. 3 022002
Google Scholar
[21] Ly T H, Chiu M H, Li M Y, Zhao J, Perello D J, Cichocka M O, Oh H M, Chae S H, Jeong, Hye Yun, Yao F, Li L J, Lee Y H 2014 ACS Nano 8 11401
Google Scholar
[22] Cheng J, Jiang T, Ji Q, Zhang Y, Li Z, Shan Y, Zhang Y, Gong X, Liu W, Wu S 2015 Adv. Mater. 27 4069
Google Scholar
[23] van der Zande A M, Huang P Y, Chenet D A, Berkelbach T C, You Y M, Lee G H, Heinz T F, Reichman D R, Muller D A, Hone J C 2013 Nat. Mater. 12 554
Google Scholar
[24] Barja S, Wickenburg S, Liu Z F, Zhang Y, Ryu H, Ugeda M M, Hussain Z, Shen Z X, Mo S K, Wong E, Salmeron M B, Wang F, Crommie M F, Ogletree D F, Neaton J B, Weber-Bargioni A 2016 Nat. Phys. 12 751
Google Scholar
[25] Hong J, Wang Y, Wang A, Lü D, Jin C, Xu Z, Probert M I J, Yuan J, Zhang Z 2017 Nanoscale 9 10312
Google Scholar
[26] Jiang J W, Park H S, Rabczuk T 2013 J. Appl. Phys. 114 064307
Google Scholar
[27] Stillinger F H, Weber T A 1985 Phys. Rev. B 31 5262
Google Scholar
[28] Plimpton S 1995 J. Comp. Phys. 117 1
Google Scholar
[29] Stukowski A 2010 Modelling Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[30] Xiong S, Cao G X 2015 Nanotechnology 26 185705
Google Scholar
[31] Du L J, Yu H, Xie L, Wu S, Wang S, Lu X, Liao M, Meng J, Zhao J, Zhang J, Zhu J, Chen P, Wang G, Yang R, Shi D, Zhang G 2016 Crystals 6 115
Google Scholar
[32] Dao M, Lu L, Asaro R J, De Hosson J T M, Ma E 2007 Acta Mater. 55 4041
Google Scholar
[33] Wang S S, Qin Z, Jung G S, Martin-Martinez F J, Zhang K, Buehler M J, Warner J H 2016 ACS Nano 10 9831
Google Scholar
[34] Peron-Luhrs V, Sansoz F, Noels L 2014 Acta Mater. 64 419
Google Scholar
[35] Dang K Q, Simpsona J P, Spearot D E 2014 Scripta Mater. 76 41
Google Scholar
[36] Lu L, Chen X, Huang X, Lu K 2009 Science 323 607
Google Scholar
-
图 1 单层MoS2的分子动力学模型 (a)含孪晶界; (b)不含孪晶界
Fig. 1. Molecular dynamics model of mono-layer MoS2: (a) With twin boundaries; (b) without twin boundary.
图 2 (a)应变能E; (b)应力σ, 其中ε表示应变
Fig. 2. (a) Strain energy E and (b) stress σ, where ε denotes strain.
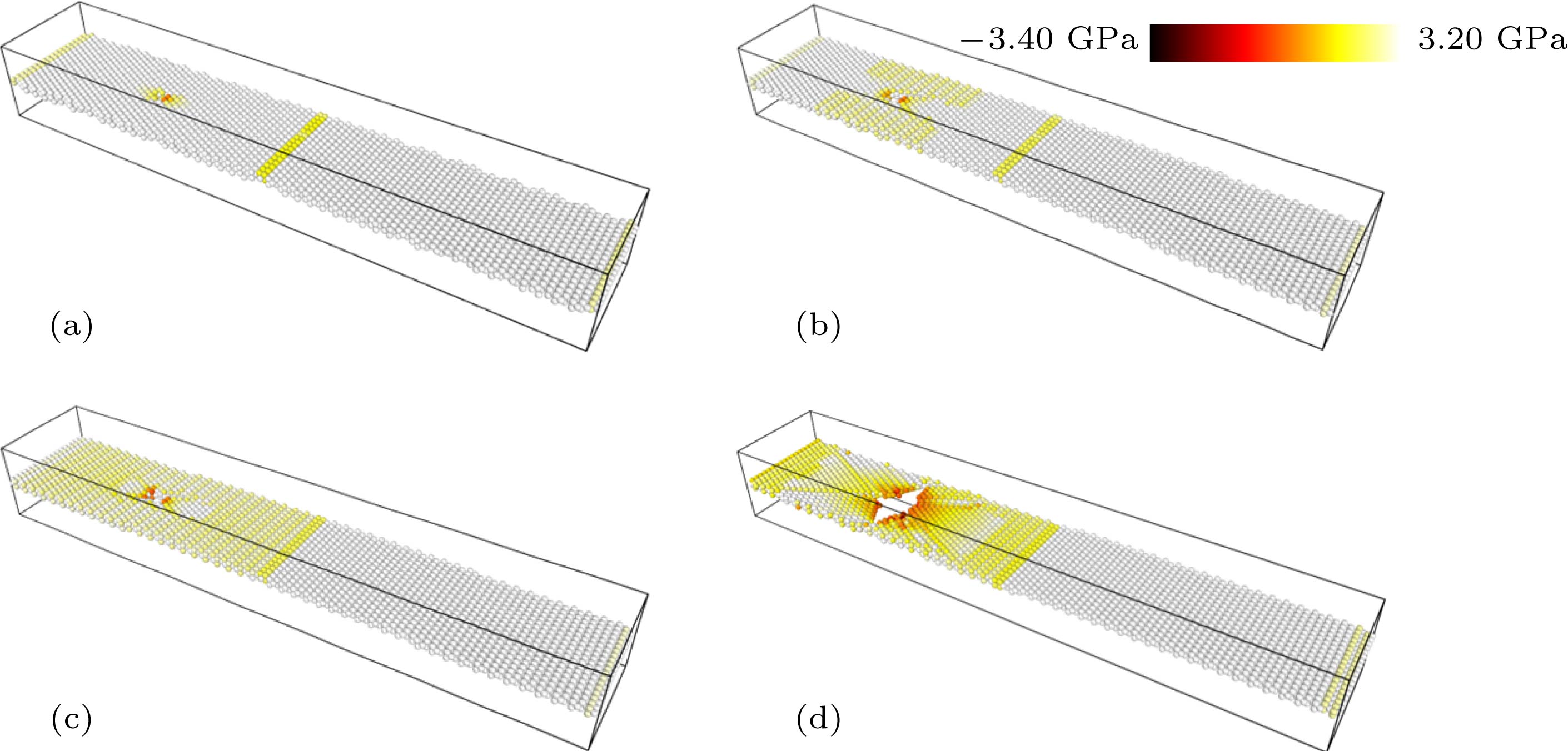
图 3 与拉伸曲线相对应的原子结构 (a)孪晶界, A点, ε = 27.74%; (b)孪晶界, B点, ε = 27.79%; (c)不含孪晶界, A点, ε = 28.94%; (d)不含孪晶界, B点, ε = 29.24%
Fig. 3. Atomic structures corresponding to the tensile curves: (a) With twin boundary, point A, ε = 27.74%; (b) with twin boundary, point B, ε = 27.79%; (c) without twin boundary, point A, ε = 28.94%; (d) without twin boundary, point B, ε = 29.24%.
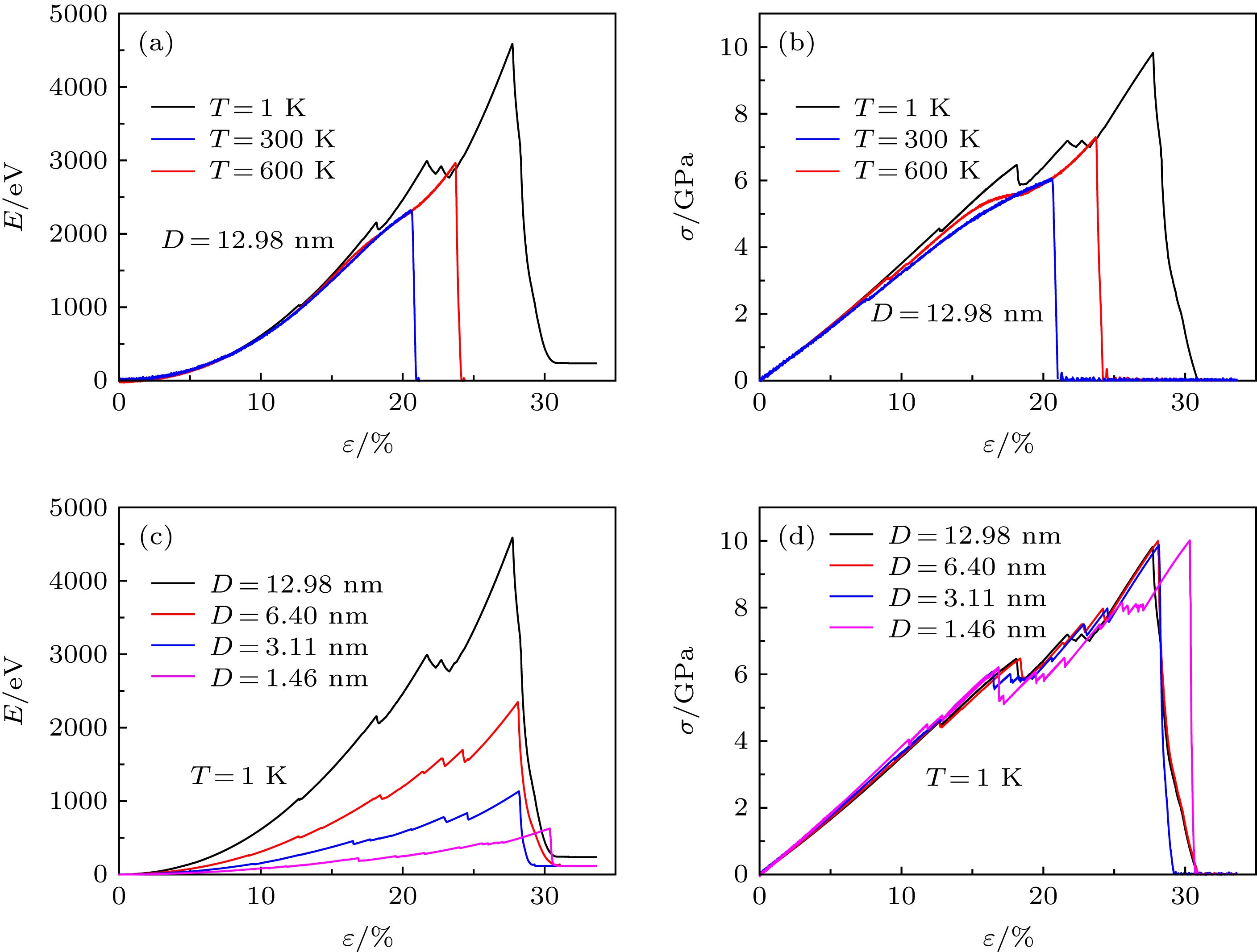
图 4 温度和孪晶界面间距的影响 (a)不同温度下的应变能; (b)不同温度下的应力; (c)不同孪晶片层间距下的应变能; (d)不同孪晶片层间距下的应力
Fig. 4. Effects of temperature and the twin lamellar spacing: (a) Effect of temperature on strain energy; (b) effect of temperature on stress; (c) effect of twin lamellar spacing effect on strain energy; (d) effect of twin lamellar spacing effect on stress.
图 6 不含孪晶界的带孔洞的单层MoS2 (a) ε = 0; (b) ε = 22.514%; (c) ε = 22.514%, 放大视图; (d) ε = 23.345%, 放大视图
Fig. 6. Voided mono-layer MoS2 without twin boundary: (a) ε = 0; (b) ε = 22.514%; (c) ε = 22.514%, enlarged view; (d) ε = 23.345%, enlarged view.
图 7 含孪晶界的带孔洞的单层MoS2 (a) ε = 0; (b) ε = 19.971%; (c) ε = 19.971%, 放大视图; (d) ε = 20.779%, 放大视图
Fig. 7. Voided mono-layer MoS2 with twin boundaries: (a) ε = 0; (b) ε = 19.971%; (c) ε = 19.971%, enlarged view; (d) ε = 20.779%, enlarged view.
图 8 带孔洞的含孪晶界模型断裂前后应力分布状态 (a) ε = 14.34%; (b) ε = 16.92%; (c) ε = 18.25%; (d) ε = 20.87%
Fig. 8. Distribution of tensile stress in the voided mono-layer MoS2 sheet with twin boundaries: (a) ε = 14.34%; (b) ε = 16.92%; (c) ε = 18.25%; (d) ε = 20.87%.
图 9 断裂应变εA与孪晶片层间距D的关联
Fig. 9. Correlation of the fracture strain εA and the twin lamellar spacing D.
表 1 模型平面内初始尺寸
Table 1. Initial in-plane size of model.
Lx/nm Ly/nm 含孪晶模型 25.96 5.70 不含孪晶模型 13.16 5.70 
下载: 导出CSV
-
[1] Novoselov K S, Geim A K, Morozov S V, Jiang D, Zhang Y, Dubonos S V, Grigorieva I V, Firsov A A 2004 Science 306 666
Google Scholar
[2] Geim A K, Novoselov K S 2007 Nat. Mater. 6 183
Google Scholar
[3] Radisavljevic B, Radenovic A, Brivio J, Giacometti V, Kis A 2011 Nat. Nanotech. 6 147
Google Scholar
[4] Wang Q H, Kalantar-Zadeh K, Kis A, Coleman J N, Strano M S 2012 Nat. Nanotech. 7 699
Google Scholar
[5] Yin X B, Ye Z L, Chenet D A, Ye Y, Brien K O, Hone J C, Zhang X 2014 Science 344 488
Google Scholar
[6] 魏争, 王琴琴, 郭玉拓, 李佳蔚, 时东霞, 张广宇 2018 67 128103
Google Scholar
Wei Z, Wang Q Q, Guo Y T, Li J W, Shi D X, Zhang G Y 2018 Acta Phys. Sin. 67 128103
Google Scholar
[7] 吴木生, 徐波, 刘刚, 欧阳楚英 2012 61 227102
Google Scholar
Wu M S, Xu B, Liu G, Ouyang C Y 2012 Acta Phys. Sin. 61 227102
Google Scholar
[8] Tao P, Guo H, Yang T, Zhang Z 2014 J. Appl. Phys. 115 054305
Google Scholar
[9] Dang K Q, Spearot D E 2014 J. Appl. Phys. 116 013508
Google Scholar
[10] Casillas G, Santiago U, Barron H, Alducin D, Ponce A, José-Yacamán M 2015 J. Phys. Chem. C 119 710
Google Scholar
[11] 李明林, 万亚玲, 胡建玥, 王卫东 2016 65 176201
Google Scholar
Li M L, Wan Y L, Hu J Y, Wang W D 2016 Acta Phys. Sin. 65 176201
Google Scholar
[12] Wang W D, Li L L, Yang C G, Soler-Crespo R A, Meng Z X, Li M L, Zhang X, Keten S, Espinosa H D 2017 Nanotechnology 28 164005
Google Scholar
[13] Wu J Y, Cao P Q, Zhang Z S, Ning F L, Zheng S S, He J Y, Zhang Z L 2018 Nano Lett. 18 1543
Google Scholar
[14] Zhang R, Koutsos V, Cheung R 2016 Appl. Phys. Lett. 108 042104
Google Scholar
[15] Hao S, Yang B, Gao Y 2017 Appl. Phys. Lett. 110 153105
Google Scholar
[16] Yang Y, Li X, Wen M, Hacopian E, Chen W, Gong Y, Zhang J, Li B, Zhou W, Ajayan P M, Chen Q, Zhu T, Lou J 2017 Adv. Mater. 29 1604201
Google Scholar
[17] Yun W S, Han S W, Hong S C, Kim I G, Lee J D 2012 Phys. Rev. B 85 033305
Google Scholar
[18] Wang X, Tabarraei A, Spearot D E 2015 Nanotechnology 26 175703
Google Scholar
[19] Zhou W, Zou X, Najmaei S, Liu Z, Shi Y, Kong J, Lou J, Ajayan P M, Yakobson B I, Idrobo J C 2013 Nano Lett. 13 2615
Google Scholar
[20] Lin Z, Carvalho B R, Kahn E, Lü R, Rao R, Terrones H, Pimenta M A, Terrones M 2016 2D Mater. 3 022002
Google Scholar
[21] Ly T H, Chiu M H, Li M Y, Zhao J, Perello D J, Cichocka M O, Oh H M, Chae S H, Jeong, Hye Yun, Yao F, Li L J, Lee Y H 2014 ACS Nano 8 11401
Google Scholar
[22] Cheng J, Jiang T, Ji Q, Zhang Y, Li Z, Shan Y, Zhang Y, Gong X, Liu W, Wu S 2015 Adv. Mater. 27 4069
Google Scholar
[23] van der Zande A M, Huang P Y, Chenet D A, Berkelbach T C, You Y M, Lee G H, Heinz T F, Reichman D R, Muller D A, Hone J C 2013 Nat. Mater. 12 554
Google Scholar
[24] Barja S, Wickenburg S, Liu Z F, Zhang Y, Ryu H, Ugeda M M, Hussain Z, Shen Z X, Mo S K, Wong E, Salmeron M B, Wang F, Crommie M F, Ogletree D F, Neaton J B, Weber-Bargioni A 2016 Nat. Phys. 12 751
Google Scholar
[25] Hong J, Wang Y, Wang A, Lü D, Jin C, Xu Z, Probert M I J, Yuan J, Zhang Z 2017 Nanoscale 9 10312
Google Scholar
[26] Jiang J W, Park H S, Rabczuk T 2013 J. Appl. Phys. 114 064307
Google Scholar
[27] Stillinger F H, Weber T A 1985 Phys. Rev. B 31 5262
Google Scholar
[28] Plimpton S 1995 J. Comp. Phys. 117 1
Google Scholar
[29] Stukowski A 2010 Modelling Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[30] Xiong S, Cao G X 2015 Nanotechnology 26 185705
Google Scholar
[31] Du L J, Yu H, Xie L, Wu S, Wang S, Lu X, Liao M, Meng J, Zhao J, Zhang J, Zhu J, Chen P, Wang G, Yang R, Shi D, Zhang G 2016 Crystals 6 115
Google Scholar
[32] Dao M, Lu L, Asaro R J, De Hosson J T M, Ma E 2007 Acta Mater. 55 4041
Google Scholar
[33] Wang S S, Qin Z, Jung G S, Martin-Martinez F J, Zhang K, Buehler M J, Warner J H 2016 ACS Nano 10 9831
Google Scholar
[34] Peron-Luhrs V, Sansoz F, Noels L 2014 Acta Mater. 64 419
Google Scholar
[35] Dang K Q, Simpsona J P, Spearot D E 2014 Scripta Mater. 76 41
Google Scholar
[36] Lu L, Chen X, Huang X, Lu K 2009 Science 323 607
Google Scholar
下载:
计量
- 文章访问数: 9677
- PDF下载量: 172
- 被引次数: 0
