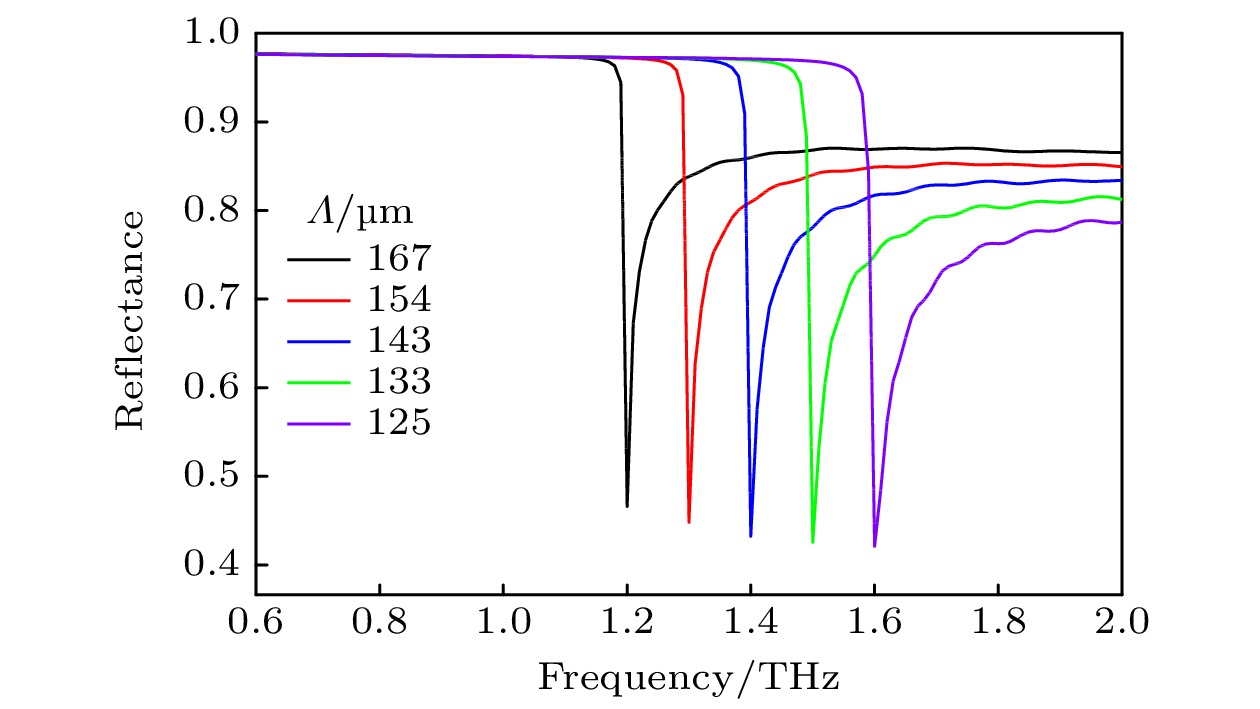
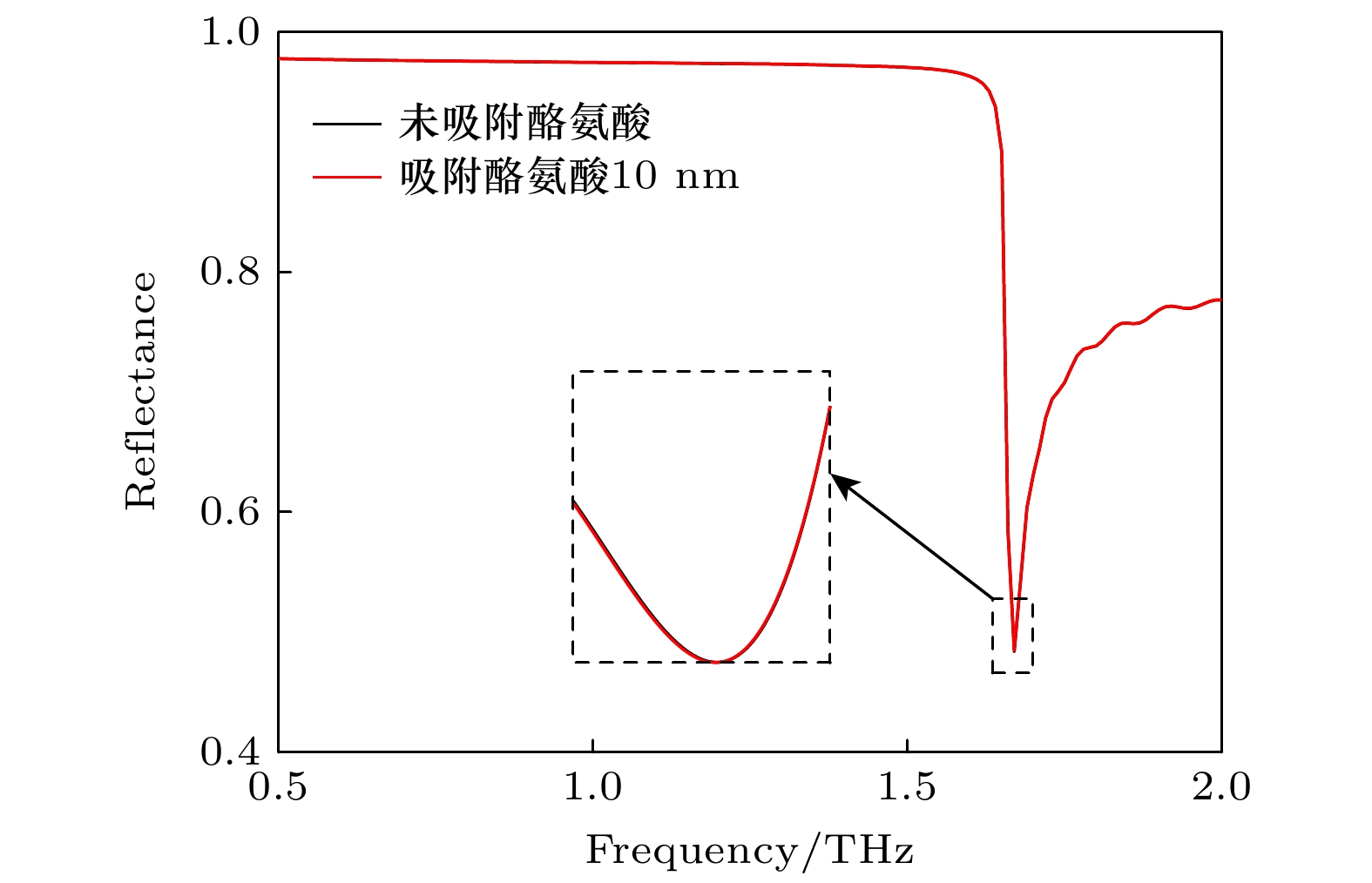
-
A grating-coupled terahertz (THz) surface plasmon polariton (SPP) resonant biochemical sensing structure is designed with simulation, which can be easily prepared by etching a submillimeter grating on the surface of indium antimonide (InSb) substrate. The simulation results based on the phase matching equation show that when the TM-polarized broadband terahertz collimated beam is incident on the InSb grating at a 30° angle, the low-frequency SPP and high-frequency SPP with opposite propagation directions can be simultaneously excited by the –1st and +1st order diffraction beams of the grating, respectively. Since the low-frequency SPP is easy to accurately measure with a commercial THz time-domain spectroscopy devices, the dependence of the resonance characteristics and sensing characteristics of low-frequency SPP on the grating structure parameters is systematically simulated in this paper. The simulation results show that the refractive-index sensitivity of the InSb grating-coupled THz-SPP resonant sensor chip decreases with the increase of the grating period, and is 1.05 THz/RIU at a grating period of 120 μm and an incident angle of 30°. Under these conditions, the sensor chip cannot make a detectable response to the monolayer adsorption of biomolecules, because the evanescent field penetration depth of the low-frequency SPP is much greater than the biomolecular size, resulting in insufficient field-biomolecular interaction at the surface. In order to detect biomolecules, a sensitivity enhancement method based on porous thin films is proposed and analyzed with simulation. The porous films enable not only to enrich biomolecules, but also to extend the interaction between THz-SPP and biomolecules from the molecular size to the entire film thickness, thereby improving the sensitivity of the sensor to biomolecular adsorption. Taking tyrosine adsorption as an example, the simulation results show that when the InSb grating is covered with a porous polymethyl methacrylate (PMMA) film with a thickness of 120 μm and a porosity of 0.4, the sensor sensitivity to tyrosine adsorption is 0.39 THz/unit volume fraction.
-
Keywords:
- terahertz surface plasmon resonance /
- InSb grating /
- biochemical sensing /
- porous materials
[1] 张喆, 柳倩, 祁志美 2013 62 060703
Google Scholar
Zhang Z, Liu Q, Qi Z M 2013 Acta Phys. Sin. 62 060703
Google Scholar
[2] Zhang C L, Liu Z W, Cai C, Yang Z H, Qi Z M 2022 Opt. Lett. 47 4155
Google Scholar
[3] Anker J N, Hall W P, Lyandres O, Shah N C, Zhao J, Van Duyne R P 2008 Nat. Mater. 7 442
Google Scholar
[4] 刘濮鲲, 黄铁军 2020 红外与毫米波学报 39 169
Google Scholar
Liu P K, Huang T J 2020 J. Infrared Millim. Waves 39 169
Google Scholar
[5] Pendry J B, Martín-Moreno L, Garcia-Vidal F J 2004 Science 305 847
Google Scholar
[6] Hibbins A P, Evans B R, Sambles J R 2005 Science 308 670
Google Scholar
[7] Williams C R, Andrews S R, Maier S A, Fernández-Domínguez A I, Martín-Moreno L, García-Vidal F J 2008 Nat. Photonics 2 175
Google Scholar
[8] Ng B H, Wu J F, Hanham S M, Fernández-Domínguez A I, Klein N, Liew Y F, Breese M B H, Hong M H, Maier S A 2013 Adv. Opt. Mater. 1 543
Google Scholar
[9] Ng B H, Hanham S M, Wu J F, Fernández-Domínguez A I, Klein N, Liew Y F, Breese M B H, Hong M H, Maier S A 2014 ACS Photonics 1 1059
Google Scholar
[10] Shen X P, Cui T J 2014 Laser Photonics Rev. 8 137
Google Scholar
[11] Chochol J, Postava K, Čada M, Vanwolleghem M, Halagačka L, Lampin J F, Pištora J 2016 AIP Adv. 6 115021
Google Scholar
[12] Marschall N, Fischer B, Queisser H J 1971 Phy. Rev. Lett. 27 95
Google Scholar
[13] Cheng B H, Ye Y S, Lan Y C, Tsai D P 2017 Sci. Rep. 7 6443
Google Scholar
[14] Chochol J, Postava K, Cada M, Pistora J 2017 Sci. Rep. 7 13117
Google Scholar
[15] Isaac T H, Barnes W L, Hendry E 2008 Appl. Phys. Lett. 93 241115
Google Scholar
[16] Madelung O 1991 Semiconductors: Group IV Elements and III-V Compounds (Berlin, Heidelberg: Springer) p147
[17] Shibayama J, Shimizu K, Yamauchi J, Nakano H 2016 J. Lightwave Technol. 34 2518
Google Scholar
[18] Tao H, Chieffo L R, Brenckle M A, Siebert S M, Liu M K, Strikwerda A C, Fan K B, Kaplan D L, Zhang X, Averitt R D, Omenetto F C 2011 Adv. Mater. 23 3197
Google Scholar
[19] Wang Y, Cui Z J, Zhu D Y, Wang X M, Chen S G, Nie P C 2019 Opt. Express 27 14133
Google Scholar
[20] Nie P C, Zhu D Y, Cui Z J, Qu F F, Lin L, Wang Y 2020 Sens. Actuator B-Chem. 307 127642
Google Scholar
[21] 向星诚, 马海贝, 王磊, 田达, 张伟, 张彩虹, 吴敬波, 范克斌, 金飚兵, 陈健, 吴培亨 2023 72 128701
Google Scholar
Xiang X, Ma H, Wang L, Tian D, Zhang W, Zhang C, Wu J, Fan K, Jin B, Chen J, Wu P 2023 Acta Phys. Sin. 72 128701
Google Scholar
[22] 岳伟伟, 王卫宁, 赵国忠, 张存林, 闫海涛 2005 54 3094
Google Scholar
Yue W W, Wang W N, Zhao G Z, Zhang C L, Yan H T 2005 Acta Phys. Sin. 54 3094
Google Scholar
[23] 王国阳, 白志晨, 王佳慧, 苏波, 张存林 2021 光谱学与光谱分析 41 1678
Google Scholar
Wang G Y, Bai Z C, Wang J H, Su B, Zhang C L 2021 Spectrosc. Spectral Anal. 41 1678
Google Scholar
-
图 1 使用Drude模型计算的InSb介电常数
Figure 1. InSb dielectric constant calculated with the Drude model.
图 2 光栅耦合原理示意图
Figure 2. Schematic diagram of grating coupling principle.
图 3 掺杂型InSb色散曲线
Figure 3. Dispersion curve of doped InSb.
图 4 低频SPP与高频SPP的共振频率随光栅周期的变化
Figure 4. Resonance frequency variation curves of low-frequency SPP and high-frequency SPP with grating period.
图 5 光栅高度为10 μm、占空比为0.4的条件下, THz反射谱随光栅周期的变化
Figure 5. Variation of THz reflection spectrum with grating period under the condition of a grating height of 10 μm and a duty cycle of 0.4.
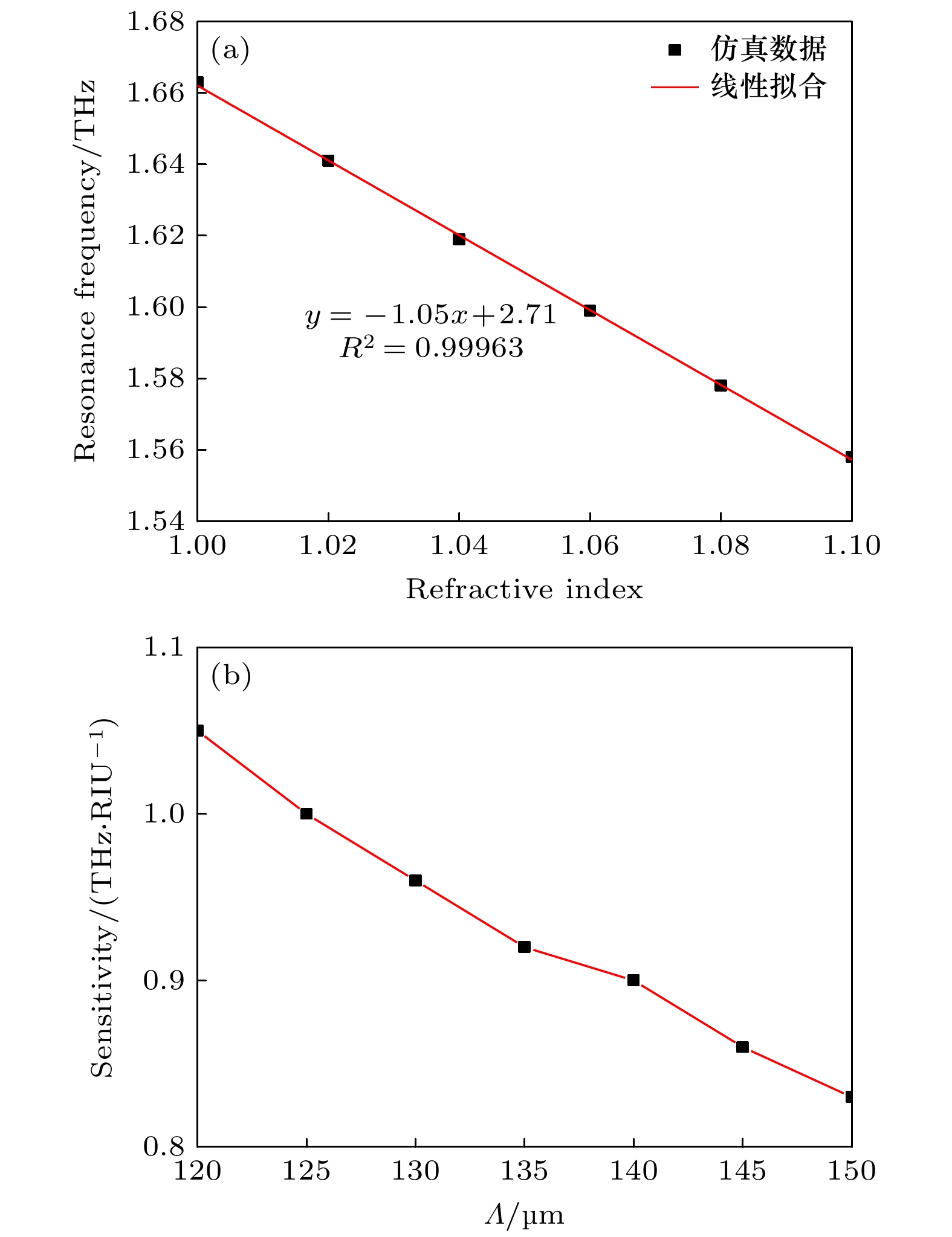
图 6 (a) 光栅周期为120 μm时共振频率随介质折射率的变化, 及线性拟合得到的折射率灵敏度; (b) 折射率灵敏度随光栅周期的变化
Figure 6. 6. (a) Resonance frequency variation curve with medium refractive index when the grating period is 120 μm, and the refractive index sensitivity obtained by linear fitting; (b) variation curve of refractive index sensitivity with grating period.
图 7 光栅表面吸附10 nm酪氨酸与未吸附时的THz反射谱
Figure 7. THz reflection spectrum of 10 nm tyrosine adsorbed on grating surface and without adsorption.
图 8 InSb光栅表面覆盖多孔PMMA薄膜制备流程示意图
Figure 8. Schematic diagram of the preparation process of InSb grating covered with porous PMMA film.
图 9 多孔PMMA等效折射率n随孔隙率P的变化
Figure 9. Variation curve of equivalent refractive index n of porous PMMA with porosity P.
图 10 在厚度h = 25 μm情况下的仿真结果 (a) 折射率灵敏度随多孔PMMA孔隙率P的变化; (b) 介质层平均电场模随多孔PMMA孔隙率P的变化; (c) 不同孔隙率下的场分布示意图; (d) 不同孔隙率下介质层与多孔PMMA层界面处的电场强度; 其中图(b), (c), (d)均为介质层折射率$ {n}_{{\mathrm{d}}}=1 $的结果
Figure 10. Simulation results in the case of h = 25 μm: (a) Variation curve of refractive index sensitivity with porosity P of porous PMMA; (b) variation curve of average electric field mode of dielectric layer with porosity P of porous PMMA; (c) schematic diagram of field distribution under different porosity; (d) the electric field intensity curve at the interface between the dielectric layer and the porous PMMA layer under different porosity. Note: panels (b), (c), (d) are the results under the condition that the refractive index of the dielectric layer $ {n}_{{\mathrm{d}}}=1 $.
图 11 在孔隙率P = 0.4情况下的仿真结果 (a) 折射率灵敏度随多孔PMMA厚度h的变化; (b) 介质层平均电场模随多孔PMMA厚度h的变化; (c) 不同厚度下的场分布示意图; (d) 不同厚度下介质层与多孔PMMA层界面处的电场强度曲线; 其中图(b), (c), (d)均为介质层折射率$ {n}_{{\mathrm{d}}}=1 $的结果
Figure 11. Simulation results in the case of P = 0.4: (a) Variation curve of refractive index sensitivity with porous PMMA thickness h; (b) variation curve of average electric field mode of dielectric layer with thickness h of porous PMMA; (c) schematic diagram of field distribution under different thickness; (d) the electric field intensity curves at the interface between the dielectric layer and the porous PMMA layer under different thickness. Note: panels (b), (c), (d) are the results of the refractive index of the dielectric layer $ {n}_{{\mathrm{d}}}=1 $.
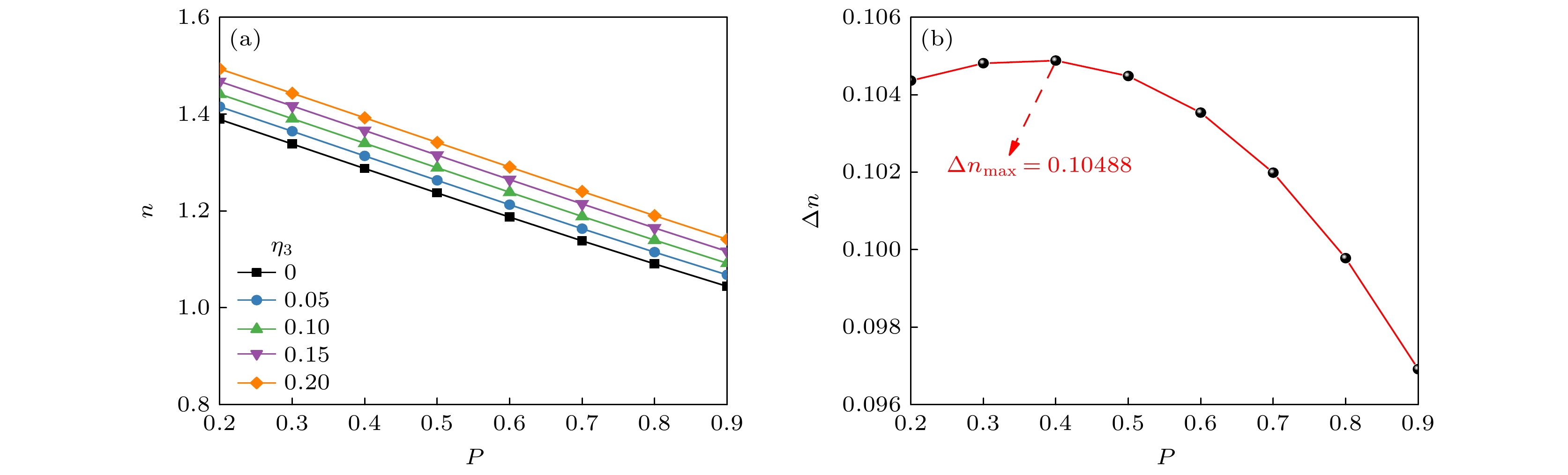
图 12 (a) 不同孔隙率下多孔PMMA吸附酪氨酸体积分数$ {\eta }_{3}= $0, 0.05, 0.10, 0.15, 0.20时的等效折射率n; (b) $ {\eta }_{3} $由0变为0.2时, 不同孔隙率多孔PMMA的等效折射率变化量$ \Delta n $
Figure 12. (a) Equivalent refractive index n when the volume fraction of tyrosine adsorbed by porous PMMA $ {\eta }_{3} $ is 0, 0.05, 0.10, 0.15 and 0.20 under different porosity; (b) the change of equivalent refractive index $ \Delta n $ of porous PMMA with different porosity when $ {\eta }_{3} $ changing from 0 to 0.2.
图 13 在孔隙率P = 0.4条件下的仿真结果 (a) 覆盖厚度为40 μm的多孔PMMA, 传感器吸附不同体积分数酪氨酸的反射光谱; (b) 覆盖厚度为40 μm的多孔PMMA, 传感器吸附不同体积分数酪氨酸的共振频率变化曲线, 及线性拟合得到的吸附灵敏度; (c) 覆盖厚度为120 μm的多孔PMMA, 传感器吸附不同体积分数酪氨酸的反射光谱; (d) 覆盖20—240 μm厚度的多孔PMMA, 传感器吸附灵敏度变化曲线
Figure 13. Simulation results in the case of porosity P = 0.4: (a) The reflection spectra of the sensor after adsorption of tyrosine with different volume fractions when the thickness of the covered porous PMMA is 40 μm; (b) the resonance frequency curve of the sensor after adsorption of tyrosine with different volume fractions and the adsorption sensitivity obtained by linear fitting when the thickness of the covered porous PMMA is 40 μm; (c) the reflection spectra of the sensor after adsorption of tyrosine with different volume fractions when the thickness of the covered porous PMMA is 40 μm; (d) variation curves of sensor adsorption sensitivity when the thickness of covered porous PMMA is 20–240 μm.
-
[1] 张喆, 柳倩, 祁志美 2013 62 060703
Google Scholar
Zhang Z, Liu Q, Qi Z M 2013 Acta Phys. Sin. 62 060703
Google Scholar
[2] Zhang C L, Liu Z W, Cai C, Yang Z H, Qi Z M 2022 Opt. Lett. 47 4155
Google Scholar
[3] Anker J N, Hall W P, Lyandres O, Shah N C, Zhao J, Van Duyne R P 2008 Nat. Mater. 7 442
Google Scholar
[4] 刘濮鲲, 黄铁军 2020 红外与毫米波学报 39 169
Google Scholar
Liu P K, Huang T J 2020 J. Infrared Millim. Waves 39 169
Google Scholar
[5] Pendry J B, Martín-Moreno L, Garcia-Vidal F J 2004 Science 305 847
Google Scholar
[6] Hibbins A P, Evans B R, Sambles J R 2005 Science 308 670
Google Scholar
[7] Williams C R, Andrews S R, Maier S A, Fernández-Domínguez A I, Martín-Moreno L, García-Vidal F J 2008 Nat. Photonics 2 175
Google Scholar
[8] Ng B H, Wu J F, Hanham S M, Fernández-Domínguez A I, Klein N, Liew Y F, Breese M B H, Hong M H, Maier S A 2013 Adv. Opt. Mater. 1 543
Google Scholar
[9] Ng B H, Hanham S M, Wu J F, Fernández-Domínguez A I, Klein N, Liew Y F, Breese M B H, Hong M H, Maier S A 2014 ACS Photonics 1 1059
Google Scholar
[10] Shen X P, Cui T J 2014 Laser Photonics Rev. 8 137
Google Scholar
[11] Chochol J, Postava K, Čada M, Vanwolleghem M, Halagačka L, Lampin J F, Pištora J 2016 AIP Adv. 6 115021
Google Scholar
[12] Marschall N, Fischer B, Queisser H J 1971 Phy. Rev. Lett. 27 95
Google Scholar
[13] Cheng B H, Ye Y S, Lan Y C, Tsai D P 2017 Sci. Rep. 7 6443
Google Scholar
[14] Chochol J, Postava K, Cada M, Pistora J 2017 Sci. Rep. 7 13117
Google Scholar
[15] Isaac T H, Barnes W L, Hendry E 2008 Appl. Phys. Lett. 93 241115
Google Scholar
[16] Madelung O 1991 Semiconductors: Group IV Elements and III-V Compounds (Berlin, Heidelberg: Springer) p147
[17] Shibayama J, Shimizu K, Yamauchi J, Nakano H 2016 J. Lightwave Technol. 34 2518
Google Scholar
[18] Tao H, Chieffo L R, Brenckle M A, Siebert S M, Liu M K, Strikwerda A C, Fan K B, Kaplan D L, Zhang X, Averitt R D, Omenetto F C 2011 Adv. Mater. 23 3197
Google Scholar
[19] Wang Y, Cui Z J, Zhu D Y, Wang X M, Chen S G, Nie P C 2019 Opt. Express 27 14133
Google Scholar
[20] Nie P C, Zhu D Y, Cui Z J, Qu F F, Lin L, Wang Y 2020 Sens. Actuator B-Chem. 307 127642
Google Scholar
[21] 向星诚, 马海贝, 王磊, 田达, 张伟, 张彩虹, 吴敬波, 范克斌, 金飚兵, 陈健, 吴培亨 2023 72 128701
Google Scholar
Xiang X, Ma H, Wang L, Tian D, Zhang W, Zhang C, Wu J, Fan K, Jin B, Chen J, Wu P 2023 Acta Phys. Sin. 72 128701
Google Scholar
[22] 岳伟伟, 王卫宁, 赵国忠, 张存林, 闫海涛 2005 54 3094
Google Scholar
Yue W W, Wang W N, Zhao G Z, Zhang C L, Yan H T 2005 Acta Phys. Sin. 54 3094
Google Scholar
[23] 王国阳, 白志晨, 王佳慧, 苏波, 张存林 2021 光谱学与光谱分析 41 1678
Google Scholar
Wang G Y, Bai Z C, Wang J H, Su B, Zhang C L 2021 Spectrosc. Spectral Anal. 41 1678
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1935
- PDF Downloads: 50
- Cited By: 0
