Coherent coupling in a driven qubit-magnon hybrid quantum system

Xu Da; Wang Yi-Pu; Li Tie-Fu; You Jian-Qiang

doi:10.7498/aps.71.20220260

Abstract

We experimentally demonstrate the strong coupling between the ferromagnetic magnons in an yttrium-iron-garnet (YIG) sphere and the drive-field-induced dressed states of a superconducting qubit, which gives rise to the double dressing of the superconducting qubit. The YIG sphere and the superconducting qubit are embedded in a microwave cavity, and are coupled to the magnetic and electrical fields of the cavity $\mathrm{TE}_{102}$ mode, respectively. The effective coupling between them is mediated by the virtual cavity photons of cavity $\mathrm{TE}_{102}$ mode. Our experimental results indicate that as the power for driving the qubit increases, an additional split of the qubit-magnon polariton occurs. These supplemental splittings indicate a double-dressed state. We theoretically analyze the experimental results by using a particle-hole symmetric model. The theoretical results fit the experimental observations well in a broad range of drive-field power parameters, revealing that the driven qubit-magnon hybrid quantum system can be used to emulate a particle-hole symmetric pair coupled to a bosonic mode. Our hybrid quantum system holds great promise for quantum simulations of composite quasiparticles consisting of fermions and bosons.

Keywords:

Authors and contacts

1.
Department of Physics, Zhejiang University, Hangzhou 310058, China
2.
School of Integrated Circuits, Tsinghua University, Beijing 100084, China

Corresponding author: Wang Yi-Pu, yipuwang@zju.edu.cn

Funds: Project supported by the China Postdoctoral Science Foundation (Grant No. 2019M660137) and the National Natural Science Foundation of China (Grant Nos. 11934010, 12174329)

FullText HTML : translate this paragraph

References

[1]	Xiang Z L, Ashhab S, You J Q, Nori F 2013 Rev. Mod. Phys. 85 623 Google Scholar
[2]	Kurizki G, Bertet P, Kubo Y, Mølmer K, Petrosyan D, Rabl P, Schmiedmayer J 2015 Proc. Natl. Acad. Sci. U.S.A. 112 3866 Google Scholar
[3]	Wallquist M, Hammerer K, Rabl P, Lukin M, Zoller P 2009 Phys. Scr. T137 014001 Google Scholar
[4]	Kimble H J 2008 Nature 453 1023 Google Scholar
[5]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405 Google Scholar
[6]	Lachance-Quirion D, Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Nakamura Y 2017 Sci. Adv. 3 e1603150 Google Scholar
[7]	Lachance-Quirion D, Wolski S P, Tabuchi Y, Kono S, Usami K, Nakamura Y 2020 Science 367 425 Google Scholar
[8]	White R M 2007 Quantum Theory of Magnetism: Magnetic Properties of Materials (3rd Ed.) (Berlin: Springer) pp5–7
[9]	Soykal Ö O, Flatté M E 2010 Phys. Rev. Lett. 104 077202 Google Scholar
[10]	Soykal Ö O, Flatté M E 2010 Phys. Rev. B 82 104413 Google Scholar
[11]	Huebl H, Zollitsch C W, Lotze J, Hocke F, Greifenstein M, Marx A, Gross R, Goennenwein S T B 2013 Phys. Rev. Lett. 111 127003 Google Scholar
[12]	Tabuchi Y, Ishino S, Ishikawa Y, Yamazaki R, Usami K, Nakamura Y 2014 Phys. Rev. Lett. 113 083603 Google Scholar
[13]	Zhang X, Zou C L, Jiang L, Tang H X 2014 Phys. Rev. Lett. 113 156401 Google Scholar
[14]	Goryachev M, Farr W G, Creedon D L, Fan Y, Kostylev M, Tobar M E 2014 Phys. Rev. Appl. 2 054002 Google Scholar
[15]	Haigh J A, Langenfeld S, Lambert N J, Baumberg J J, Ramsay A J, Nunnenkamp A, Ferguson A J 2015 Phys. Rev. A 92 063845 Google Scholar
[16]	Zhang X, Zou C L, Jiang L, Tang H X 2016 Sci. Adv. 2 e1501286 Google Scholar
[17]	Bai L, Harder M, Chen Y P, Fan X, Xiao J Q, Hu C M 2015 Phys. Rev. Lett. 114 227201 Google Scholar
[18]	Zhang D, Wang X M, Li T F, Luo X Q, Wu W, Nori F, You J Q 2015 npj Quantum Inf. 1 15014 Google Scholar
[19]	Rameshti B Z, Cao Y, Bauer G E W 2015 Phys. Rev. B 91 214430 Google Scholar
[20]	Cao Y P, Huebl H, Goennenwein S T B, Bauer G E W 2015 Phys. Rev. B 91 094423 Google Scholar
[21]	Haigh J A, Lambert N J, Doherty A C, Ferguson A J 2015 Phys. Rev. B 91 104410 Google Scholar
[22]	Liu T, Zhang X, Tang H X, Flatté M E 2016 Phys. Rev. B 94 060405 Google Scholar
[23]	Wang Y P, Zhang G Q, Zhang D, Luo X Q, Xiong W, Wang S P, Li T F, Hu C M, You J Q 2016 Phys. Rev. B 94 224410 Google Scholar
[24]	Sharma S, Blanter Y M, Bauer G E W 2017 Phys. Rev. B 96 094412 Google Scholar
[25]	Sharma S, Blanter Y M, Bauer G E W 2018 Phys. Rev. Lett. 121 087205 Google Scholar
[26]	Osada A, Gloppe A, Hisatomi R, Noguchi A, Yamazaki R, Nomura M, Nakamura Y, Usami K 2018 Phys. Rev. Lett. 120 133602 Google Scholar
[27]	Liu Z X, Wang B, Xiong H, Wu Y 2018 Opt. Lett. 43 3698 Google Scholar
[28]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2016 C. R. Physique 17 729 Google Scholar
[29]	Quirion D L, Tabuchi Y, Gloppe A, Usami K, Nakamura Y 2019 Appl. Phys. Express 12 070101 Google Scholar
[30]	Wang Y P, Rao J W, Yang Y, Xu P C, Gui Y S, Yao B M, You J Q, Hu C M 2019 Phys. Rev. Lett. 123 127202 Google Scholar
[31]	Lambert N J, Haigh J A, Langenfeld S, Doherty A C, Ferguson A J 2016 Phys. Rev. A 93 021803(R Google Scholar
[32]	Zhang X, Zou C L, Zhu N, Marquardt F, Jiang L, Tang H X 2015 Nat. Commun. 6 8914 Google Scholar
[33]	Bai L, Harder M, Hyde P, Zhang Z, Hu C M, Chen Y P, Xiao J Q 2017 Phys. Rev. Lett. 118 217201 Google Scholar
[34]	Hisatomi R, Osada A, Tabuchi Y, Ishikawa T, Noguchi A, Yamazaki R, Usami K, Nakamura Y 2016 Phys. Rev. B 93 174427 Google Scholar
[35]	Osada A, Hisatomi R, Noguchi A, Tabuchi Y, Yamazaki R, Usami K, Sadgrove M, Yalla R, Nomura M, Nakamura Y 2016 Phys. Rev. Lett. 116 223601 Google Scholar
[36]	Zhang X, Zhu N, Zou C L, Tang H X 2016 Phys. Rev. Lett. 117 123605 Google Scholar
[37]	Haigh J A, Nunnenkamp A, Ramsay A J, Ferguson A J 2016 Phys. Rev. Lett. 117 133602 Google Scholar
[38]	Braggio C, Carugno G, Guarise M, Ortolan A, Ruoso G 2017 Phys. Rev. Lett. 118 107205 Google Scholar
[39]	Wu W J, Wang Y P, Wu J Z, Li J, You J Q 2021 Phys. Rev. A 104 023711 Google Scholar
[40]	Sun F X, Zheng S S, Xiao Y, Gong Q H, He Q Y, Xia K 2021 Phys. Rev. Lett. 127 087203 Google Scholar
[41]	Li J, Wang Y P, Wu W J, Zhu S Y, You J Q 2017 PRX Quantum 2 040344
[42]	Zhang D, Luo X Q, Wang Y P, Li T F, You J Q 2017 Nat. Commun. 8 1368 Google Scholar
[43]	Cao Y, Yan P 2019 Phys. Rev. B 99 214415 Google Scholar
[44]	Zhao J, Liu Y, Wu L, Duan C K, Liu Y X, Du J 2020 Phys. Rev. Appl. 13 014053 Google Scholar
[45]	Yang Y, Wang Y P, Rao J W, Gui Y S, Yao B M, Lu W, Hu C M 2020 Phys. Rev. Lett. 125 147202 Google Scholar
[46]	Wang Y P, Zhang G Q, Zhang D, Li T F, Hu C M, You J Q 2018 Phys. Rev. Lett. 120 057202 Google Scholar
[47]	Hyde P, Yao B M, Gui Y S, Zhang G Q, You J Q, Hu C M 2018 Phys. Rev. B 98 174423 Google Scholar
[48]	Bi M X, Yan X H, Zhang Y, Xiao Y 2021 Phys. Rev. B 103 104411 Google Scholar
[49]	Shen R C, Wang Y P, Li J, Zhu S Y, Agarwal G S, You J Q 2021 Phys. Rev. Lett. 127 183202 Google Scholar
[50]	Li J, Zhu S Y, Agarwal G S 2018 Phys. Rev. Lett. 121 203601 Google Scholar
[51]	Li J, Zhu S Y 2019 New J. Phys. 21 085001 Google Scholar
[52]	Zhang Z, Scully M, Agarwal G S 2019 Phys. Rev. Res. 1 023021 Google Scholar
[53]	Yuan H Y, Zhang S, Ficek Z, He Q Y, Yung M H 2020 Phys. Rev. B 101 014419 Google Scholar
[54]	Yuan H Y, Yan P, Zheng S, He Q Y, Xia K, Yung M H 2020 Phys. Rev. Lett. 124 053602 Google Scholar
[55]	Liu Z X, Xiong H, Wu Y 2019 Phys. Rev. B 100 134421 Google Scholar
[56]	Li X, Wang X, Yang W X 2021 Phys. Rev. B 104 224434 Google Scholar
[57]	Bourhill J, Kostylev N, Goryachev M, Creedon D L, Tobar M E 2016 Phys. Rev. B 93 144420 Google Scholar
[58]	Kostylev N, Goryachev M, Tobar M E 2016 Appl. Phys. Lett. 108 062402 Google Scholar
[59]	Harder M, Yang Y, Yao B M, Yu C H, Rao J W, Gui Y S, Stamps R L, Hu C M 2018 Phys. Rev. Lett. 121 137203 Google Scholar
[60]	Grigoryan V L, Shen K, Xia K 2018 Phys. Rev. B 98 024406 Google Scholar
[61]	Bhoi B, Kim B, Jang S H, Kim J, Yang J, Cho Y J, Kim S K 2019 Phys. Rev. B 99 134426 Google Scholar
[62]	Yu W, Wang J, Yuan H Y, Xiao J 2019 Phys. Rev. Lett. 123 227201 Google Scholar
[63]	Grigoryan V L, Xia K 2019 Phys. Rev. B 100 014415 Google Scholar
[64]	Wang Y P, Hu C M 2020 J. Appl. Phys. 127 130901 Google Scholar
[65]	Laucht A, Kalra R, Simmons S, Dehollain J P, Muhonen J T, Mohiyaddin F A, Freer S, Hudson F E, Itoh K M, Jamieson D N, McCallum J C, Dzurak A S, Morello A 2017 Nat. Nanotechnol. 12 61 Google Scholar
[66]	Timoney N, Baumgart I, Johanning M, Varón A F, Plenio M B, Retzker A, Wunderlich Ch 2011 Nature 476 185 Google Scholar
[67]	Oelsner G, Macha P, Astafiev O V, Il’ichev E, Grajcar E, Hübner U, Ivanov B I, Neilinger P, Meyer H G 2013 Phys. Rev. Lett. 110 053602 Google Scholar
[68]	Xue Z Y, Gu F L, Hong Z P, Yang Z H, Zhang D W, Hu Y, You J Q 2017 Phys. Rev. Appl. 7 054022 Google Scholar
[69]	Koshino K, Lin Z, Inomata K, Yamamoto T, Nakamura Y 2016 Phys. Rev. A 93 023824 Google Scholar
[70]	Scully M O, Zubairy M S 1997 Quantum Optics (Cambrudge: Cambridge University Press) pp193–219
[71]	Paik H, Schuster D I, Bishop L S, Kirchmair G, Catelani G, Sears A P, Johnson B R, Reagor M J, Frunzio L, Glazman L I, Devoret M H, Schoelkopf R J 2011 Phys. Rev. Lett. 107 240501 Google Scholar
[72]	Jin X Y, Kamal A, Sears A P, Gudmundsen T, Hover D, Miloshi J, Slattery R, Yan F, Yoder J, Orlando T P, Gustavsson S, Oliver W D 2015 Phys. Rev. Lett. 114 240501 Google Scholar
[73]	You J Q, Hu X, Ashhab S, Nori F 2007 Phys. Rev. B 75 140515(R Google Scholar
[74]	Yan F, Gustavsson S, Kamal A, Birenbaum J, Sears A P, Hover D, Gudmundsen T J, Rosenberg D, Samach G, Weber S, Yoder J L, Orlando T P, Clarke J, Kerman A J, Oliver W D 2016 Nat. Commun. 7 12964 Google Scholar
[75]	Koch J, Yu T M, Gambetta J, Houck A A, Schuster D I, Majer J, Blais A, Devoret M H, Girvin S M, Schoelkopf R J 2007 Phys. Rev. A 76 042319 Google Scholar
[76]	Barends R, Kelly J, Megrant A, Veitia A, Sank D, Jeffrey E, White T C, Mutus J, Fowler A G, Campbell B, Chen Y, Chen Z, Chiaro B, Dunsworth A, Neill C, OMalley P, Roushan P, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2014 Nature 508 500 Google Scholar
[77]	Shevchenko S N, Oelsner G, Greenberg Ya S, Macha P, Karpov D S, Grajcar M, Hübner U, Omelyanchouk A N, Il’ichev E 2014 Phys. Rev. B 89 184504 Google Scholar
[78]	Mahan G D 2000 Many-Particle Physics (3rd Ed.) (New York: Kluwer Academic/Plenum) pp411–413

Cited By

图 1 量子比特与磁振子耦合系统示意图　(a) 超导量子比特与磁振子耦合系统示意图, 其中量子比特与YIG小球同时放置在三维谐振腔中. 谐振腔由无氧铜腔和铝腔两部分组成, YIG小球放置在谐振腔无氧铜材质的半腔内使得磁场可以穿透, 量子比特放置在铝的半腔内以得到更好的磁屏蔽效果. YIG小球放置在谐振腔TE₁₀₂模式磁场的波腹位置以获得两者之间更大的耦合强度, 同时量子比特放置在TE₁₀₂模式电场的波腹. (b) 腔TE₁₀₂和TE₁₀₃模式电磁场分布的示意图. 上半图为腔TE₁₀₂模式的电场分布, 下半图左侧为腔TE₁₀₂模式的磁场分布, 右侧为腔TE₁₀₃模式的电场分布. 可以看到, YIG小球和超导量子比特芯片分别放置在腔TE₁₀₂模式磁场最大值和TE₁₀₂模式电场较大值位置, 考虑到需要通过TE₁₀₃模式读取比特, 并没有把芯片放置在TE₁₀₂模式电场最大处. (c) 谐振腔TE₁₀₂的透射谱. 改变外加静磁场强度来改变YIG小球中基泰尔模磁振子的共振频率, 图中展示的是磁振子与腔TE₁₀₂ 模式近共振附近的谱线, 可以看到两者相干耦合产生的反交叉劈裂

Figure 1. Schematic of qubit-magnon hybrid system in a rectranglar 3D microwave cavity: (a) A small YIG sphere is placed in cavity made of oxygen-free copper at the magnetic-field antinode of the cavity mode TE₁₀₂. The qubit is mounted in the part of the cavity made of aluminium near the antinode of cavity TE₁₀₂ mode. (b) Electrical and magnetic field distribution of cavity TE₁₀₂ and TE₁₀₃ mode. The upper half figure shows the electric field distribution of cavity TE₁₀₂ mode, the left of lower half shows the magnetic field distribution of cavity TE₁₀₂ mode, TE₁₀₃ mode electric field distribution is shown on the right-hand side. As shown in figure, the YIG sphere and the qubit chip are placed near the antinodes of cavity TE₁₀₃ magnetic field and TE₁₀₂ electric field, respectively. (c) Transmission spectrum of the cavity when the Kittel mode of magnons in the YIG sphere is magnetically tuned to be near resonance with the cavity TE₁₀₂ mode

DownLoad: Full-Size Img PowerPoint

图 2 量子比特与磁振子的耦合　(a) 量子比特与磁振子耦合系统的能级结构, 图中只考虑了基泰尔模磁振子的单量子态和基态. 调节磁振子的谐振频率使得磁振子的能级差与比特能级差对上, 能级$ |g, 1\rangle $与$ |e, 0\rangle $简并并出现劈裂, 耦合强度即真空拉比劈裂 2g_qm, 进一步给比特施加微波驱动Ω_d, 劈裂的两个能级进一步劈裂成四个能级. (b) 量子比特-磁振子相干耦合系统的真空拉比劈裂. 实验上改变磁振子的外加磁场把两者频率调到近共振, 对超导量子比特加驱动微波, 同时用谐振腔TE₁₀₃模式读取腔的传输谱来反映比特的变化

Figure 2. Coherent coupling between qubit and the magnon: (a) Energy levels of qubit-magnon system with only the vacuum and single-magnon states involved for the Kittle mode. If we adjusting the magnetic field to set magnon Kittle mode frequency resonance with the qubit, $ |g, 1\rangle $ and $ |e, 0\rangle $degenerates, the coupling between $ |g, 1\rangle $and $ |e, 0\rangle $ induces the vacuum Rabi splitting 2g_qm. If we apply a microwave drive with amplitude Ω_d to qubit, the degenerated levels further split into 4 levels. (b) Vacuum Rabi splitting of the qubit-magnon system measured via the transmission spectrum of the cavity by both tuning the static magnetic field and scanning the frequency of the excitation field. The porbe field is applied in resonance with the cavity TE₁₀₃ mode

DownLoad: Full-Size Img PowerPoint

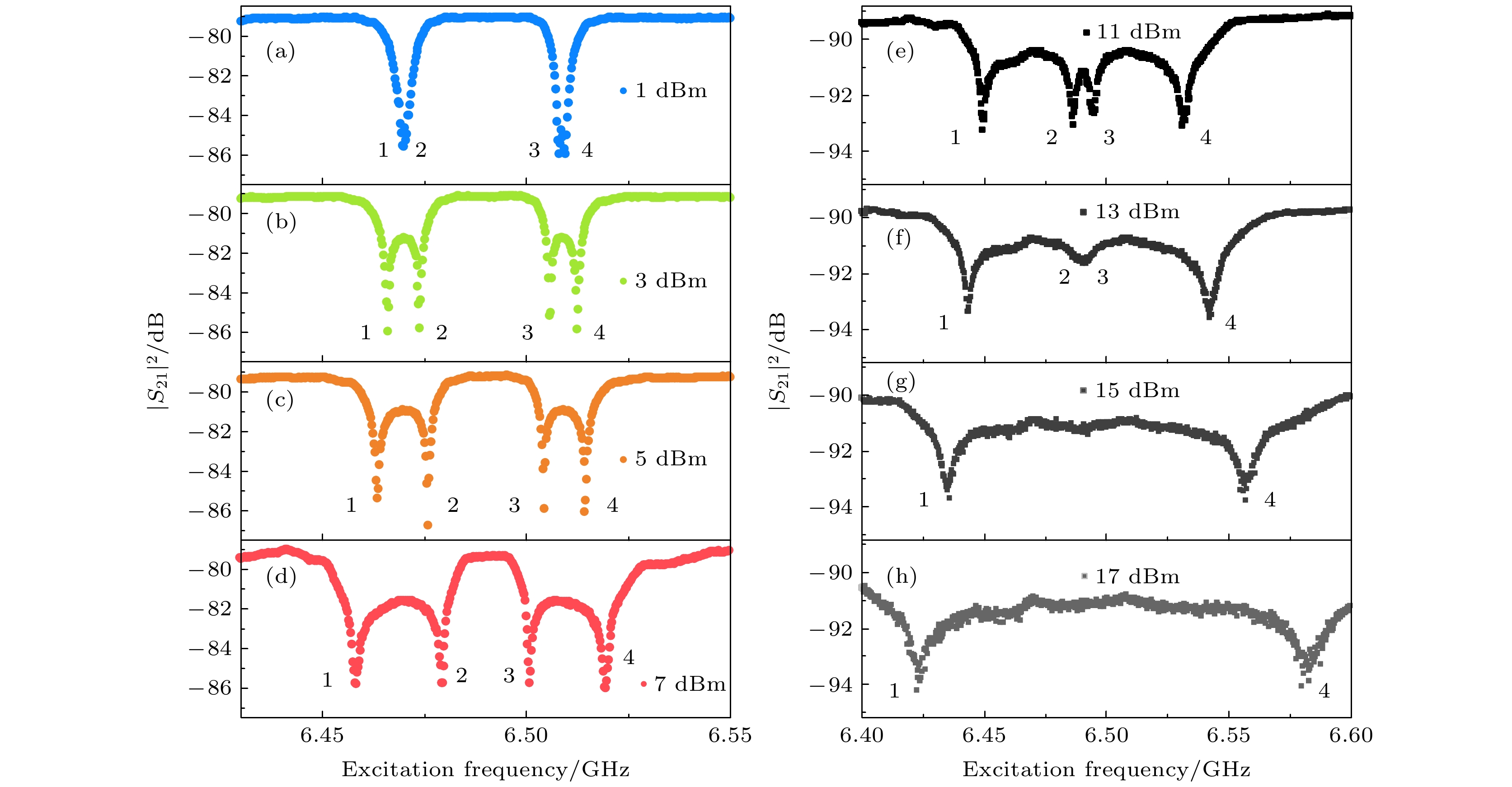
图 3 量子比特-磁振子耦合系统能级劈裂随外加微波场驱动强度的变化, 其中$ \delta_{\rm{q}}=\delta_{\rm{m}}=0 $. 使用驱动微波去激发比特, 同时用一个与读取腔${\rm{TE}}_{103}$共振的微波进行色散读取. 驱动微波的功率分别是(a) $0.04\;{\text{μW}}$, (b) $0.06\;{\text{μW}}$, (c) $0.1\;{\text{μW}}$, (d) $0.16\;{\text{μW}}$, (e) $0.4\;{\text{μW}}$, (f) $0.63\;{\text{μW}}$, (g) $1\;{\text{μW}}$, (h) $1.6\;{\text{μW}}$

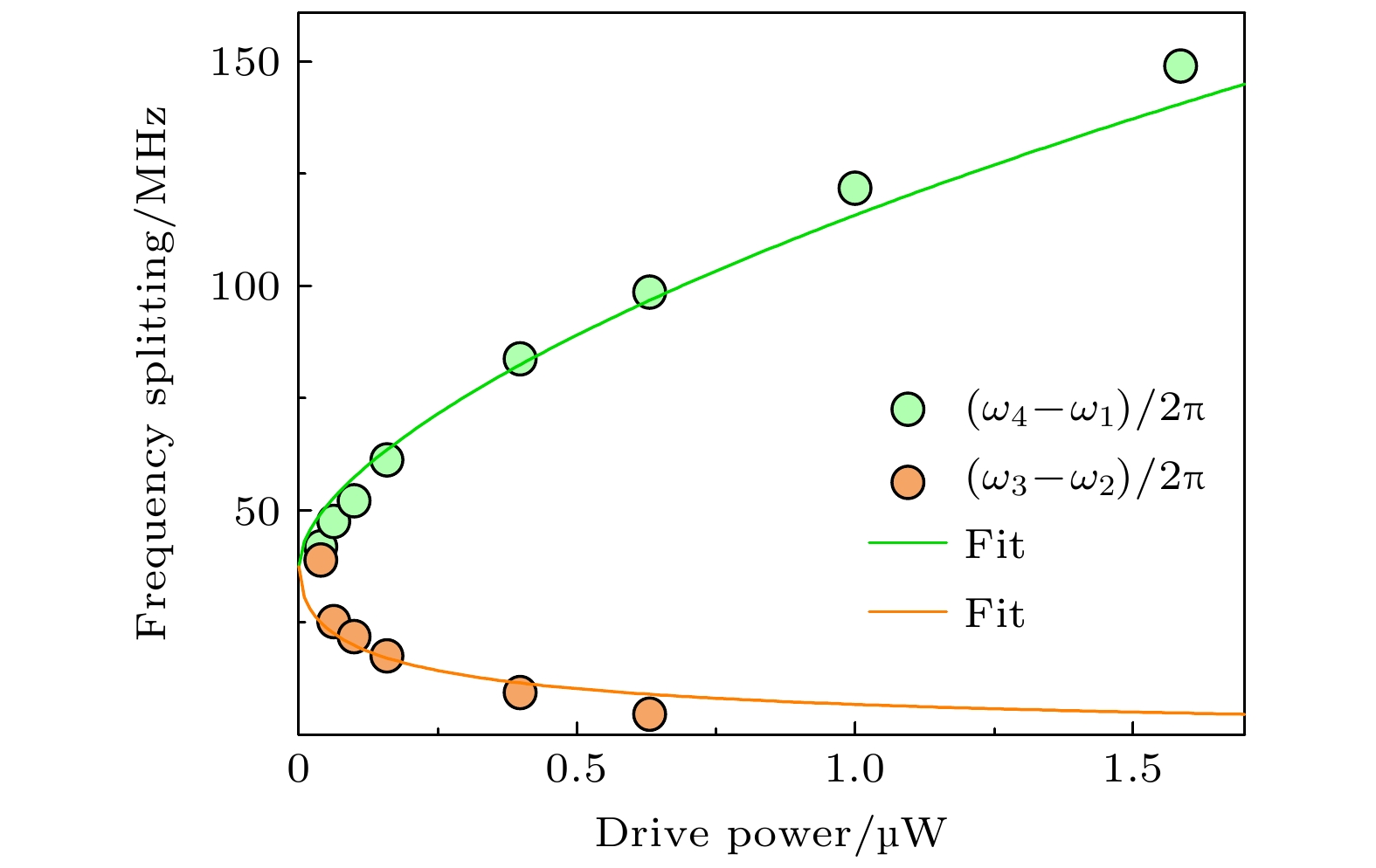
Figure 3. Dispersive readout of the hybridized normal modes of the driven qubit-magnon system. An excitation field is tuned to excite the hybridized normal modes and a probe field is applied in resonance with the cavity mode ${\rm{TE}}_{103}$. The power of the microwave field to drive the superconducting qubit is tuned to be (a) $0.04\;{\text{μW}}$, (b) $0.06\;{\text{μW}}$, (c) $0.1\;{\text{μW}}$, (d) $0.16\;{\text{μW}}$, (e) $0.4\;{\text{μW}}$, (f) $0.63\;{\text{μW}}$, (g) $1\;{\text{μW}}$, (h) $1.6\;{\text{μW}}$, respectively

DownLoad: Full-Size Img PowerPoint

图 4 量子比特-磁振子耦合系统频率劈裂随外加微波场驱动强度变化的拟合. 上半图数据(绿色圆点和绿线)是耦合模式1和模式4 (见正文)之间能级差随驱动功率$ P_{\rm{d }}$的变化. 下半图数据(橙色圆点和橙线)是耦合模式3和模式2能级差随驱动功率$ P_{\rm{d}} $的变化. 使用(5)式进行拟合, 其中$ |\tilde{g}_{{\rm{qm}}}|=|g_{{\rm{qm}}}| $, $ n_{\rm{m}}=0 $, 以及$\varOmega_{\rm{d}}=k\sqrt{P_{\rm{d}}}$, 这里$k= $$103{\text{ MHz/}{\text{μW}}^{1/2}}$

Figure 4. Fitting the experimental data of the frequency splitting between hybridized normal modes 1 and 4 (3 and 2) versus the drive power $ P_{\rm{d}} $. To fit the data, we use Eq. (5), where $ |\tilde{g}_{{\rm{qm}}}|=|g_{{\rm{qm}}}| $, $ n_{\rm{m}}=0 $, and $\varOmega_{\rm{d}}=k\sqrt{P_{\rm{d}}}$ with $k=103{\text{ MHz/}{\text{μW}}^{1/2}}$

DownLoad: Full-Size Img PowerPoint

map

[1]	Xiang Z L, Ashhab S, You J Q, Nori F 2013 Rev. Mod. Phys. 85 623 Google Scholar
[2]	Kurizki G, Bertet P, Kubo Y, Mølmer K, Petrosyan D, Rabl P, Schmiedmayer J 2015 Proc. Natl. Acad. Sci. U.S.A. 112 3866 Google Scholar
[3]	Wallquist M, Hammerer K, Rabl P, Lukin M, Zoller P 2009 Phys. Scr. T137 014001 Google Scholar
[4]	Kimble H J 2008 Nature 453 1023 Google Scholar
[5]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2015 Science 349 405 Google Scholar
[6]	Lachance-Quirion D, Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Nakamura Y 2017 Sci. Adv. 3 e1603150 Google Scholar
[7]	Lachance-Quirion D, Wolski S P, Tabuchi Y, Kono S, Usami K, Nakamura Y 2020 Science 367 425 Google Scholar
[8]	White R M 2007 Quantum Theory of Magnetism: Magnetic Properties of Materials (3rd Ed.) (Berlin: Springer) pp5–7
[9]	Soykal Ö O, Flatté M E 2010 Phys. Rev. Lett. 104 077202 Google Scholar
[10]	Soykal Ö O, Flatté M E 2010 Phys. Rev. B 82 104413 Google Scholar
[11]	Huebl H, Zollitsch C W, Lotze J, Hocke F, Greifenstein M, Marx A, Gross R, Goennenwein S T B 2013 Phys. Rev. Lett. 111 127003 Google Scholar
[12]	Tabuchi Y, Ishino S, Ishikawa Y, Yamazaki R, Usami K, Nakamura Y 2014 Phys. Rev. Lett. 113 083603 Google Scholar
[13]	Zhang X, Zou C L, Jiang L, Tang H X 2014 Phys. Rev. Lett. 113 156401 Google Scholar
[14]	Goryachev M, Farr W G, Creedon D L, Fan Y, Kostylev M, Tobar M E 2014 Phys. Rev. Appl. 2 054002 Google Scholar
[15]	Haigh J A, Langenfeld S, Lambert N J, Baumberg J J, Ramsay A J, Nunnenkamp A, Ferguson A J 2015 Phys. Rev. A 92 063845 Google Scholar
[16]	Zhang X, Zou C L, Jiang L, Tang H X 2016 Sci. Adv. 2 e1501286 Google Scholar
[17]	Bai L, Harder M, Chen Y P, Fan X, Xiao J Q, Hu C M 2015 Phys. Rev. Lett. 114 227201 Google Scholar
[18]	Zhang D, Wang X M, Li T F, Luo X Q, Wu W, Nori F, You J Q 2015 npj Quantum Inf. 1 15014 Google Scholar
[19]	Rameshti B Z, Cao Y, Bauer G E W 2015 Phys. Rev. B 91 214430 Google Scholar
[20]	Cao Y P, Huebl H, Goennenwein S T B, Bauer G E W 2015 Phys. Rev. B 91 094423 Google Scholar
[21]	Haigh J A, Lambert N J, Doherty A C, Ferguson A J 2015 Phys. Rev. B 91 104410 Google Scholar
[22]	Liu T, Zhang X, Tang H X, Flatté M E 2016 Phys. Rev. B 94 060405 Google Scholar
[23]	Wang Y P, Zhang G Q, Zhang D, Luo X Q, Xiong W, Wang S P, Li T F, Hu C M, You J Q 2016 Phys. Rev. B 94 224410 Google Scholar
[24]	Sharma S, Blanter Y M, Bauer G E W 2017 Phys. Rev. B 96 094412 Google Scholar
[25]	Sharma S, Blanter Y M, Bauer G E W 2018 Phys. Rev. Lett. 121 087205 Google Scholar
[26]	Osada A, Gloppe A, Hisatomi R, Noguchi A, Yamazaki R, Nomura M, Nakamura Y, Usami K 2018 Phys. Rev. Lett. 120 133602 Google Scholar
[27]	Liu Z X, Wang B, Xiong H, Wu Y 2018 Opt. Lett. 43 3698 Google Scholar
[28]	Tabuchi Y, Ishino S, Noguchi A, Ishikawa T, Yamazaki R, Usami K, Nakamura Y 2016 C. R. Physique 17 729 Google Scholar
[29]	Quirion D L, Tabuchi Y, Gloppe A, Usami K, Nakamura Y 2019 Appl. Phys. Express 12 070101 Google Scholar
[30]	Wang Y P, Rao J W, Yang Y, Xu P C, Gui Y S, Yao B M, You J Q, Hu C M 2019 Phys. Rev. Lett. 123 127202 Google Scholar
[31]	Lambert N J, Haigh J A, Langenfeld S, Doherty A C, Ferguson A J 2016 Phys. Rev. A 93 021803(R Google Scholar
[32]	Zhang X, Zou C L, Zhu N, Marquardt F, Jiang L, Tang H X 2015 Nat. Commun. 6 8914 Google Scholar
[33]	Bai L, Harder M, Hyde P, Zhang Z, Hu C M, Chen Y P, Xiao J Q 2017 Phys. Rev. Lett. 118 217201 Google Scholar
[34]	Hisatomi R, Osada A, Tabuchi Y, Ishikawa T, Noguchi A, Yamazaki R, Usami K, Nakamura Y 2016 Phys. Rev. B 93 174427 Google Scholar
[35]	Osada A, Hisatomi R, Noguchi A, Tabuchi Y, Yamazaki R, Usami K, Sadgrove M, Yalla R, Nomura M, Nakamura Y 2016 Phys. Rev. Lett. 116 223601 Google Scholar
[36]	Zhang X, Zhu N, Zou C L, Tang H X 2016 Phys. Rev. Lett. 117 123605 Google Scholar
[37]	Haigh J A, Nunnenkamp A, Ramsay A J, Ferguson A J 2016 Phys. Rev. Lett. 117 133602 Google Scholar
[38]	Braggio C, Carugno G, Guarise M, Ortolan A, Ruoso G 2017 Phys. Rev. Lett. 118 107205 Google Scholar
[39]	Wu W J, Wang Y P, Wu J Z, Li J, You J Q 2021 Phys. Rev. A 104 023711 Google Scholar
[40]	Sun F X, Zheng S S, Xiao Y, Gong Q H, He Q Y, Xia K 2021 Phys. Rev. Lett. 127 087203 Google Scholar
[41]	Li J, Wang Y P, Wu W J, Zhu S Y, You J Q 2017 PRX Quantum 2 040344
[42]	Zhang D, Luo X Q, Wang Y P, Li T F, You J Q 2017 Nat. Commun. 8 1368 Google Scholar
[43]	Cao Y, Yan P 2019 Phys. Rev. B 99 214415 Google Scholar
[44]	Zhao J, Liu Y, Wu L, Duan C K, Liu Y X, Du J 2020 Phys. Rev. Appl. 13 014053 Google Scholar
[45]	Yang Y, Wang Y P, Rao J W, Gui Y S, Yao B M, Lu W, Hu C M 2020 Phys. Rev. Lett. 125 147202 Google Scholar
[46]	Wang Y P, Zhang G Q, Zhang D, Li T F, Hu C M, You J Q 2018 Phys. Rev. Lett. 120 057202 Google Scholar
[47]	Hyde P, Yao B M, Gui Y S, Zhang G Q, You J Q, Hu C M 2018 Phys. Rev. B 98 174423 Google Scholar
[48]	Bi M X, Yan X H, Zhang Y, Xiao Y 2021 Phys. Rev. B 103 104411 Google Scholar
[49]	Shen R C, Wang Y P, Li J, Zhu S Y, Agarwal G S, You J Q 2021 Phys. Rev. Lett. 127 183202 Google Scholar
[50]	Li J, Zhu S Y, Agarwal G S 2018 Phys. Rev. Lett. 121 203601 Google Scholar
[51]	Li J, Zhu S Y 2019 New J. Phys. 21 085001 Google Scholar
[52]	Zhang Z, Scully M, Agarwal G S 2019 Phys. Rev. Res. 1 023021 Google Scholar
[53]	Yuan H Y, Zhang S, Ficek Z, He Q Y, Yung M H 2020 Phys. Rev. B 101 014419 Google Scholar
[54]	Yuan H Y, Yan P, Zheng S, He Q Y, Xia K, Yung M H 2020 Phys. Rev. Lett. 124 053602 Google Scholar
[55]	Liu Z X, Xiong H, Wu Y 2019 Phys. Rev. B 100 134421 Google Scholar
[56]	Li X, Wang X, Yang W X 2021 Phys. Rev. B 104 224434 Google Scholar
[57]	Bourhill J, Kostylev N, Goryachev M, Creedon D L, Tobar M E 2016 Phys. Rev. B 93 144420 Google Scholar
[58]	Kostylev N, Goryachev M, Tobar M E 2016 Appl. Phys. Lett. 108 062402 Google Scholar
[59]	Harder M, Yang Y, Yao B M, Yu C H, Rao J W, Gui Y S, Stamps R L, Hu C M 2018 Phys. Rev. Lett. 121 137203 Google Scholar
[60]	Grigoryan V L, Shen K, Xia K 2018 Phys. Rev. B 98 024406 Google Scholar
[61]	Bhoi B, Kim B, Jang S H, Kim J, Yang J, Cho Y J, Kim S K 2019 Phys. Rev. B 99 134426 Google Scholar
[62]	Yu W, Wang J, Yuan H Y, Xiao J 2019 Phys. Rev. Lett. 123 227201 Google Scholar
[63]	Grigoryan V L, Xia K 2019 Phys. Rev. B 100 014415 Google Scholar
[64]	Wang Y P, Hu C M 2020 J. Appl. Phys. 127 130901 Google Scholar
[65]	Laucht A, Kalra R, Simmons S, Dehollain J P, Muhonen J T, Mohiyaddin F A, Freer S, Hudson F E, Itoh K M, Jamieson D N, McCallum J C, Dzurak A S, Morello A 2017 Nat. Nanotechnol. 12 61 Google Scholar
[66]	Timoney N, Baumgart I, Johanning M, Varón A F, Plenio M B, Retzker A, Wunderlich Ch 2011 Nature 476 185 Google Scholar
[67]	Oelsner G, Macha P, Astafiev O V, Il’ichev E, Grajcar E, Hübner U, Ivanov B I, Neilinger P, Meyer H G 2013 Phys. Rev. Lett. 110 053602 Google Scholar
[68]	Xue Z Y, Gu F L, Hong Z P, Yang Z H, Zhang D W, Hu Y, You J Q 2017 Phys. Rev. Appl. 7 054022 Google Scholar
[69]	Koshino K, Lin Z, Inomata K, Yamamoto T, Nakamura Y 2016 Phys. Rev. A 93 023824 Google Scholar
[70]	Scully M O, Zubairy M S 1997 Quantum Optics (Cambrudge: Cambridge University Press) pp193–219
[71]	Paik H, Schuster D I, Bishop L S, Kirchmair G, Catelani G, Sears A P, Johnson B R, Reagor M J, Frunzio L, Glazman L I, Devoret M H, Schoelkopf R J 2011 Phys. Rev. Lett. 107 240501 Google Scholar
[72]	Jin X Y, Kamal A, Sears A P, Gudmundsen T, Hover D, Miloshi J, Slattery R, Yan F, Yoder J, Orlando T P, Gustavsson S, Oliver W D 2015 Phys. Rev. Lett. 114 240501 Google Scholar
[73]	You J Q, Hu X, Ashhab S, Nori F 2007 Phys. Rev. B 75 140515(R Google Scholar
[74]	Yan F, Gustavsson S, Kamal A, Birenbaum J, Sears A P, Hover D, Gudmundsen T J, Rosenberg D, Samach G, Weber S, Yoder J L, Orlando T P, Clarke J, Kerman A J, Oliver W D 2016 Nat. Commun. 7 12964 Google Scholar
[75]	Koch J, Yu T M, Gambetta J, Houck A A, Schuster D I, Majer J, Blais A, Devoret M H, Girvin S M, Schoelkopf R J 2007 Phys. Rev. A 76 042319 Google Scholar
[76]	Barends R, Kelly J, Megrant A, Veitia A, Sank D, Jeffrey E, White T C, Mutus J, Fowler A G, Campbell B, Chen Y, Chen Z, Chiaro B, Dunsworth A, Neill C, OMalley P, Roushan P, Vainsencher A, Wenner J, Korotkov A N, Cleland A N, Martinis J M 2014 Nature 508 500 Google Scholar
[77]	Shevchenko S N, Oelsner G, Greenberg Ya S, Macha P, Karpov D S, Grajcar M, Hübner U, Omelyanchouk A N, Il’ichev E 2014 Phys. Rev. B 89 184504 Google Scholar
[78]	Mahan G D 2000 Many-Particle Physics (3rd Ed.) (New York: Kluwer Academic/Plenum) pp411–413

[1]	Yang Dong-Chao, Yi Li-Zhi, Ding Lin-Jie, Liu Min, Zhu Li-Ya, Xu Yun-Li, He Xiong, Shen Shun-Qing, Pan Li-Qing, John Q. Xiao. Nonequilibrium steady-state transport properties of magnons in ferromagnetic insulators. Acta Physica Sinica, 2024, 73(14): 147101. doi: 10.7498/aps.73.20240498
[2]	Lv Qing-Xian, Li Sai, Tu Hai-Tao, Liao Kai-Yu, Liang Zhen-Tao, Yan Hui, Zhu Shi-Liang. Research progress of superconductor and cold atoms hybrid quantum system. Acta Physica Sinica, 2023, 72(20): 200306. doi: 10.7498/aps.72.20230985
[3]	Cheng En-Hong, Lang Li-Jun. Electrical circuit simulation of nonreciprocal Aubry-André models. Acta Physica Sinica, 2022, 71(16): 160301. doi: 10.7498/aps.71.20220219
[4]	Li Zhu-Bai, Wei Lei, Zhang Zhen, Duan Dong-Wei, Zhao Qian. Macroeffect of magnons and thermal fluctiation on magnetization reversal. Acta Physica Sinica, 2022, 71(12): 127502. doi: 10.7498/aps.71.20220168
[5]	Gao Xue-Er, Li Dai-Li, Liu Zhi-Hang, Zheng Chao. Recent progress of quantum simulation of non-Hermitian systems. Acta Physica Sinica, 2022, 71(24): 240303. doi: 10.7498/aps.71.20221825
[6]	Wang Chen-Xu, He Ran, Li Rui-Rui, Chen Yan, Fang Ding, Cui Jin-Ming, Huang Yun-Feng, Li Chuan-Feng, Guo Guang-Can. Advances in the study of ion trap structures in quantum computation and simulation. Acta Physica Sinica, 2022, 71(13): 133701. doi: 10.7498/aps.71.20220224
[7]	Chen Yang, Zhang Tian-Yang, Guo Guang-Can, Ren Xi-Feng. Research progress of integrated photonic quantum simulation. Acta Physica Sinica, 2022, 71(24): 244207. doi: 10.7498/aps.71.20221938
[8]	Su Fei-Fan, Yang Zhao-Hua, Zhao Shou-Kuan, Yan Hai-Sheng, Tian Ye, Zhao Shi-Ping. Fabrication of superconducting qubits and auxiliary devices with niobium base layer. Acta Physica Sinica, 2022, 71(5): 050303. doi: 10.7498/aps.71.20211865
[9]	Luo Yu-Chen, Li Xiao-Peng. Quantum simulation of interacting fermions. Acta Physica Sinica, 2022, 71(22): 226701. doi: 10.7498/aps.71.20221756
[10]	Lin Jian, Ye Meng, Zhu Jia-Wei, Li Xiao-Peng. Machine learning assisted quantum adiabatic algorithm design. Acta Physica Sinica, 2021, 70(14): 140306. doi: 10.7498/aps.70.20210831
[11]	Zhao Xing-Dong, Zhang Ying-Ying, Liu Wu-Ming. Magnetic excitation of ultra-cold atoms trapped in optical lattice. Acta Physica Sinica, 2019, 68(4): 043703. doi: 10.7498/aps.68.20190153
[12]	Yu Wan-Rang, Ji Xin. Superadiabatic scheme for fast generating Greenberger-Horne-Zeilinger state of three superconducting qubits. Acta Physica Sinica, 2019, 68(3): 030302. doi: 10.7498/aps.68.20181922
[13]	Kong Xiang-Yu, Zhu Yuan-Ye, Wen Jing-Wei, Xin Tao, Li Ke-Ren, Long Gui-Lu. New research progress of nuclear magnetic resonance quantum information processing. Acta Physica Sinica, 2018, 67(22): 220301. doi: 10.7498/aps.67.20180754
[14]	Yu Xiang-Min, Tan Xin-Sheng, Yu Hai-Feng, Yu Yang. Topological quantum material simulated with superconducting quantum circuits. Acta Physica Sinica, 2018, 67(22): 220302. doi: 10.7498/aps.67.20181857
[15]	Fan Heng. Quantum computation and quantum simulation. Acta Physica Sinica, 2018, 67(12): 120301. doi: 10.7498/aps.67.20180710
[16]	Zhao Shi-Ping, Liu Yu-Xi, Zheng Dong-Ning. Novel superconducting qubits and quantum physics. Acta Physica Sinica, 2018, 67(22): 228501. doi: 10.7498/aps.67.20180845
[17]	Zhao Xu, Zhao Xing-Dong, Jing Hui. Simulating dnamical Casimir effect at finite temperature with magnons in spin chain within an optical lattice. Acta Physica Sinica, 2013, 62(6): 060302. doi: 10.7498/aps.62.060302
[18]	Zhao Na, Liu Jian-She, Li Tie-Fu, Chen Wei. Progress of coupled superconducting qubits. Acta Physica Sinica, 2013, 62(1): 010301. doi: 10.7498/aps.62.010301
[19]	Zhao Hu, Li Tie-Fu, Liu Jian-She, Chen Wei. Progress of electromagnetically induced transparency based on superconducting qubits. Acta Physica Sinica, 2012, 61(15): 154214. doi: 10.7498/aps.61.154214
[20]	Shi Qing-Fan, Li Liang-Sheng, Zhang Mei. Effectivity of Hamiltonian terms of "forbidden" 3-magnon interaction. Acta Physica Sinica, 2004, 53(11): 3916-3919. doi: 10.7498/aps.53.3916

Catalog

Vol. 71, Issue 15 - 2022-08-05

Metrics

Abstract views: 5535
PDF Downloads: 272
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板