-
玻色子体系中的非平衡输运过程研究是极具挑战性的工作. 磁振子是玻色子, 具有与电子等费米子截然不同的自旋输运行为. 本文以钇铁石榴石(YIG)铁磁绝缘体为研究对象, 聚焦影响稳态下YIG中磁振子非平衡输运过程的关键因素. 通过将具有非零化学势
$ {\mu }_{{\mathrm{m}}} $ 的玻色-爱因斯坦统计函数引入到玻尔兹曼输运方程中, 获得了以$ \alpha $ 为幂次的输运方程严格解析表达式(当α($ =-{\mu }_{{\mathrm{m}}}/\left({k}_{{\mathrm{B}}}T\right) $ )<1时). 结果显示, 当α$\ll1 $ 时, 我们得到了与以往研究不同的化学势$ {\mu }_{{\mathrm{m}}} $ 与非平衡粒子浓度$ \delta {n}_{{\mathrm{m}}} $ 之间的非线性关系$ \delta {n}_{{\mathrm{m}}}\propto -{\alpha }^{1/2}\propto -{(-{\mu }_{{\mathrm{m}}})}^{1/2} $ ;$ \alpha $ 较大时, 则还须考虑其高阶项. 正因这种非线性关系, 导致磁振子扩散方程显著不同于电子自旋扩散特性, 其由线性微分方程演变为更复杂的非线性微分方程. 本文重点研究了在两种极端温度梯度(即$ \nabla T \sim 1\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}} $ 和$ {10}^{4}\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}} $ )下非平衡磁振子浓度$ \delta {n}_{{\mathrm{m}}} $ 和化学势$ {\mu }_{{\mathrm{m}}} $ 的空间分布, 它们分别对应于$ {\mu }_{{\mathrm{m}}} $ 的值约为$ -0.1\;{\text{μ}}{\mathrm{e}}{\mathrm{V}} $ 和$ -6.2\;{\mathrm{m}}{\mathrm{e}}{\mathrm{V}} $ , 均满足前提条件α < 1. 在远离平衡态的大温度梯度分布下, 本文理论计算与实验结果吻合很好. 这些理论研究结果将加深人们对铁磁绝缘体中磁振子非平衡输运行为的认识.Understanding nonequilibrium transport phenomena in bosonic systems is highly challenging. Magnons, as bosons, exhibit different transport behavior from fermionic electron spins. This study focuses on the key factors influencing the nonequilibrium transport of magnons in steady states within magnetic insulators by taking Y3Fe5O12 (YIG) for example. By incorporating the Bose-Einstein distribution function with a non-zero chemical potential$ {\mu }_{m} $ into the Boltzmann transport equation, analytical expressions for transport parameters in power of$ \alpha $ ($ =-{\mu }_{{\mathrm{m}}}/({k}_{{\mathrm{B}}}T) $ ) are obtained under the condition α<1. It is the biggest different from previous researches that our theory establishes a nonlinear relationship between the chemical potential and the nonequilibrium particle density$ \delta {n}_{{\mathrm{m}}}\propto -{\alpha }^{1/2}\propto $ $ -{(-{\mu }_{{\mathrm{m}}})}^{1/2} $ for magnons under α$\ll 1 $ . For a large chemical potential, higher-order terms of α must be taken into account. Owing to this nonlinear relationship, the magnon diffusion equation markedly differs from that governing electron spin,which evolves into more complex nonlinear differential equation. We specifically focus on the ferrimagnetic insulator YIG by making a comparison of the spatial distribution of the nonequilibrium magnon density$ \delta {n}_{m} $ and chemical potential$ {\mu }_{m} $ between two extreme temperature gradients, namely,$ \nabla T \sim 1\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}} $ and$ {10}^{4}\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}}, $ which correspond to$ {\mu }_{{\mathrm{m}}} $ values on the order of$ -0.1\;{\text{μ}}{\mathrm{e}}{\mathrm{V}} $ and$ -6.2\;{\mathrm{m}}{\mathrm{e}}{\mathrm{V}} $ , respectively, while still satisfying the prerequisite α < 1. Given the known temperature gradient distribution, the nonequilibrium magnon density$ \delta {n}_{{\mathrm{m}}} $ calculated based on our theory is in good agreement with the experimental result. Our theoretical and numerical findings greatly contribute to a profound understanding of the nonequilibrium magnon transport characteristics in magnetic insulators.
-
Keywords:
- magnon /
- nonequilibrium transport /
- chemical potential /
- magnon diffusion equation /
- Boltzmann transport equation
[1] Bauer G E W, Saitoh E, van Wees B J 2012 Nat. Mater. 11 391
Google Scholar
[2] Chumak A V, Vasyuchka V I, Serga A A, Hillebrands B 2015 Nat. Phys. 11 453
Google Scholar
[3] Demokritov S O, Demidov V E, Dzyapko O, Melkov G A, Serga A A, Hillebrands B, Slavin A N 2006 Nature 443 430
Google Scholar
[4] Uchida K, Xiao J, Adachi H, et al. 2010 Nat. Mater. 9 894
Google Scholar
[5] Maehrlein S F, Radu I, Maldonado P, et al. 2018 Sci. Adv. 4 eaar5164
Google Scholar
[6] Shi Z, Xi Q, Li J X, Li Y F, Aldosary M, Xu Y D, Zhou J, Zhou S M, Shi J 2021 Phys. Rev. Lett. 127 277203
Google Scholar
[7] Uchida K I, Adachi H, Ota T, Nakayama H, Maekawa S, Saitoh E 2010 Appl. Phys. Lett. 97 172505
Google Scholar
[8] Uchida K, Takahashi S, Harii K, Ieda J, Koshibae W, Ando K, Maekawa S, Saitoh E 2008 Nature 455 778
Google Scholar
[9] Jaworski C M, Yang J, Mack S, Awschalom D D, Heremans J P, Myers R C 2010 Nat. Mater. 9 898
Google Scholar
[10] Jaworski C M, Myers R C, Johnston-Halperin E, Heremans J P 2012 Nature 487 210
Google Scholar
[11] Seki S, Ideue T, Kubota M, et al. 2015 Phys. Rev. Lett. 115 266601
Google Scholar
[12] Jiménez-Cavero P, Lucas I, Anadón A, et al. 2017 APL Mater. 5 026103
Google Scholar
[13] Li J, Shi Z, Ortiz V H, Aldosary M, Chen C, Aji V, Wei P, Shi J 2019 Phys. Rev. Lett. 122 217204
Google Scholar
[14] Ito N, Kikkawa T, Barker J, Hirobe D, Shiomi Y, Saitoh E 2019 Phys. Rev. B 100 60402
[15] Pirro P, Vasyuchka V I, Serga A A, Hillebrands B 2021 Nat. Rev. Mater. 6 1114
Google Scholar
[16] Chumak A V 2019 arXiv: 1901.08934.
[17] Cornelissen L J, Peters K J H, Bauer G E W, Duine R A, van Wees B J 2016 Phys. Rev. B 94 014412
Google Scholar
[18] Zhang S S L, Zhang S F 2012 Phys. Rev. Lett. 109 096603
Google Scholar
[19] Rezende S M, Rodríguez-Suárez R L, Cunha R O, et al. 2014 Phys. Rev. B 89 014416
Google Scholar
[20] Olsson K S, An K, Fiete G A, Zhou J S, Shi L, Li X Q 2020 Phys. Rev. X 10 021029
[21] Demidov V E, Urazhdin S, Divinskiy B, et al. 2017 Nat. Commun. 8 1579
Google Scholar
[22] Du C, Sar T van der, Zhou T X, Upadhyaya P, Casola F, Zhang H, Onbasli M C, Ross C A, Walsworth R L, Tserkovnyak Y, Yacoby A 2017 Science 357 195
Google Scholar
[23] Robinson J E 1951 Phys. Rev. 83 678
Google Scholar
[24] Yi L Z, Yang D C, Liu M, Fu H H, Ding L J, Xu Y L, Zhang B B, Pan L Q, Xiao J Q 2020 Adv. Funct. Mater. 30 2004024
Google Scholar
[25] Princep A J, Ewings R A, Ward S, et al. 2017 Npj Quantum Mater. 2 63
Google Scholar
[26] Son P C van, Kempen H van, Wyder P 1987 Phys. Rev. Lett. 58 2271
Google Scholar
[27] Valet T, Fert A 1993 Phys. Rev. B 48 7099
Google Scholar
[28] Kato Y K, Myers R C, Gossard A C, Awschalom D D 2004 Science 306 1910
Google Scholar
-
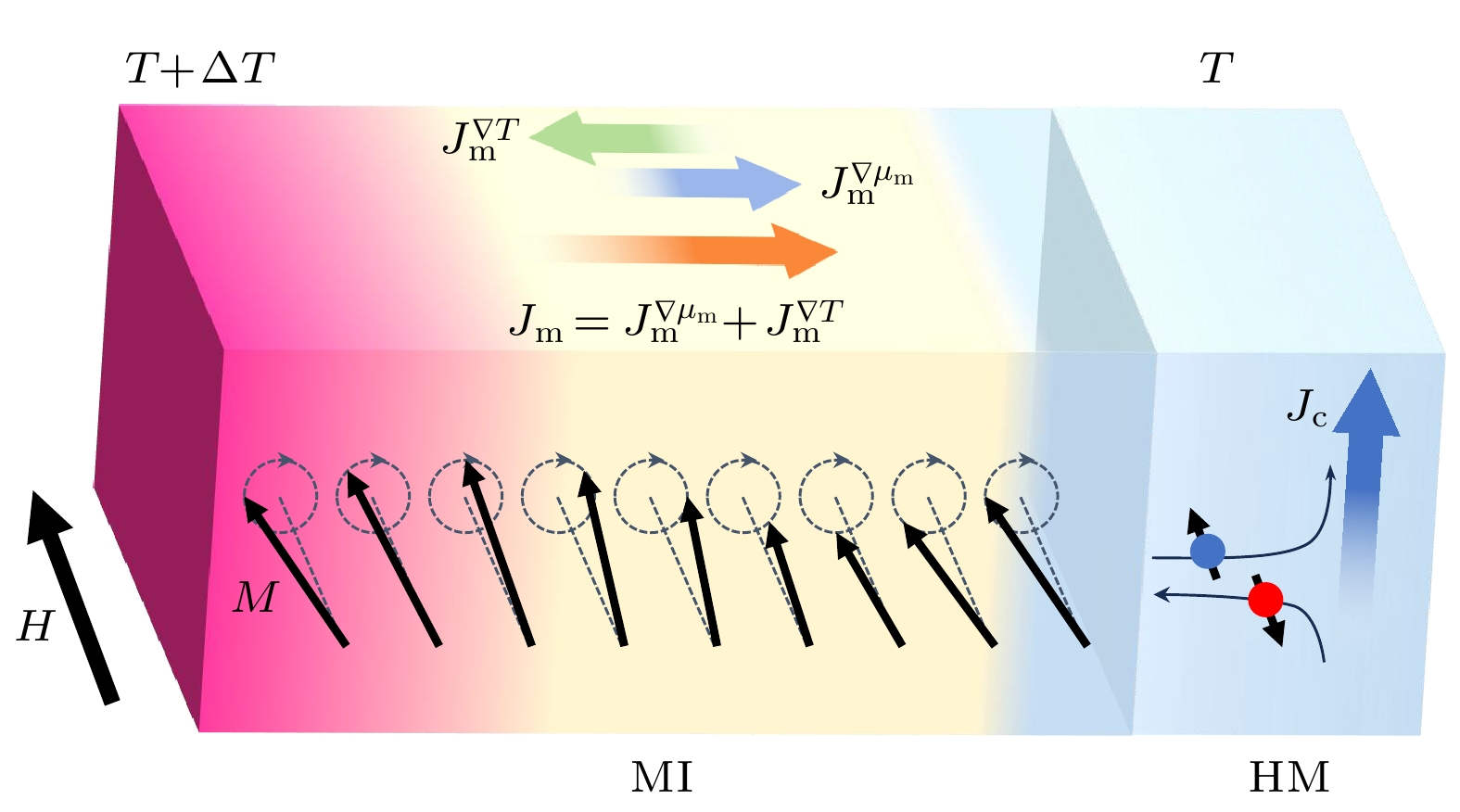
图 1 磁振子自旋塞贝克效应示意图, 铁磁绝缘体(MI)磁振子流的两个来源是温度梯度$ \nabla T $和化学势梯度$ \nabla \mu $, 磁振子流进入近邻的重金属层(HM), 由HM中逆自旋霍尔效应进行探测
Fig. 1. Schematic diagram of magnon spin Seebeck effect. The two sources of magnon current in ferromagnetic insulators (MI) are temperature gradient $ \nabla T $ and chemical potential gradient $ \nabla \mu $. Magnon current enters the neighboring heavy metal layer (HM) and is detected by the inverse spin Hall effect.
图 2 非平衡粒子数$ {\delta n}_{{\mathrm{m}}} $随化学势$ {\mu }_{{\mathrm{m}}} $和$ \alpha $的变化规律
Fig. 2. The variation of nonequilibrium magnon density $ {\delta n}_{{\mathrm{m}}} $with chemical potential $ {\mu }_{{\mathrm{m}}} $ and $ \alpha $.
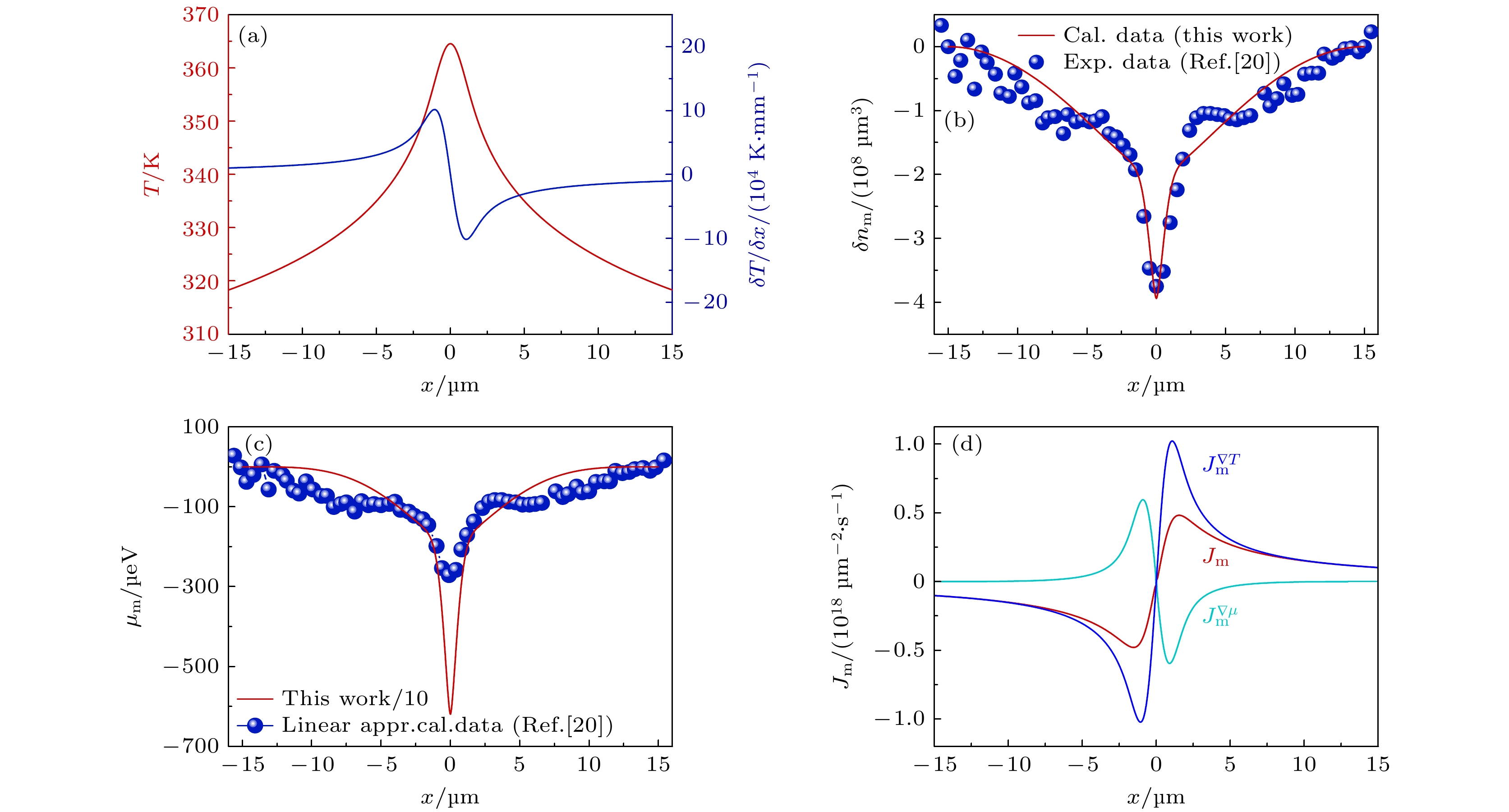
图 3 非平衡磁振子流在非线性温度梯度$ \nabla T \sim {10}^{4}\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}} $下的输运 (a) YIG薄膜上激光辐照光斑附近的温度分布和温度梯度, 数据取自文献[20]; (b)本文的理论结果与位置相关的非平衡磁振子浓度$ \delta {n}_{{\mathrm{m}}} $, 及其与实验数据的对比; (c)本文的理论结果与采用线性近似计算的化学势$ {\mu }_{{\mathrm{m}}} $的对比; (d) MI中的总磁振子流$ {J}_{{\mathrm{m}}} $由两个统计力驱动, 温度梯度$ \nabla T $和化学势$ \nabla {\mu }_{{\mathrm{m}}} $. $ {J}_{{\mathrm{m}}}^{\nabla \mu } $和$ {J}_{{\mathrm{m}}}^{\nabla T} $对总磁振子流$ {J}_{{\mathrm{m}}} $的贡献
Fig. 3. Out-of-equilibrium magnon transport under a nonlinear temperature gradient $ \nabla T \sim {10}^{4}\;{\mathrm{K}}/{\mathrm{m}}{\mathrm{m}} $: (a) Distribution of temperature and temperature gradient near the heating laser spot on the YIG film, data taken from[20]; (b) comparison between our theoretical results and the experimental data for position-dependent nonequilibrium magnon density $ \delta {n}_{{\mathrm{m}}} $; (c) comparison between our theoretical results and the calculated data of linear approximation for position-dependent chemical potential $ {\mu }_{{\mathrm{m}}} $; (d) total magnon current $ {J}_{{\mathrm{m}}} $ in MI driven by two statistical forces: temperature gradient $ \nabla T $ and chemical potential $ \nabla {\mu }_{{\mathrm{m}}}. $ Contribution of $ {J}_{{\mathrm{m}}}^{\nabla \mu } $and $ {J}_{{\mathrm{m}}}^{\nabla T} $ to the total magnon current $ {J}_{{\mathrm{m}}} $.
图 4 MI中恒定温度梯度下的准平衡磁振子输运 (a), (b)恒定温度梯度下非平衡磁振子浓度$ \delta {n}_{{\mathrm{m}}} $和化学势$ {\mu }_{{\mathrm{m}}} $的数值结果, 插图化学势$ {\mu }_{{\mathrm{m}}} $空间分布示意图; (c), (d)不同的$ \nabla T $值下计算得到的样品中心处 $ \delta {n}_{{\mathrm{m}}}^{0} $和$ {\mu }_{{\mathrm{m}}}^{0} $的值
Fig. 4. Quasi-equilibrium magnon transport under a constant temperature gradient in MI: (a), (b) Numerical results for the nonequilibrium magnon density $ \delta {n}_{{\mathrm{m}}} $ and chemical potential $ {\mu }_{{\mathrm{m}}} $ at a constant temperature gradient. Inset is schematic diagram illustrating the spatial-dependent chemical potential $ {\mu }_{{\mathrm{m}}} $; (c), (d) calculated values of $ \delta {n}_{{\mathrm{m}}} $ and $ {\mu }_{{\mathrm{m}}} $ at the center of the sample for different values of $ \nabla T $.
-
[1] Bauer G E W, Saitoh E, van Wees B J 2012 Nat. Mater. 11 391
Google Scholar
[2] Chumak A V, Vasyuchka V I, Serga A A, Hillebrands B 2015 Nat. Phys. 11 453
Google Scholar
[3] Demokritov S O, Demidov V E, Dzyapko O, Melkov G A, Serga A A, Hillebrands B, Slavin A N 2006 Nature 443 430
Google Scholar
[4] Uchida K, Xiao J, Adachi H, et al. 2010 Nat. Mater. 9 894
Google Scholar
[5] Maehrlein S F, Radu I, Maldonado P, et al. 2018 Sci. Adv. 4 eaar5164
Google Scholar
[6] Shi Z, Xi Q, Li J X, Li Y F, Aldosary M, Xu Y D, Zhou J, Zhou S M, Shi J 2021 Phys. Rev. Lett. 127 277203
Google Scholar
[7] Uchida K I, Adachi H, Ota T, Nakayama H, Maekawa S, Saitoh E 2010 Appl. Phys. Lett. 97 172505
Google Scholar
[8] Uchida K, Takahashi S, Harii K, Ieda J, Koshibae W, Ando K, Maekawa S, Saitoh E 2008 Nature 455 778
Google Scholar
[9] Jaworski C M, Yang J, Mack S, Awschalom D D, Heremans J P, Myers R C 2010 Nat. Mater. 9 898
Google Scholar
[10] Jaworski C M, Myers R C, Johnston-Halperin E, Heremans J P 2012 Nature 487 210
Google Scholar
[11] Seki S, Ideue T, Kubota M, et al. 2015 Phys. Rev. Lett. 115 266601
Google Scholar
[12] Jiménez-Cavero P, Lucas I, Anadón A, et al. 2017 APL Mater. 5 026103
Google Scholar
[13] Li J, Shi Z, Ortiz V H, Aldosary M, Chen C, Aji V, Wei P, Shi J 2019 Phys. Rev. Lett. 122 217204
Google Scholar
[14] Ito N, Kikkawa T, Barker J, Hirobe D, Shiomi Y, Saitoh E 2019 Phys. Rev. B 100 60402
[15] Pirro P, Vasyuchka V I, Serga A A, Hillebrands B 2021 Nat. Rev. Mater. 6 1114
Google Scholar
[16] Chumak A V 2019 arXiv: 1901.08934.
[17] Cornelissen L J, Peters K J H, Bauer G E W, Duine R A, van Wees B J 2016 Phys. Rev. B 94 014412
Google Scholar
[18] Zhang S S L, Zhang S F 2012 Phys. Rev. Lett. 109 096603
Google Scholar
[19] Rezende S M, Rodríguez-Suárez R L, Cunha R O, et al. 2014 Phys. Rev. B 89 014416
Google Scholar
[20] Olsson K S, An K, Fiete G A, Zhou J S, Shi L, Li X Q 2020 Phys. Rev. X 10 021029
[21] Demidov V E, Urazhdin S, Divinskiy B, et al. 2017 Nat. Commun. 8 1579
Google Scholar
[22] Du C, Sar T van der, Zhou T X, Upadhyaya P, Casola F, Zhang H, Onbasli M C, Ross C A, Walsworth R L, Tserkovnyak Y, Yacoby A 2017 Science 357 195
Google Scholar
[23] Robinson J E 1951 Phys. Rev. 83 678
Google Scholar
[24] Yi L Z, Yang D C, Liu M, Fu H H, Ding L J, Xu Y L, Zhang B B, Pan L Q, Xiao J Q 2020 Adv. Funct. Mater. 30 2004024
Google Scholar
[25] Princep A J, Ewings R A, Ward S, et al. 2017 Npj Quantum Mater. 2 63
Google Scholar
[26] Son P C van, Kempen H van, Wyder P 1987 Phys. Rev. Lett. 58 2271
Google Scholar
[27] Valet T, Fert A 1993 Phys. Rev. B 48 7099
Google Scholar
[28] Kato Y K, Myers R C, Gossard A C, Awschalom D D 2004 Science 306 1910
Google Scholar
下载:
计量
- 文章访问数: 1295
- PDF下载量: 51
- 被引次数: 0
