-
Absolute gravimeter has played an important role in geophysics, metrology, geological exploration, etc. It is an instrument applying laser interferometry or atom interferometry to the measurement of gravitational acceleration g (approximately 9.8 m/s2). To achieve a high accuracy, a vibration correction method is often employed to reduce the influence of the vibration of the reference object (a retro-reflector or a mirror) on the measurement result of absolute gravimeter. Specifically, in an atomic-interferometry absolute gravimeter, the phase noise caused by the vibration of the reference mirror, namely the vibration phase, can be calculated from the output signal of a sensor, either a seismometer or an accelerometer, placed below or next to the mirror. Considering this vibration phase, the fringe signal of the atomic interferometer as a function of the phase shift set by the control system of the gravimeter can be corrected to approach to an ideal sinusoidal curve, thus reducing the fitting residual. Currently, the parameters in the algorithm of most vibration correction methods used in atomic-interferometry absolute gravimeters are set to be constant. As a result, the performances of these methods may be limited when the practical transfer function between the real vibration of the reference mirror and the signal of the sensor has a variation due to the change of measurement environments. In this paper, based on a simplified model of the practical transfer function previously proposed in an algorithm used in laser-interferometry absolute gravimeter, a new vibration correction method for atomic-interferometry absolute gravimeter is presented. Firstly, a detailed description of its principle is introduced. With a searching algorithm, the time delay and the proportional element in the simplified model can be obtained from the fringe signal of the atomic interferometer and the output of the vibration sensor. In this way, the parameters used to calculate the vibration phase can be adjusted to approach to their true values in different environments, causing the fitting residual of the corrected fringe to decrease as much as possible. Then the measurement results of the homemade NIM-AGRb-1 atomic-interferometry absolute gravimeters using this method is analyzed. It is indicated that with the vibration correction algorithm, the standard deviation of the fitting residual of the measured fringe signal can be reduced by 58% at the best level in a quiet environment. In the future, the performance of this vibration correction algorithm will be further improved in other atomic-interferometry absolute gravimeters during their measurements in hostile environments.
-
Keywords:
- absolute gravimeter /
- vertical correction /
- seismometer /
- atomic interferometry
[1] Marson I, Faller J E 1986 J. Phys. E: Sci. Instrum. 19 22
Google Scholar
[2] Faller J E 2002 Metrologia 39 425
Google Scholar
[3] Steiner R L, Williams E R, Newell D B, Liu R 2005 Metrologia 42 431
Google Scholar
[4] Timmen L, Gitlein O, Klemann V, Wolf D 2011 Pure Appl. Geophys. 169 1331
Google Scholar
[5] Niebauer T M, Sasagawa G S, Faller J E, Hilt R, Klopping F 1995 Metrologia 32 159
Google Scholar
[6] D’Agostino G, Desogus S, Germak A, Origlia C, Quagliotti D, Berrino G, Corrado G, D’errico V, Ricciardi G 2008 Ann. Geophys. 51 39
[7] 胡华, 伍康, 申磊, 李刚, 王力军 2012 61 099101
Google Scholar
Hu H, Wu K, Shen L, Li G, Wang L J 2012 Acta Phys. Sin. 61 099101
Google Scholar
[8] 吴书清, 李春剑, 徐进义, 粟多武, 冯金扬, 吉望西 2017 计量学报 38 127
Google Scholar
Wu S Q, Li C J, Xu J Y, Su D W, Feng J Y, Ji W X 2017 Acta Metrol. Sin. 38 127
Google Scholar
[9] Kasevich M, Chu S 1991 Phys. Rev. Lett. 67 181
Google Scholar
[10] Peters A, Chung K Y, Chu S 1999 Nature 400 6747
Google Scholar
[11] Le Gouët J, Mehlstäubler T, Kim J, Merlet S, Clairon A, Landragin A, Pereira Dos Santos F 2008 Appl. Phys. B 92 133
Google Scholar
[12] Wang S K, Zhao Y, Zhuang W, Li T C, Wu S Q, Feng J Y, Li C J 2018 Metrologia 55 360
Google Scholar
[13] Hu Z K, Sun B L, Duan X C, Zhou M K, Chen L L, Zhan S, Zhang Q Z, Luo J 2013 Phys. Rev. A 88 043610
Google Scholar
[14] Wang Q, Wang Z, Fu Z, Liu W, Lin Q 2016 Opt. Commun. 358 82
Google Scholar
[15] Observations and modeling of seismic background noise, Peterson J https://pubs.er.usgs.gov/publication/ofr93322 [2021-03-29]
[16] Sorrells G G, Douze E J 1974 J. Geophys. Res. 79 4908
Google Scholar
[17] Cessaro R K, Chan W 1989 J. Geophys. Res.-Solid Earth 94 15555
Google Scholar
[18] Richardson L L 2019 Ph. D. Dissertation (Hannover: Institutionelles Repositorium der Leibniz Universität Hannover)
[19] 许翱鹏 2016 博士学位论文 (杭州: 浙江大学)
Xu A P 2016 Ph. D. Dissertation (Hangzhou: Zhejiang University) (in Chinese)
[20] 张旭 2019 硕士学位论文 (长沙: 国防科技大学)
Zhang X 2019 Master Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[21] 王吉鹏, 胡栋, 白金海, 贡昊 2020 计测技术 40 26
Google Scholar
Wang J P, Hu D, Bai J H, Gong H 2020 Metrology & Measurement Technology 40 26
Google Scholar
[22] Tang B, Zhou L, Xiong Z, Wang J, Zhan M 2014 Rev. Sci. Instrum. 85 093109
Google Scholar
[23] 陈斌 2020 博士学位论文 (合肥: 中国科技大学)
Chen B 2020 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[24] 胡青青 2017 博士学位论文(长沙: 国防科技大学)
Hu Q Q 2017 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[25] Hensley J M, Peters A, Chu S 1999 Rev. Sci. Instrum. 70 2735
Google Scholar
[26] Freier C 2010 Ph. D. Dissertation (Berlin: University of Humboldt)
[27] Wang G, Hu H, Wu K, Wang L J 2017 Meas. Sci. Technol. 28 3
Google Scholar
[28] Qian J, Wang G, Wu K, Wang L J 2018 Meas. Sci. Technol. 29 2
Google Scholar
[29] Guo M, Wu K, Yao J, Wen Y, Wang L 2021 IEEE Trans. Instrum. Meas. 70 1004310
Google Scholar
[30] 吴书清, 李天初 2021 光学学报 41 44
Google Scholar
Wu S, Li T 2021 Acta. Optica. Sinica. 41 44
Google Scholar
[31] CMG-3 ESP Operator’s Guide, Guralp Systems Limited, https://www.guralp.com/documents/MAN-ESP-0001.pdf [2021-11-25]
[32] Device Specifications NI 6361, National Instruments, https://www.ni.com/pdf/manuals/374650 c.pdf [2021-11-25]
[33] 伯特瑟卡斯 D P, 齐齐克利斯 J N 著 (郑忠国, 童行伟 译) 2016 概率导论 (北京: 人民邮电出版社) 第190—194页
Bertsekas D P, Tsitsiklis J N (translated by Zheng Z G, Tong X W) 2016 Introduction to Probability (Beijing: POSTS & TELECOM PRESS) pp190–194 (in Chinese)
-
图 1 原子干涉条纹示意图
Figure 1. Schematic diagram of the fringe signal of atomic interferometer.
图 2 地震计输出信号与参考镜真实振动的关系
Figure 2. Transfer function between the vibration of reference mirror and the output signal of seismometer.
图 3 基于系数搜索的振动补偿算法流程
Figure 3. The algorithm of the vibration correction method based on element searching.
图 4 (a) 仿真干涉条纹; (b) 仿真振动信号
Figure 4. (a) The simulated fringe signal; (b) the simulated output of seismometer.
图 5 其他相位噪声为0时仿真得到的拟合RMSE值与(a)延时系数和(b)增益系数的关系
Figure 5. The RMSE value of fringe fitting as a function of (a) time delay and (b) proportional element, without considering other phase noises, calculated by simulation.
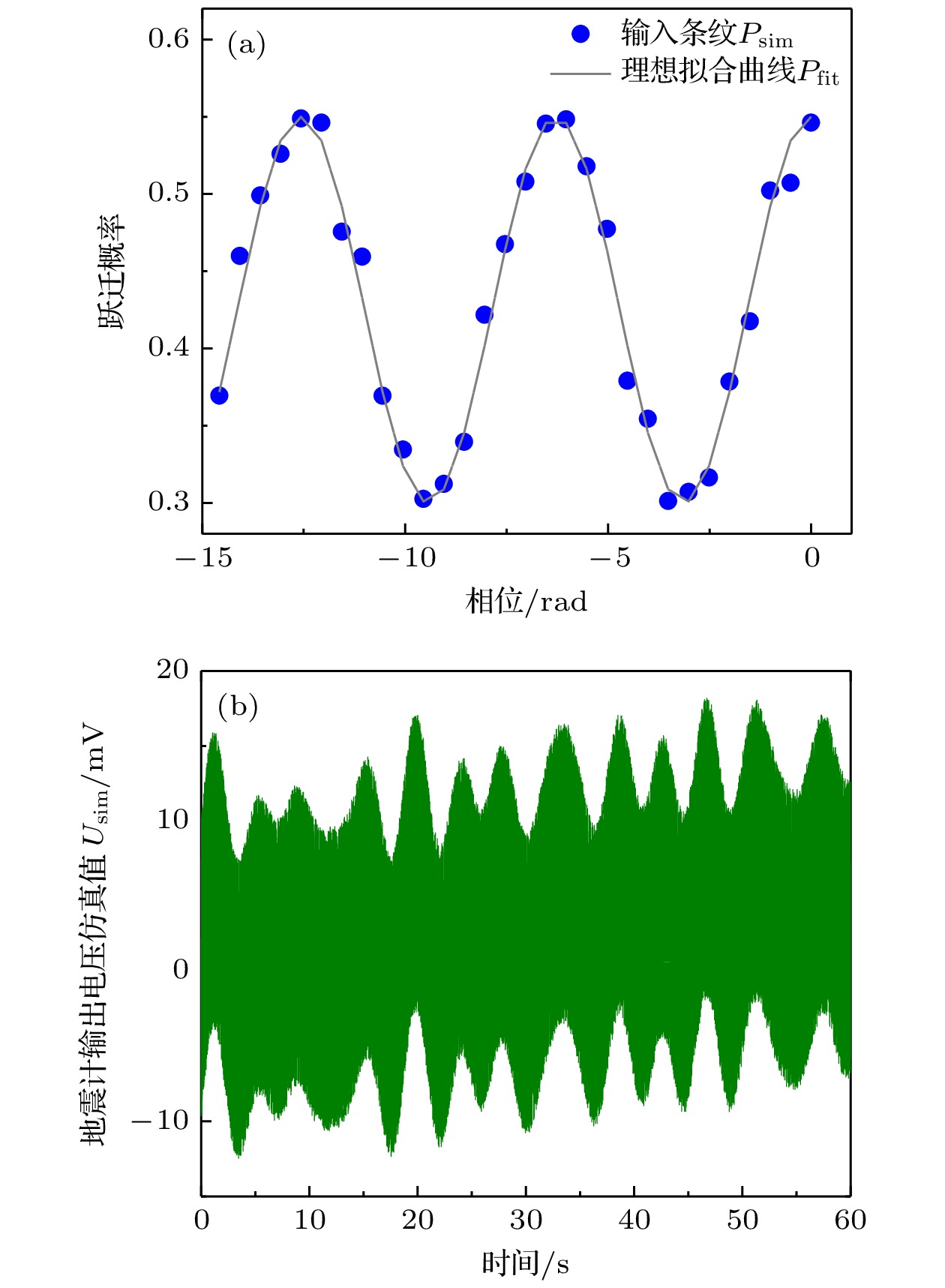
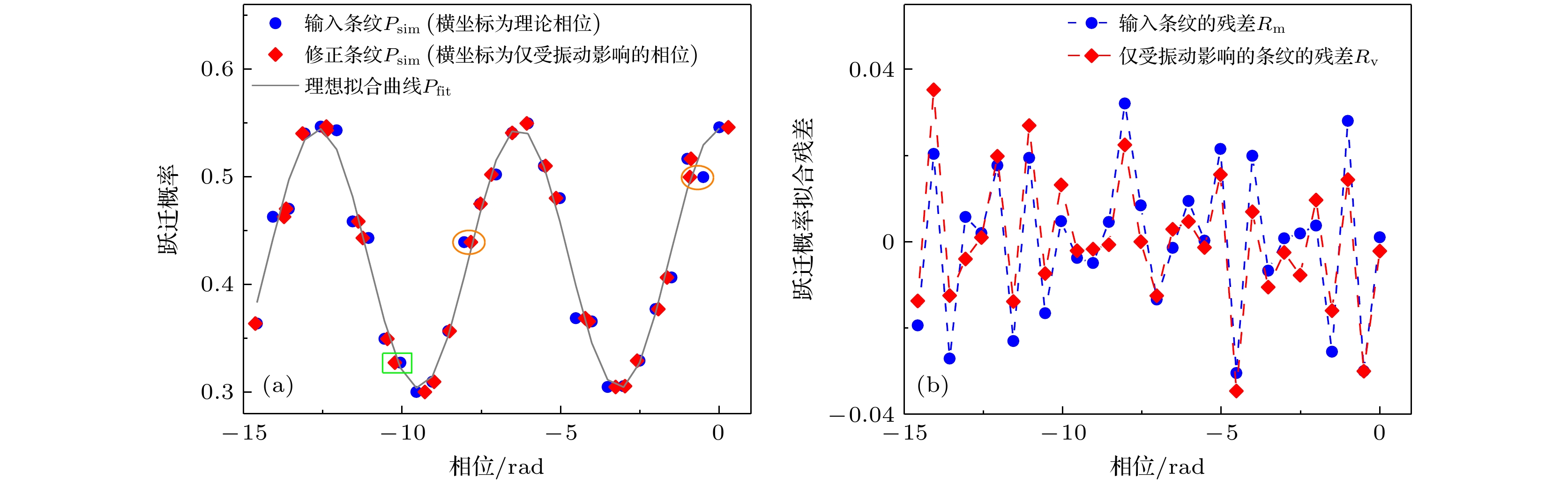
图 6 其他相位噪声为0时仿真得到的(a)修正前后的干涉条纹与理论拟合曲线(典型修正点用橘色圆圈标出), (b)原始条纹的拟合残差与计算出的仅由振动导致的条纹的拟合残差
Figure 6. (a) The simulated fringe signals before and after vibration correction with the fitted curve as the reference (with the typical corrected data marked by orange circles), and (b) the fitting residual of the simulated fringe signals, without considering other phase noises.
图 7 考虑其他相位噪声时仿真得到的拟合RMSE值与(a)延时系数和(b)增益系数的关系
Figure 7. The RMSE value of fringe fitting as a function of (a) time delay and (b) proportional element, with other phase noises considered, calculated by simulation.
图 8 考虑其他相位噪声时仿真得到的(a)修正前后的干涉条纹与理论拟合曲线(典型修正点用橘色圆圈和绿色方框标出), (b)原始残差与计算出的仅由振动导致的条纹残差
Figure 8. (a) The simulated fringe signals before and after vibration correction with the fitted curve as the reference (with the typical corrected data marked by orange circles and green square), and (b) the fitting residual of the simulated fringe signals, with other phase noises considered.
图 9 实验现场图 (a) NIM-AGRb-1型原子重力仪; (b)地震计信号及触发信号采集系统
Figure 9. Photograph of (a) NIM-AGRb-1 atomic-interferometry absolute gravimeter, and (b) the data acquisition device recording the voltage signal of seismometer and trigger.
图 10 测试地点的地面振动加速度功率谱密度
Figure 10. The power spectrum density of the ground vibration acceleration at the measurement site.
图 11 第1组实测数据的拟合RMSE值与(a)延时系数和(b)增益系数的关系
Figure 11. The RMSE value of fringe fitting as a function of (a) time delay and (b) proportional element, calculated from the 1st dataset of measurement.
图 12 第1组实测数据(a)修正前后的干涉条纹与理论拟合曲线(典型修正点用橘色圆圈标出), (b)原始残差与计算出的仅由振动导致的条纹残差
Figure 12. (a) The fringe signals before and after vibration correction with the fitted curve as the reference (with the typical corrected data marked by orange circles), and (b) the fitting residual of the fringe signals, calculated from the 1st dataset of measurement.
图 13 修正前后60组实测干涉条纹的(a)残差标准差和(b)残差标准差的衰减比
Figure 13. (a) The standard deviations of the fitting residuals of 60 sets of fringe signals with and without the vibration correction, and (b) the reducing factor between them.
图 14 由60组实测干涉条纹得到的修正前后的重力偏差
Figure 14. The variation of the measured g value deduced from 60 sets of fringe signals with and without the vibration correction.
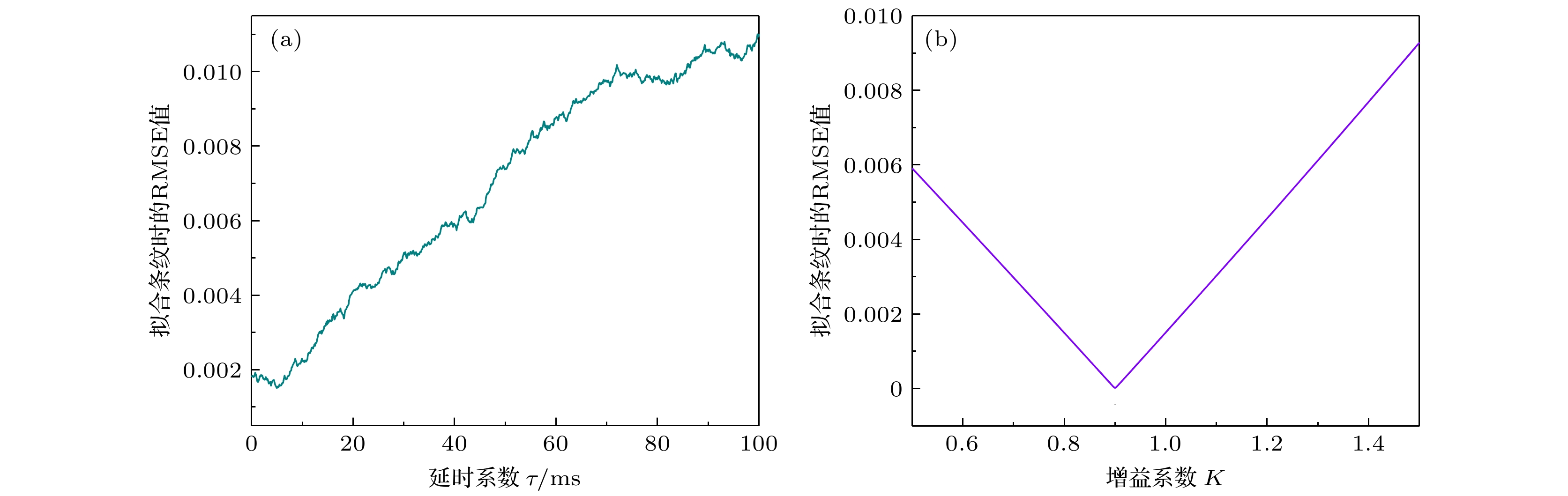
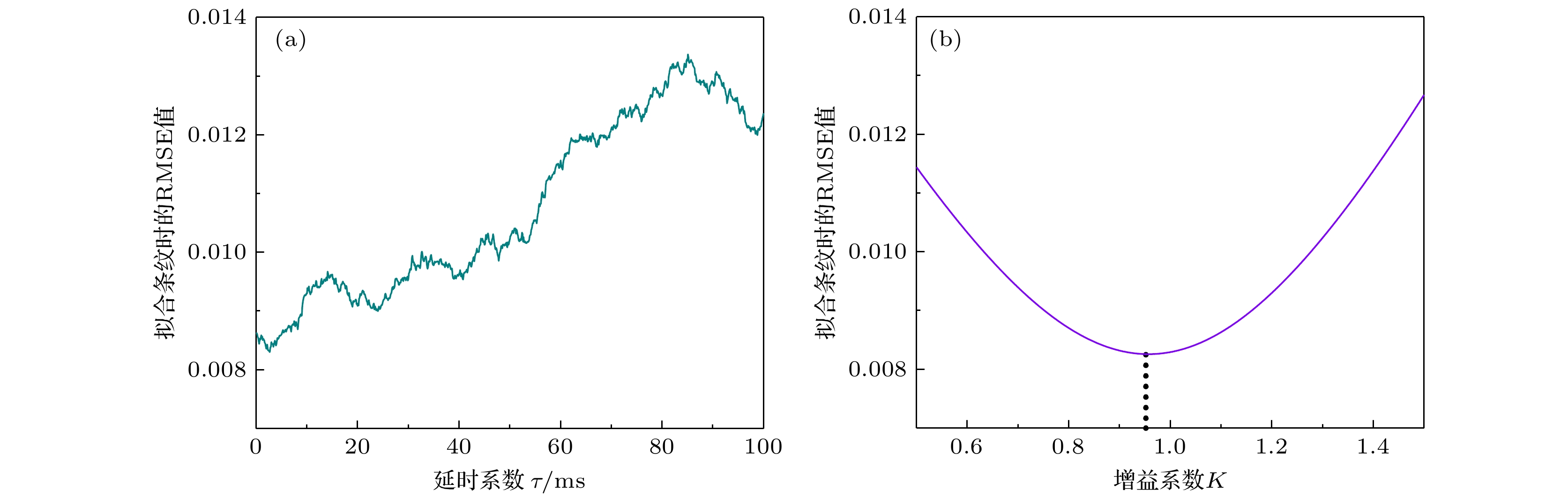
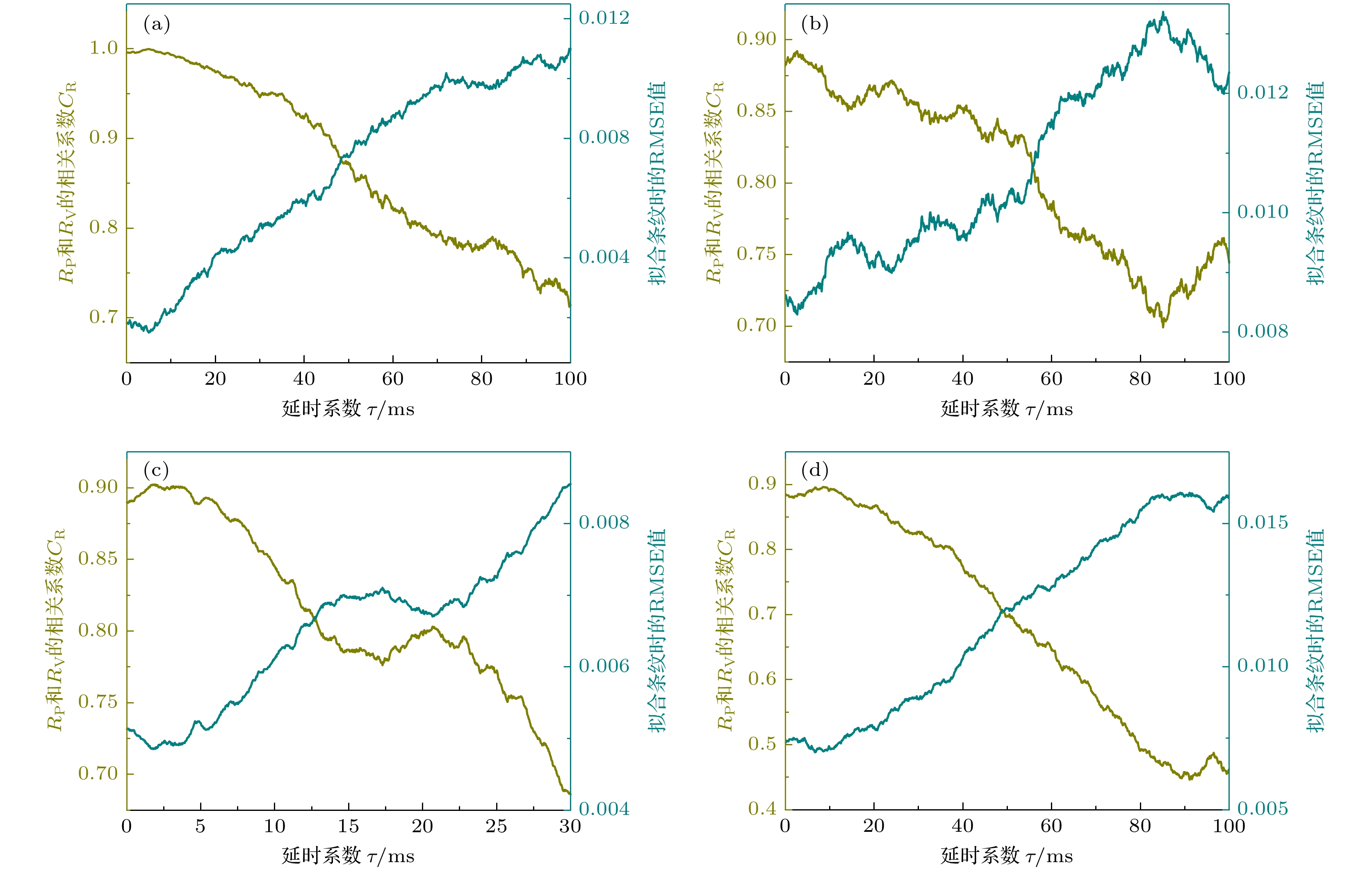
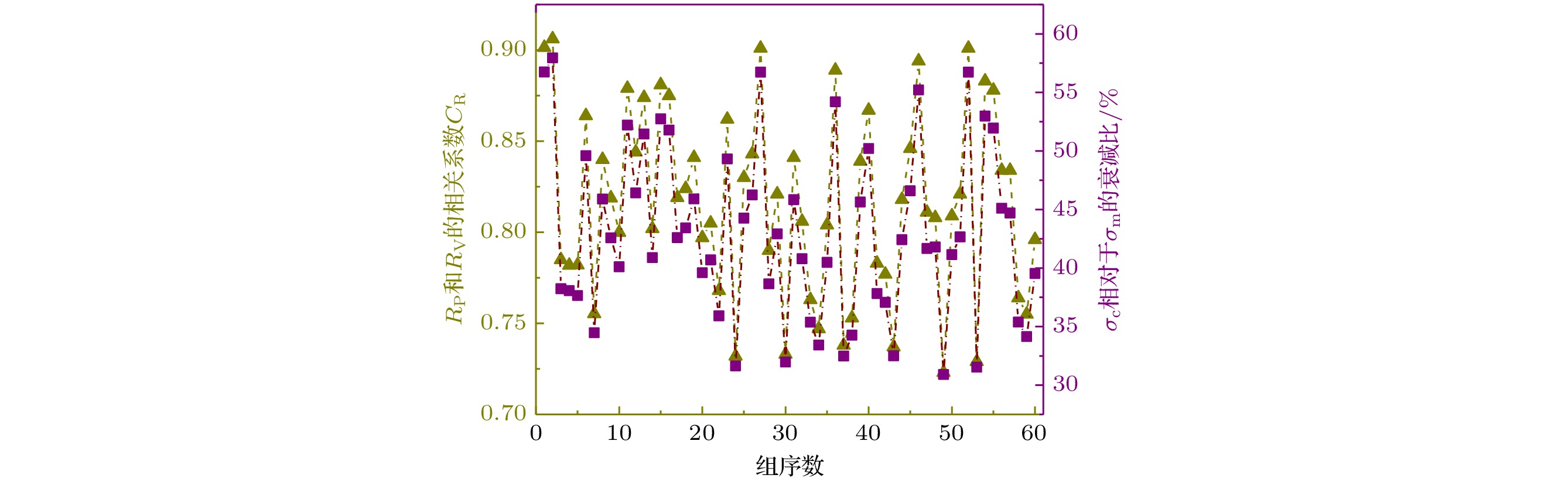
图 15 拟合RMSE值和相关系数CR与延时系数的关系 (a) 仿真时的第1种情况; (b) 仿真时的第2种情况; (c) 实测时的第1组数据; (d) 仿真时的第4种情况
Figure 15. The RMSE value of fringe fitting and the correlation value CR between the fitting residual of fringes before and after the vibration correction as a function of time delay of (a) 1st simulated dataset, (b) 2nd simulated dataset, (c) 1st measured dataset and (d) 4th simulated dataset.
图 16 最终确定的Rv和Rm的相关系数CR与修正前后条纹残差标准差的衰减比
Figure 16. The correlation value CR between the fitting residual of fringes before and after the vibration correction, and the corresponding reducing factor of the standard deviation of the fitting residuals of fringe signals after the vibration correction.
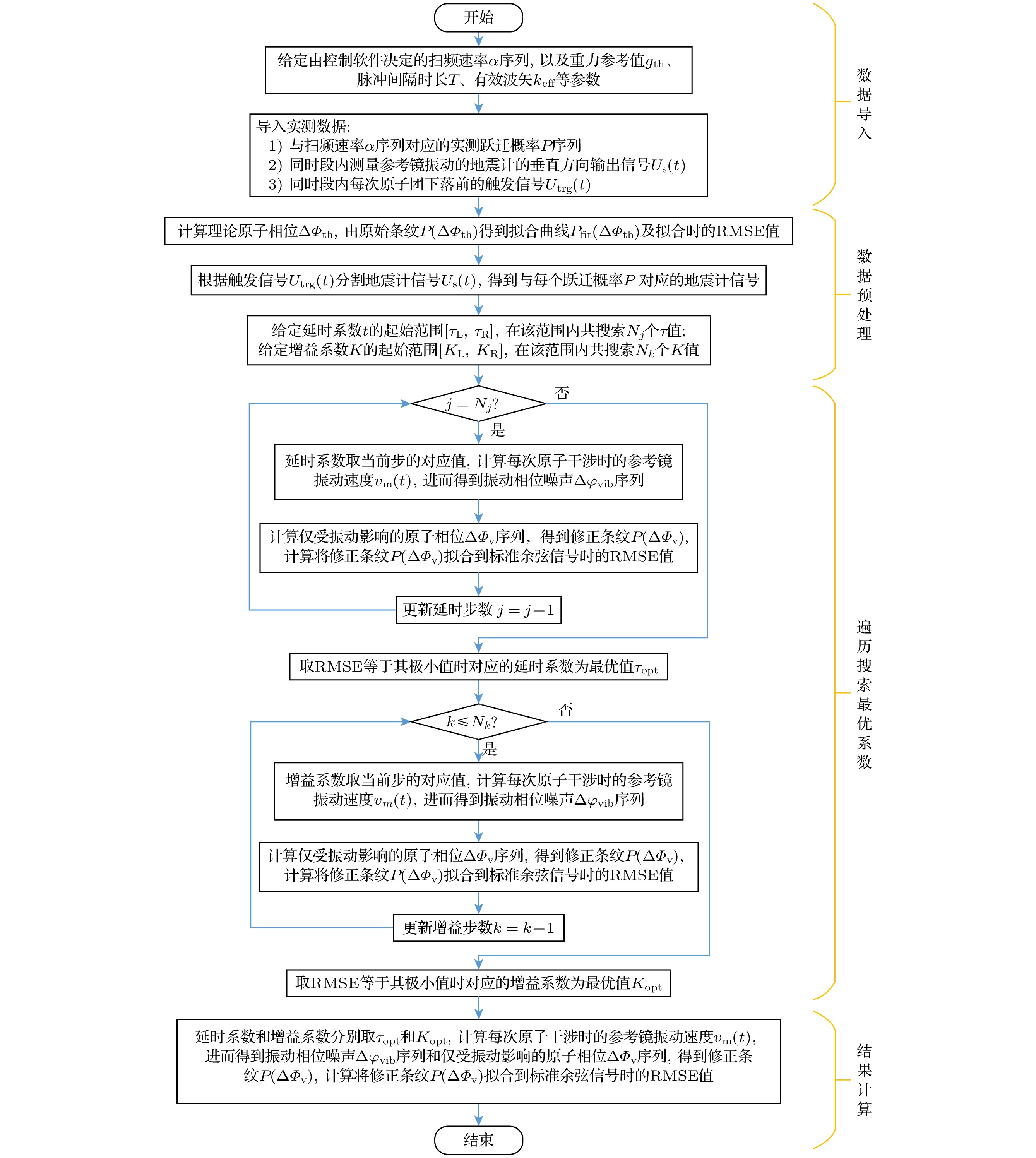
表 1 主要物理符号描述
Table 1. Description of main symbols.
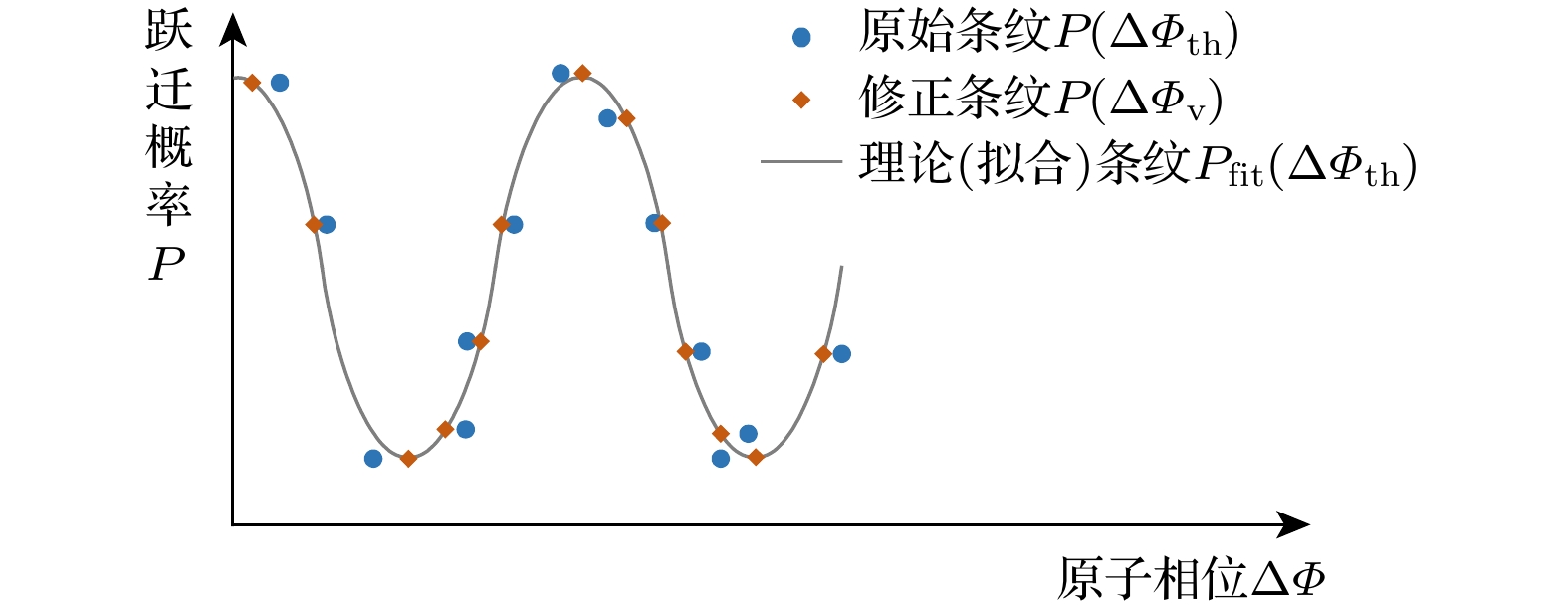
符号 含义 说明 P(ΔФth) 原始干涉条纹 横坐标为理论原子相位ΔФth, 纵坐标为跃迁概率的实测值P Pfit(ΔФth) 理论干涉条纹 横坐标仍为ΔФth, 纵坐标为对P进行余弦拟合得到的拟合值Pfit Us, vm 地震计输出电压, 参考镜真实速度 — τ, K 延时系数, 增益系数 从Us到vm的传递函数H的简化模型中的系数 τopt, Kopt 最优延时系数, 最优增益系数 振动补偿算法搜索出的最优值 Δφvib 推算出的振动相位噪声 从Us根据搜索出的τopt和Kopt以及重力仪灵敏度函数S(t)计算得到 ΔФv 推算出的仅受振动影响的原子相位 — P(ΔФv) 修正干涉条纹 与原始干涉条纹相比, 横坐标由ΔФth变为ΔФv 
DownLoad: CSV
表 2 仿真运算结果
Table 2. Simulated results.
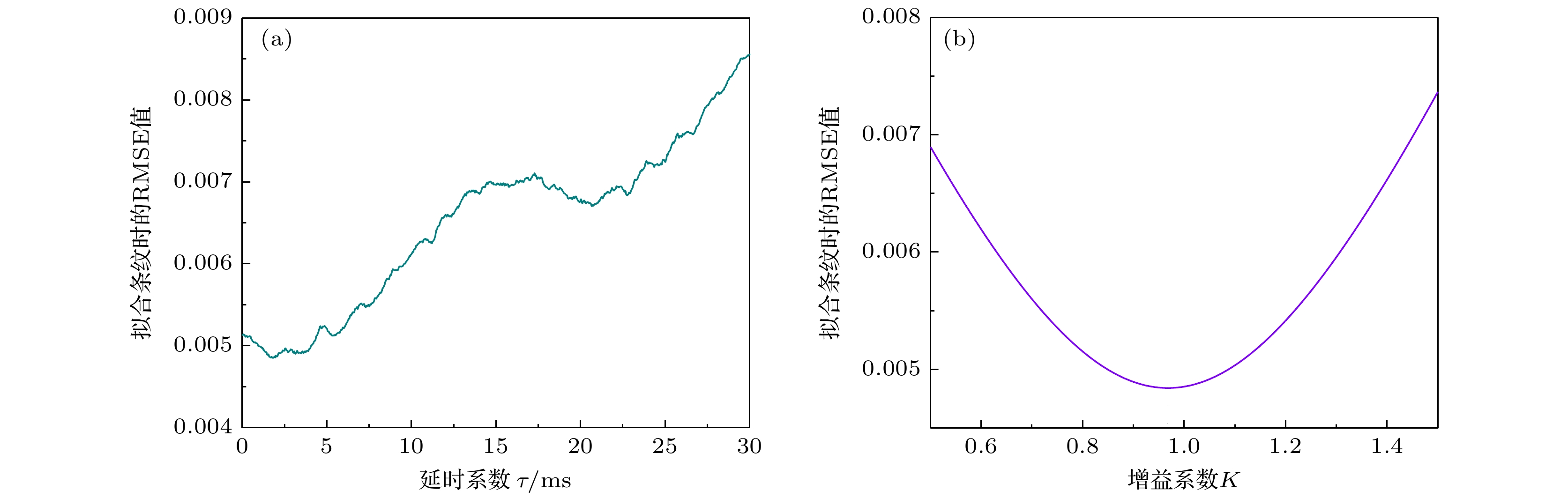
序号 1 2 3 4 输入参数 |Δφvib| ≤ 400 mrad, Δφothers = 0, N = 30 |Δφvib| ≤ 400 mrad, |Δφothers| ≤ 10 mrad, N = 30 |Δφvib| ≤ 4000 mrad, |Δφothers| ≤ 10 mrad, N = 30 |Δφvib| ≤ 400 mrad, |Δφothers| ≤ 10 mrad, N = 60 设定值τset/ms 5 计算值τopt/ms 5.0 2.7 4.1 6.8 设定值Kset 0.9 计算值Kopt 0.900 0.957 0.919 0.876 条纹拟合RMSE值 补偿前/10–3 13.1 18.3 30.9 15.2 补偿后/10–3 0.02 8.3 7.2 6.7 衰减比/% 99.8 54.6 76.7 55.9 条纹残差
标准差补偿前σm/10–3 12.39 17.32 29.28 14.80 补偿后σc/10–3 0.02 7.82 6.78 6.50 衰减比/% 99.8 54.8 76.8 55.7
DownLoad: CSV
表 3 10组数据的实际测量结果
Table 3. Measurement results obtained from 10 data sets.
组号 延时系数τopt/ms 增益系数Kopt 条纹拟合RMSE值 条纹残差标准差 补偿前/10–3 补偿后/10–3 补偿前σm/10–3 补偿后σc/10–3 衰减比/% 1 1.79 0.970 11.2 4.8 10.62 4.58 56.9 2 1.17 1.210 17.3 7.2 16.33 6.83 58.2 3 5.06 1.109 11.4 7.0 10.82 6.67 38.3 4 5.42 1.357 13.8 8.5 13.08 8.05 38.5 5 4.23 1.037 9.1 5.6 8.59 5.30 38.3 6 7.29 1.025 11.7 5.8 11.03 5.52 50.0 7 1.43 1.223 15.1 9.9 14.31 9.34 34.7 8 4.53 1.118 13.6 7.3 12.86 6.94 46.0 9 -0.62 1.057 14.0 8.0 13.19 7.54 42.8 10 5.25 1.079 10.9 6.5 10.31 6.14 40.4 均值 3.56 1.119 — — — — 44.4
DownLoad: CSV
表 4 以相关系数CR为优化目标时的仿真运算结果
Table 4. Simulated results with the correlation value CR as the objective of the algorithm.
序号 输入参数 设定值
τset/ms延时系数τopt/ms 相关系数
CR的极大值以RMSE值为优化目标 以CR为优化目标 1 |Δφvib| ≤ 400 mrad, Δφothers = 0, N = 30 5 5.0 5.0 0.999 2 |Δφvib| ≤ 400 mrad, Δφothers ≤ 10 mrad, N = 30 2.7 2.7 0.892 3 |Δφvib| ≤ 4000 mrad, Δφothers ≤ 10 mrad, N = 30 4.1 4.2 0.972 4 |Δφvib| ≤ 400 mrad, Δφothers ≤ 10 mrad, N = 60 6.8 8.6 0.896
DownLoad: CSV
表 5 以相关系数CR为优化目标时的10组数据的实际测量结果
Table 5. Measurement results obtained from 10 data sets with the correlation value CR as the objective of the algorithm.
组号 计算值τopt /ms 相关系数CR
的最大值以RMSE值为
优化目标以CR为优
化目标1 1.79 1.84 0.902 2 1.17 –4.38 0.909 3 5.06 5.05 0.785 4 5.42 5.27 0.785 5 4.23 4.24 0.787 6 7.29 7.29 0.866 7 1.43 1.42 0.757 8 4.53 4.70 0.841 9 –0.62 –0.83 0.821 10 5.25 5.25 0.802
DownLoad: CSV
表 6 以残差标准差为优化目标时的仿真及实测数据运算结果
Table 6. Simulated and measurement results with the standard deviation of the fitting residuals as the objective of the algorithm
类型 设定值τset /ms 计算值τopt /ms 设定值Kset 计算值Kopt 以RMSE值为优化目标 以σc为优化目标 以RMSE值为优化目标 以σc为优化目标 仿真数据
第3种情况5 4.1 4.2 0.9 0.919 0.918 仿真数据
第4种情况6.8 8.6 0.876 0.865 实测数据
第9组— –0.62 1.32 — 1.057 1.033
DownLoad: CSV
-
[1] Marson I, Faller J E 1986 J. Phys. E: Sci. Instrum. 19 22
Google Scholar
[2] Faller J E 2002 Metrologia 39 425
Google Scholar
[3] Steiner R L, Williams E R, Newell D B, Liu R 2005 Metrologia 42 431
Google Scholar
[4] Timmen L, Gitlein O, Klemann V, Wolf D 2011 Pure Appl. Geophys. 169 1331
Google Scholar
[5] Niebauer T M, Sasagawa G S, Faller J E, Hilt R, Klopping F 1995 Metrologia 32 159
Google Scholar
[6] D’Agostino G, Desogus S, Germak A, Origlia C, Quagliotti D, Berrino G, Corrado G, D’errico V, Ricciardi G 2008 Ann. Geophys. 51 39
[7] 胡华, 伍康, 申磊, 李刚, 王力军 2012 61 099101
Google Scholar
Hu H, Wu K, Shen L, Li G, Wang L J 2012 Acta Phys. Sin. 61 099101
Google Scholar
[8] 吴书清, 李春剑, 徐进义, 粟多武, 冯金扬, 吉望西 2017 计量学报 38 127
Google Scholar
Wu S Q, Li C J, Xu J Y, Su D W, Feng J Y, Ji W X 2017 Acta Metrol. Sin. 38 127
Google Scholar
[9] Kasevich M, Chu S 1991 Phys. Rev. Lett. 67 181
Google Scholar
[10] Peters A, Chung K Y, Chu S 1999 Nature 400 6747
Google Scholar
[11] Le Gouët J, Mehlstäubler T, Kim J, Merlet S, Clairon A, Landragin A, Pereira Dos Santos F 2008 Appl. Phys. B 92 133
Google Scholar
[12] Wang S K, Zhao Y, Zhuang W, Li T C, Wu S Q, Feng J Y, Li C J 2018 Metrologia 55 360
Google Scholar
[13] Hu Z K, Sun B L, Duan X C, Zhou M K, Chen L L, Zhan S, Zhang Q Z, Luo J 2013 Phys. Rev. A 88 043610
Google Scholar
[14] Wang Q, Wang Z, Fu Z, Liu W, Lin Q 2016 Opt. Commun. 358 82
Google Scholar
[15] Observations and modeling of seismic background noise, Peterson J https://pubs.er.usgs.gov/publication/ofr93322 [2021-03-29]
[16] Sorrells G G, Douze E J 1974 J. Geophys. Res. 79 4908
Google Scholar
[17] Cessaro R K, Chan W 1989 J. Geophys. Res.-Solid Earth 94 15555
Google Scholar
[18] Richardson L L 2019 Ph. D. Dissertation (Hannover: Institutionelles Repositorium der Leibniz Universität Hannover)
[19] 许翱鹏 2016 博士学位论文 (杭州: 浙江大学)
Xu A P 2016 Ph. D. Dissertation (Hangzhou: Zhejiang University) (in Chinese)
[20] 张旭 2019 硕士学位论文 (长沙: 国防科技大学)
Zhang X 2019 Master Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[21] 王吉鹏, 胡栋, 白金海, 贡昊 2020 计测技术 40 26
Google Scholar
Wang J P, Hu D, Bai J H, Gong H 2020 Metrology & Measurement Technology 40 26
Google Scholar
[22] Tang B, Zhou L, Xiong Z, Wang J, Zhan M 2014 Rev. Sci. Instrum. 85 093109
Google Scholar
[23] 陈斌 2020 博士学位论文 (合肥: 中国科技大学)
Chen B 2020 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[24] 胡青青 2017 博士学位论文(长沙: 国防科技大学)
Hu Q Q 2017 Ph. D. Dissertation (Changsha: National University of Defense Technology) (in Chinese)
[25] Hensley J M, Peters A, Chu S 1999 Rev. Sci. Instrum. 70 2735
Google Scholar
[26] Freier C 2010 Ph. D. Dissertation (Berlin: University of Humboldt)
[27] Wang G, Hu H, Wu K, Wang L J 2017 Meas. Sci. Technol. 28 3
Google Scholar
[28] Qian J, Wang G, Wu K, Wang L J 2018 Meas. Sci. Technol. 29 2
Google Scholar
[29] Guo M, Wu K, Yao J, Wen Y, Wang L 2021 IEEE Trans. Instrum. Meas. 70 1004310
Google Scholar
[30] 吴书清, 李天初 2021 光学学报 41 44
Google Scholar
Wu S, Li T 2021 Acta. Optica. Sinica. 41 44
Google Scholar
[31] CMG-3 ESP Operator’s Guide, Guralp Systems Limited, https://www.guralp.com/documents/MAN-ESP-0001.pdf [2021-11-25]
[32] Device Specifications NI 6361, National Instruments, https://www.ni.com/pdf/manuals/374650 c.pdf [2021-11-25]
[33] 伯特瑟卡斯 D P, 齐齐克利斯 J N 著 (郑忠国, 童行伟 译) 2016 概率导论 (北京: 人民邮电出版社) 第190—194页
Bertsekas D P, Tsitsiklis J N (translated by Zheng Z G, Tong X W) 2016 Introduction to Probability (Beijing: POSTS & TELECOM PRESS) pp190–194 (in Chinese)
DownLoad:
Catalog
Metrics
- Abstract views: 5048
- PDF Downloads: 80
- Cited By: 0
