-
Semi-quantum secure direct communication allows the quantum party and the classical party to transmit secure messages directly, but does not need sharing a secret key in advance. To increase the information transmission efficiency and practicability of semi-quantum secure direct communication, a bidirectional semi-quantum secure direct communication protocol with high-dimensional single-particle states is designed. The proposed protocol involves quantum party Alice and classical party Bob. Each participant can receive a secret message while sending a secret message. Unlike most of existing quantum secure direct communication protocols, it is not necessary for the classical party Bob in the proposed protocol to possess the capability of measuring quantum states, which greatly enhances the feasibility of the protocol. The protocol allows the classical party Bob to implement the unitary operations on particles and reorder the quantum sequence. Furthermore, the quantum party Alice and the classical party Bob can verify the correctness of the received secret message with the Hash function. Security analysis indicates that without being discovered by the legitimate participants, Eve cannot obtain the secret message with common attack, such as intercept-resend attack, measure-resend attack, tampering attack and entanglement-measure attack. Compared with the typical semi-quantum secure direct communication protocols, the proposed protocol has a high qubit efficiency of about 28.6%. In addition, the transmission efficiency of secret message is greatly enhanced, since the proposed protocol utilizes the high-dimensional single-particle states as the carrier of secret message.
-
Keywords:
- semi-quantum secure direct communication /
- high-dimensional single-particle state /
- bi-directional communication /
- security analysis
[1] Bennett C H, Brassard G, Crepeau C, Jozsa R, Peres A, Wootters W K 1993 Phys. Rev. Lett. 70 1895
Google Scholar
[2] Li X H, Ghose S 2015 Phys. Rev. A 91 012320
Google Scholar
[3] 杨璐, 马鸿洋, 郑超, 丁晓兰, 高健存, 龙桂鲁 2017 66 230303
Google Scholar
Yang L, Ma H Y, Zheng C, Ding X L, Gao J C, Long G L 2017 Acta Phys. Sin. 66 230303
Google Scholar
[4] Vlachou C, Krawec W, Mateus P, Paunković N, Souto A 2018 Quantum Inf. Process. 17 288
Google Scholar
[5] 吴承峰, 杜亚男, 王金东, 魏正军, 秦晓娟, 赵峰, 张智明 2016 65 100302
Google Scholar
Wu C F, Du Y N, Wang J D, Wei Z J, Qin X J, Zhao F, Zhang Z M 2016 Acta Phys. Sin. 65 100302
Google Scholar
[6] 安雪碧, 银振强, 韩正甫 2015 64 140303
Google Scholar
An X B, Yin Z Q, Han Z F 2015 Acta Phys. Sin. 64 140303
Google Scholar
[7] 冯艳艳, 施荣华, 石金晶, 郭迎 2019 68 120302
Google Scholar
Feng Y Y, Shi R H, Shi J J, Guo Y 2019 Acta Phys. Sin. 68 120302
Google Scholar
[8] 荣民希, 辛向军, 李发根 2020 69 190302
Google Scholar
Rong M X, Xin X J, Li F G 2020 Acta Phys. Sin. 69 190302
Google Scholar
[9] 张沛, 周小清, 李智伟 2014 63 130301
Google Scholar
Zhang P, Zhou X Q, Li Z W 2014 Acta Phys. Sin. 63 130301
Google Scholar
[10] Chen F L, Zhang H, Chen S G, Cheng W T 2021 Quantum Inf. Process. 20 178
Google Scholar
[11] Jiang D H, Tang K K, Xu G B 2021 Int. J. Theor. Phys. 60 4122
Google Scholar
[12] Ma Z H, Chen J Y, Li Z, Tang C, Sua Y M, Fan H, Huang Y P 2020 Phys. Rev. Lett. 125 263602
Google Scholar
[13] Wang Q Q, Zheng Y, Zhai C H, Li X D, Gong Q H, Wang J W 2021 J. Semicond. 42 091901
Google Scholar
[14] Long G L, Liu X S 2002 Phys. Rev. A 65 032302
Google Scholar
[15] Boström K, Felbinger T 2002 Phys. Rev. Lett. 89 187902
Google Scholar
[16] Deng F G, Long G L, Liu X S 2003 Phys. Rev. A 68 042317
Google Scholar
[17] Deng F G, Long G L 2004 Phys. Rev. A 69 052319
Google Scholar
[18] Wang C, Deng F G, Li Y S, Liu X S, Long G L 2005 Phys. Rev. A 71 044305
Google Scholar
[19] Shi J, Gong Y X, Xu P, Zhu S N, Zhan Y B 2011 Commun. Theor. Phys. 56 831
Google Scholar
[20] 郑晓毅 龙银香 2017 66 180303
Google Scholar
Zheng X Y, Long Y X 2017 Acta Phys. Sin. 66 180303
Google Scholar
[21] Chen S S, Zhou L, Zhong W, Sheng Y B 2018 Sci. Chin. -Phys. Mech. Astron. 61 90312
Google Scholar
[22] Gao Z K, Li T, Li Z H 2019 EPL 125 40004
Google Scholar
[23] Zhou L, Sheng Y B, Long G L 2020 Sci. Bull. 65 12
Google Scholar
[24] Sheng Y B, Zhou L, Long G L 2022 Sci. Bull. 67 367
Google Scholar
[25] Hu J Y, Yu B, Jing M Y, Xiao L T, Jia S T, Qin G Q, Long G L 2016 Light Sci. Appl. 5 e16144
Google Scholar
[26] Zhang W, Ding D S, Sheng Y B, Zhou L, Shi B S, Guo G C 2017 Phys. Rev. Lett. 118 220501
Google Scholar
[27] Zhu F, Zhang W, Sheng Y B, Huang Y D 2017 Sci. Bull. 62 1519
Google Scholar
[28] Qi Z T, Li Y H, Huang Y W, Feng J, Zheng Y L, Chen X F 2021 Light Sci. Appl. 10 183
Google Scholar
[29] Boyer M, Kenigsberg D, Mor T 2007 Phys. Rev. Lett. 99 140501
Google Scholar
[30] Zhou N R, Zhu K N, Bi W, Gong L H 2019 Quantum Inf. Process. 18 197
Google Scholar
[31] Tsai C W, Yang C W 2021 Sci. Rep. 11 23222
Google Scholar
[32] Han S Y, Huang Y T, Mi S, Qin X J, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2021 EPJ Quantum Technol. 8 28
Google Scholar
[33] Jiang S Q, Zhou R G, Hu W W 2021 Int. J. Theor. Phys. 60 3353
Google Scholar
[34] Zhou N R, Xu Q D, Du N S, Gong L H 2021 Quantum Inf. Process. 20 124
Google Scholar
[35] Ye C Q, Li J, Chen X B, Yuan T 2021 Quantum Inf. Process. 20 262
Google Scholar
[36] Zou X F, Qiu D W 2014 Sci. Chin. -Phys. Mech. Astron. 57 1696
Google Scholar
[37] Gu J, Lin P H, Hwang T 2018 Quantum Inf. Process. 17 182
Google Scholar
[38] Zhang M H, Li H F, Xia Z Q, Feng X Y, Peng J Y 2017 Quantum Inf. Process. 16 117
Google Scholar
[39] Xie C, Li L Z, Situ H Z, He J H 2018 Int. J. Theor. Phys. 57 1881
Google Scholar
[40] Sun Y H, Yan L L, Chang Y, Zhang S B, Shao T T, Zhang Y 2019 Mod. Phys. Lett. A 34 1950004
[41] Rong Z B, Qiu D W, Zou X F 2020 Int. J. Theor. Phys. 59 1807
Google Scholar
[42] Ye C Q, Ye T Y, He D, Gan Z G 2019 Int. J. Theor. Phys. 58 3797
Google Scholar
[43] Wen X J, Zhao X Q, Gong L H, Zhou N R 2019 Laser Phys. Lett. 16 075206
Google Scholar
-
图 1 窃听检测概率
Figure 1. Eavesdropping detection probability.
图 2 单粒子传输秘密信息-维数
Figure 2. Single particle transport secret information - dimension.
表 1 操作后粒子的分类
Table 1. Classification of the particles after operation.
原始状态所属基 Bob的操作 标记为 $\overline Z $ CTRL $\overline Z - {\text{CTRL}}$ $\overline Z $ ${U_m}$ $\overline Z - U$ $\overline X $ CTRL $\overline X - {\text{CTRL}}$ $\overline X $ ${U_m}$ $\overline X - U$ 
DownLoad: CSV
表 2 Alice的窃听检测策略
Table 2. Eavesdropping detection strategy for Alice.
原始状态 Bob的操作 Alice的操作 预期结果 $\left| k \right\rangle $ CTRL $\overline Z $基测量 $\left| k \right\rangle $ $\left| k \right\rangle $ ${U_m}$ $\overline Z $基测量 $\left| {k \oplus m} \right\rangle $ $F\left| k \right\rangle $ CTRL $\overline X $基测量 $F\left| k \right\rangle $ $F\left| k \right\rangle $ ${U_m}$ $\overline X $基测量 $F\left| k \right\rangle $
DownLoad: CSV
表 3 Eve的截获重发攻击
Table 3. Intercept-resend attack by Eve.
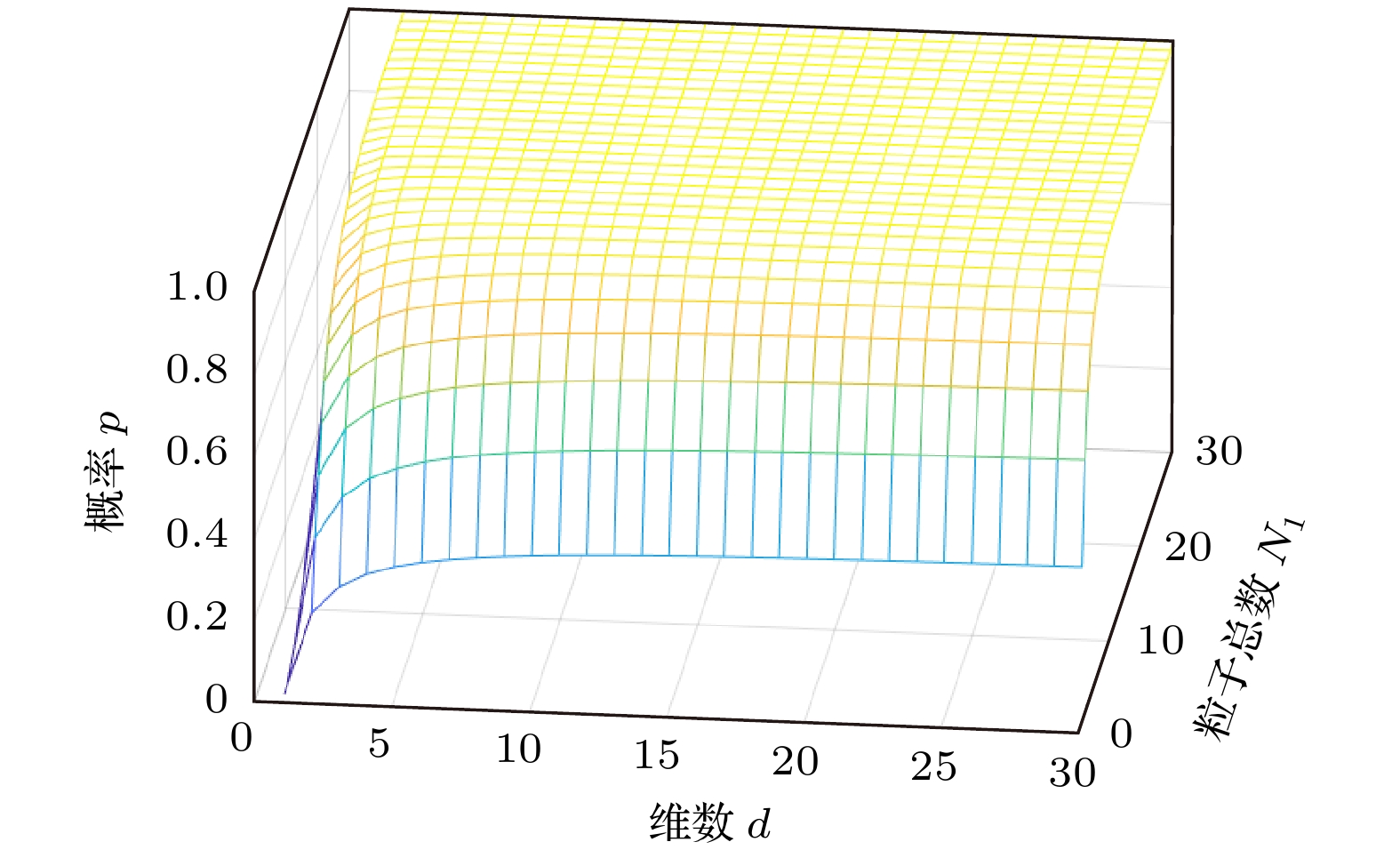
Alice发送的粒子 Bob的操作 Eve的操作 Alice的操作 窃听是否会被发现 $\overline Z $ CTRL CTRL 用$\overline Z $基测量 否 $\overline Z $ CTRL ${U_m}$ 用$\overline Z $基测量 是 $\overline Z $ ${U_m}$ CTRL 用$\overline Z $基测量 $\dfrac{ {d - 1} }{ {2 d} }$的概率被发现 $\overline Z $ ${U_m}$ ${U_m}$ 用$\overline Z $基测量 $\dfrac{ {d - 1} }{ {2 d} }$的概率被发现 $\overline X $ CTRL CTRL 用$\overline X $基测量 否 $\overline X $ CTRL ${U_m}$ 用$\overline X $基测量 否 $\overline X $ ${U_m}$ CTRL 用$\overline X $基测量 否 $\overline X $ ${U_m}$ ${U_m}$ 用$\overline X $基测量 否
DownLoad: CSV
表 4 Eve的测量重发攻击
Table 4. Measurement-resend attack by Eve.
Alice发送的粒子 Eve的测量基 Bob得到的粒子 Bob的操作 Alice的操作 窃听是否被发现 $\overline Z $ $\overline Z $ $\overline Z $ CTRL $\overline Z $基测量 否 $\overline Z $ $\overline Z $ $\overline Z $ ${U_m}$ $\overline Z $基测量 否 $\overline Z $ $\overline X $ $\overline X $ CTRL $\overline Z $基测量 $\dfrac{ {d - 1} }{d}$概率被发现 $\overline Z $ $\overline X $ $\overline X $ ${U_m}$ $\overline Z $基测量 $\dfrac{ {d - 1} }{ {2 d} }$概率被发现 $\overline X $ $\overline Z $ $\overline Z $ CTRL $\overline X $基测量 $\dfrac{ {d - 1} }{d}$概率被发现 $\overline X $ $\overline Z $ $\overline Z $ ${U_m}$ $\overline X $基测量 $\dfrac{ {d - 1} }{d}$概率被发现 $\overline X $ $\overline X $ $\overline X $ CTRL $\overline X $基测量 否 $\overline X $ $\overline X $ $\overline X $ ${U_m}$ $\overline X $基测量 否
DownLoad: CSV
表 5 本协议与现有经典SQSDC协议的比较
Table 5. Comparison of the proposed protocol with existing classical SQSDC protocols.
DownLoad: CSV
-
[1] Bennett C H, Brassard G, Crepeau C, Jozsa R, Peres A, Wootters W K 1993 Phys. Rev. Lett. 70 1895
Google Scholar
[2] Li X H, Ghose S 2015 Phys. Rev. A 91 012320
Google Scholar
[3] 杨璐, 马鸿洋, 郑超, 丁晓兰, 高健存, 龙桂鲁 2017 66 230303
Google Scholar
Yang L, Ma H Y, Zheng C, Ding X L, Gao J C, Long G L 2017 Acta Phys. Sin. 66 230303
Google Scholar
[4] Vlachou C, Krawec W, Mateus P, Paunković N, Souto A 2018 Quantum Inf. Process. 17 288
Google Scholar
[5] 吴承峰, 杜亚男, 王金东, 魏正军, 秦晓娟, 赵峰, 张智明 2016 65 100302
Google Scholar
Wu C F, Du Y N, Wang J D, Wei Z J, Qin X J, Zhao F, Zhang Z M 2016 Acta Phys. Sin. 65 100302
Google Scholar
[6] 安雪碧, 银振强, 韩正甫 2015 64 140303
Google Scholar
An X B, Yin Z Q, Han Z F 2015 Acta Phys. Sin. 64 140303
Google Scholar
[7] 冯艳艳, 施荣华, 石金晶, 郭迎 2019 68 120302
Google Scholar
Feng Y Y, Shi R H, Shi J J, Guo Y 2019 Acta Phys. Sin. 68 120302
Google Scholar
[8] 荣民希, 辛向军, 李发根 2020 69 190302
Google Scholar
Rong M X, Xin X J, Li F G 2020 Acta Phys. Sin. 69 190302
Google Scholar
[9] 张沛, 周小清, 李智伟 2014 63 130301
Google Scholar
Zhang P, Zhou X Q, Li Z W 2014 Acta Phys. Sin. 63 130301
Google Scholar
[10] Chen F L, Zhang H, Chen S G, Cheng W T 2021 Quantum Inf. Process. 20 178
Google Scholar
[11] Jiang D H, Tang K K, Xu G B 2021 Int. J. Theor. Phys. 60 4122
Google Scholar
[12] Ma Z H, Chen J Y, Li Z, Tang C, Sua Y M, Fan H, Huang Y P 2020 Phys. Rev. Lett. 125 263602
Google Scholar
[13] Wang Q Q, Zheng Y, Zhai C H, Li X D, Gong Q H, Wang J W 2021 J. Semicond. 42 091901
Google Scholar
[14] Long G L, Liu X S 2002 Phys. Rev. A 65 032302
Google Scholar
[15] Boström K, Felbinger T 2002 Phys. Rev. Lett. 89 187902
Google Scholar
[16] Deng F G, Long G L, Liu X S 2003 Phys. Rev. A 68 042317
Google Scholar
[17] Deng F G, Long G L 2004 Phys. Rev. A 69 052319
Google Scholar
[18] Wang C, Deng F G, Li Y S, Liu X S, Long G L 2005 Phys. Rev. A 71 044305
Google Scholar
[19] Shi J, Gong Y X, Xu P, Zhu S N, Zhan Y B 2011 Commun. Theor. Phys. 56 831
Google Scholar
[20] 郑晓毅 龙银香 2017 66 180303
Google Scholar
Zheng X Y, Long Y X 2017 Acta Phys. Sin. 66 180303
Google Scholar
[21] Chen S S, Zhou L, Zhong W, Sheng Y B 2018 Sci. Chin. -Phys. Mech. Astron. 61 90312
Google Scholar
[22] Gao Z K, Li T, Li Z H 2019 EPL 125 40004
Google Scholar
[23] Zhou L, Sheng Y B, Long G L 2020 Sci. Bull. 65 12
Google Scholar
[24] Sheng Y B, Zhou L, Long G L 2022 Sci. Bull. 67 367
Google Scholar
[25] Hu J Y, Yu B, Jing M Y, Xiao L T, Jia S T, Qin G Q, Long G L 2016 Light Sci. Appl. 5 e16144
Google Scholar
[26] Zhang W, Ding D S, Sheng Y B, Zhou L, Shi B S, Guo G C 2017 Phys. Rev. Lett. 118 220501
Google Scholar
[27] Zhu F, Zhang W, Sheng Y B, Huang Y D 2017 Sci. Bull. 62 1519
Google Scholar
[28] Qi Z T, Li Y H, Huang Y W, Feng J, Zheng Y L, Chen X F 2021 Light Sci. Appl. 10 183
Google Scholar
[29] Boyer M, Kenigsberg D, Mor T 2007 Phys. Rev. Lett. 99 140501
Google Scholar
[30] Zhou N R, Zhu K N, Bi W, Gong L H 2019 Quantum Inf. Process. 18 197
Google Scholar
[31] Tsai C W, Yang C W 2021 Sci. Rep. 11 23222
Google Scholar
[32] Han S Y, Huang Y T, Mi S, Qin X J, Wang J D, Yu Y F, Wei Z J, Zhang Z M 2021 EPJ Quantum Technol. 8 28
Google Scholar
[33] Jiang S Q, Zhou R G, Hu W W 2021 Int. J. Theor. Phys. 60 3353
Google Scholar
[34] Zhou N R, Xu Q D, Du N S, Gong L H 2021 Quantum Inf. Process. 20 124
Google Scholar
[35] Ye C Q, Li J, Chen X B, Yuan T 2021 Quantum Inf. Process. 20 262
Google Scholar
[36] Zou X F, Qiu D W 2014 Sci. Chin. -Phys. Mech. Astron. 57 1696
Google Scholar
[37] Gu J, Lin P H, Hwang T 2018 Quantum Inf. Process. 17 182
Google Scholar
[38] Zhang M H, Li H F, Xia Z Q, Feng X Y, Peng J Y 2017 Quantum Inf. Process. 16 117
Google Scholar
[39] Xie C, Li L Z, Situ H Z, He J H 2018 Int. J. Theor. Phys. 57 1881
Google Scholar
[40] Sun Y H, Yan L L, Chang Y, Zhang S B, Shao T T, Zhang Y 2019 Mod. Phys. Lett. A 34 1950004
[41] Rong Z B, Qiu D W, Zou X F 2020 Int. J. Theor. Phys. 59 1807
Google Scholar
[42] Ye C Q, Ye T Y, He D, Gan Z G 2019 Int. J. Theor. Phys. 58 3797
Google Scholar
[43] Wen X J, Zhao X Q, Gong L H, Zhou N R 2019 Laser Phys. Lett. 16 075206
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3858
- PDF Downloads: 99
- Cited By: 0
