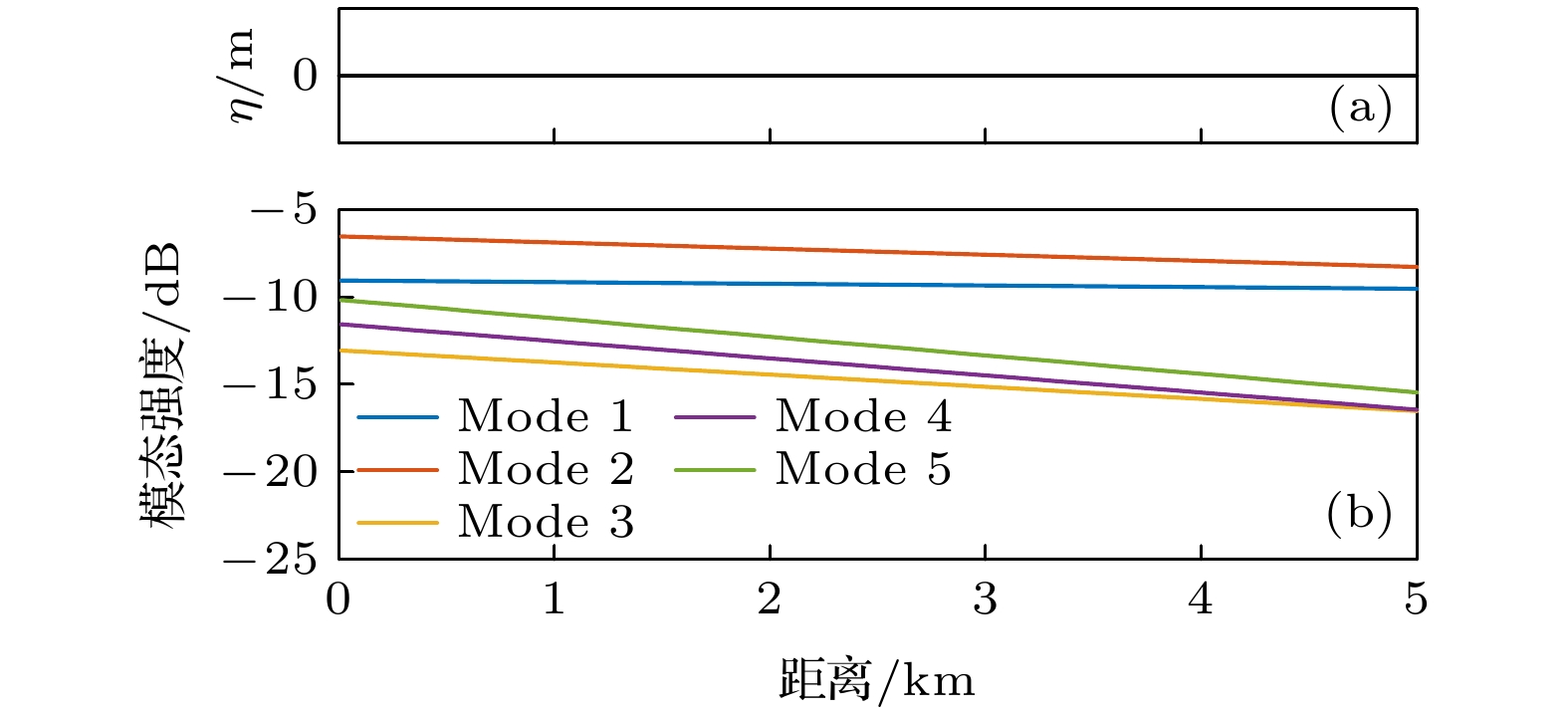
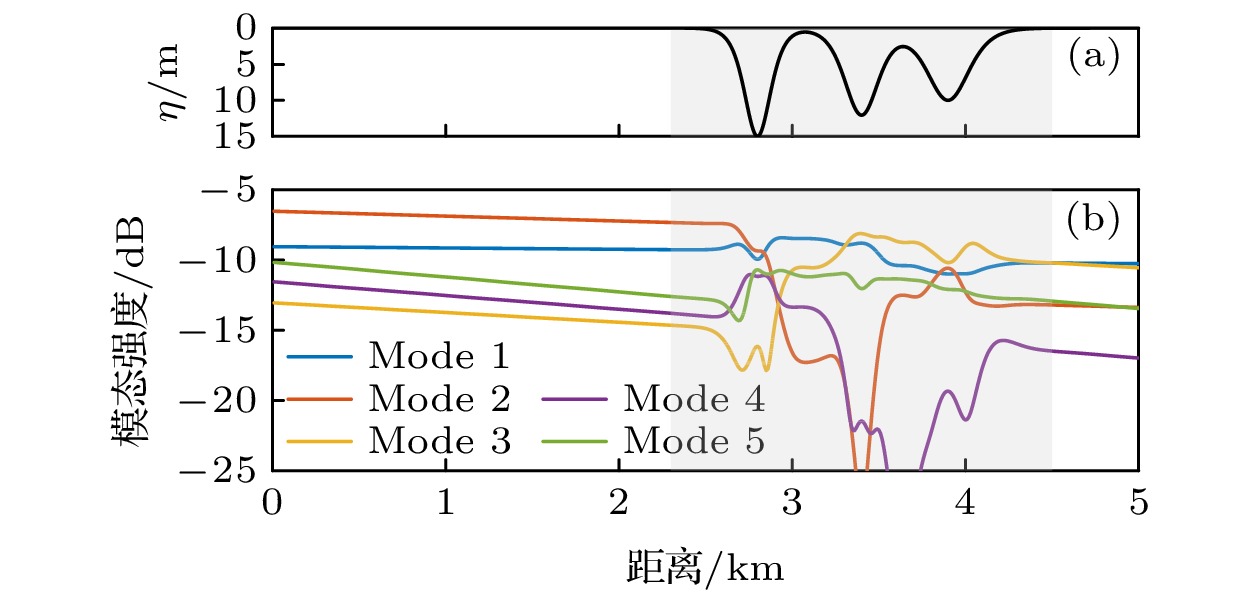
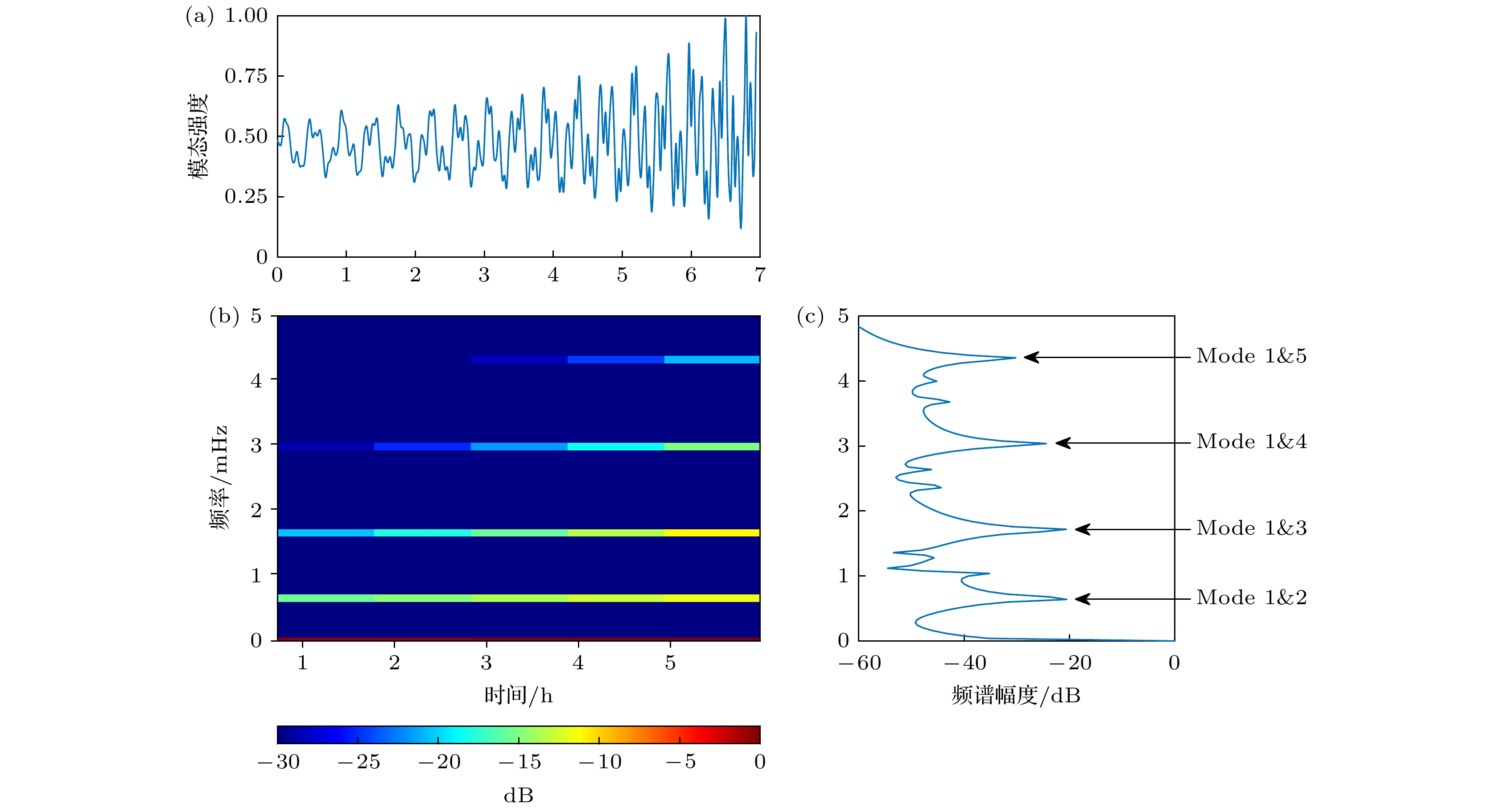
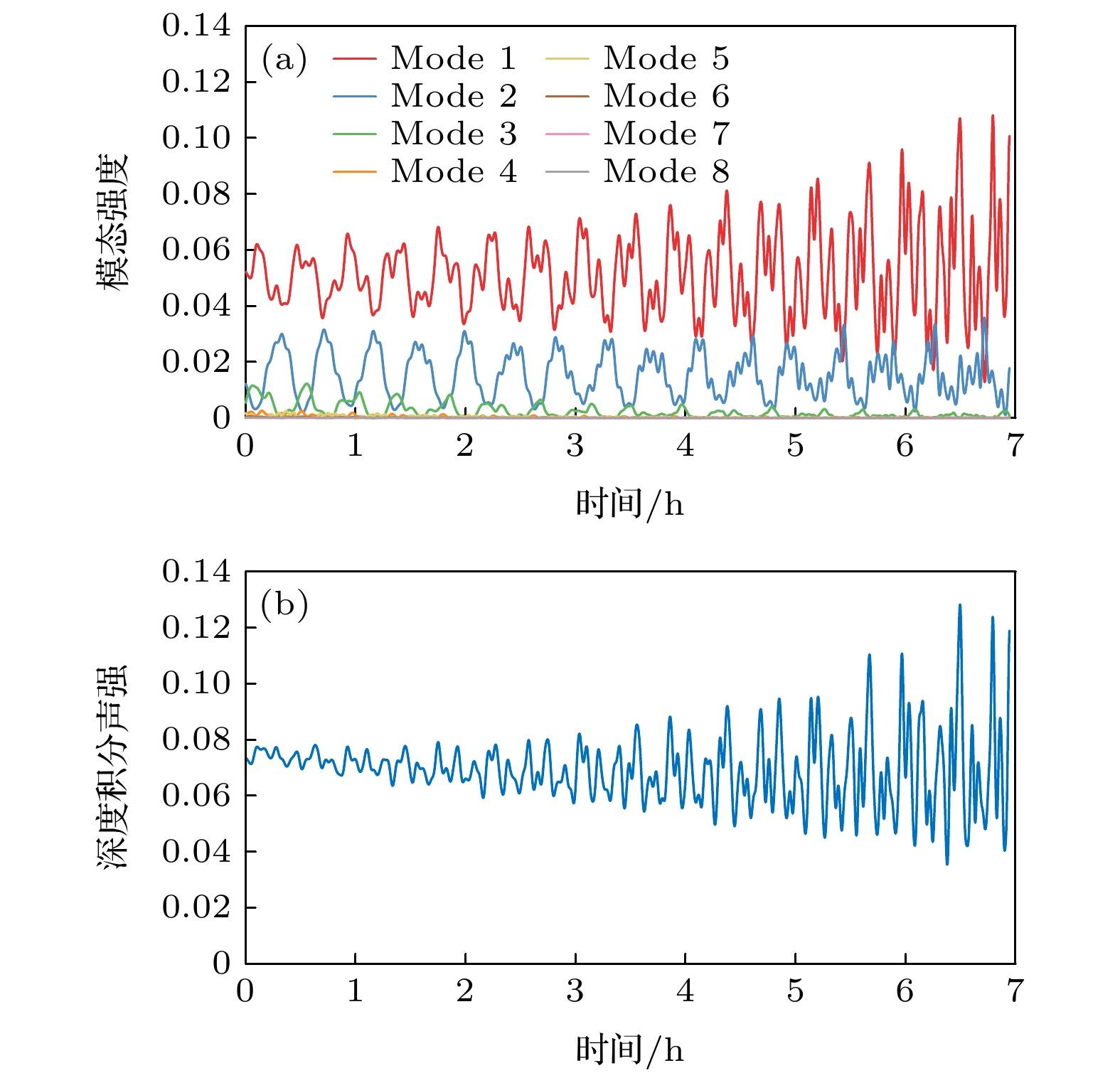
-
Internal solitary wave (ISW) is a kind of nonlinear internal wave commonly observed in the shallow water, which has the characteristics of large amplitude, short period and strong current velocity. With the distribution of the temperature and the salinity in the water column perturbed by ISWs, the sound speed profile becomes range-dependent, and thus affecting the characteristics of the underwater acoustic propagation. The ISWs usually propagate at a speed of the order of 1 m/s , and moving internal waves cause the energy in each acoustic mode to fluctuate dramatically. In this paper, the modal intensity is defined as the squared modulus of the modal coefficient, and is used to measure the sound energy in each mode. Based on the coupled mode theory, the expression of the acoustic modal intensity during the propagation of internal waves is derived in this paper, and the modal intensity is taken as the linear superposition of the oscillating term and the trend term. Most of previous researches were limited to the study of the time-varying characteristics of the acoustic modal intensity during the propagation of internal waves in the time domain or frequency domain. In this paper, the mechanism of modal intensity fluctuations is studied simultaneously in the time domain and the frequency domain with the aid of the short-time Fourier transform. Both the theoretical derivation and the numerical simulation show that the internal solitary wave gives rise to the energy transfer among acoustic modes, i.e., the mode coupling. The dynamic propagation of internal waves further leads to the modal interference, which behaves as an oscillating term in the modal intensity, and causes the modal intensity to fluctuate rapidly with time. The amplitude of the trend term changes with time due to the mode stripping (the difference in attenuation coefficients between different modes), which in turn adds a time-varying offset to the oscillations induced by the modal interference. The trend of the modal intensity and the time-varying characteristics of the amplitude of each frequency component in the oscillating term are closely associated with the modal attenuation. Meanwhile, the depth-integrated intensity is chosen as the measure of the total received acoustic intensity, and the influences of modal intensity fluctuations on the acoustic energy at the receivers during the propagation of internal waves are studied. It is demonstrated that the modal intensity with high energy which oscillates most dramatically will dominate the temporal variation of the received acoustic energy.
[1] 方欣华, 杜涛 2005 海洋内波基础和中国海内波 (青岛: 中国海洋大学出版社) 第109页
Fang X H, Du T 2005 Fundamentals of Oceanic Internal Waves and Internal Waves in the China Seas (Qingdao: China Ocean University Press) p109 (in Chinese)
[2] Magalhaes J M, da Silva J C B, Buijsman M C 2020 Sci. Rep. 10 1
Google Scholar
[3] Chiu C S, Ramp S R, Miller C W, Lynch J F, Duda T F, Tang T Y 2004 IEEE J. Oceanic Eng. 29 1249
Google Scholar
[4] Duda T F, Lynch J F, Newhall A E, Wu L X, Chiu C S 2004 IEEE J. Oceanic Eng. 29 1264
Google Scholar
[5] Oba R, Finette S 2002 J. Acoust. Soc. Am. 111 769
Google Scholar
[6] Duda T F 2006 J. Acoust. Soc. Am. 119 3717
Google Scholar
[7] Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Apel J, Badiey M, Chiu C S, Finette S, Orr M, and Pasewark B, Turgot A, Wolf S, Tielbuerger D 2000 J. Acoust. Soc. Am. 107 201
Google Scholar
[8] Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Apel J, Badiey M, Chiu C S, Finette S, Orr M, Pasewark B, Turgot A, Wolf S, Tielbuerger D 2000 J. Acoust. Soc. Am. 107 221
Google Scholar
[9] Zhou J X, Zhang X Z, Rogers P H 1991 J. Acoust. Soc. Am. 90 2042
Google Scholar
[10] Duda T F, Preisig J C 1999 IEEE J. Oceanic Eng. 24 16
Google Scholar
[11] Apel J R, Badiey M, Chiu C S, Finette S, Headrick R, Kemp J, Lynch J F, Newhall A, Orr M H, Pasewark B H, Tielbuerger D, Turgut A, von der Heydt K, Wolf S 1997 IEEE J. Oceanic Eng. 22 465
Google Scholar
[12] Rouseff D, Turgut A, Wolf S N, Finette S, Orr M H, Pasewark B H, Apel J R, Badiey M, Chiu C S, Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Tielbuerger D 2002 J. Acoust. Soc. Am. 111 1655
Google Scholar
[13] 刘进忠, 王宁, 高大治 2006 声学学报 31 322
Google Scholar
Liu J Z, Wang N, Gao D Z 2006 Acta Acustia 31 322
Google Scholar
[14] 胡涛, 王臻, 郭圣明, 马力 2020 哈尔滨工程大学学报 41 1518
Hu T, Wang Z, Guo S M, Ma L 2020 J. Harbin Eng. Univ. 41 1518
[15] Finette S, Orr M H, Turgut A, Apel J R, Badiey M, Chiu C S, Headrick R H, Kemp J N, Lynch J F, Newhall A E, von der Heydt K, Pasewark B, Wolf S N, Tielbuerger D 2000 J. Acoust. Soc. Am. 108 957
Google Scholar
[16] Apel J R 2003 J. Phys. Oceanogr. 33 2247
Google Scholar
[17] Yang T C 2014 J. Acoust. Soc. Am. 135 610
Google Scholar
[18] Dozier L B, Tappert F D 1978 J. Acoust. Soc. Am. 63 353
Google Scholar
[19] 秦继兴, Katsnelson Boris, 李整林, 张仁和, 骆文于 2016 声学学报 41 145
Qin J X, Boris K, Li Z L, Zhang R H, Luo W Y 2016 Acta Acustia 41 145
[20] 宋文华, 胡涛, 郭圣明, 马力 2014 63 194303
Google Scholar
Song W H, Hu T, Guo S M, Ma L 2014 Acta Phys. Sin. 63 194303
Google Scholar
[21] Song W H, Wang N, Gao D Z, Wang H Z, Hu T, Guo S M 2017 J. Acoust. Soc. Am. 142 1848
Google Scholar
[22] Evans R B 1983 J. Acoust. Soc. Am. 74 188
Google Scholar
[23] Sperry B J 1999 Ph. D. Dissertation (Woods Hole: Massachussetts Institute of Technology/Woods Hole Oceanographic Institution)
[24] Colosi J A 2016 Sound Propagation Through the Stochastic Ocean (Cambridge: Cambridge University Press) p83
[25] Buck J R, Preisig J C, Wage K E 1998 J. Acoust. Soc. Am. 103 1813
Google Scholar
[26] Katsnelson B, Petnikov V, Lynch J 2012 Fundamentals of Shallow Water Acoustics (Boston: Springer) pp83–85
[27] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) p85, 473
[28] Lee D, McDaniel S T 1988 Ocean Acoustic Propagation by Finite Difference Methods (Amsterdam: Elsevier) p386
[29] Porter M B 1992 The KRAKEN Normal Mode Program (Washington DC: Naval Research Laboratory)
-
图 1 声源与接收器的布放位置及内波运动情况
Figure 1. Locations of the source and receivers and the details of internal wave motion.
图 2 波导环境声速剖面 (a) 背景声速剖面; (b) 内波扰动后的声速剖面, 白色虚线标注了内波波形
Figure 2. Sound speed profile in the waveguide: (a) Background sound speed profile; (b) sound speed profile perturbed by internal waves, with the waveform of internal waves indicated by white dashed lines.
图 3 模态系数耦合矩阵的模值
$ |{\boldsymbol{R}}| $ (a) 无内波; (b) 有内波Figure 3. Modulus of the coupling matrix
$ |{\boldsymbol{R}}| $ : (a) Internal waves are not present in the waveguide; (b) internal waves are present in the waveguide.
图 4 无内波时模态强度随距离的变化 (a) 内波波形; (b) 模态强度变化
Figure 4. Modal intensity variation with range when internal waves are not present in the waveguide: (a) Waveform of internal waves; (b) modal intensity variation.
图 5 内波位于
$ r_{\rm{p}} = 3\; {\rm{km}} $ 时模态强度随距离的变化 (a) 内波波形; (b) 模态强度变化Figure 5. Modal intensity variation with range when internal waves are at
$ r_{\rm{p }}= 3\; {\rm{km}} $ : (a) Waveform of internal waves; (b) modal intensity variation.
图 6 内波位于
$ r_{\rm{p}} = 2.8\; {\rm{km}} $ 时模态强度随距离的变化 (a) 内波波形; (b) 模态强度变化Figure 6. Modal intensity variation with range when internal waves are at
$ r_{\rm{p}} = 2.8\; {\rm{km}} $ : (a) Waveform of internal waves; (b) modal intensity variation.
图 7 第 1 阶接收模态强度时频分析结果 (a) 时间序列; (b) 时频图; (c) 频谱
Figure 7. Time-frequency analysis results of the first received mode: (a) Time series; (b) time-frequency plot; (c) frequency spectrum.
图 8 第 3 阶接收模态强度时频分析结果 (a) 时间序列; (b) 时频图; (c) 频谱
Figure 8. Time-frequency analysis results of the third received mode: (a) Time series; (b) time-frequency plot; (c) frequency spectrum.
图 9 第1阶 (蓝色曲线) 和第3阶 (红色曲线) 接收模态强度时频图中0 Hz成分随时间的变化
Figure 9. Variation of the 0 Hz-component in the time-frequency plane of the first (blue line) and the third (red line) modal intensity.
图 10 各阶传播模态归一化的激励程度和衰减系数: (a) 模态激励; (b) 模态衰减
Figure 10. Normalized excitation and attenuation of each propagating mode: (a) Modal excitation; (b) modal attenuation.
图 11 (a) 第
$ l = 3 $ 阶接收模态强度中各直流分量的归一化时不变幅度$ B_{ln}|_{l = 3} $ ; (b) 第$ l = 3 $ 阶接收模态强度中归一化的直流成分Figure 11. (a) Normalized time-invariant amplitude of each DC component in the third modal intensity; (b) normalized DC part in the third modal intensity.
图 12 (a) 各阶传播模态强度随时间的变化; (b) 深度积分声强随时间的变化
Figure 12. (a) Temporal variations of modal intensities of propagating modes; (b) temporal variation of the depth-integrated intensity.
表 1 深度积分声强和各阶模态强度起伏的均值和标准差
Table 1. Mean values and the standard deviations of the depth-integrated intensity and the modal intensities.
声学量 均值 标准差 $ \bar I(T) \times{r_{\rm{e}}} $ 0.0694 0.0116 $|a_l(T)|^{2}_{l=1}\times{r_{\rm{e}}}$ 0.0514 0.0144 $|a_l(T)|^{2}_{l=2}\times{r_{\rm{e}}}$ 0.0151 0.0079 $|a_l(T)|^{2}_{l=3}\times{r_{\rm{e}}}$ 0.0024 0.0026 $|a_l(T)|^{2}_{l=4}\times{r_{\rm{e}}}$ 3.60 × 10–4 4.45 × 10–4 $|a_l(T)|^{2}_{l=5}\times{r_{\rm{e}}}$ 2.96 × 10–4 3.87 × 10–4 $|a_l(T)|^{2}_{l=6}\times{r_{\rm{e}}}$ 4.43 × 10–5 8.52 × 10–5 $ |a_l(T)|^{2}_{l=7}\times{r_{\rm{e}}} $ 4.30 × 10–6 1.22 × 10–5 $ |a_l(T)|^{2}_{l=8}\times{r_{\rm{e}}} $ 7.34 × 10–9 3.11 × 10–8 
DownLoad: CSV
-
[1] 方欣华, 杜涛 2005 海洋内波基础和中国海内波 (青岛: 中国海洋大学出版社) 第109页
Fang X H, Du T 2005 Fundamentals of Oceanic Internal Waves and Internal Waves in the China Seas (Qingdao: China Ocean University Press) p109 (in Chinese)
[2] Magalhaes J M, da Silva J C B, Buijsman M C 2020 Sci. Rep. 10 1
Google Scholar
[3] Chiu C S, Ramp S R, Miller C W, Lynch J F, Duda T F, Tang T Y 2004 IEEE J. Oceanic Eng. 29 1249
Google Scholar
[4] Duda T F, Lynch J F, Newhall A E, Wu L X, Chiu C S 2004 IEEE J. Oceanic Eng. 29 1264
Google Scholar
[5] Oba R, Finette S 2002 J. Acoust. Soc. Am. 111 769
Google Scholar
[6] Duda T F 2006 J. Acoust. Soc. Am. 119 3717
Google Scholar
[7] Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Apel J, Badiey M, Chiu C S, Finette S, Orr M, and Pasewark B, Turgot A, Wolf S, Tielbuerger D 2000 J. Acoust. Soc. Am. 107 201
Google Scholar
[8] Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Apel J, Badiey M, Chiu C S, Finette S, Orr M, Pasewark B, Turgot A, Wolf S, Tielbuerger D 2000 J. Acoust. Soc. Am. 107 221
Google Scholar
[9] Zhou J X, Zhang X Z, Rogers P H 1991 J. Acoust. Soc. Am. 90 2042
Google Scholar
[10] Duda T F, Preisig J C 1999 IEEE J. Oceanic Eng. 24 16
Google Scholar
[11] Apel J R, Badiey M, Chiu C S, Finette S, Headrick R, Kemp J, Lynch J F, Newhall A, Orr M H, Pasewark B H, Tielbuerger D, Turgut A, von der Heydt K, Wolf S 1997 IEEE J. Oceanic Eng. 22 465
Google Scholar
[12] Rouseff D, Turgut A, Wolf S N, Finette S, Orr M H, Pasewark B H, Apel J R, Badiey M, Chiu C S, Headrick R H, Lynch J F, Kemp J N, Newhall A E, von der Heydt K, Tielbuerger D 2002 J. Acoust. Soc. Am. 111 1655
Google Scholar
[13] 刘进忠, 王宁, 高大治 2006 声学学报 31 322
Google Scholar
Liu J Z, Wang N, Gao D Z 2006 Acta Acustia 31 322
Google Scholar
[14] 胡涛, 王臻, 郭圣明, 马力 2020 哈尔滨工程大学学报 41 1518
Hu T, Wang Z, Guo S M, Ma L 2020 J. Harbin Eng. Univ. 41 1518
[15] Finette S, Orr M H, Turgut A, Apel J R, Badiey M, Chiu C S, Headrick R H, Kemp J N, Lynch J F, Newhall A E, von der Heydt K, Pasewark B, Wolf S N, Tielbuerger D 2000 J. Acoust. Soc. Am. 108 957
Google Scholar
[16] Apel J R 2003 J. Phys. Oceanogr. 33 2247
Google Scholar
[17] Yang T C 2014 J. Acoust. Soc. Am. 135 610
Google Scholar
[18] Dozier L B, Tappert F D 1978 J. Acoust. Soc. Am. 63 353
Google Scholar
[19] 秦继兴, Katsnelson Boris, 李整林, 张仁和, 骆文于 2016 声学学报 41 145
Qin J X, Boris K, Li Z L, Zhang R H, Luo W Y 2016 Acta Acustia 41 145
[20] 宋文华, 胡涛, 郭圣明, 马力 2014 63 194303
Google Scholar
Song W H, Hu T, Guo S M, Ma L 2014 Acta Phys. Sin. 63 194303
Google Scholar
[21] Song W H, Wang N, Gao D Z, Wang H Z, Hu T, Guo S M 2017 J. Acoust. Soc. Am. 142 1848
Google Scholar
[22] Evans R B 1983 J. Acoust. Soc. Am. 74 188
Google Scholar
[23] Sperry B J 1999 Ph. D. Dissertation (Woods Hole: Massachussetts Institute of Technology/Woods Hole Oceanographic Institution)
[24] Colosi J A 2016 Sound Propagation Through the Stochastic Ocean (Cambridge: Cambridge University Press) p83
[25] Buck J R, Preisig J C, Wage K E 1998 J. Acoust. Soc. Am. 103 1813
Google Scholar
[26] Katsnelson B, Petnikov V, Lynch J 2012 Fundamentals of Shallow Water Acoustics (Boston: Springer) pp83–85
[27] Jensen F B, Kuperman W A, Porter M B, Schmidt H 2011 Computational Ocean Acoustics (New York: Springer) p85, 473
[28] Lee D, McDaniel S T 1988 Ocean Acoustic Propagation by Finite Difference Methods (Amsterdam: Elsevier) p386
[29] Porter M B 1992 The KRAKEN Normal Mode Program (Washington DC: Naval Research Laboratory)
DownLoad:
Catalog
Metrics
- Abstract views: 4905
- PDF Downloads: 99
- Cited By: 0
