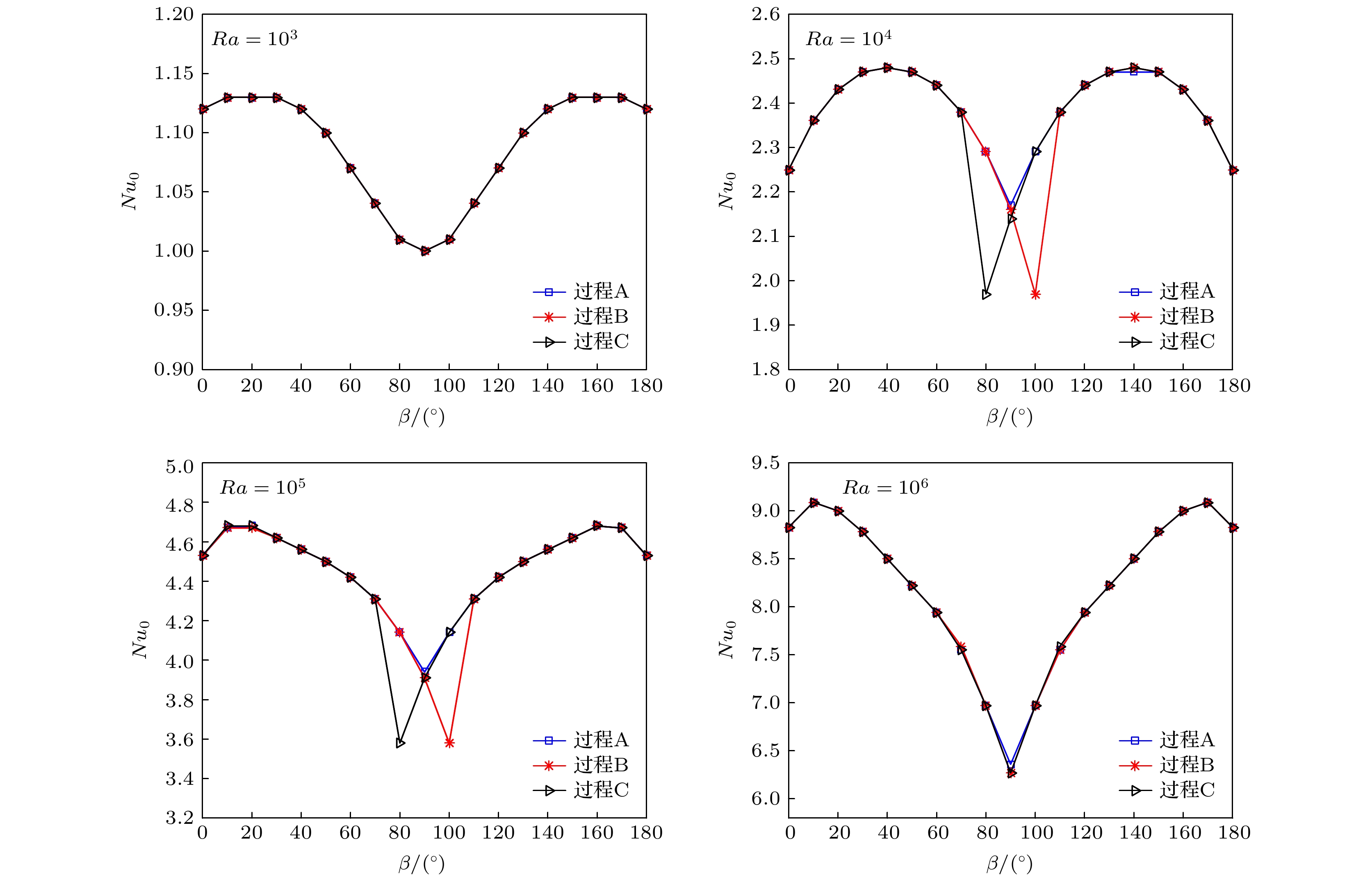
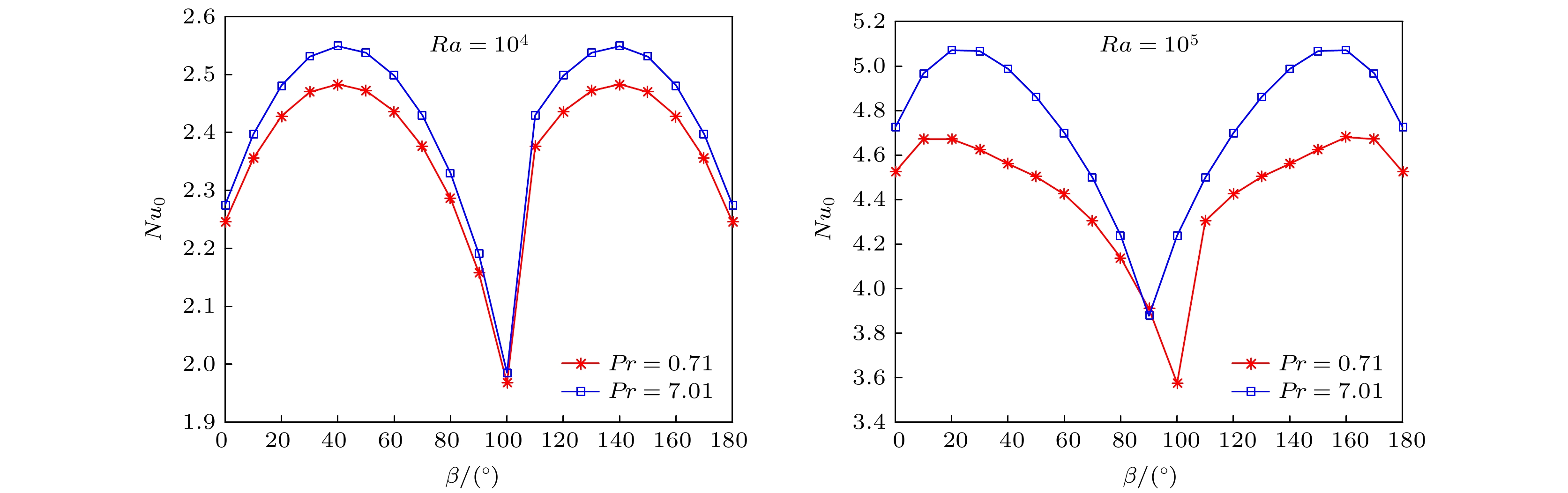
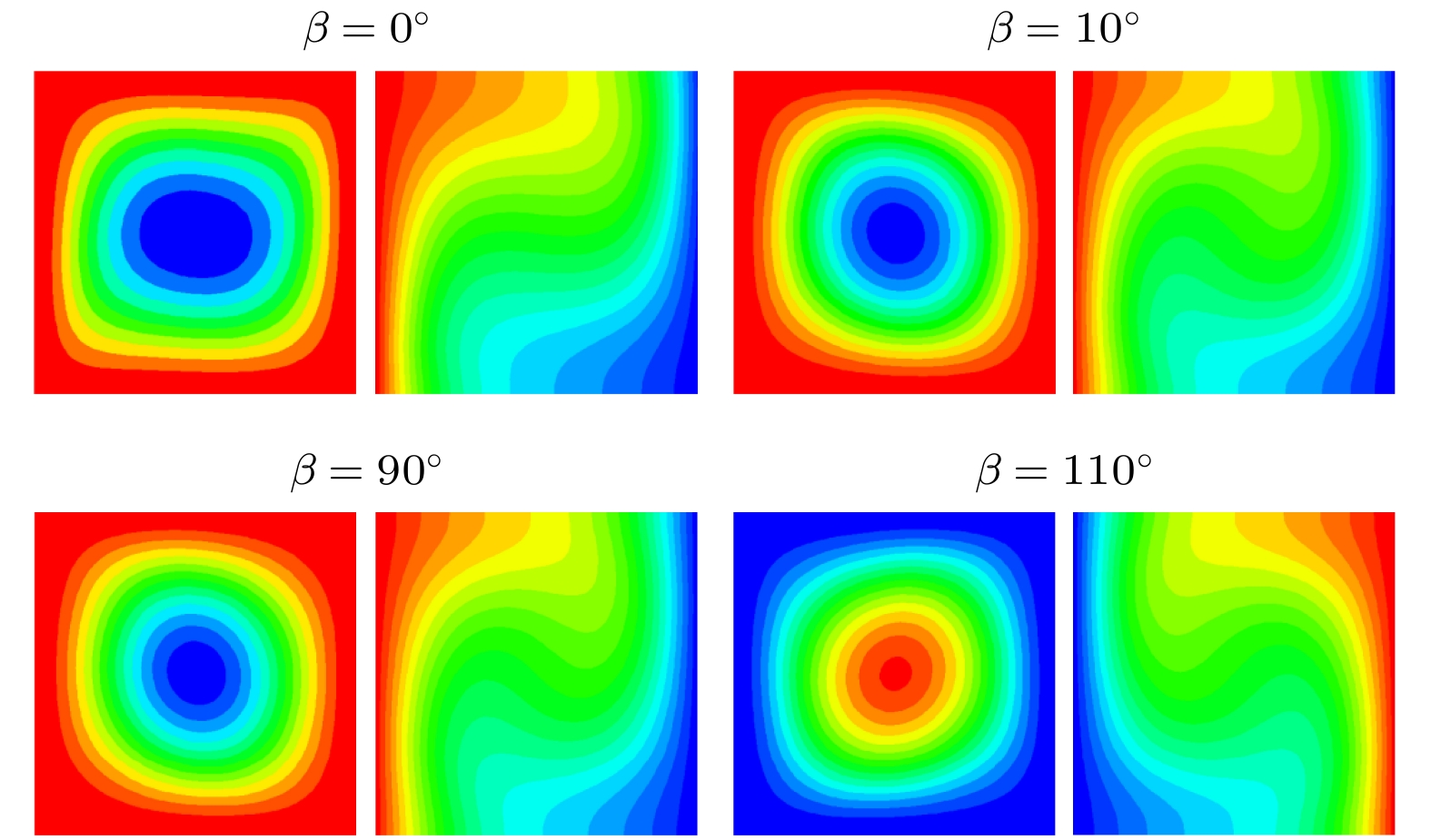
-
Heat transfer of natural convection in inclined cavities is one of the hot research topics in nonlinear non-equilibrium systems. In this paper, direct numerical simulations of natural convection in an inclined square cavity are carried out by using a high-accuracy numerical method. The effects of the different trends of inclination angle in a range of 0°–180° on the nonlinear evolution of flow field, heat transfer efficiency, and bifurcation are investigated. The Rayleigh number varies in a range from 103 to 106. The results show that the heat transfer efficiency characterized by Nusselt number is highly dependent on the Rayleigh number, Prandtl number, and the inclination angle. When the Rayleigh number is high, the Nusselt number will have a small jump near the inclination angle in a range of 80°–100°. The evolution of the flow field and temperature field are more complicated at high Rayleigh number. There are one to three vortices of different intensities in the cavity. At low Rayleigh number and inclination angle of the cavity being close to 90°, the flow state is composed mainly of heat conduction state. In addition, it is found that there exist two stable branches of solutions in a range of Rayleigh number (4949, 314721) when the inclination angle is in the interval of (70°, 110°).
-
Keywords:
- thermal convection /
- direct numerical simulation /
- bifurcation /
- inclination angle /
- high-accuracy
[1] Batchelor G K 1954 Q. Appl. Math. 12 209
Google Scholar
[2] Eckert E R G, Carlson W O 1961 Int. J. Heat Mass Transfer 2 106
Google Scholar
[3] Patterson J C, Armfield S W 1990 J. Fluid Mech. 219 469
Google Scholar
[4] Xin S, Quéré P L 1995 J. Fluid Mech. 304 87
Google Scholar
[5] Das D, Roy M, Basak T 2017 Int. J. Heat Mass Transfer 106 356
Google Scholar
[6] Arnold J N, Catton I, Edwards D K 1976 J. Heat Transfer 98 67
Google Scholar
[7] John P, Jorg I 1980 J. Fluid Mech. 100 65
Google Scholar
[8] Khezzar L, Siginer D, Vinogradov I 2012 Heat Mass Transfer 48 227
Google Scholar
[9] Dider S, Abdelmadjid B, François P 2012 Exp. Therm Fluid Sci. 38 74
Google Scholar
[10] Torres J F, Henry D, Komiya A, Maruyama S 2014 J. Fluid Mech. 756 650
Google Scholar
[11] Torres J F, Henry D, Komiya A, Maruyama S 2015 Phys. Rev. E 92 023031
Google Scholar
[12] Miroshnichenko I V, Sheremet M A 2018 Renewable Sustainable Energy Rev. 82 40
Google Scholar
[13] 徐丰, 崔会敏 2014 力学进展 44 201403
Xu F, Cui H M 2014 Adv. Mech. 44 201403
[14] Hamady F J, Lloyd J R, Yang H Q, Yang K T 1989 Int. J. Heat Mass Transfer 32 1697
Google Scholar
[15] Kuyper R A, Meer T H V D, Hoogendoorn C J 1994 Chem. Eng. Sci. 49 851
Google Scholar
[16] Rasoul J, Prinos P 1997 Int. J. Numer. Methods Heat Fluid Flow 7 438
Google Scholar
[17] Janssen R J A, Armfield S 1996 Int. J. Heat Fluid Flow 17 547
Google Scholar
[18] Varol Y, Oztop H F 2008 Build. Environ. 43 1535
Google Scholar
[19] Corcione M 2003 Int. J. Therm. Sci. 42 199
Google Scholar
[20] Wang H, Hamed M S 2006 Int. J. Therm. Sci. 45 782
Google Scholar
[21] Armfield S W, Janssen R 1996 Int. J. Heat Fluid Flow 17 539
Google Scholar
[22] Zhao B X, Tian Z F 2016 Int. J. Heat Mass Transfer 98 313
Google Scholar
[23] Sheremet M A, Pop I, Mahian O 2018 Int. J. Heat Mass Transfer 116 751
Google Scholar
[24] Boudjeniba B, Laouer A, Laouar S, Mezaache E H 2019 Int. J. Heat Technol. 37 413
Google Scholar
[25] Wang Q, Xia S N, Wang B F, Sun D J, Zhou Q, Wan Z H 2018 J. Fluid Mech. 849 355
Google Scholar
[26] Wang Q, Wan Z H, Yan R, Sun D J 2018 Phys. Rev. Fluids 3 113503
Google Scholar
[27] Wang Q, Chong K L, Stevens R J A M, Verzicco R, Lohse D 2020 J. Fluid Mech. 905 A21
Google Scholar
[28] Wang Q, Wan Z H, Yan R, Sun D J 2019 Phys. Fluids 31 025102
Google Scholar
[29] Wang Q, Verzicco R, Lohse D, Shishkina O 2020 Phys. Rev. Lett. 125 074501
Google Scholar
[30] Sugiyama K, Ni R, Stevens R J A M, Chan T S, Zhou S Q, Xi H D, Sun C, Grossmann S, Xia K Q, Lohse D 2010 Phys. Rev. Lett. 105 034503
Google Scholar
[31] Tian Z F, Liang X, Yu P X 2011 Int. J. Numer. Methods Eng. 88 511
Google Scholar
[32] Davis G D V 1983 Int. J. Numer. Methods Fluids 3 249
Google Scholar
[33] Kalita J C, Dalal D C, Dass A K 2001 Phys. Rev. E 64 066703
Google Scholar
[34] Tian Z F, Ge Y B 2003 Int. J. Numer. Methods Fluids 41 495
Google Scholar
[35] Yu P X, Tian Z F 2012 Phys. Rev. E 85 036703
Google Scholar
-
图 1 带边界条件的倾斜方腔示意图
Figure 1. Schematic diagram of the inclined square cavity with boundary conditions.
图 2 Nusselt数随Rayleigh数的变化(
$ \beta = 0^\circ $ )Figure 2. Variation of Nusselt number as a function of Rayleigh number (
$ \beta = 0^\circ $ ).
图 3 Nusselt数随Prandtl数的变化(
$ \beta = {\rm{0}}^\circ $ )Figure 3. Variation of Nusselt number as a function of Prandtl number (
$ \beta = {\rm{0}}^\circ $ ).
图 4 不同Rayleigh数下Nusselt数随倾斜角度的变化(
$ Pr = 0.71 $ )Figure 4. Variation of Nusselt number with inclination angle for different Rayleigh numbers (
$ Pr = 0.71 $ ).
图 5 不同Prandtl数下Nusselt数随倾斜角度的变化(以过程B为例)
Figure 5. Variation of Nusselt number with inclination angle for different Prandtl numbers (Process B).
图 6
$Ra=10^{3}$ 时流线与等温线图Figure 6. Streamlines and isotherms for
$Ra=10^{3}$ .
图 8
$ Ra = 10^{5} $ 时流线与等温线图Figure 8. Streamlines and isotherms for
$ Ra = 10^{5} $ .
图 7
$Ra=10^{4}$ 时流线与等温线图Figure 7. Streamlines and isotherms for
$Ra=10^{4}$ .
图 9
$ Ra = 10^{6} $ 时流场结构随倾角的变化Figure 9. Variation of flow field with inclination angle for
$ Ra = 10^{6} $ .
图 10 分岔点附近Nusselt数随倾角的变化 (a) Ra = 4949; (b) Ra = 4950
Figure 10. Variation of Nusselt number with inclination angle near the bifurcation point: (a) Ra = 4949; (b) Ra = 4950.
图 11 分岔区间上界附近Nusselt数随倾角的变化曲线 (a)
$Ra=314720 $ ; (b) Ra = 314721Figure 11. Variation of Nusselt number with inclination angle near the upper bound of bifurcation interval: (a)
$Ra=314720$ ; (b) Ra = 314721.
图 12
$ \beta = 80^\circ $ 时分岔点处过程B与过程C的对流斑图Figure 12. Flow field of process B and process C at the bifurcation point for
$ \beta = 80^\circ $ .表 1 与其他文献结果的对比(
$ Pr = 0.71 $ ,$ \beta = {\rm{0}}^\circ $ )Table 1. Comparison of the results by different numerical methods for
$ Pr = 0.71 $ and$ \beta = {\rm{0}}^\circ $ .文献 $ \left| \psi \right|_{\rm {max}} $ $ \left| {\psi _{\rm {mid}} } \right| $ $ Nu_{0} $ $ \overline{Nu} $ 文献 $ \left| \psi \right|_{\rm {max}} $ $ \left| {\psi _{\rm {mid}} } \right| $ $ Nu_{0} $ $ \overline{Nu} $ $ Ra=10^{5} $ $ Ra=10^{6} $ 本文 9.615 9.115 4.520 4.522 本文 16.807 16.383 8.815 8.827 [32] 9.612 9.111 4.509 4.519 [32] 16.750 16.320 8.817 8.800 [33] — 9.123 4.512 4.522 [33] — 16.420 8.763 8.829 [34] 9.6173 9.1161 4.5195 — [34] 16.8107 16.3863 8.8216 — [35] 9.6202 9.1194 4.5214 — [35] 16.8411 16.4183 8.8091 — 
DownLoad: CSV
表 2
$ Pr = 0.71 $ ,$ \beta = {\rm{0}}^\circ $ ,$ Ra = 10^{6} $ 下的网格检验结果Table 2. Grid test results for
$ Pr = 0.71 $ ,$ \beta = {\rm{0}}^\circ $ and$ Ra = 10^{6} $ .网格尺寸 $\left| \psi \right|_{\rm {max}}$ 误差/% $\left| {\psi _{\rm {mid}} } \right|$ 误差/% $ Nu_0 $ 误差/% $ 31\times31 $ 16.460 2.086 16.118 1.631 9.293 5.301 $ 61\times61 $ 16.830 0.119 16.410 0.148 8.798 0.315 $ 91\times91 $ 16.802 0.051 16.385 0.002 8.786 0.445 $ 121\times121 $ 16.807 0.017 16.383 0.014 8.815 0.119 $ 241\times241 $ 16.810 — 16.386 — 8.825 —
DownLoad: CSV
表 3
$ Pr = 0.71 $ ,$ \beta = {\rm{45}}^\circ $ ,$ Ra = 10^{6} $ 下的网格检验结果Table 3. Grid test results for
$ Pr = 0.71 $ ,$ \beta = {\rm{45}}^\circ $ and$ Ra = 10^{6} $ .网格尺寸 $\left| \psi \right|_{\rm {max}}$ 误差/% $\left| {\psi _{\rm {mid}} } \right|$ 误差/% $ Nu_0 $ 误差/% $ 31\times31 $ 32.400 3.276 27.974 3.306 9.077 9.345 $ 61\times61 $ 33.252 0.734 28.707 0.771 8.332 0.381 $ 91\times91 $ 33.438 0.176 28.874 0.195 8.301 0.001 $ 121\times121 $ 33.477 0.062 28.911 0.068 8.304 0.039 $ 241\times241 $ 33.498 — 28.931 — 8.301 —
DownLoad: CSV
表 4
$ Pr = 7.01 $ ,$ \beta = {\rm{0}}^\circ $ ,$ Ra = 10^{6} $ 下的网格检验结果Table 4. Grid test results for
$ Pr = 7.01 $ ,$ \beta = {\rm{0}}^\circ $ and$ Ra = 10^{6} $ .网格尺寸 $\left| \psi \right|_{\rm {max}}$ 误差/% $\left| {\psi _{\rm {mid}} } \right|$ 误差/% $ Nu_0 $ 误差/% $ 31\times31 $ 18.625 5.075 17.873 5.021 9.548 3.514 $ 61\times61 $ 19.634 0.067 18.838 0.110 9.195 0.310 $ 91\times91 $ 19.609 0.059 18.814 0.020 9.206 0.193 $ 121\times121 $ 19.612 0.044 18.812 0.029 9.221 0.037 $ 241\times241 $ 19.621 — 18.818 — 9.224 —
DownLoad: CSV
表 5
$ Pr = 7.01 $ ,$ \beta = 45^\circ $ ,$ Ra = 10^{6} $ 下的网格检验结果Table 5. The grid test results for
$ Pr = 7.01 $ ,$ \beta = {\rm{45}}^\circ $ and$ Ra = 10^{6} $ .网格尺寸 $\left| \psi \right|_{\rm {max}}$ 误差 $\left| {\psi _{\rm {mid}} } \right|$ 误差 $ Nu_0 $ 误差 $ {\rm{31}} \times {\rm{31}} $ 38.233 6.689% 34.649 6.739% 9.791 7.723% $ {\rm{61}} \times {\rm{61}} $ 40.665 0.752% 36.858 0.793% 9.114 0.271% $ {\rm{91}} \times {\rm{91}} $ 40.902 0.174% 37.090 0.167% 9.089 0.001% $ {\rm{121}} \times {\rm{121}} $ 40.950 0.057% 37.131 0.058% 9.092 0.025% $ {\rm{241}} \times {\rm{241}} $ 40.973 — 37.152 — 9.089 —
DownLoad: CSV
-
[1] Batchelor G K 1954 Q. Appl. Math. 12 209
Google Scholar
[2] Eckert E R G, Carlson W O 1961 Int. J. Heat Mass Transfer 2 106
Google Scholar
[3] Patterson J C, Armfield S W 1990 J. Fluid Mech. 219 469
Google Scholar
[4] Xin S, Quéré P L 1995 J. Fluid Mech. 304 87
Google Scholar
[5] Das D, Roy M, Basak T 2017 Int. J. Heat Mass Transfer 106 356
Google Scholar
[6] Arnold J N, Catton I, Edwards D K 1976 J. Heat Transfer 98 67
Google Scholar
[7] John P, Jorg I 1980 J. Fluid Mech. 100 65
Google Scholar
[8] Khezzar L, Siginer D, Vinogradov I 2012 Heat Mass Transfer 48 227
Google Scholar
[9] Dider S, Abdelmadjid B, François P 2012 Exp. Therm Fluid Sci. 38 74
Google Scholar
[10] Torres J F, Henry D, Komiya A, Maruyama S 2014 J. Fluid Mech. 756 650
Google Scholar
[11] Torres J F, Henry D, Komiya A, Maruyama S 2015 Phys. Rev. E 92 023031
Google Scholar
[12] Miroshnichenko I V, Sheremet M A 2018 Renewable Sustainable Energy Rev. 82 40
Google Scholar
[13] 徐丰, 崔会敏 2014 力学进展 44 201403
Xu F, Cui H M 2014 Adv. Mech. 44 201403
[14] Hamady F J, Lloyd J R, Yang H Q, Yang K T 1989 Int. J. Heat Mass Transfer 32 1697
Google Scholar
[15] Kuyper R A, Meer T H V D, Hoogendoorn C J 1994 Chem. Eng. Sci. 49 851
Google Scholar
[16] Rasoul J, Prinos P 1997 Int. J. Numer. Methods Heat Fluid Flow 7 438
Google Scholar
[17] Janssen R J A, Armfield S 1996 Int. J. Heat Fluid Flow 17 547
Google Scholar
[18] Varol Y, Oztop H F 2008 Build. Environ. 43 1535
Google Scholar
[19] Corcione M 2003 Int. J. Therm. Sci. 42 199
Google Scholar
[20] Wang H, Hamed M S 2006 Int. J. Therm. Sci. 45 782
Google Scholar
[21] Armfield S W, Janssen R 1996 Int. J. Heat Fluid Flow 17 539
Google Scholar
[22] Zhao B X, Tian Z F 2016 Int. J. Heat Mass Transfer 98 313
Google Scholar
[23] Sheremet M A, Pop I, Mahian O 2018 Int. J. Heat Mass Transfer 116 751
Google Scholar
[24] Boudjeniba B, Laouer A, Laouar S, Mezaache E H 2019 Int. J. Heat Technol. 37 413
Google Scholar
[25] Wang Q, Xia S N, Wang B F, Sun D J, Zhou Q, Wan Z H 2018 J. Fluid Mech. 849 355
Google Scholar
[26] Wang Q, Wan Z H, Yan R, Sun D J 2018 Phys. Rev. Fluids 3 113503
Google Scholar
[27] Wang Q, Chong K L, Stevens R J A M, Verzicco R, Lohse D 2020 J. Fluid Mech. 905 A21
Google Scholar
[28] Wang Q, Wan Z H, Yan R, Sun D J 2019 Phys. Fluids 31 025102
Google Scholar
[29] Wang Q, Verzicco R, Lohse D, Shishkina O 2020 Phys. Rev. Lett. 125 074501
Google Scholar
[30] Sugiyama K, Ni R, Stevens R J A M, Chan T S, Zhou S Q, Xi H D, Sun C, Grossmann S, Xia K Q, Lohse D 2010 Phys. Rev. Lett. 105 034503
Google Scholar
[31] Tian Z F, Liang X, Yu P X 2011 Int. J. Numer. Methods Eng. 88 511
Google Scholar
[32] Davis G D V 1983 Int. J. Numer. Methods Fluids 3 249
Google Scholar
[33] Kalita J C, Dalal D C, Dass A K 2001 Phys. Rev. E 64 066703
Google Scholar
[34] Tian Z F, Ge Y B 2003 Int. J. Numer. Methods Fluids 41 495
Google Scholar
[35] Yu P X, Tian Z F 2012 Phys. Rev. E 85 036703
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4427
- PDF Downloads: 51
- Cited By: 0
