Non-contrast-enhanced ultrafast ultrasound Doppler imaging of spinal cord micro-vessels

Zang Jia-Qi; Xu Kai-Liang; Han Qing-Jian; Lu Qi-Yong; Mei Yong-Feng; Ta De-An

doi:10.7498/aps.70.20201878

Abstract

With the advantages of non-radiation and low-cost, ultrasound imaging has been widely used in clinical diagnosis. However, due to the compromise between temporal and spatial resolution, the traditional ultrasound imaging method which collects images line by line fails to obtain the images at a high frame rate. Ultrafast ultrasound imaging method based on the plane-wave emission can achieve a high frame rate with the capability for instantaneous hemodynamic imaging of neurovascular response. Besides, by the coherent compounding of the echo signals received from emitting a set of tilting plane-waves, the image quality can be improved in terms of resolution, contrast and signal-to-noise ratio (SNR). Considering the fact that received signals are the mixture of echo signals from the low-speed soft tissue and high-speed blood flow, a clutter filtering method is used to remove the signals of soft tissue. In this study, the ultrafast ultrasound sequence of emission and reception is designed to image the spinal cord by using the groups of multiple steering-angle plane-waves. The so-called f-k migration algorithm based on an exploding reflector model (ERM) is used for coherent plane-wave compounding. Eigenvalue decomposition (EVD) is then applied to tissue and blood signal extraction. The static tissue signals correspond to eigenvectors with low Doppler frequency shift and large magnitude eigenvalue while the high-speed blood flow signals correspond to eigenvectors with high Doppler frequency shift and small eigenvalue. Therefore, frequency and amplitude thresholds can be applied to the accurate separation of the blood component and the tissue component. After the signal extraction, the hemodynamic imaging of blood vessels can thus be obtained from the power Doppler results. The experiments are carried out by using a programmable ultrasonic array system and a high-frequency linear array transducer L22-14vX with a central frequency of 15.625 MHz. The sample acquisition frequency is set to be 62.5 MHz. In rat experiments in vivo, 14040 angle-stilting images per second are compounded into 520 images, each of which is compounded from 27 tilting images (the tilting angles ranging from –10° to 10°). The experiments are conducted on the anesthetized rats with part of the vertebral plate removed. The ultrafast B-mode images are acquired from a 14.0 mm ×12.7 mm region-of-interest. The 520 frames acquired in one second are processed by the clutter filter based on eigenvalue decomposition and Doppler shift analysis. The eigenvectors and eigenvalues corresponding to the soft tissue are discarded. The power Doppler images of the spinal cord micro-vessels are obtained. The experimental results indicate that the ultrafast ultrasound Doppler imaging method is effective for monitoring the hemodynamic variation in spinal cord. The micro-hemorrhage can be identified from the power Doppler images. The quantitative results indicate that the SNR increases with the steering angles increasing. Compared with the results obtained by using 9 steering angles, 5 dB SNR enhancement can be obtained by using the 27 steering angles. In conclusion, the ultrafast ultrasound Doppler technology has the potential applications in spinal cord microvascular imaging and hemodynamic evaluation of neurovascular function of spinal cord.

Keywords:

Authors and contacts

1.
Center for Biomedical Engineering, School of Information Science and Technology, Fudan University, Shanghai 200433, China
2.
Institutes of Brain Science, Fudan University, Shanghai 200433, China
3.
Department of Materials Science, Fudan University, Shanghai 200433, China
4.
Shanghai Key Laboratory of Medical Imaging Computing and Computer Assisted Intervention, Shanghai 200433, China

Corresponding author: Xu Kai-Liang, xukl@fudan.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 11974081, 11827808, 51961145108), the Natural Science Foundation of Shanghai, China (Grant No. 19ZR1402700), the Shanghai Rising Star Program, China (Grant No. 20QC1400200), and the Shanghai Academic Research Leader Program, China (Grant No. 19XD1400500)

FullText HTML : translate this paragraph

References

[1]	Ducker T B, Assenmacher D R 1951 Surg. Forum 3 428 Google Scholar
[2]	Guha A, Tator C H, Rochon J 1989 Stroke 20 372 Google Scholar
[3]	Mautes A E M, Weinzierl M R, Donovan F, Noble L J 2000 Phys. Ther. 80 673 Google Scholar
[4]	Pickett G E, Campos-Benitez M, Keller J L, Duggal N 2006 Spine (Phila Pa 1976) 31 799 Google Scholar
[5]	Song P, Cuellar C A, Tang S, Islam R, Wen H, Huang C, Manduca A, Trzasko J D, Knudsen B E, Lee K H, Chen S, Lavrov I A 2019 Front. Neurol. 10 279 Google Scholar
[6]	Bruce M, Hannah A, Hammond R, Khaing Z Z, Tremblay-Darveau C, Burns P N, Hofstetter C P 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. 67 1776 Google Scholar
[7]	Khaing Z Z, Cates L N, DeWees D M, Hannah A, Mourad P, Bruce M, Hofstetter C P 2018 J. Neurosurg. Spine 29 306 Google Scholar
[8]	Kornblum H I, Araujo D M, Annala A J, Tatsukawa K J, Phelps M E, Cherry S R 2000 Nat. Biotechnol. 18 655 Google Scholar
[9]	Phelps M E 1981 Semin. Nucl. Med. 11 32 Google Scholar
[10]	Ogawa S, Lee T M, Kay A R, Tank D W 1990 Proc. Natl. Acad. Sci. U. S. A. 87 9868 Google Scholar
[11]	Voorneveld J, Muralidharan A, Hope T, Vos H J, Kruizinga P, van der Steen A F W, Gijsen F J H, Kenjeres S, de Jong N, Bosch J G 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. 65 2222 Google Scholar
[12]	Tremblay-Darveau C, Sheeran P S, Vu C K, Williams R, Bruce M, Burns P N 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. 65 2286 Google Scholar
[13]	Starosolski Z, Villamizar C A, Rendon D, Paldino M J, Milewicz D M, Ghaghada K B, Annapragada A V 2015 Sci. Rep. 5 10178 Google Scholar
[14]	Osmanski B F, Pezet S, Ricobaraza A, Lenkei Z, Tanter M 2014 Nat. Commun. 5 5023 Google Scholar
[15]	Xia J, Yang Y, Hu C, Meng R, Jiang Q, Liu R, Yu Y, Sheng Z, Yan F, Zhang L, Shi Z, Zheng H, Qiu W 2019 Ultrasound Med. Biol. 45 811 Google Scholar
[16]	Deffieux T, Demene C, Pernot M, Tanter M 2018 Curr. Opin. Neurobiol. 50 128 Google Scholar
[17]	Correia M, Provost J, Tanter M, Pernot M 2016 Phys. Med. Biol. 61 48 Google Scholar
[18]	Provost J, Papadacci C, Arango J E, Imbault M, Fink M, Gennisson J L, Tanter M, Pernot M 2014 Phys. Med. Biol. 59 1 Google Scholar
[19]	Tanter M, Bercoff J, Sandrin L, Fink M 2002 IEEE Trans. Ultrason. Ferroelectr. Freq. 49 1363 Google Scholar
[20]	Montaldo G, Tanter M, Bercoff J, Benech N, Fink M 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. 56 489 Google Scholar
[21]	Mace E, Montaldo G, Cohen I, Baulac M, Fink M, Tanter M 2011 Nat. Methods 8 662 Google Scholar
[22]	Mace E, Montaldo G, Osmanski B F, Cohen I, Fink M, Tanter M 2013 IEEE Trans. Ultrason. Ferroelectr. Freq. 60 492 Google Scholar
[23]	Demene C, Baranger J, Bernal M, Delanoe C, Auvin S, Biran V, Alison M, Mairesse J, Harribaud E, Pernot M, Tanter M, Baud O 2017 Sci. Transl. Med. 9 6756 Google Scholar
[24]	Soloukey S, Harhangi B S, Generowicz B S, Slenter J P H, De Zeeuw C I, Kruizinga P, Koekkoek S K E 2019 IEEE International Ultrasonics Symposium Glasgow England Oct. 06–09, 2019 2259
[25]	Garcia D, Le Tarnec L, Muth S, Montagnon E, Poree J, Cloutier G 2013 IEEE Trans. Ultrason. Ferroelectr. Freq. 60 1853 Google Scholar
[26]	Gazdag J, Sguazzero P 1984 Proc. IEEE 72 1302 Google Scholar
[27]	Demene C, Deffieux T, Pernot M, Osmanski B F, Biran V, Gennisson J L, Sieu L A, Bergel A, Franqui S, Correas J M 2015 IEEE Trans. Med. Imaging 34 2271 Google Scholar
[28]	Yu A C H, Lovstakken L 2010 IEEE Trans. Ultrason. Ferroelectr. Freq. 57 1096 Google Scholar
[29]	Mazensky D, Flesarova S, Sulla I 2017 Anat. Rec. 300 2091 Google Scholar
[30]	Kang J, Go D, Song I, Yoo Y 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. in press
[31]	Jiang C, Li Y, Xu K, Ta D 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. 68 72 Google Scholar
[32]	Guasch L, Calderon Agudo O, Tang M X, Nachev P, Warner M 2020 NPJ Digital Medicine 3 28 Google Scholar

Cited By

图 1 方法流程示意

Figure 1. Flow chart of the proposed method.

DownLoad: Full-Size Img PowerPoint

图 2 超快超声成像序列示意图

Figure 2. Schematic diagram of ultrafast ultrasound imaging sequence.

DownLoad: Full-Size Img PowerPoint

图 3 超声波传播模型　(a) 超声波发射与回波接收模型; (b) ERM模型

Figure 3. Ultrasonic propagation model: (a) Ultrasonic transmitting and echo receiving model; (b) exploding reflector model (ERM).

DownLoad: Full-Size Img PowerPoint

图 4 大鼠脊髓血流超声成像实验装置示意

Figure 4. Schematic diagram of experimental ultrasonic imaging set-up for blood flow of rat spinal cord.

DownLoad: Full-Size Img PowerPoint

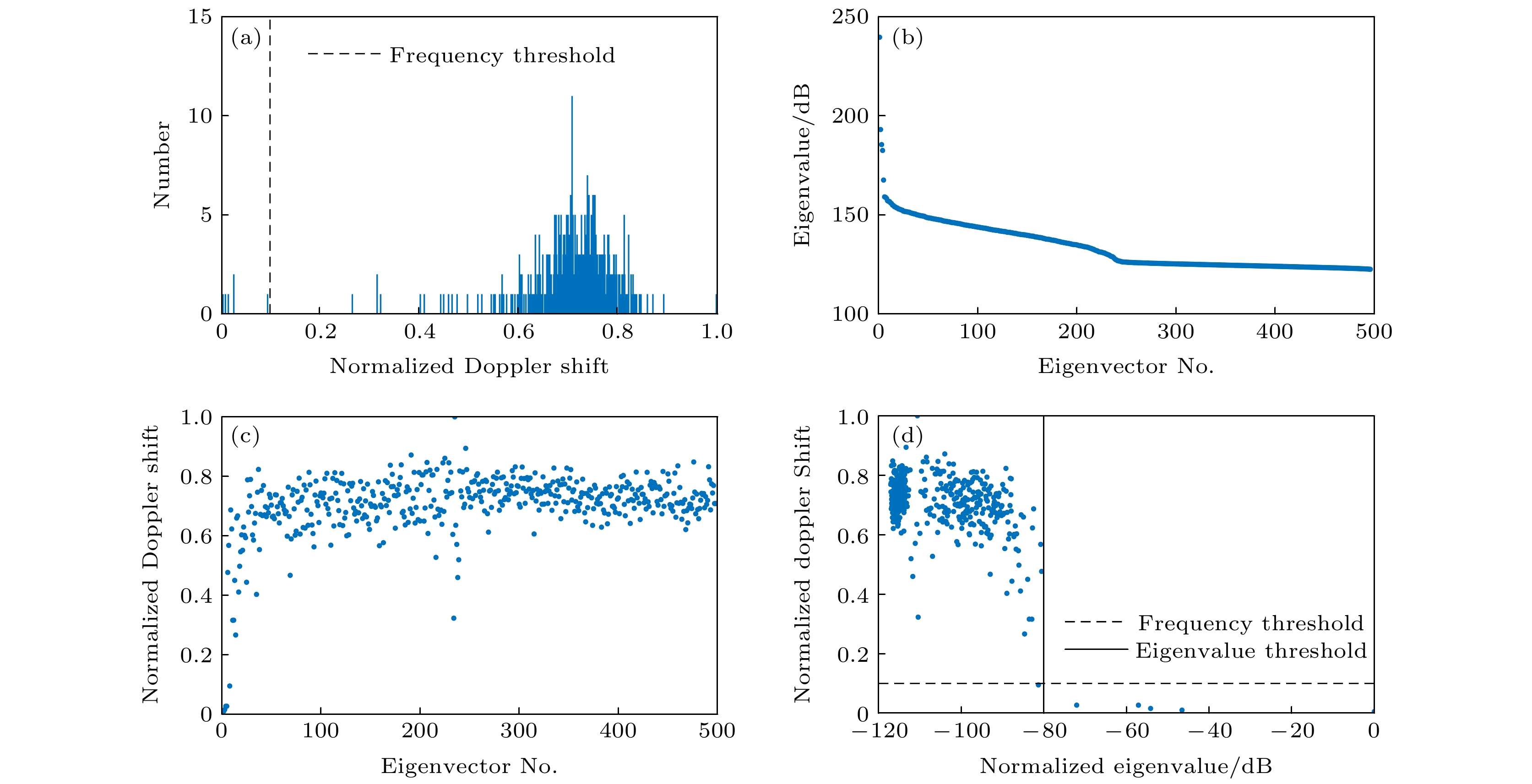
图 5 特征值分解与多普勒频移分析结果　(a) 归一化多普勒频移对应特征向量个数的直方图; (b)特征向量的特征值; (c) 特征向量的归一化多普勒频移; (d) 特征值对应的归一化多普勒频移

Figure 5. Eigenvalue decomposition and Doppler shift analysis results: (a) Histogram of the number of eigenvectors corresponding to normalized Doppler shifts; (b) eigenvalues of eigenvectors; (c) normalized Doppler shifts of eigenvectors; (d) eigenvalues versus normalized Doppler shifts.

DownLoad: Full-Size Img PowerPoint

图 6 仿体血流成像结果(第400帧)　(a) 滤波前的成像结果; (b) 滤波后的血流成像结果; (c) 滤波后的软组织成像结果; (d) 功率多普勒成像结果

Figure 6. Imaging results of the 400th frame of the phantom blood flow: (a) Original image before clutter filtering; (b) blood flow image after clutter filtering; (c) soft tissue image after clutter filtering; (d) power Doppler imaging result.

DownLoad: Full-Size Img PowerPoint

图 7 特征值分解与多普勒频移分析结果　(a) 归一化多普勒频移对应特征向量个数的直方图; (b)特征向量的特征值; (c) 特征向量的归一化多普勒频移; (d) 特征值对应的归一化多普勒频移

Figure 7. Eigenvalue decomposition and Doppler shift analysis results: (a) Histogram of the number of eigenvectors corresponding to normalized Doppler shifts; (b) eigenvalues of eigenvectors; (c) normalized Doppler shifts of eigenvectors; (d) eigenvalues versus normalized Doppler shifts.

DownLoad: Full-Size Img PowerPoint

图 8 基于多角度平面波复合成像的大鼠脊髓血流成像结果　(a) 单角度平面波发射成像; (b) 多角度平面波复合成像; (c) 杂波滤除结果. 每一次发射超声平面波的时间间隔为71.225 μs, 27次发射倾斜平面波与接收反射回波的总时长为1.923 ms, 对多角度信号经相干复合可获得单帧超声图像, 其所对应的成像帧率为每秒520帧

Figure 8. Blood flow imaging results of rat spinal cord based on multi-angle compounding method: (a) Beamforming results after a single emission; (b) multi-angle compounding images; (c) images after clutter filtering. The time interval between each emission is 71.225 μs. Each compounded frame is obtained using 27 steering-angle plane-waves within a period of 1.923 ms. Consequently the frame rate is 520 frames per second.

DownLoad: Full-Size Img PowerPoint

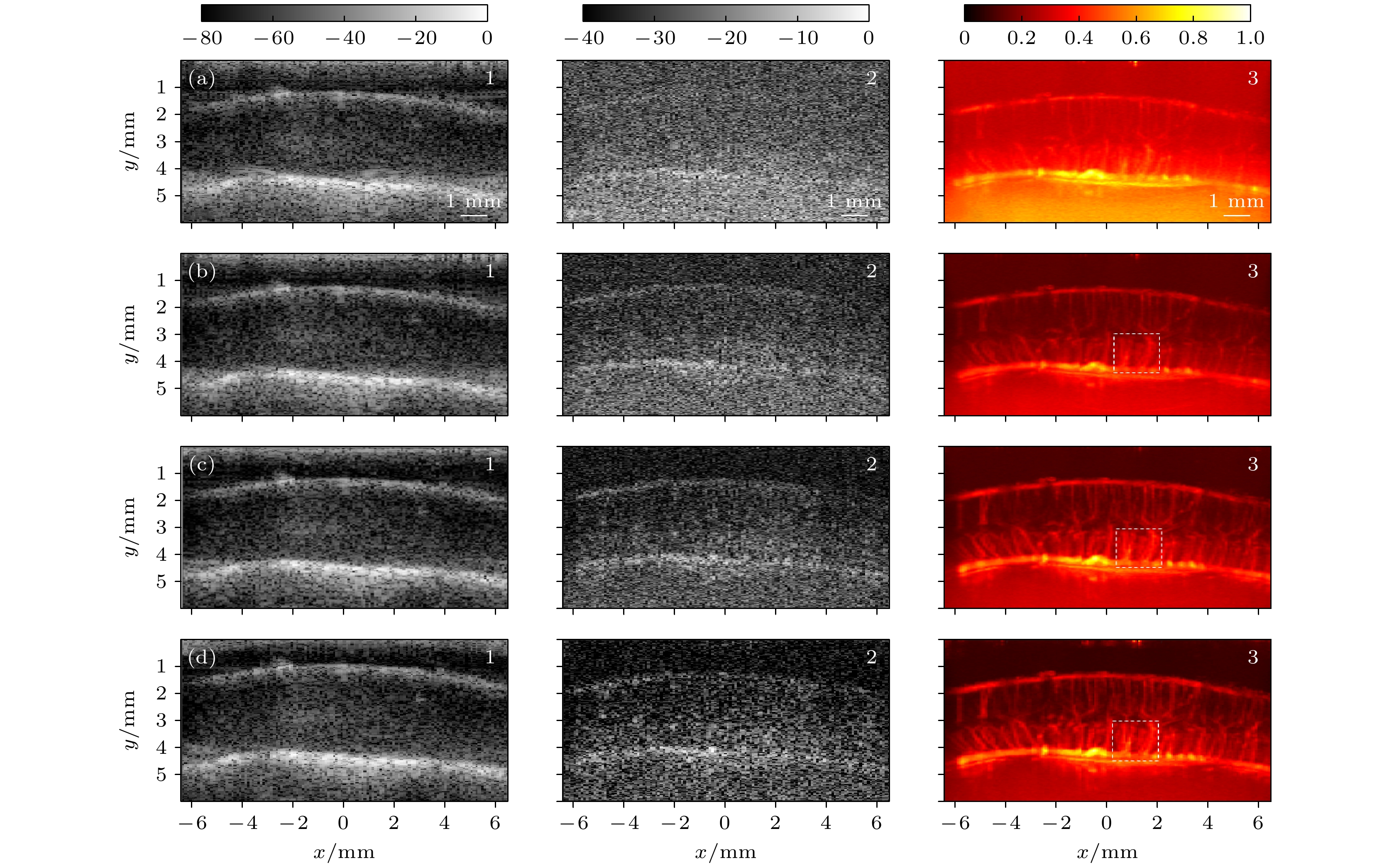
图 9 不同角度复合平面波成像结果对比图(复合帧频均为每秒520帧)　(a) 3个角度[–1°—1°]倾斜平面波复合成像结果; (b) 9个角度[–3°—3°]倾斜平面波复合成像结果; (c) 17个角度[–7°—7°]倾斜平面波复合成像结果; (d) 27个角度[–10°—10°]倾斜平面波复合成像结果. 1为单帧原始B模式图像, 2为杂波滤除之后的成像结果, 其中可见微血流变化, 3为1 s内采集数据得到的功率多普勒血流图(1, 2色标单位为dB, 3为归一化数值的多普勒成像结果)

Figure 9. Comparison of compounded images with different numbers of steering angles (composite frame rate is 520 frames per second): (a) Images compounded of data from emitting 3 [–1°—1°] steering plane-waves; (b) images compounded of data from emitting 9 [–3°—3°] steering plane-waves; (c) images compounded of data from emitting 17 [–7°—7°] steering plane-waves; (d) images compounded of data from emitting 27 [–10°—10°] steering plane-waves. Images labeled 1 are original B-mode images; images labeled 2 are imaging results after clutter filtering in which changes of blood flow can be observed; images labeled 3 are power Doppler images of micro-vessels (data was obtained within 1 s).

DownLoad: Full-Size Img PowerPoint

图 10 对比分辨率随复合平面波角度数增加的变化情况　(a) 当角度数N = 9, 17, 27时, 图9(b)-(d)编号3的图中矩形虚线框中图块的放大结果; (b) 当角度数N = 9, 17, 27时, 图10(a)中虚线深度处的幅值曲线图

Figure 10. Change of contrast resolution with the increase of the number of steering angles: (a) Enlarged image blocks in the rectangular dashed box in No.3 figure in Fig.9 (b)-(d) (angle numbers are 9, 17, 27, respectively); (b) amplitude curve of the dotted line position in Fig.10(a) (angle numbers are 9, 17, 27, respectively).

DownLoad: Full-Size Img PowerPoint

图 11 SNR随复合平面波角度数增加的变化结果

Figure 11. SNR versus number of steering angles.

DownLoad: Full-Size Img PowerPoint

map

[1]	Ducker T B, Assenmacher D R 1951 Surg. Forum 3 428 Google Scholar
[2]	Guha A, Tator C H, Rochon J 1989 Stroke 20 372 Google Scholar
[3]	Mautes A E M, Weinzierl M R, Donovan F, Noble L J 2000 Phys. Ther. 80 673 Google Scholar
[4]	Pickett G E, Campos-Benitez M, Keller J L, Duggal N 2006 Spine (Phila Pa 1976) 31 799 Google Scholar
[5]	Song P, Cuellar C A, Tang S, Islam R, Wen H, Huang C, Manduca A, Trzasko J D, Knudsen B E, Lee K H, Chen S, Lavrov I A 2019 Front. Neurol. 10 279 Google Scholar
[6]	Bruce M, Hannah A, Hammond R, Khaing Z Z, Tremblay-Darveau C, Burns P N, Hofstetter C P 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. 67 1776 Google Scholar
[7]	Khaing Z Z, Cates L N, DeWees D M, Hannah A, Mourad P, Bruce M, Hofstetter C P 2018 J. Neurosurg. Spine 29 306 Google Scholar
[8]	Kornblum H I, Araujo D M, Annala A J, Tatsukawa K J, Phelps M E, Cherry S R 2000 Nat. Biotechnol. 18 655 Google Scholar
[9]	Phelps M E 1981 Semin. Nucl. Med. 11 32 Google Scholar
[10]	Ogawa S, Lee T M, Kay A R, Tank D W 1990 Proc. Natl. Acad. Sci. U. S. A. 87 9868 Google Scholar
[11]	Voorneveld J, Muralidharan A, Hope T, Vos H J, Kruizinga P, van der Steen A F W, Gijsen F J H, Kenjeres S, de Jong N, Bosch J G 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. 65 2222 Google Scholar
[12]	Tremblay-Darveau C, Sheeran P S, Vu C K, Williams R, Bruce M, Burns P N 2018 IEEE Trans. Ultrason. Ferroelectr. Freq. 65 2286 Google Scholar
[13]	Starosolski Z, Villamizar C A, Rendon D, Paldino M J, Milewicz D M, Ghaghada K B, Annapragada A V 2015 Sci. Rep. 5 10178 Google Scholar
[14]	Osmanski B F, Pezet S, Ricobaraza A, Lenkei Z, Tanter M 2014 Nat. Commun. 5 5023 Google Scholar
[15]	Xia J, Yang Y, Hu C, Meng R, Jiang Q, Liu R, Yu Y, Sheng Z, Yan F, Zhang L, Shi Z, Zheng H, Qiu W 2019 Ultrasound Med. Biol. 45 811 Google Scholar
[16]	Deffieux T, Demene C, Pernot M, Tanter M 2018 Curr. Opin. Neurobiol. 50 128 Google Scholar
[17]	Correia M, Provost J, Tanter M, Pernot M 2016 Phys. Med. Biol. 61 48 Google Scholar
[18]	Provost J, Papadacci C, Arango J E, Imbault M, Fink M, Gennisson J L, Tanter M, Pernot M 2014 Phys. Med. Biol. 59 1 Google Scholar
[19]	Tanter M, Bercoff J, Sandrin L, Fink M 2002 IEEE Trans. Ultrason. Ferroelectr. Freq. 49 1363 Google Scholar
[20]	Montaldo G, Tanter M, Bercoff J, Benech N, Fink M 2009 IEEE Trans. Ultrason. Ferroelectr. Freq. 56 489 Google Scholar
[21]	Mace E, Montaldo G, Cohen I, Baulac M, Fink M, Tanter M 2011 Nat. Methods 8 662 Google Scholar
[22]	Mace E, Montaldo G, Osmanski B F, Cohen I, Fink M, Tanter M 2013 IEEE Trans. Ultrason. Ferroelectr. Freq. 60 492 Google Scholar
[23]	Demene C, Baranger J, Bernal M, Delanoe C, Auvin S, Biran V, Alison M, Mairesse J, Harribaud E, Pernot M, Tanter M, Baud O 2017 Sci. Transl. Med. 9 6756 Google Scholar
[24]	Soloukey S, Harhangi B S, Generowicz B S, Slenter J P H, De Zeeuw C I, Kruizinga P, Koekkoek S K E 2019 IEEE International Ultrasonics Symposium Glasgow England Oct. 06–09, 2019 2259
[25]	Garcia D, Le Tarnec L, Muth S, Montagnon E, Poree J, Cloutier G 2013 IEEE Trans. Ultrason. Ferroelectr. Freq. 60 1853 Google Scholar
[26]	Gazdag J, Sguazzero P 1984 Proc. IEEE 72 1302 Google Scholar
[27]	Demene C, Deffieux T, Pernot M, Osmanski B F, Biran V, Gennisson J L, Sieu L A, Bergel A, Franqui S, Correas J M 2015 IEEE Trans. Med. Imaging 34 2271 Google Scholar
[28]	Yu A C H, Lovstakken L 2010 IEEE Trans. Ultrason. Ferroelectr. Freq. 57 1096 Google Scholar
[29]	Mazensky D, Flesarova S, Sulla I 2017 Anat. Rec. 300 2091 Google Scholar
[30]	Kang J, Go D, Song I, Yoo Y 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. in press
[31]	Jiang C, Li Y, Xu K, Ta D 2020 IEEE Trans. Ultrason. Ferroelectr. Freq. 68 72 Google Scholar
[32]	Guasch L, Calderon Agudo O, Tang M X, Nachev P, Warner M 2020 NPJ Digital Medicine 3 28 Google Scholar

[1]	Fu Ya-Peng, Sun Qian-Dong, Li Bo-Yi, Ta De-An, Xu Kai-Liang. Three-dimensional ultrafast ultrasound imaging of blood flow using row-column addressing array: A simulation study. Acta Physica Sinica, 2023, 72(7): 074302. doi: 10.7498/aps.72.20222106
[2]	Wang Kang-Yu, Zhou Yu-Lin, He Li-Yuan, Lu Chun-Yao, Yu Run, Wu Da-Wei. Blood flow image by multi-angle composite ultrasonic Doppler vector. Acta Physica Sinica, 2022, 71(10): 104303. doi: 10.7498/aps.71.20211825
[3]	Yu Jun-Jin, Guo Xing-Yi, Sui Yi-Hui, Song Jian-Ping, Ta De-An, Mei Yong-Feng, Xu Kai-Liang. Ultrafast ultrasound localization microscopy method for spinal cord mircovasculature imaging. Acta Physica Sinica, 2022, 71(17): 174302. doi: 10.7498/aps.71.20220629
[4]	Zhang Jin-Peng, Zhang Yu-Shi, Li Qing-Liang, Wu Jia-Ji. A time-varying Doppler spectrum model of radar sea clutter based on different scattering mechanisms. Acta Physica Sinica, 2018, 67(3): 034101. doi: 10.7498/aps.67.20171612
[5]	Liu Song, Luo Chun-Rong, Zhai Shi-Long, Chen Huai-Jun, Zhao Xiao-Peng. Inverse Doppler effect of acoustic metamaterial with negative mass density. Acta Physica Sinica, 2017, 66(2): 024301. doi: 10.7498/aps.66.024301
[6]	Sun Juan, Li Xiao-Xia, Zhang Jin-Hao, Shen Yu-Zhuo, Li Yan-Yu. Synchronizability and eigenvalues of multilayer star networks through unidirectionally coupling. Acta Physica Sinica, 2017, 66(18): 188901. doi: 10.7498/aps.66.188901
[7]	Zhou Li-Ping, Li Pei, Pan Cong, Guo Li, Ding Zhi-Hua, Li Peng. System of label-free three-dimensional optical coherence tomography angiography with high sensitivity and motion contrast and its applications in brain science. Acta Physica Sinica, 2016, 65(15): 154201. doi: 10.7498/aps.65.154201
[8]	Jiao Bao-Bao. Eigenvalue problems solved by reorthogonalization Lanczos method for the large non-orthonormal sparse matrix. Acta Physica Sinica, 2016, 65(19): 192101. doi: 10.7498/aps.65.192101
[9]	Xu Ming-Ming, Lu Jun-An, Zhou Jin. Synchronizability and eigenvalues of two-layer star networks. Acta Physica Sinica, 2016, 65(2): 028902. doi: 10.7498/aps.65.028902
[10]	Tian Bao-Feng, Zhou Yuan-Yuan, Wang Yue, Li Zhen-Yu, Yi Xiao-Feng. Noise cancellation method for full-wave magnetic resonance sounding signal based on independent component analysis. Acta Physica Sinica, 2015, 64(22): 229301. doi: 10.7498/aps.64.229301
[11]	Xing Hong-Yan, Zhang Qiang, Xu Wei. Fractal property of sea clutter FRFT spectrum for small target detection. Acta Physica Sinica, 2015, 64(11): 110502. doi: 10.7498/aps.64.110502
[12]	Li Jia-Qiang, Cai Hong-Yuan, Chen Jin-Li, Li Peng, Ge Jun-Xiang. A method of wall clutter removal for through-wall radar based on entropy of expanded antenna source. Acta Physica Sinica, 2015, 64(19): 198402. doi: 10.7498/aps.64.198402
[13]	Hao Ben-Jian, Li Zan, Wan Peng-Wu, Si Jiang-Bo. Passive source localization using RROA based on eigenvalue decomposition algorithm in WSNs. Acta Physica Sinica, 2014, 63(5): 054304. doi: 10.7498/aps.63.054304
[14]	Zheng An-Zong, Leng Yong-Gang, Fan Sheng-Bo. Features extraction based on singular value decomposition and stochastic resonance. Acta Physica Sinica, 2012, 61(21): 210503. doi: 10.7498/aps.61.210503
[15]	Liang Yi, Wang Xing-Yuan. Pinning chaotic synchronization in complex networks on maximum eigenvalue of low order matrix. Acta Physica Sinica, 2012, 61(3): 038901. doi: 10.7498/aps.61.038901
[16]	Guo Yi, Guo Yong-Ming, Liu Yang-Yang, Han Chun-Xiao, Wang Jiang, Che Yan-Qiu, Deng Bin. Nonlinear characteristics extraction from electrical signals of dorsal spinal nerve root evoked by acupuncture at Zusanli point. Acta Physica Sinica, 2010, 59(8): 5880-5887. doi: 10.7498/aps.59.5880
[17]	Song Wei, Hou Jian-Jun, Li Zhao-Hong, Huang Liang. A novel zero-bit watermarking algorithm based on Logistic chaotic system and singular value decomposition. Acta Physica Sinica, 2009, 58(7): 4449-4456. doi: 10.7498/aps.58.4449
[18]	Guo Cheng-Bao, Xiao Chang-Han, Liu Da-Ming. Research on the continuations of magnetic field of magnetic object based on integral equation method and singular value decomposition. Acta Physica Sinica, 2008, 57(7): 4182-4188. doi: 10.7498/aps.57.4182
[19]	Huang Qun-Xing, Liu Dong, Wang Fei, Yan Jian-Hua, Chi Yong, Cen Ke-Fa. Study on three-dimensional flame temperature distribution reconstruction based on truncated singular value decomposition. Acta Physica Sinica, 2007, 56(11): 6742-6748. doi: 10.7498/aps.56.6742
[20]	H.C.Lee. On the Factorization Method for Quantum Mechanical Eigenvalue Problems. Acta Physica Sinica, 1944, 5(2): 89-104. doi: 10.7498/aps.5.89

Catalog

Vol. 70, Issue 11 - 2021-06-05

Metrics

Abstract views: 7732
PDF Downloads: 180
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板