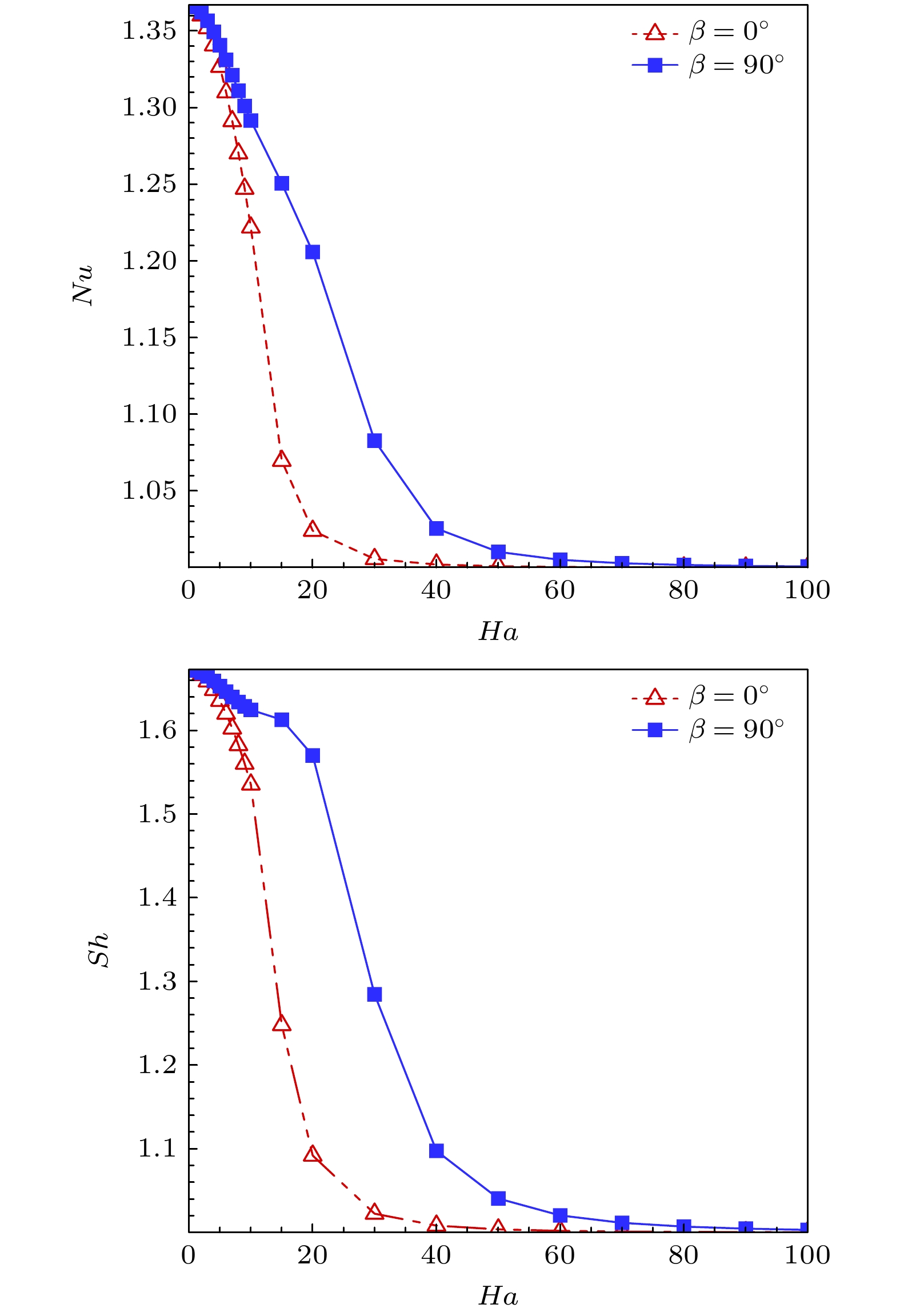
-
Thermal convection in conducting fluids under the influence of a magnetic field is a hot research topic. In this study, a high-precision and high-resolution numerical method is used to directly simulate the double-diffusive convection of liquid metal in a two-dimensional cavity. The study covers the effects of magnetic field strength (Ha), Prandtl number (Pr), Lewis number (Le), and aspect ratio on the dynamics of flow and heat/mass transfer under both horizontal magnetic field and vertical magnetic field. The study considers magnetic field intensities ranging from 0 to 100, Prandtl numbers from 0.01 to 1, Lewis numbers varying from 1 to 100, and aspect ratios spanning from 1 to 12. Within these specified parameter ranges, the outcomes under conditions of no magnetic field (Ha = 0), weak magnetic field (Ha = 10), and strong magnetic field (Ha = 50) are compared with each other. The results show that the magnetic field primarily suppresses flow, heat transfer, and mass transfer. Under the same strength of the magnetic field, the horizontal magnetic field has a greater suppressing effect than the vertical magnetic field. However, the cases of weak magnetic field and strong magnetic field, their effects on heat and mass transfer are similar. Regardless of the orientation and strength of the magnetic field, the heat transfer efficiency and mass transfer efficiency always increase with Prandtl number increasing. The application of a magnetic field can reduce the increase in heat and mass transfer, and when the magnetic field strength reaches a certain level, the Lorentz force predominates, making the influence of the Prandtl number on heat and mass transfer very small. In the presence of a magnetic field, a bifurcation phenomenon is observed around Pr = 0.9. Additionally, as the Lewis number increases, the flow transforms from steady flow to periodic flow, and the influence on mass transfer efficiency becomes more significant. For example, under a horizontal magnetic field, the mass transfer efficiency at the maximum Lewis number is about six times that at the minimum Lewis number. Similarly, under a weak vertical magnetic field, the mass transfer efficiency is about nine times higher. The influence of Lewis number on heat transfer efficiency is relatively minor. Within the range of considered aspect ratios, the heat transfer efficiency and mass transfer efficiency exhibit oscillatory behavior under no magnetic field and weak magnetic field as the aspect ratio increases. However, the heat transfer efficiency and mass transfer efficiency under a strong magnetic field are less affected by the aspect ratio. For flows with the same number of vortices, lower aspect ratio can lead to stronger heat and mass transfer. -
Keywords:
- double-diffusive convection /
- high-accuracy /
- magnetic fluid /
- direct numerical simulation
[1] Degl'Innocenti E L 2003 The Differential Saturation Mechanism: An Application of the Zeeman Effect to the Diagnostic of Magnetic Fields Toulouse, France, September 17–21, 2002 pp71–75
[2] 倪明玖 2013 中国科学: 物理学 力学 天文学 43 1570
Ni M J 2013 Sci. Sin-Phys Mech As 43 1570
[3] Ihli T, Basu T K, Giancarli L M, Konishi S, Malang S, Najmabadi F, Nishio S, Raffray A R, Rao C V S, Sagara A, Wu Y 2008 Fusion Eng. Des. 83 912
Google Scholar
[4] Sun Z H I, Guo M, Vleugels J, Van der Biest O, Blanpain B 2012 Curr. Opin. Solid ST M 16 254
Google Scholar
[5] Zhao R X, Wang J, Cao T W, Hu T, Shuai S S, Xu S Z, Chen C Y, Ren Z M, Qian M 2023 Mat. Sci. Eng. A-Struct 871 144926
Google Scholar
[6] Bachmann M, Avilov V, Gumenyuk A, Rethmeier M 2013 Int. J. Heat Mass Tran. 60 309
Google Scholar
[7] Zhu X O, Liu Z Q, Yin G L, Wang H J, Ren J T 2023 Mater. Res. Express 10 096502
Google Scholar
[8] Bendaraa A, Charafi M M, Hasnaoui A 2019 Eur. Phys. J. Plus 134 468
Google Scholar
[9] Hussain S H, Hussein A K, Mohammed R N 2012 Comput. Math. Appl. 64 476
Google Scholar
[10] Manogaran G, Anbalagan S 2024 Therm. Sci. online first
Google Scholar
[11] Yu P X, Xiao Z C, Wu S 2017 Int. J. Heat Mass Tran. 110 613
Google Scholar
[12] Teamah M A, Shehata A I 2016 Alex. Eng. J. 55 1037
Google Scholar
[13] 李炜, 姜燕妮, 颜君毅, 陈启生 2012 力学学报 44 481
Google Scholar
Li W, Jiang Y N, Yan J Y, Chen Q S 2012 Chin. J. Theor. Appl. Mech. 44 481
Google Scholar
[14] Uddin M B, Rahman M M, Khan M A H 2015 Numer. Heat TR A-Appl. 68 205
Google Scholar
[15] Mondal S, Sibanda P 2016 Int. J. Comp. Meth-Sign. 13 1641015
Google Scholar
[16] Reddy N, Murugesan K 2017 Numer. Heat TR A-Appl. 71 448
Google Scholar
[17] Makayssi T, Lamsaadi M, Kaddiri M 2021 Eur. Phys. J. Plus 136 996
Google Scholar
[18] Liao C C, Li W K, Chu C C 2022 Int. Commun. Heat Mass 130 105817
Google Scholar
[19] Sivaraj C, Sheremet M A 2017 J. Magn. Magn. Mater. 426 351
Google Scholar
[20] Moolya S, Satheesh A 2020 Int. Commun. Heat Mass 118 104814
Google Scholar
[21] Moolya S, Anbalgan S 2021 Int. Commun. Heat Mass 126 105358
Google Scholar
[22] Singh R J, Gohil T B 2019 Comput. Fluids 179 476
Google Scholar
[23] Singh R J, Chandy A J 2020 Int. J. Heat Mass Tran. 157 119823
Google Scholar
[24] Yasin A, Ullah N, Nadeem S, Ghazwani H A 2022 Int. Commun. Heat Mass 135 106066
Google Scholar
[25] Pirmohammadi M, Ghassemi M 2009 Int. Comm Heat Mass 36 7766
[26] Tasaka Y, Yanagisawa T, Fujita K, Miyagoshi T, Sakuraba A 2021 J. Fluid Mech. 911 A19
Google Scholar
[27] Ghosh M, Ghosh P, Nandukumar Y, Pal P 2020 Phys. Fluids 32 024110
Google Scholar
[28] Listratov Y, Ognerubov D, Zikanov O, Sviridov V 2018 Fluid. Dyn. Res. 50 051407
Google Scholar
[29] Ren D W, Wu S, Yang J C, Ni M J 2020 Phys. Fluids 32 053311
Google Scholar
[30] Parsaee S, Payan S, Payan A 2021 Int. J. Therm Sci 169 107072
Google Scholar
[31] Han D Z, Hernandez M, Wang Q 2018 Chaos Soliton. Fract. 114 370
Google Scholar
[32] Zürner T, Liu W J, Krasnov D, Schumacher J 2016 Phys. Rev. E 94 043108
Google Scholar
[33] Yan M, Calkins M A, Maffei S, Julien K, Tobias S M, Marti P 2019 J. Fluid Mech. 877 1186
Google Scholar
[34] Yang J Q, Zhao B X 2021 Comput. Math. Appl. 94 155
Google Scholar
[35] Zhao B X, Yang J Q 2022 Phys. Fluids 34 034120
Google Scholar
[36] Zhao B X, Tian Z F 2015 Phys. Fluids 27 074102
Google Scholar
-
图 1 物理模型
Figure 1. Physical model.
图 2 $Nu, Sh$随Hartmann数的变化
Figure 2. Variation of Nusselt number and Sherwood number as a function of Hartmann number.
图 3 不同磁场下Nu和Sh 随Prandtl数的变化
Figure 3. Variation of Nusselt number and Sherwood number as a function of Prandtl number for different magnetic fields.
图 4 解的分岔现象
Figure 4. Bifurcation of the solution.
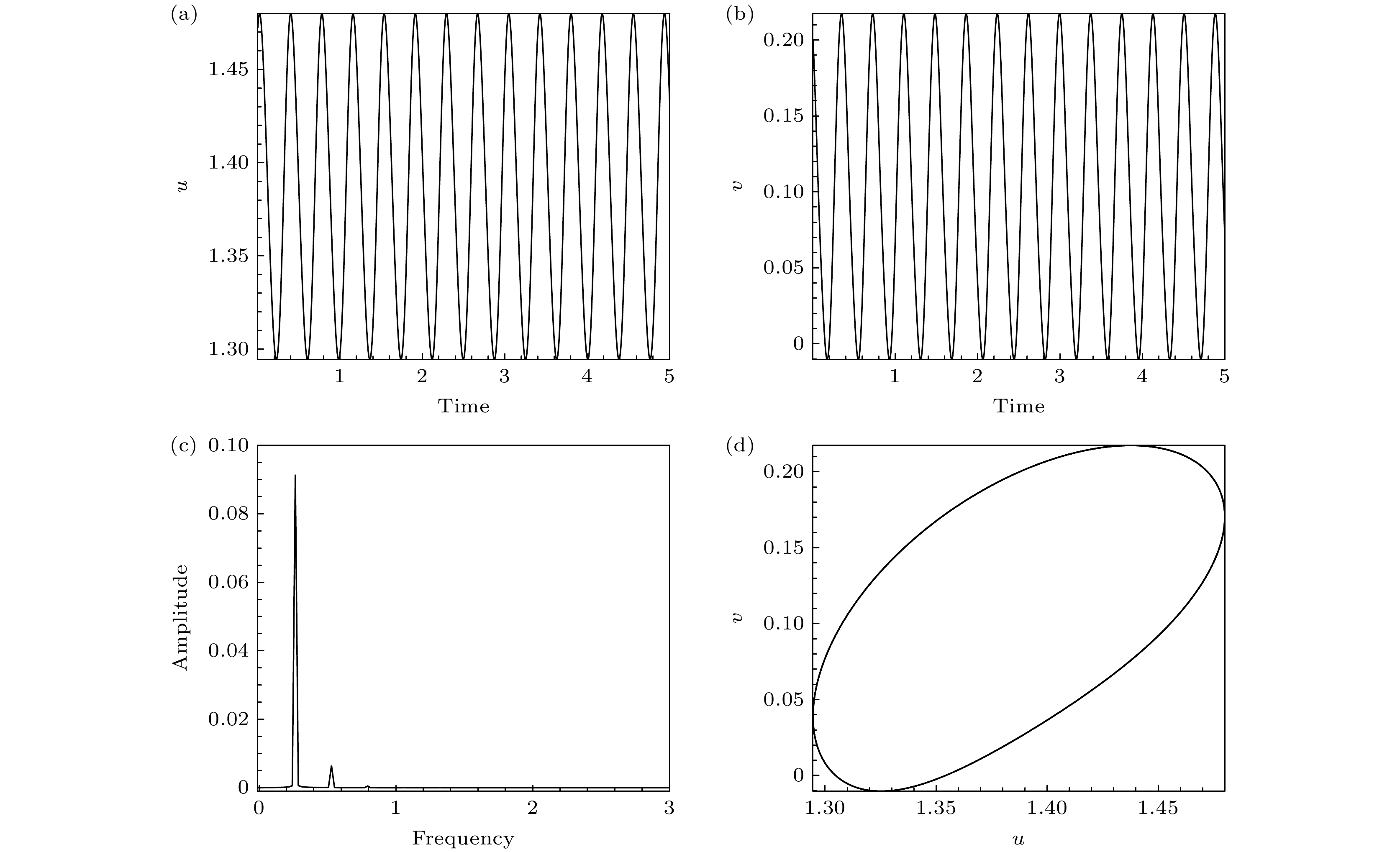
图 5 Pr = 0.01时流场各特征量的变化情况 (a) $u$随时间$t$变化; (b) $v$随时间$t$变化; (c)速度$u$的频谱分析; (d) $u \text{-} v$相位图
Figure 5. Variations of flow field characteristics when Pr = 0.01: (a) Time trace of the u-velocity; (b) time trace of the v-velocity; (c) Fourier frequency spectrum of the u-velocity; (d) phase-space trajectories.
图 6 Pr = 0.01时一个周期内的流场结构
Figure 6. The evolution of the flow pattern over a period for Pr = 0.01.
图 7 不同磁场下Nu和 Sh随Le的变化
Figure 7. Variation of Nusselt number and Sherwood number as a function of Lewis number for different magnetic fields.
图 8 速度$u$的频率随Le数的变化
Figure 8. Variation of the frequency of the $u$-velocity as a function of Lewis number.
图 9 不同磁场下Nu和 Sh随高宽比的变化
Figure 9. Variation of Nusselt number and Sherwood number as a function of the aspect ratio for different magnetic fields.
图 10 $Ha = 10,\; \beta = 90^\circ $时不同高宽比下的流场结构
Figure 10. Flow patterns in cavities of different aspect ratio for $Ha = 10,\; \beta = 90^\circ $.
表 1 $\beta = 90^\circ , Ha = 10$的网格无关性验证
Table 1. Grid independence verification of $\beta = 90^\circ , Ha = 10$.
网格尺寸 umax Error/% vmax Error/% Nu Error/% Sh Error/% $21 \times 41$ 7.928265 4.70 10.26546 4.84 1.27839 1.13 1.609945 0.98 $31 \times 61$ 8.166999 1.83 10.60808 1.66 1.287215 0.44 1.619112 0.42 $41 \times 81$ 8.269498 0.59 10.73112 0.52 1.291532 0.11 1.624656 0.08 $51 \times 101$ 8.318981 10.78753 1.292946 1.625911 
DownLoad: CSV
表 2 $\beta = 0^\circ , Ha = 5$的网格无关性验证
Table 2. Grid independence verification of $\beta = 0^\circ , Ha = 5$.
网格尺寸 umax Error/% vmax Error/% Nu Error/% Sh Error/% $21 \times 41$ 9.799929 8.07 11.37238 7.23 1.307235 1.76 1.618727 1.29 $31 \times 61$ 10.29227 3.45 11.89485 2.97 1.318517 0.91 1.625802 0.86 $41 \times 81$ 10.51703 1.34 12.11855 1.15 1.326589 0.31 1.635453 0.27 $51 \times 101$ 10.6599 12.25902 1.330674 1.639908
DownLoad: CSV
表 3 弱磁场($Ha = 10$)下Lewis数对流动的影响
Table 3. Effect of Lewis number on the flow for $Ha = 10$.
磁场方向 定常解范围(Le) 周期解范围(Le) $\beta = 0^\circ $ $\left[ {1, 8} \right]$ $\left[ {9, 100} \right]$ $\beta = 90^\circ $ $\left[ {1, 6} \right]$ $\left[ {7, 100} \right]$
DownLoad: CSV
-
[1] Degl'Innocenti E L 2003 The Differential Saturation Mechanism: An Application of the Zeeman Effect to the Diagnostic of Magnetic Fields Toulouse, France, September 17–21, 2002 pp71–75
[2] 倪明玖 2013 中国科学: 物理学 力学 天文学 43 1570
Ni M J 2013 Sci. Sin-Phys Mech As 43 1570
[3] Ihli T, Basu T K, Giancarli L M, Konishi S, Malang S, Najmabadi F, Nishio S, Raffray A R, Rao C V S, Sagara A, Wu Y 2008 Fusion Eng. Des. 83 912
Google Scholar
[4] Sun Z H I, Guo M, Vleugels J, Van der Biest O, Blanpain B 2012 Curr. Opin. Solid ST M 16 254
Google Scholar
[5] Zhao R X, Wang J, Cao T W, Hu T, Shuai S S, Xu S Z, Chen C Y, Ren Z M, Qian M 2023 Mat. Sci. Eng. A-Struct 871 144926
Google Scholar
[6] Bachmann M, Avilov V, Gumenyuk A, Rethmeier M 2013 Int. J. Heat Mass Tran. 60 309
Google Scholar
[7] Zhu X O, Liu Z Q, Yin G L, Wang H J, Ren J T 2023 Mater. Res. Express 10 096502
Google Scholar
[8] Bendaraa A, Charafi M M, Hasnaoui A 2019 Eur. Phys. J. Plus 134 468
Google Scholar
[9] Hussain S H, Hussein A K, Mohammed R N 2012 Comput. Math. Appl. 64 476
Google Scholar
[10] Manogaran G, Anbalagan S 2024 Therm. Sci. online first
Google Scholar
[11] Yu P X, Xiao Z C, Wu S 2017 Int. J. Heat Mass Tran. 110 613
Google Scholar
[12] Teamah M A, Shehata A I 2016 Alex. Eng. J. 55 1037
Google Scholar
[13] 李炜, 姜燕妮, 颜君毅, 陈启生 2012 力学学报 44 481
Google Scholar
Li W, Jiang Y N, Yan J Y, Chen Q S 2012 Chin. J. Theor. Appl. Mech. 44 481
Google Scholar
[14] Uddin M B, Rahman M M, Khan M A H 2015 Numer. Heat TR A-Appl. 68 205
Google Scholar
[15] Mondal S, Sibanda P 2016 Int. J. Comp. Meth-Sign. 13 1641015
Google Scholar
[16] Reddy N, Murugesan K 2017 Numer. Heat TR A-Appl. 71 448
Google Scholar
[17] Makayssi T, Lamsaadi M, Kaddiri M 2021 Eur. Phys. J. Plus 136 996
Google Scholar
[18] Liao C C, Li W K, Chu C C 2022 Int. Commun. Heat Mass 130 105817
Google Scholar
[19] Sivaraj C, Sheremet M A 2017 J. Magn. Magn. Mater. 426 351
Google Scholar
[20] Moolya S, Satheesh A 2020 Int. Commun. Heat Mass 118 104814
Google Scholar
[21] Moolya S, Anbalgan S 2021 Int. Commun. Heat Mass 126 105358
Google Scholar
[22] Singh R J, Gohil T B 2019 Comput. Fluids 179 476
Google Scholar
[23] Singh R J, Chandy A J 2020 Int. J. Heat Mass Tran. 157 119823
Google Scholar
[24] Yasin A, Ullah N, Nadeem S, Ghazwani H A 2022 Int. Commun. Heat Mass 135 106066
Google Scholar
[25] Pirmohammadi M, Ghassemi M 2009 Int. Comm Heat Mass 36 7766
[26] Tasaka Y, Yanagisawa T, Fujita K, Miyagoshi T, Sakuraba A 2021 J. Fluid Mech. 911 A19
Google Scholar
[27] Ghosh M, Ghosh P, Nandukumar Y, Pal P 2020 Phys. Fluids 32 024110
Google Scholar
[28] Listratov Y, Ognerubov D, Zikanov O, Sviridov V 2018 Fluid. Dyn. Res. 50 051407
Google Scholar
[29] Ren D W, Wu S, Yang J C, Ni M J 2020 Phys. Fluids 32 053311
Google Scholar
[30] Parsaee S, Payan S, Payan A 2021 Int. J. Therm Sci 169 107072
Google Scholar
[31] Han D Z, Hernandez M, Wang Q 2018 Chaos Soliton. Fract. 114 370
Google Scholar
[32] Zürner T, Liu W J, Krasnov D, Schumacher J 2016 Phys. Rev. E 94 043108
Google Scholar
[33] Yan M, Calkins M A, Maffei S, Julien K, Tobias S M, Marti P 2019 J. Fluid Mech. 877 1186
Google Scholar
[34] Yang J Q, Zhao B X 2021 Comput. Math. Appl. 94 155
Google Scholar
[35] Zhao B X, Yang J Q 2022 Phys. Fluids 34 034120
Google Scholar
[36] Zhao B X, Tian Z F 2015 Phys. Fluids 27 074102
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1726
- PDF Downloads: 35
- Cited By: 0
