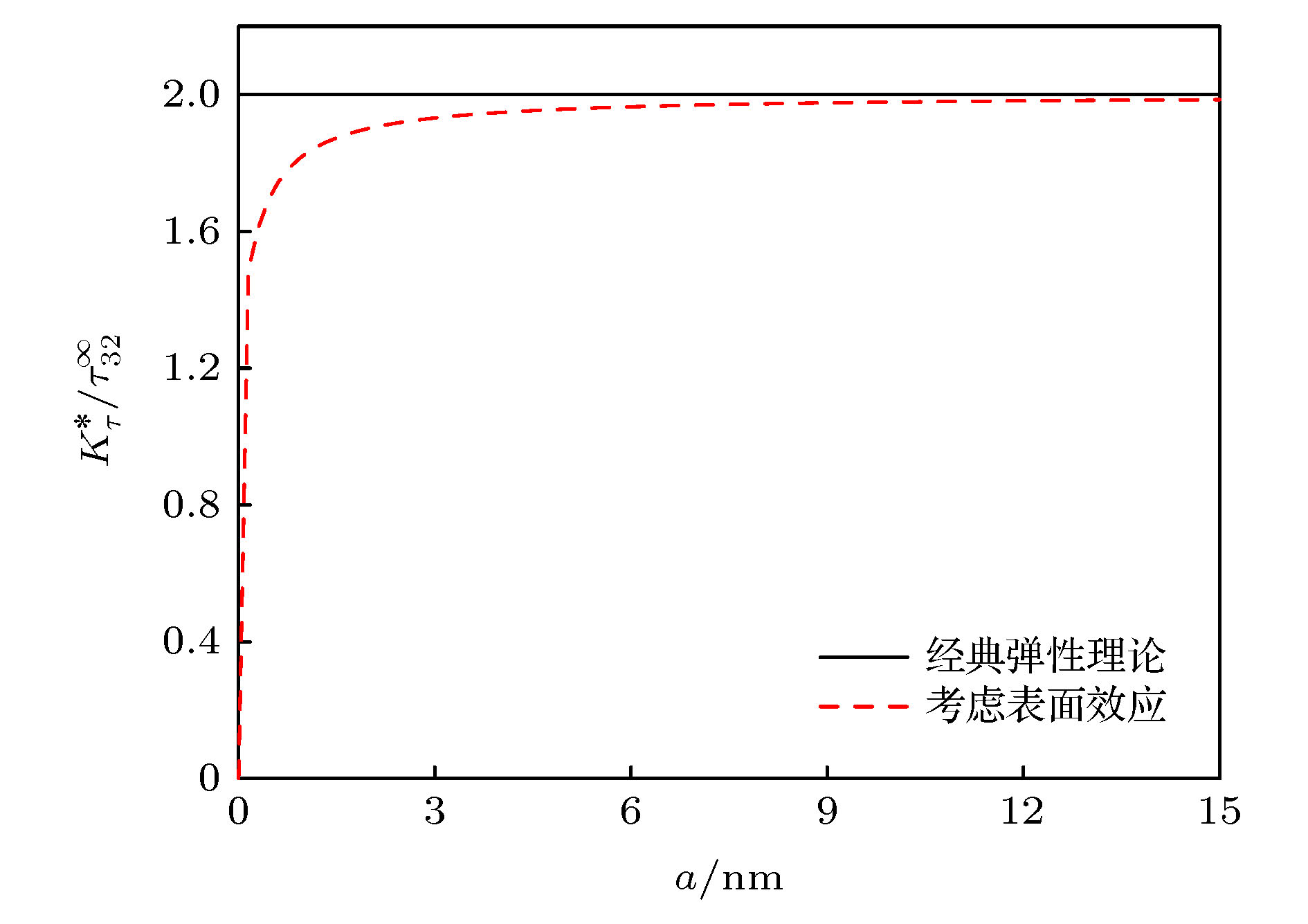
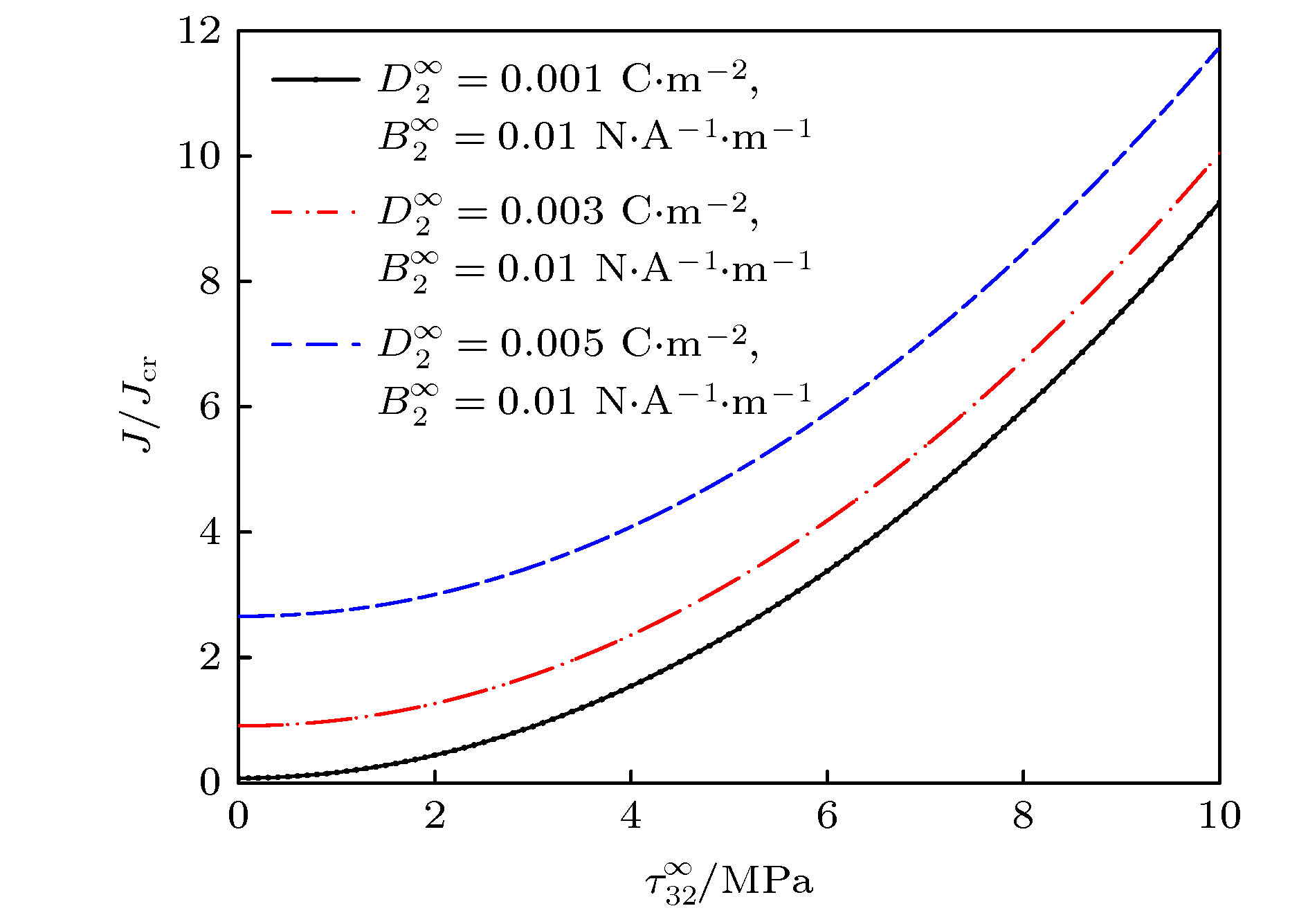
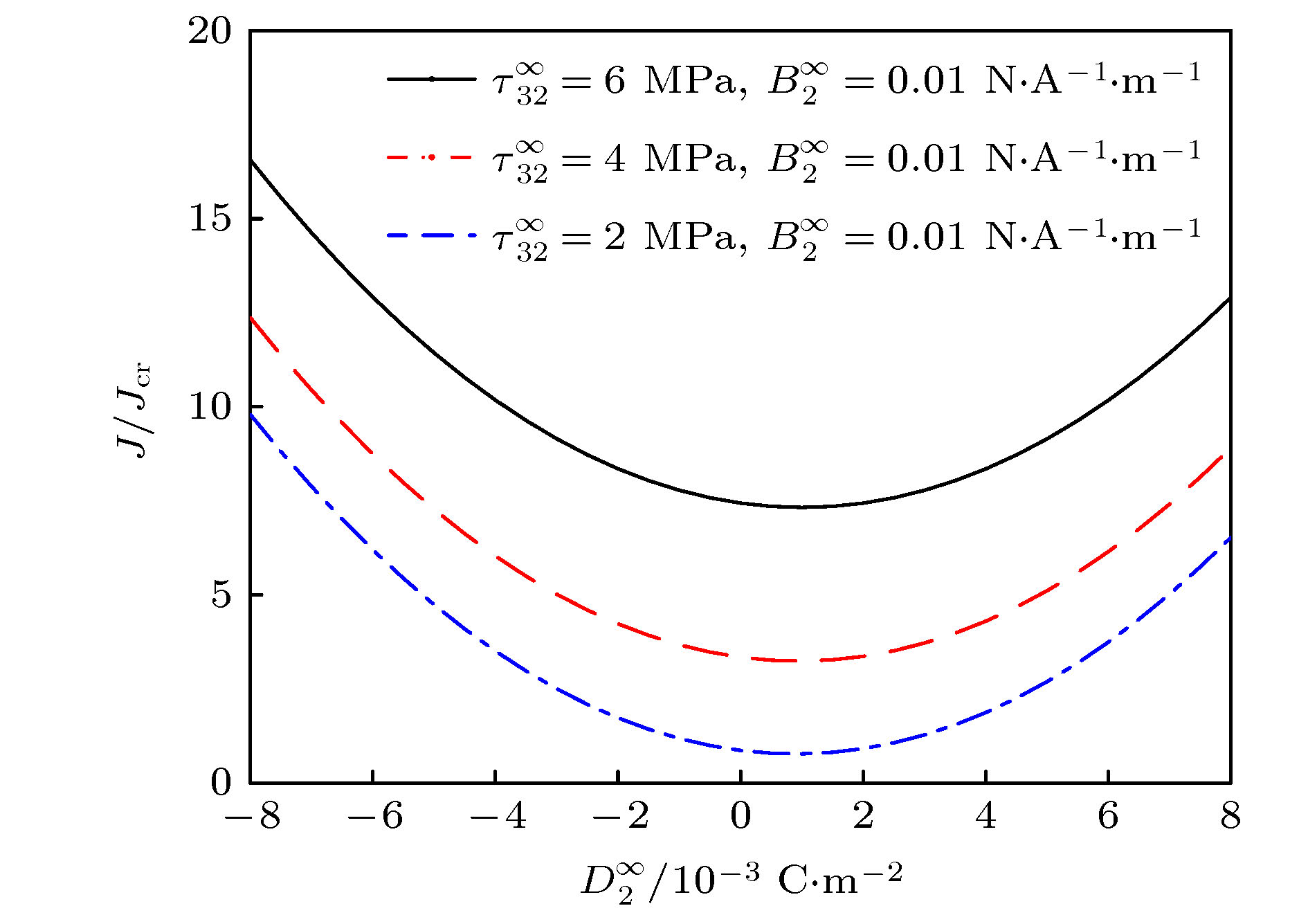
-
According to the conformal mapping from the exterior region of the regular n-polygon hole to the exterior region of a unit circle and from the exterior region of four cracks emanating from a circle to the interior region of a unit circle, a new conformal mapping is constructed to map the exterior region of four cracks emanating from a regular 4n-polygon hole to the interior of a unit circle. Then, based on the Gurtin-Murdoch surface/interface model and complex method, the anti-plane fracture of four nano-cracks emanating from a regular 4n-polygon nano-hole in magnetoelectroelastic material is studied. The exact solutions of stress intensity factor, electric displacement intensity factor, magnetic induction intensity factor, and energy release rate are obtained under the boundary condition of magnetoelectrically impermeable with considering the surface effect. Without considering the effect of the surface effect, the exact solution of four cracks emanating from a regular 4n-polygon hole in a magnetoelectroelastic material can be obtained. The numerical results show the influences of surface effect and the size of defect on the stress intensity factor, electric displacement intensity factor, magnetic induction intensity factor and energy release rate under the magnetoelectrically impermeable boundary condition. It can be seen that the stress intensity factor, electric displacement intensity factor, and magnetic induction intensity factor are significantly size-dependent when considering the surface effects of the nanoscale defects. And when the size of defect increases to a certain extent, the influence of surface effect begins to decrease and finally tends to follow the classical elasticity theory. When the distance between the center and the vertex of the regular 4n-polygon nano-hole is constant, the dimensionless field intensity factor decreases gradually with the increase of the number of edges, and approaches to the conclusion of a circular hole with four cracks. With the increase of the relative size of the crack, the dimensionless field intensity factor increases gradually. The dimensionless energy release rate of the nanoscale cracked hole has a significant size effect. The increase of mechanical load will increase the normalized energy release rate. The normalized energy release rate first decreases and then increases with electrical load increasing. The normalized energy release rate decreases with magnetic load increasing.
-
Keywords:
- magnetoelectroelastic materials /
- surface effect /
- nanoscale
[1] Nan C W 1994 Phys. Rev. B 50 6082
Google Scholar
[2] Guo J H, Lu Z X 2010 Int. J. solids Struct. 47 1847
Google Scholar
[3] Rogowski B 2011 Arch. Appl. Mech. 81 1607
Google Scholar
[4] 刘鑫, 郭俊宏, 于静 2016 内蒙古大学学报(自然科学版) 41 37
Google Scholar
Liu X, Guo J H, Yu J 2016 J. Inner Monglia Univ. (Natural Science Edition) 41 37
Google Scholar
[5] Gao C F, Kessler H, Balke H 2003 Int. J. Eng. Sci. 41 969
Google Scholar
[6] Gao C F, Kessler H, Balke H 2003 Int. J. Eng. Sci. 41 983
Google Scholar
[7] Liu X, Guo J H 2016 Theor. Appl. Fract. Mech. 86 225
Google Scholar
[8] 齐敏 2005 硕士学位论文 (石家庄: 石家庄铁道大学)
Qi M 2005 M. S. Thesis (Shijiazhuang: Shijiazhuang Tiedao University) (in Chinese)
[9] Lv X, Liu G T 2018 Chin. Phys. B 27 074601
Google Scholar
[10] Zhong X C, Li C F 2008 Arch. Appl. Mech. 78 117
Google Scholar
[11] Gurtin M E, Murdoch A I 1975 Arch. Ration. Mech. Anal. 57 291
Google Scholar
[12] Gurtin M E, Murdoch A I 1978 Int. J. Solids Struct. 14 431
Google Scholar
[13] Gurtin M E, Weissmuller J, Larche F 1998 Philos. Mag. A 78 1093
Google Scholar
[14] Xiao J H, XU Y L, Zhang F C 2018 Acta. Mech. 229 4915
Google Scholar
[15] 肖俊华, 崔友强, 徐耀玲, 张福成 2018 中国机械工程 29 2347
Google Scholar
Xiao J H, Cui Y Q, Xu Y L, Zhang F C 2018 China Mech. Eng. 29 2347
Google Scholar
[16] Xiao J H, Cui Y Q, Xu Y L, Zhang F C 2018 Theor. Appl. Fract. Mech. 96 476
Google Scholar
[17] Guo J H, Li X F 2018 Acta Mech. 229 4251
Google Scholar
[18] Liu Y Z, Guo J H, Zhang X Y 2019 Z. Angew. Math. Mech. 99 e201900043
[19] Guo J H, He L T, Liu Y Z, Li L H 2020 Theor. Appl. Fract. Mech. 107 102553
Google Scholar
[20] Guo J H, Lu Z X 2011 Appl. Math. Comput. 217 9397
Google Scholar
[21] 王永健 2012 博士学位论文 (南京: 南京航空航天大学)
Wang Y J 2012 Ph. D. Dissertation (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[22] Fan S W, Guo J H, Yu J 2017 Chin. J. Aeronaut. 30 461
Google Scholar
[23] Dharmendra S, Sharma 2014 Int. J. Mech. Sci. 78 177
Google Scholar
[24] Wang Y B, Guo J H 2018 Appl. Math. Mech. -Engl. 39 797
Google Scholar
[25] Fang X Q, Gupta V, Liu J X 2013 Philos. Mag. Lett. 93 58
Google Scholar
[26] 穆斯海里什维里 著(赵惠元 译) 1958 数学弹性力学的几个基本问题 (北京: 科学出版社) 第233页
Muskhelishvili N I (translated by Zhao H Y) 1958 Some Basic Problems of the Mathematical Theory of Elasticity (Beijing: Science Press) p233 (in Chinese)
-
图 1 磁电弹性材料中含有带四条裂纹的正方形孔
Figure 1. Four cracks emanating from a square hole in magnetoelectroelastic materials
图 2 保角变换
Figure 2. Conformal mapping
图 3 只受机械载荷作用时表面效应对应力强度因子的影响
Figure 3. Surface effect on the stress intensity factor near the crack tip induced by anti-plane mechanical load
$\tau_{32}^{\infty}$ only
图 4 只受电载荷作用时表面效应对应力强度因子的影响
Figure 4. Surface effect on the stress intensity factor near the crack tip induced by in-plane electrical load
$D_2^{\infty}$ only
图 5 只受磁载荷作用时表面效应对应力强度因子的影响
Figure 5. Surface effect on the stress intensity factor near the crack tip induced by in-plane magnetic load
$B_2^{\infty}$ only
图 6 只受机械荷作用时表面效应对电位移强度因子的影响
Figure 6. Surface effect on the electric displacement intensity factor near the crack tip induced by anti-plane mechanical load
$\tau_{32}^{\infty}$ only
图 7 只受电载荷作用时表面效应对电位移强度因子的影响
Figure 7. Surface effect on the electric displacement intensity factor near the crack tip induced by in-plane electrical load
$D_2^{\infty}$ only
图 8 只受磁载荷作用时表面效应对电位移强度因子的影响
Figure 8. Surface effect on the electric displacement intensity factor near the crack tip induced by in-plane magnetic load
$B_2^{\infty}$ only
图 9 只受机械载荷作用时表面效应对磁感应强度因子的影响
Figure 9. Surface effect on the magnetic induction intensity factor near the crack tip induced by anti-plane mechanical load
$\tau_{32}^{\infty}$ only
图 10 只受电载荷作用时表面效应对磁感应强度因子的影响
Figure 10. Surface effect on the magnetic induction intensity factor near the crack tip induced by in-plane electrical load
$D_2^{\infty}$ only
图 11 只受磁载荷作用时表面效应对磁感应强度因子的影响
Figure 11. Surface effect on the magnetic induction intensity factor near the crack tip induced by in-plane magnetic load
$B_2^{\infty}$ only
图 12 无量纲应力强度因子随右侧裂纹长度变化
Figure 12. Variations of the dimensionless stress intensity factor at crack tip with the crack length
$L_1$ 
图 13 无量纲应力强度因子随n的变化
Figure 13. Variations of the dimensionless stress intensity factor at crack tip with n
图 14 无量纲能量释放率随孔口尺寸的变化
Figure 14. Variations of the dimensionless energy release rate with the size of the cracked hole
图 15 正则化能量释放率随机械载荷的变化
Figure 15. Variations of the normalized energy release rate with the applied mechanical load
图 16 正则化能量释放率随电载荷的变化
Figure 16. Variations of the normalized energy release rate with the applied eletrical load
图 17 正则化能量释放率随磁载荷的变化
Figure 17. Variations of the normalized energy release rate with the applied magnetic load
-
[1] Nan C W 1994 Phys. Rev. B 50 6082
Google Scholar
[2] Guo J H, Lu Z X 2010 Int. J. solids Struct. 47 1847
Google Scholar
[3] Rogowski B 2011 Arch. Appl. Mech. 81 1607
Google Scholar
[4] 刘鑫, 郭俊宏, 于静 2016 内蒙古大学学报(自然科学版) 41 37
Google Scholar
Liu X, Guo J H, Yu J 2016 J. Inner Monglia Univ. (Natural Science Edition) 41 37
Google Scholar
[5] Gao C F, Kessler H, Balke H 2003 Int. J. Eng. Sci. 41 969
Google Scholar
[6] Gao C F, Kessler H, Balke H 2003 Int. J. Eng. Sci. 41 983
Google Scholar
[7] Liu X, Guo J H 2016 Theor. Appl. Fract. Mech. 86 225
Google Scholar
[8] 齐敏 2005 硕士学位论文 (石家庄: 石家庄铁道大学)
Qi M 2005 M. S. Thesis (Shijiazhuang: Shijiazhuang Tiedao University) (in Chinese)
[9] Lv X, Liu G T 2018 Chin. Phys. B 27 074601
Google Scholar
[10] Zhong X C, Li C F 2008 Arch. Appl. Mech. 78 117
Google Scholar
[11] Gurtin M E, Murdoch A I 1975 Arch. Ration. Mech. Anal. 57 291
Google Scholar
[12] Gurtin M E, Murdoch A I 1978 Int. J. Solids Struct. 14 431
Google Scholar
[13] Gurtin M E, Weissmuller J, Larche F 1998 Philos. Mag. A 78 1093
Google Scholar
[14] Xiao J H, XU Y L, Zhang F C 2018 Acta. Mech. 229 4915
Google Scholar
[15] 肖俊华, 崔友强, 徐耀玲, 张福成 2018 中国机械工程 29 2347
Google Scholar
Xiao J H, Cui Y Q, Xu Y L, Zhang F C 2018 China Mech. Eng. 29 2347
Google Scholar
[16] Xiao J H, Cui Y Q, Xu Y L, Zhang F C 2018 Theor. Appl. Fract. Mech. 96 476
Google Scholar
[17] Guo J H, Li X F 2018 Acta Mech. 229 4251
Google Scholar
[18] Liu Y Z, Guo J H, Zhang X Y 2019 Z. Angew. Math. Mech. 99 e201900043
[19] Guo J H, He L T, Liu Y Z, Li L H 2020 Theor. Appl. Fract. Mech. 107 102553
Google Scholar
[20] Guo J H, Lu Z X 2011 Appl. Math. Comput. 217 9397
Google Scholar
[21] 王永健 2012 博士学位论文 (南京: 南京航空航天大学)
Wang Y J 2012 Ph. D. Dissertation (Nanjing: Nanjing University of Aeronautics and Astronautics) (in Chinese)
[22] Fan S W, Guo J H, Yu J 2017 Chin. J. Aeronaut. 30 461
Google Scholar
[23] Dharmendra S, Sharma 2014 Int. J. Mech. Sci. 78 177
Google Scholar
[24] Wang Y B, Guo J H 2018 Appl. Math. Mech. -Engl. 39 797
Google Scholar
[25] Fang X Q, Gupta V, Liu J X 2013 Philos. Mag. Lett. 93 58
Google Scholar
[26] 穆斯海里什维里 著(赵惠元 译) 1958 数学弹性力学的几个基本问题 (北京: 科学出版社) 第233页
Muskhelishvili N I (translated by Zhao H Y) 1958 Some Basic Problems of the Mathematical Theory of Elasticity (Beijing: Science Press) p233 (in Chinese)
-
[1] Xiao Dong-Dong, Gu Lin. Picoscale structure of functional materials constructed by functional units. Acta Physica Sinica, 2023, 72(11): 116801. doi: 10.7498/aps.72.20230045 [2] Yang Zhi-Ping, Kong Xi, Shi Fa-Zhan, Du Jiang-Feng. Phase transition observation of nanoscale water on diamond surface. Acta Physica Sinica, 2022, 71(6): 067601. doi: 10.7498/aps.71.20211348 [3] Chen Xiao-Ming, Li Guo-Rong. Large electrostrictive coefficients of BaTiO3-based lead-free ceramics. Acta Physica Sinica, 2022, 71(16): 167701. doi: 10.7498/aps.71.20220451 [4] Shang Shuai-Peng, Lu Yong-Jun, Wang Feng-Hui. Surface effects on buckling of nanowire electrode. Acta Physica Sinica, 2022, 71(3): 033101. doi: 10.7498/aps.71.20211864 [5] . Phase Transition Observation of Nanoscale Water on Diamond Surface. Acta Physica Sinica, 2021, (): . doi: 10.7498/aps.70.20211348 [6] Zhang Meng, Yao Ruo-He, Liu Yu-Rong. A channel thermal noise model of nanoscaled metal-oxide-semiconductor field-effect transistor. Acta Physica Sinica, 2020, 69(5): 057101. doi: 10.7498/aps.69.20191512 [7] Liang Jin-Jie, Gao Ning, Li Yu-Hong. Surface effect on ${\langle 100 \rangle }$ interstitial dislocation loop in iron. Acta Physica Sinica, 2020, 69(3): 036101. doi: 10.7498/aps.69.20191379[8] Zhang Ye, Zhang Ran, Chang Qing, Li Hua. Surface effects on Couette gas flows in nanochannels. Acta Physica Sinica, 2019, 68(12): 124702. doi: 10.7498/aps.68.20190248 [9] Peng Jie-Yang, Wang Jia-Hai, Shen Bin, Li Hao-Liang, Sun Hao-Ming. Influences of nanoscale particles and interparticle compression in electrodes on voltage hysteresis of lithium ion batteries. Acta Physica Sinica, 2019, 68(9): 090202. doi: 10.7498/aps.68.20182302 [10] Dong Yang, Du Bo, Zhang Shao-Chun, Chen Xiang-Dong, Sun Fang-Wen. Solid quantum sensor based on nitrogen-vacancy center in diamond. Acta Physica Sinica, 2018, 67(16): 160301. doi: 10.7498/aps.67.20180788 [11] Zhang Long-Yan, Xu Jin-Liang, Lei Jun-Peng. Molecular dynamics study of bubble nucleation on a nanoscale. Acta Physica Sinica, 2018, 67(23): 234702. doi: 10.7498/aps.67.20180993 [12] Li Sheng-Kun, Tang Jun, Mao Hong-Qing, Wang Ming-Huan, Chen Guo-Bin, Zhai Chao, Zhang Xiao-Ming, Shi Yun-Bo, Liu Jun. Effect of magnetic capacitance in the Fe3O4 nanopartides and polydimethylsiloxane composite material. Acta Physica Sinica, 2014, 63(5): 057501. doi: 10.7498/aps.63.057501 [13] Tang Dong-He, Du Lei, Wang Ting-Lan, Chen Hua, Chen Wen-Hao. Qualitative analysis of excess noise in nanoscale MOSFET. Acta Physica Sinica, 2011, 60(10): 107201. doi: 10.7498/aps.60.107201 [14] Yang Chang-Hai, Wen Yu-Mei, Li Ping, Bian Lei-Xiang. Influence of bias magnetic field on magnetoelectric effect of magnetostrictive/elastic/piezoelectric laminated composite. Acta Physica Sinica, 2008, 57(11): 7292-7297. doi: 10.7498/aps.57.7292 [15] Zhang Dong-Xian, Liu Chao, Zhang Hai-Jun. The effect of infrared laser-induced micro/nano photothermal expansion and the novel method of photothermal actuation. Acta Physica Sinica, 2008, 57(5): 3107-3112. doi: 10.7498/aps.57.3107 [16] Luo Wen-Xiong, Huang Shi-Hua, You Fang-Tian, Peng Hong-Shang. The luminescent properties of YBO3:Eu3+ nanocrystal. Acta Physica Sinica, 2007, 56(3): 1765-1769. doi: 10.7498/aps.56.1765 [17] Tian Jian-Hui, Han Xu, Liu Gui-Rong, Long Shu-Yao, Qin Jin-Qi. Investigation of the SiC nano-bar relaxation characteristics. Acta Physica Sinica, 2007, 56(2): 643-648. doi: 10.7498/aps.56.643 [18] Zeng Hua-Rong, Yu Han-Feng, Chu Rui-Qing, Li Guo-Rong, Yin Qing-Rui, Tang Xin-Gui. Field-induced displacement properties of nanoscale domain structure in PZT thin film. Acta Physica Sinica, 2005, 54(3): 1437-1441. doi: 10.7498/aps.54.1437 [19] DU YOU-WEI, CHEN PENG, ZHU JIAN-MIN, XING DING-YU. GIANT TUNNELING MAGNETORESISTANCE IN NANOSTRUCTURED ZnxFe3-xO4-α-Fe2O3 POLYCRYSTALLINE MATERIAL. Acta Physica Sinica, 2001, 50(11): 2275-2277. doi: 10.7498/aps.50.2275 [20] YU HONG-BIN, YANG WEI-SHENG. SCANNING TUNNELING MICROSCOPY INVESTIGATION OF GOLD ISLANDS FORMED ON HOPG SURFACE BY VACUUM DEPOSITION. Acta Physica Sinica, 1997, 46(3): 500-504. doi: 10.7498/aps.46.500
DownLoad:
Catalog
Metrics
- Abstract views: 4635
- PDF Downloads: 58
- Cited By: 0
