-
在材料辐照损伤过程中, 间隙型位错环的形成及动力学行为严重影响材料在辐照条件下的服役行为. 在常用的以体心立方铁为基的合金材料中, 1/2
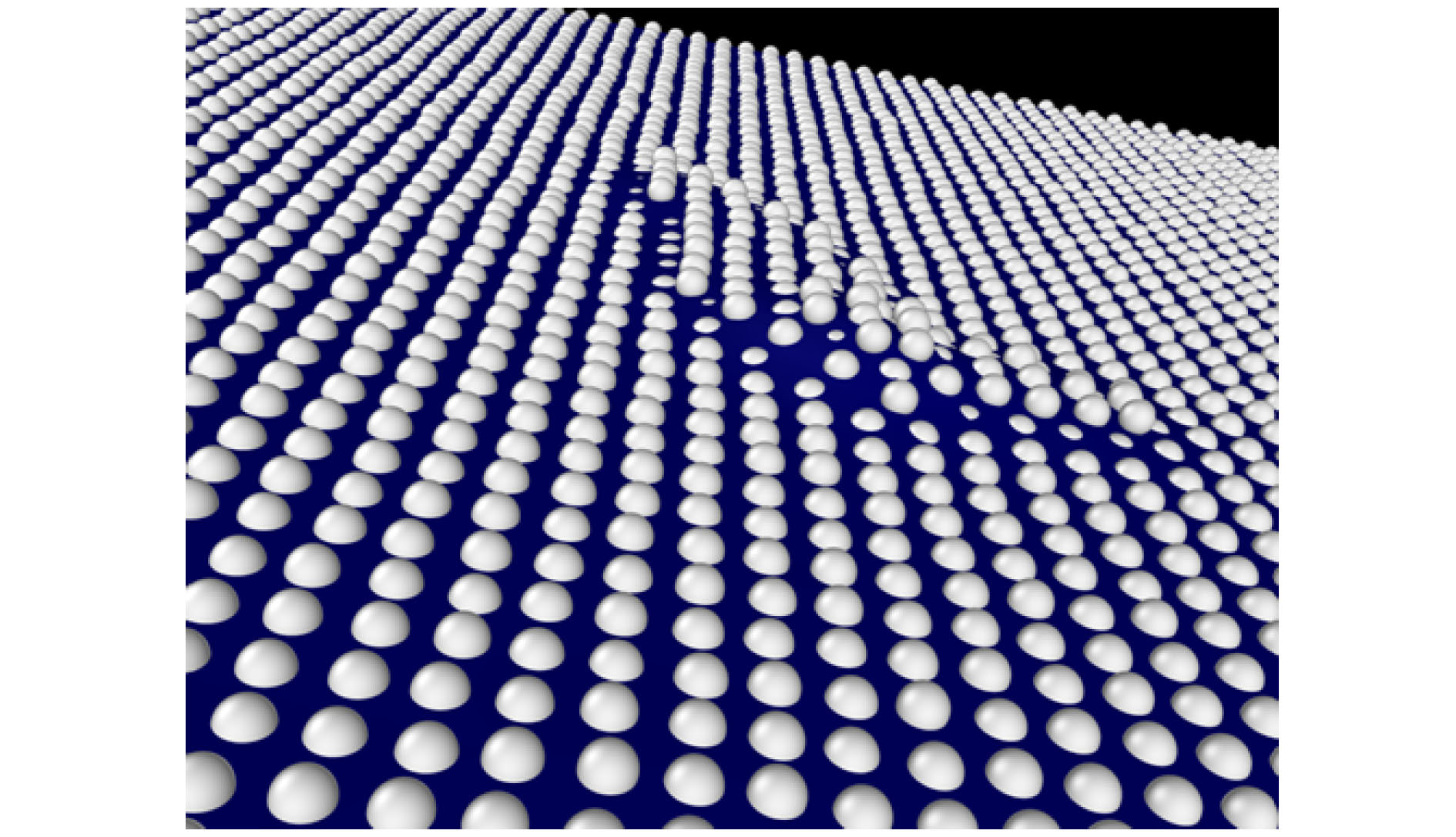
$\left\langle 111 \right\rangle $ 和$\left\langle 100 \right\rangle $ 是两种主要的位错环, 其对辐照损伤的影响一直都是核材料领域研究的热点之一. 在之前的研究中, 人们对{111}面与单个1/2$\left\langle 111 \right\rangle $ 位错环的相互作用进行了深入研究, 发现表面对位错环性质确实有重要的影响. 采用分子动力学方法, 在原子尺度详细研究了另一个重要的表面铁{100}面对$\left\langle 100 \right\rangle $ 间隙型位错环动力学过程的影响. 模拟发现位错环伯格斯矢量与表面法线方向的关系、距表面的深度、位错环之间的相互作用以及温度等, 都对位错环与表面的相互作用产生重要影响, 其中, 表面作用下的伯格斯矢量的演化以及$\left\langle 100 \right\rangle $ 位错环在此过程中的一维运动首次被发现. 基于这些模拟结果, 就$\left\langle 100 \right\rangle $ 位错环对表面辐照损伤结构的影响进行详细地研究, 给出$\left\langle 100 \right\rangle $ 位错环对表面凹凸结构的贡献, 这些结果为理解辐照过程中材料表面的演化提供一种可能的解释.Formation and evolution of interstitial dislocation loop induced by radiation damage in a material are confirmed to seriously affect the performance of the material under irradiation. For example, in body-centered cubic Fe based alloy, 1/2$\left\langle 111 \right\rangle $ and$\left\langle 100 \right\rangle $ are mainly formed during the irradiation, which is related to various degradations of material properties. Thus, the understanding of their effect on radiation damages of material is always one of the hottest topics in nuclear material society. Previous studies have shown the surface effect on 1/2$\left\langle 111 \right\rangle $ loop through the investigation of the interaction between 1/2$\left\langle 111 \right\rangle $ loop and {111} surface. Considering the difference in property between 1/2$\left\langle 111 \right\rangle $ loop and$\left\langle 100 \right\rangle $ loop, in this work the interaction between a$\left\langle 100 \right\rangle $ loop and {100} surface is studied in detail through the molecular dynamics method. The simulation results indicate that the factors including Burgers vector of loop, loop-to-surface depth, interaction between pre-existing$\left\langle 100 \right\rangle $ loops, and temperature, all seriously affect the interaction between loop and surface. Especially, the present results show for the first time the evolution of Burgers vector of$\left\langle 100 \right\rangle $ loop from$\left\langle 100 \right\rangle $ to 1/2$\left\langle 111 \right\rangle $ and its one-dimensional diffusion to surface. According to these results, we also further explore the surface evolution after its interaction with loop. The appearance of atomic island results in the rugged surface morphology. All these results provide a new insight into the radiation damage to the surface of material.-
Keywords:
- radiation damage /
- dislocation loop /
- molecular dynamics simulation /
- surface effect
[1] Masters B C 1965 Philos. Mag. 11 881
 Google Scholar
Google Scholar
[2] Yao Z, Hernández-Mayoral M, Jenkins M L, Kirk M A 2008 Philos. Mag. 88 2851
 Google Scholar
Google Scholar
[3] Dudarev S L, Bullough R, Derlet P M 2008 Phys. Rev. Lett. 100 135503
 Google Scholar
Google Scholar
[4] Hernández-Mayoral M, Yao Z, Jenkins M L, Kirk M A 2008 Philos. Mag. 88 2881
 Google Scholar
Google Scholar
[5] Jenkins M L, Yao Z, Hernández-Mayoral M, Kirk M A 2009 J. Nucl. Mater. 389 197
[6] Yao Z, Jenkins M L, Hernández-Mayoral M, Kirk M A 2010 Philos. Mag. 90 4623
 Google Scholar
Google Scholar
[7] Prokhodtseva A, Décamps B, Schäublin R 2013 J. Nucl. Mater. 442 S786
 Google Scholar
Google Scholar
[8] Arakawa K, Hatanaka M, Kuramoto E, Ono K, Mori H 2006 Phys. Rev. Lett. 96 125506
 Google Scholar
Google Scholar
[9] 黄依娜, 万发荣, 焦治杰 2011 60 036802
 Google Scholar
Google Scholar
Huang Y N, Wan F R, Jiao Z J 2011 Acta Phys. Sin. 60 036802
 Google Scholar
Google Scholar
[10] 姜少宁, 万发荣, 龙毅, 刘传歆, 詹倩, 大貫惣明 2013 62 166801
 Google Scholar
Google Scholar
Jiang S N, Wan F R, Long Y, Liu C X, Zhan Q, Ohnuki S 2013 Acta Phys. Sin. 62 166801
 Google Scholar
Google Scholar
[11] 杜玉峰, 崔丽娟, 万发荣 2019 工程科学学报 41 1016
 Google Scholar
Google Scholar
Du Y F, Cui L J, Wan F R 2019 Chinese Journal of Engineering 41 1016
 Google Scholar
Google Scholar
[12] 崔丽娟, 高进, 杜玉峰, 张高伟, 张磊, 龙毅, 杨善武, 詹倩, 万发荣 2016 65 066102
 Google Scholar
Google Scholar
Cui L J, Gao J, Du Y F, Zhang G W, Zhang L, Long Y, Yang S W, Zhan Q, Wan F R 2016 Acta Phys. Sin. 65 066102
 Google Scholar
Google Scholar
[13] Gao N, Setyawan W, Kurtz Richard J, Wang Z G 2017 J. Nucl. Mater. 493 62
 Google Scholar
Google Scholar
[14] Gao N, Shen T L, Kurtz R, Wang Z G, Gao F 2017 Scrip. Mater. 136 64
 Google Scholar
Google Scholar
[15] Cui M H, Gao N, Wang D, Gao X, Wang Z G 2019 Nucl. Instr. Meth. B
 Google Scholar
Google Scholar
[16] Li F B, Ran G, Gao N, Zhao SQ, Li N 2019 Chin. Phys. B 28 085203
 Google Scholar
Google Scholar
[17] Gao N, Cui M H, Setyawan W, Kurtz R J 2018 J. Appl. Phys. 124 235105
 Google Scholar
Google Scholar
[18] Bastecka J 1964 Czechosovak J. Phys. 14 430
 Google Scholar
Google Scholar
[19] Terentyev D A, Osetsky Y N, Bacon D J 2010 Acta Mater. 58 2477
 Google Scholar
Google Scholar
[20] Wirth B D, Odette G R, Maroudas D, Lucas G E 2000 J. Nucl. Mater. 276 33
 Google Scholar
Google Scholar
[21] Osetsky Y N, Bacon D J, Serra A, Singh B N, Golubov S I 2000 J. Nucl. Mater. 276 65
 Google Scholar
Google Scholar
[22] Fikar J, Groger R 2015 Acta Mater 99 392
 Google Scholar
Google Scholar
[23] Fikar J, Groger R, Schaeublin R 2017 J. Nucl. Mater. 497 161
 Google Scholar
Google Scholar
[24] Fikar J, Groger R, Schaeublin R 2017 Nucl. Instr. Meth. B 393 186
 Google Scholar
Google Scholar
[25] Stukowski A 2010 Modell Simul Mater Sci Eng 18 015012
 Google Scholar
Google Scholar
[26] Ackland G J, Mendelev M I, Srolovitz D J, Han S, BarashevA V 2004 J. Phys.: Condens. Matter 16 S2629
 Google Scholar
Google Scholar
[27] 李国旺, 黄吝根, 杨顺华 1992 41 69
 Google Scholar
Google Scholar
Li G W, Huang L G, Yang S H 1992 Acta Phys. Sin. 41 69
 Google Scholar
Google Scholar
-
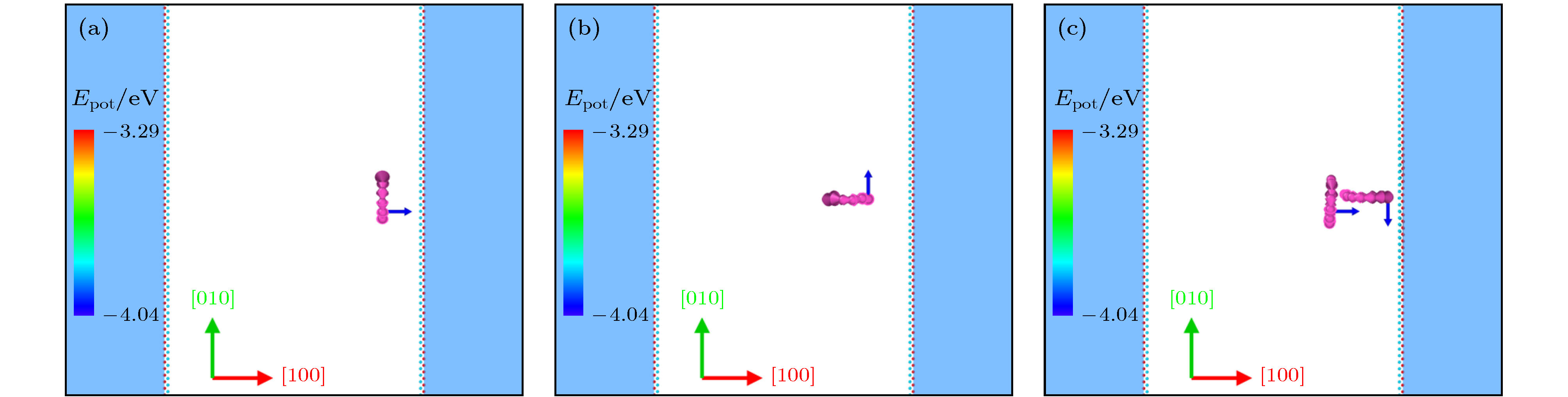
图 1
$\left\langle 100 \right\rangle $ 间隙型位错环与表面相互作用计算模型示意图 (a)和(b)单个位错环与表面的相互作用模型, 其中位错环伯格斯矢量分别平行(a)和垂直(b)于表面法线方向; (c)两个不同的具有相互作用的伯格斯矢量的$\left\langle 100 \right\rangle $ 位错环与表面相互作用的微观模型; 图中位错环和表面原子的颜色根据其势能Epot大小确定, 如图中的能量颜色棒表示, 在表面之外为真空层, 以模拟表面与位错环的相互作用过程Fig. 1. Schematic of interaction between
$\left\langle 100 \right\rangle $ loop(s) with {100} surface: (a) and (b) Interaction between a single$\left\langle 100 \right\rangle $ loop with surface with Burgers vector parallel (a) or perpendicular (b) to the normal line of surface; (c) interaction between two interacting$\left\langle 100 \right\rangle $ loops and {100} surface. The atoms in loops and surface are colored by their potential energies Epot as marked by the color bar in the Fig. 1.图 2
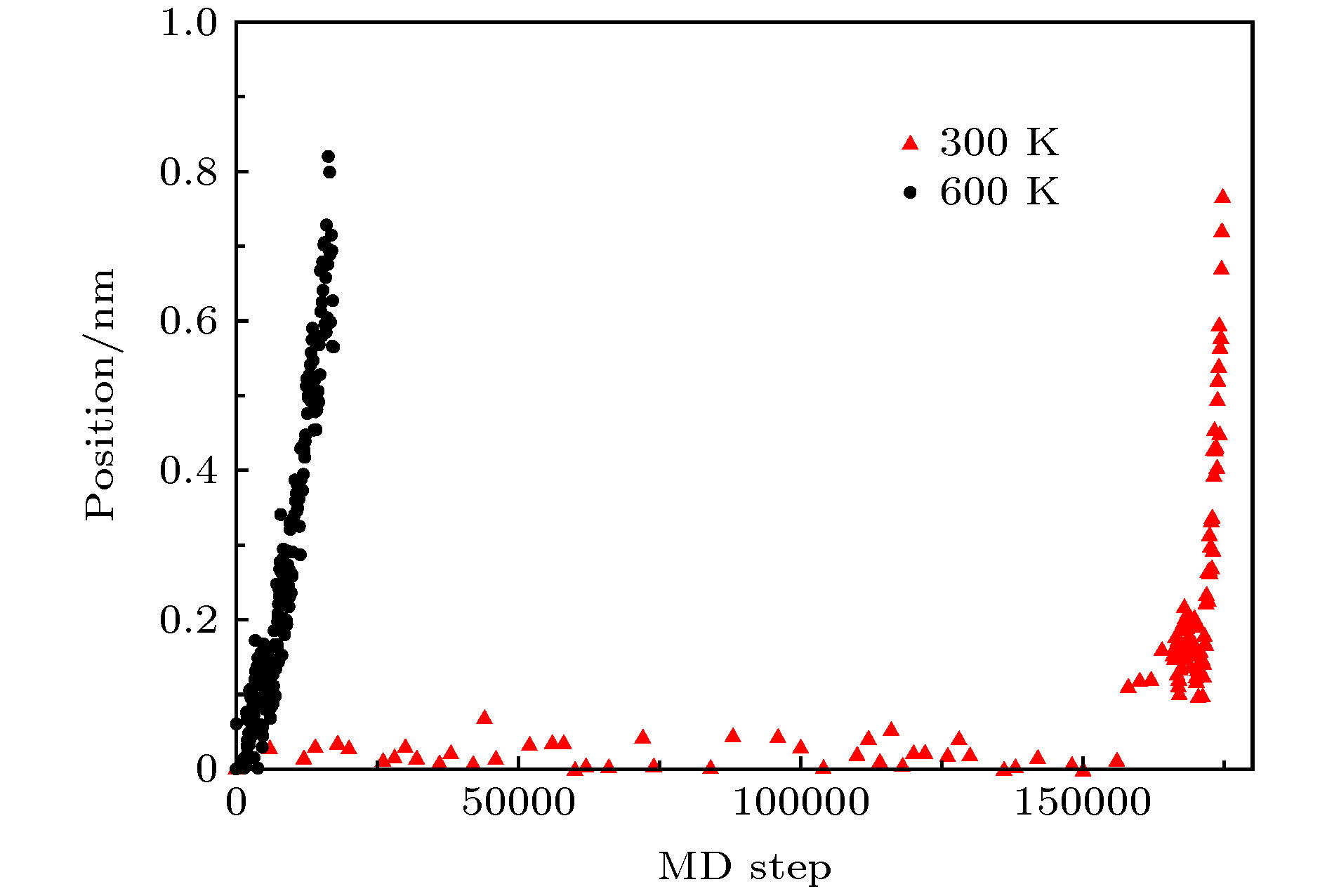
$\left\langle 100 \right\rangle $ 位错环伯格斯矢量平行于表面法线方向, 300 K时位错环的演化过程 (a)−(c)位错环向表面运动过程示意图; (d)−(g)位错环与表面发生反应过程; (h)位错环最终被表面吸收后的状态Fig. 2. Evolution of a
$\left\langle 100 \right\rangle $ dislocation loop at 300 K with its Burgers vector along the normal direction of surface of {100}. The motion processes of loop are shown in panel (a) to (c) before its interaction with surface, which are shown in panel (d) to (g). Panel (h) is the final state after the absorption of loop by surface.图 3
$\left\langle 100 \right\rangle $ 位错环伯格斯矢量垂直于表面法线方向时不同温度下位错环的演化过程 (a)温度为300 K, 模拟时间为224 ps; (b)温度为300 K、模拟时间653.6 ps; (c)温度为585 K, 模拟时间为1731.4 ps; (d)温度为740 K, 模拟时间为523.9 ps; (e)温度为970 K, 模拟时间为822.5 ps; (f)温度为1220 K, 模拟时间为129.9 ps; (g)温度为1220 K, 模拟时间为139.9 ps; (h)温度为1220 K, 模拟时间为149.9 psFig. 3. Evolution of a
$\left\langle 100 \right\rangle $ dislocation loop at different temperatures with its Burgers vector perpendicular to the normal direction of {100} surface. The results simulated at 300 K are shown in (a) and (b) with simulation time up to 224 ps and 653.6 ps, respectively. The results simulated at 585 K, 740 K and 970 K are shown in (c) to (e) with simulation time up to 1731.4 ps, 523.9 ps, 822.5 ps, respectively. The results at 1220 K with simulation time up to 129.9 ps, 139.9 ps, 149.9 ps are shown in (f) to (h), respectively.图 5 伯格斯矢量相互垂直的双位错环的演化过程 (a)−(c)模拟温度为300 K, 模拟时间分别为10 ps, 20 ps, 30 ps; (d)−(h)模拟温度为900 K, 模拟时间分别为98.5 ps, 108.4 ps, 118.3 ps, 128.1 ps, 138 ps
Fig. 5. Evolution of two
$ \left\langle 100 \right\rangle $ dislocation loops at 300 K and 900 K with Burgers vector perpendicular to each other The results obtained at 300 K are shown (a) to (c) with simulation time up to 10 ps, 20 ps, 30 ps, respectively. The results obtained at 900 K are shown (d) to (h) with simulation time up to 98.5 ps, 108.4 ps, 118.3 ps, 128.1 ps, 138 ps, respectively. -
[1] Masters B C 1965 Philos. Mag. 11 881
 Google Scholar
Google Scholar
[2] Yao Z, Hernández-Mayoral M, Jenkins M L, Kirk M A 2008 Philos. Mag. 88 2851
 Google Scholar
Google Scholar
[3] Dudarev S L, Bullough R, Derlet P M 2008 Phys. Rev. Lett. 100 135503
 Google Scholar
Google Scholar
[4] Hernández-Mayoral M, Yao Z, Jenkins M L, Kirk M A 2008 Philos. Mag. 88 2881
 Google Scholar
Google Scholar
[5] Jenkins M L, Yao Z, Hernández-Mayoral M, Kirk M A 2009 J. Nucl. Mater. 389 197
[6] Yao Z, Jenkins M L, Hernández-Mayoral M, Kirk M A 2010 Philos. Mag. 90 4623
 Google Scholar
Google Scholar
[7] Prokhodtseva A, Décamps B, Schäublin R 2013 J. Nucl. Mater. 442 S786
 Google Scholar
Google Scholar
[8] Arakawa K, Hatanaka M, Kuramoto E, Ono K, Mori H 2006 Phys. Rev. Lett. 96 125506
 Google Scholar
Google Scholar
[9] 黄依娜, 万发荣, 焦治杰 2011 60 036802
 Google Scholar
Google Scholar
Huang Y N, Wan F R, Jiao Z J 2011 Acta Phys. Sin. 60 036802
 Google Scholar
Google Scholar
[10] 姜少宁, 万发荣, 龙毅, 刘传歆, 詹倩, 大貫惣明 2013 62 166801
 Google Scholar
Google Scholar
Jiang S N, Wan F R, Long Y, Liu C X, Zhan Q, Ohnuki S 2013 Acta Phys. Sin. 62 166801
 Google Scholar
Google Scholar
[11] 杜玉峰, 崔丽娟, 万发荣 2019 工程科学学报 41 1016
 Google Scholar
Google Scholar
Du Y F, Cui L J, Wan F R 2019 Chinese Journal of Engineering 41 1016
 Google Scholar
Google Scholar
[12] 崔丽娟, 高进, 杜玉峰, 张高伟, 张磊, 龙毅, 杨善武, 詹倩, 万发荣 2016 65 066102
 Google Scholar
Google Scholar
Cui L J, Gao J, Du Y F, Zhang G W, Zhang L, Long Y, Yang S W, Zhan Q, Wan F R 2016 Acta Phys. Sin. 65 066102
 Google Scholar
Google Scholar
[13] Gao N, Setyawan W, Kurtz Richard J, Wang Z G 2017 J. Nucl. Mater. 493 62
 Google Scholar
Google Scholar
[14] Gao N, Shen T L, Kurtz R, Wang Z G, Gao F 2017 Scrip. Mater. 136 64
 Google Scholar
Google Scholar
[15] Cui M H, Gao N, Wang D, Gao X, Wang Z G 2019 Nucl. Instr. Meth. B
 Google Scholar
Google Scholar
[16] Li F B, Ran G, Gao N, Zhao SQ, Li N 2019 Chin. Phys. B 28 085203
 Google Scholar
Google Scholar
[17] Gao N, Cui M H, Setyawan W, Kurtz R J 2018 J. Appl. Phys. 124 235105
 Google Scholar
Google Scholar
[18] Bastecka J 1964 Czechosovak J. Phys. 14 430
 Google Scholar
Google Scholar
[19] Terentyev D A, Osetsky Y N, Bacon D J 2010 Acta Mater. 58 2477
 Google Scholar
Google Scholar
[20] Wirth B D, Odette G R, Maroudas D, Lucas G E 2000 J. Nucl. Mater. 276 33
 Google Scholar
Google Scholar
[21] Osetsky Y N, Bacon D J, Serra A, Singh B N, Golubov S I 2000 J. Nucl. Mater. 276 65
 Google Scholar
Google Scholar
[22] Fikar J, Groger R 2015 Acta Mater 99 392
 Google Scholar
Google Scholar
[23] Fikar J, Groger R, Schaeublin R 2017 J. Nucl. Mater. 497 161
 Google Scholar
Google Scholar
[24] Fikar J, Groger R, Schaeublin R 2017 Nucl. Instr. Meth. B 393 186
 Google Scholar
Google Scholar
[25] Stukowski A 2010 Modell Simul Mater Sci Eng 18 015012
 Google Scholar
Google Scholar
[26] Ackland G J, Mendelev M I, Srolovitz D J, Han S, BarashevA V 2004 J. Phys.: Condens. Matter 16 S2629
 Google Scholar
Google Scholar
[27] 李国旺, 黄吝根, 杨顺华 1992 41 69
 Google Scholar
Google Scholar
Li G W, Huang L G, Yang S H 1992 Acta Phys. Sin. 41 69
 Google Scholar
Google Scholar
计量
- 文章访问数: 9692
- PDF下载量: 102
- 被引次数: 0
























 下载:
下载: