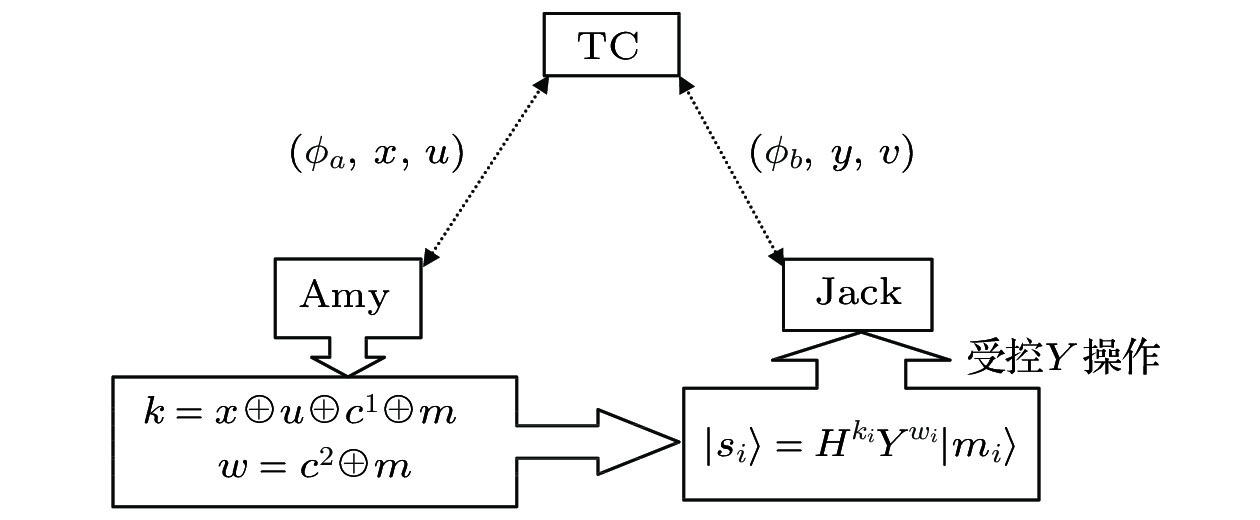
-
Most of the classical designated verifier signature schemes are insecure against quantum adversary. In this paper, a quantum signature scheme for the designated verifier is proposed. In our scheme, during the initialization phase, the partners share secret keys by performing the quantum key distribution protocol. On the other hand, by performing the quantum direct communication protocol, the key generator center shares secret keys with the signer and the designated verifier, respectively. The key generator center generates a particle sequence of Bell state and distributes the particles between the signer and the designated verifier. During the signature generation phase, the signer encrypts the particle sequence by the secret keys and Hardmard operators. After that, the signer performs the controlled unitary operations on the encrypted particle sequence so as to generate the quantum signature. The designated verifier can simulate the quantum signature by performing the same symmetric signing steps as that performed by the original signer. Hence, the quantum signature signed by the true signer is the same as the one simulated by the receiver, which makes our scheme possess the designated properties. During the signature verification phase, the designated verifier performs the controlled unitary operations on the quantum signature and obtains the quantum ciphertexts. After that, the designated verifier decrypts the quantum ciphertexts by the symmetric secret keys and Hardmard operators so that the quantum signature can be verified. Our signature is secure against forgery attack, inter-resending attacks and Trojan horse attack. Because the trace distance between the density operators of different quantum signatures is zero, the information-theoretical security of our quantum signature scheme can be proved. The unconditionally secure quantum key distribution protocol and the one-time pad encryption algorithm can guarantee the security of the secret keys shared by the partners. What is more, the security assumption about the key generation center is weak. That is, it is not necessary to assume that the key generation center should be fully trusted. On the other hand, in our scheme, the quantum one-way function is not used. To generate a quantum signature, the signer need not prepare for entangled particle sequence. To verify a quantum signature, the verifier need not apply any state comparison to the received particles. The qubit efficiency is 100%. Therefore, our scheme has the advantages in the security and efficiency over the other quantum signature schemes for the designated verifier.
-
Keywords:
- quantum signature /
- security /
- Bell state /
- non-transferability
[1] Diffie W, Hellmann M 1976 IEEE IT 22 644
Google Scholar
[2] Saeednia S, Kremer S, Markowitch O 2003 Information Security and Cryptology-ICISC Seoul, Korea, November 27–28, 2003 p40
[3] Ray I, Narasimhamurthi N 2001 Proceedings of the 3rd international workshop on advanced issues of E-commerce and web-based information systems San Juan, CA, USA, June 21–22, 2001 p188
[4] Schoenmakers B 1999 Advances in CRYPTO’99 Santa Barbara, California, USA, August 15–19, 1999 p148
[5] Huang X, Mu Y, Susilo W, Wu W 2007 Proceedings of 1st International Conference on Pairing-Based Cryptography, Pairing 2007 Tokyo, Japan, July 2–4, 2007 p367
[6] Wang B, Song Z 2009 Inf. Sci. 179 858
Google Scholar
[7] Jakobsson M, Sako K, Impagliazzo R 1996 Advances in Cryptology-Eurocrypt 1996 Santa Barbara, California, USA, August 18–22, 1996 p142
[8] Kang B, Boyd C, Dawson E 2009 J. Syst. Software 82 270
Google Scholar
[9] Lee J, Chang J, Lee D 2010 Comput. Electr. Eng. 36 948
Google Scholar
[10] Hafizul I S, Biswas G P 2015 Arab. J. Sci. Eng. 40 1069
Google Scholar
[11] Rastegari P, Susilo W, Dakhilalian M 2019 Int. J. Theor. Phys. 18 619
Google Scholar
[12] Shor P W 1997 SIAM J. Comput. 26 1484
Google Scholar
[13] Gottesman D, Chuang I 2001 arxiv: quant-ph/0105032 v2
[14] Zeng G H, Keitel C H 2002 Phys. Rev. A. 65 042312
Google Scholar
[15] Yang Y G, Lei H, Liu Z C, Zhou Y H, Shi W M 2016 Quantum Inf. Process. 15 2487
Google Scholar
[16] Yang Y G, Zhou Z, Teng Y W, Wen Q Y 2010 Eur. Phys. J. D 61 773
Google Scholar
[17] Xin X, He Q, Wang Z, Yang Q, Li F 2019 Optik 189 23
Google Scholar
[18] Wang M Q, Wang X, Zhan T 2018 Quantum Inf. Process. 17 275
Google Scholar
[19] Xin X, Wang Z, Yang Q 2019 Appl. Opt. 58 7346
Google Scholar
[20] Jiang D H, Xu Y L, Xu G B 2019 Int. J. Theor. Phys. 58 1036
Google Scholar
[21] Ma H, Li F, Mao N, Guo Y 2017 Int. J. Theor. Phys. 56 2551
Google Scholar
[22] Zhang J L, Zhang J Z, Xie S C 2018 Int. J. Theor. Phys. 57 1612
Google Scholar
[23] Zeng G, Lee M, Guo Y, He G 2007 Int. J. Quantum Inf. 5 553
Google Scholar
[24] Guo Y, Feng Y 2016 Int. J. Quantum Inf. 55 2290
[25] Shi W M, Zhou Y H, Yang Y G 2015 Int. J. Theor. Phys. 54 3115
Google Scholar
[26] Shi W M, Wang Y M, Zhou Y H, Yang Y G, Zhang J B 2018 Optik 164 753
Google Scholar
[27] Menezes A J, Oorschot P V, Vanstone S A 1996 Handbook of Applied Cryptography (Boca Raton: CRC Press) p41
[28] Yang L, Yang B, Pan J 2012 SPIE Photonics Europe Belgium, April 16–19, 2012 p8440E1
[29] Yang L, Xiang C, Li B 2013 Chin. Commun. 10 19
Google Scholar
[30] Xin X, Wang Z, Yang Q, Li F 2020 Int. J. Theor. Phys. 59 918
Google Scholar
[31] Shannon C E 1949 Bell Syst. Tech. J. 28 656
Google Scholar
[32] Bennett C H, Brassard G 2014 Theor. Comput. Sci. 560 7
Google Scholar
[33] Long G L, Liu X S 2002 Phys. Rev. A 65 2302
Google Scholar
[34] Hu Y G 2018 Int. J. Theor. Phys. 57 2831
Google Scholar
[35] Yan L, Sun Y, Chang Y, Zhang S, Wan G, Sheng Z 2018 Quantum Inf. Process. 17 315
Google Scholar
[36] Deng F G, Long G L, Liu X S 2003 Phys. Rev. A 68 042317
Google Scholar
[37] Gottesman D, Lo H K, Lütkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[38] Hwang W Y 2003 Phys. Rev. Lett. 91 057901
Google Scholar
[39] Lo H K, Ma X, Chen K 2005 Phys. Rev. Lett. 94 230504
Google Scholar
[40] Wang X B 2005 Phys. Rev. Lett. 94 230503
Google Scholar
[41] Lu H, Fung C H F, Ma X, Cai Q 2011 Phys. Rev. A 84 042344
Google Scholar
[42] Fung C H F, Ma X, Chau H F, Cai Q 2012 Phys. Rev. A 85 032308
Google Scholar
[43] Beaudry N J, Lucamarini M, Mancini S, Renner R 2013 Phys. Rev. A 88 062302
Google Scholar
[44] Hwang T, Lee K C 2007 IET Inf. Secur. 1 43
Google Scholar
[45] Shi W M, Zhou Y H, Yang U G 2015 International Journal of Theoretical Physics volume 54 3115
[46] 宋云 2019 电子学报 47 1443
Google Scholar
Song Y 2019 Acta Electr. Sin. 47 1443
Google Scholar
-
图 1 初始化和QSDV的生成过程
Figure 1. Initialization and QSDV generation.
-
[1] Diffie W, Hellmann M 1976 IEEE IT 22 644
Google Scholar
[2] Saeednia S, Kremer S, Markowitch O 2003 Information Security and Cryptology-ICISC Seoul, Korea, November 27–28, 2003 p40
[3] Ray I, Narasimhamurthi N 2001 Proceedings of the 3rd international workshop on advanced issues of E-commerce and web-based information systems San Juan, CA, USA, June 21–22, 2001 p188
[4] Schoenmakers B 1999 Advances in CRYPTO’99 Santa Barbara, California, USA, August 15–19, 1999 p148
[5] Huang X, Mu Y, Susilo W, Wu W 2007 Proceedings of 1st International Conference on Pairing-Based Cryptography, Pairing 2007 Tokyo, Japan, July 2–4, 2007 p367
[6] Wang B, Song Z 2009 Inf. Sci. 179 858
Google Scholar
[7] Jakobsson M, Sako K, Impagliazzo R 1996 Advances in Cryptology-Eurocrypt 1996 Santa Barbara, California, USA, August 18–22, 1996 p142
[8] Kang B, Boyd C, Dawson E 2009 J. Syst. Software 82 270
Google Scholar
[9] Lee J, Chang J, Lee D 2010 Comput. Electr. Eng. 36 948
Google Scholar
[10] Hafizul I S, Biswas G P 2015 Arab. J. Sci. Eng. 40 1069
Google Scholar
[11] Rastegari P, Susilo W, Dakhilalian M 2019 Int. J. Theor. Phys. 18 619
Google Scholar
[12] Shor P W 1997 SIAM J. Comput. 26 1484
Google Scholar
[13] Gottesman D, Chuang I 2001 arxiv: quant-ph/0105032 v2
[14] Zeng G H, Keitel C H 2002 Phys. Rev. A. 65 042312
Google Scholar
[15] Yang Y G, Lei H, Liu Z C, Zhou Y H, Shi W M 2016 Quantum Inf. Process. 15 2487
Google Scholar
[16] Yang Y G, Zhou Z, Teng Y W, Wen Q Y 2010 Eur. Phys. J. D 61 773
Google Scholar
[17] Xin X, He Q, Wang Z, Yang Q, Li F 2019 Optik 189 23
Google Scholar
[18] Wang M Q, Wang X, Zhan T 2018 Quantum Inf. Process. 17 275
Google Scholar
[19] Xin X, Wang Z, Yang Q 2019 Appl. Opt. 58 7346
Google Scholar
[20] Jiang D H, Xu Y L, Xu G B 2019 Int. J. Theor. Phys. 58 1036
Google Scholar
[21] Ma H, Li F, Mao N, Guo Y 2017 Int. J. Theor. Phys. 56 2551
Google Scholar
[22] Zhang J L, Zhang J Z, Xie S C 2018 Int. J. Theor. Phys. 57 1612
Google Scholar
[23] Zeng G, Lee M, Guo Y, He G 2007 Int. J. Quantum Inf. 5 553
Google Scholar
[24] Guo Y, Feng Y 2016 Int. J. Quantum Inf. 55 2290
[25] Shi W M, Zhou Y H, Yang Y G 2015 Int. J. Theor. Phys. 54 3115
Google Scholar
[26] Shi W M, Wang Y M, Zhou Y H, Yang Y G, Zhang J B 2018 Optik 164 753
Google Scholar
[27] Menezes A J, Oorschot P V, Vanstone S A 1996 Handbook of Applied Cryptography (Boca Raton: CRC Press) p41
[28] Yang L, Yang B, Pan J 2012 SPIE Photonics Europe Belgium, April 16–19, 2012 p8440E1
[29] Yang L, Xiang C, Li B 2013 Chin. Commun. 10 19
Google Scholar
[30] Xin X, Wang Z, Yang Q, Li F 2020 Int. J. Theor. Phys. 59 918
Google Scholar
[31] Shannon C E 1949 Bell Syst. Tech. J. 28 656
Google Scholar
[32] Bennett C H, Brassard G 2014 Theor. Comput. Sci. 560 7
Google Scholar
[33] Long G L, Liu X S 2002 Phys. Rev. A 65 2302
Google Scholar
[34] Hu Y G 2018 Int. J. Theor. Phys. 57 2831
Google Scholar
[35] Yan L, Sun Y, Chang Y, Zhang S, Wan G, Sheng Z 2018 Quantum Inf. Process. 17 315
Google Scholar
[36] Deng F G, Long G L, Liu X S 2003 Phys. Rev. A 68 042317
Google Scholar
[37] Gottesman D, Lo H K, Lütkenhaus N, Preskill J 2004 Quantum Inf. Comput. 4 325
Google Scholar
[38] Hwang W Y 2003 Phys. Rev. Lett. 91 057901
Google Scholar
[39] Lo H K, Ma X, Chen K 2005 Phys. Rev. Lett. 94 230504
Google Scholar
[40] Wang X B 2005 Phys. Rev. Lett. 94 230503
Google Scholar
[41] Lu H, Fung C H F, Ma X, Cai Q 2011 Phys. Rev. A 84 042344
Google Scholar
[42] Fung C H F, Ma X, Chau H F, Cai Q 2012 Phys. Rev. A 85 032308
Google Scholar
[43] Beaudry N J, Lucamarini M, Mancini S, Renner R 2013 Phys. Rev. A 88 062302
Google Scholar
[44] Hwang T, Lee K C 2007 IET Inf. Secur. 1 43
Google Scholar
[45] Shi W M, Zhou Y H, Yang U G 2015 International Journal of Theoretical Physics volume 54 3115
[46] 宋云 2019 电子学报 47 1443
Google Scholar
Song Y 2019 Acta Electr. Sin. 47 1443
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7265
- PDF Downloads: 82
- Cited By: 0
