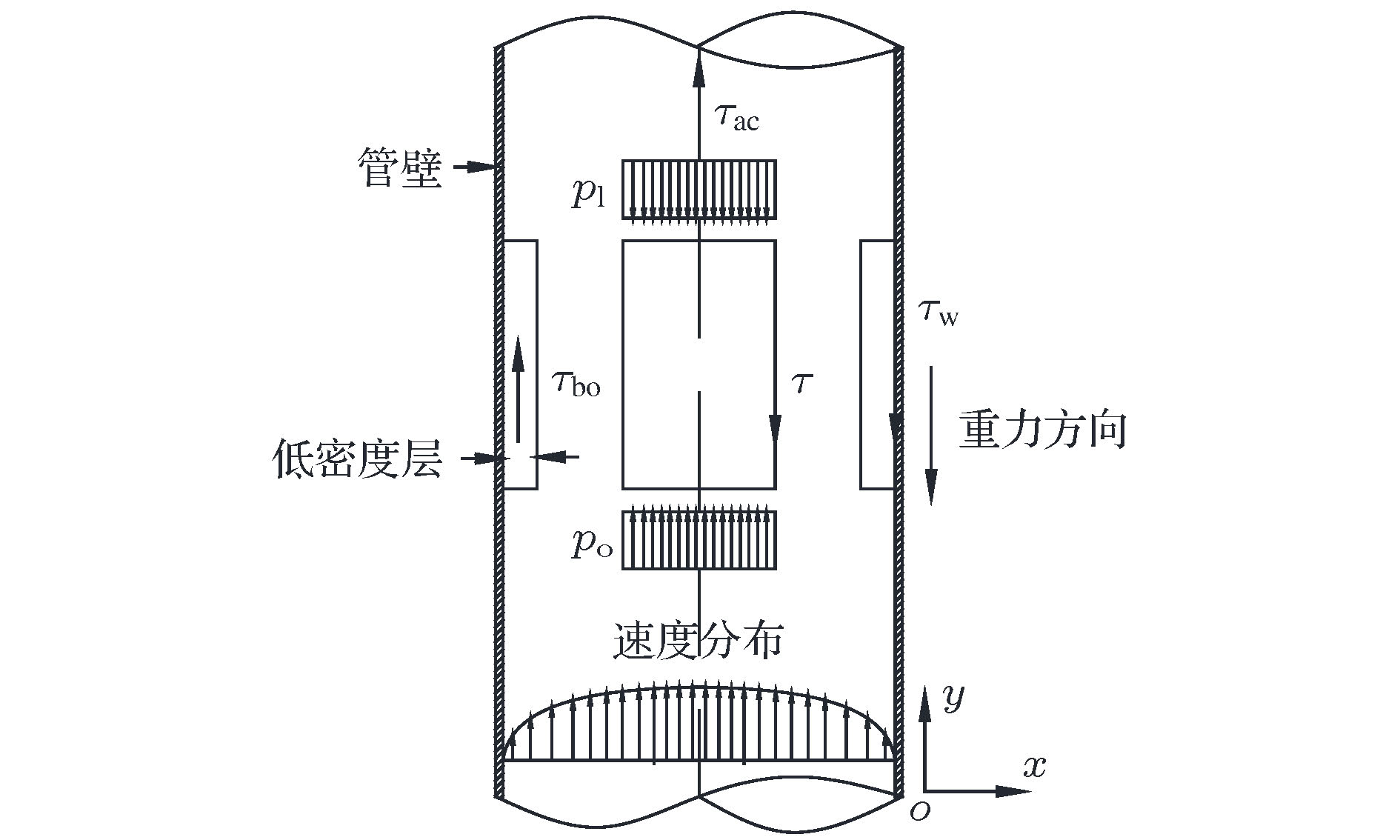
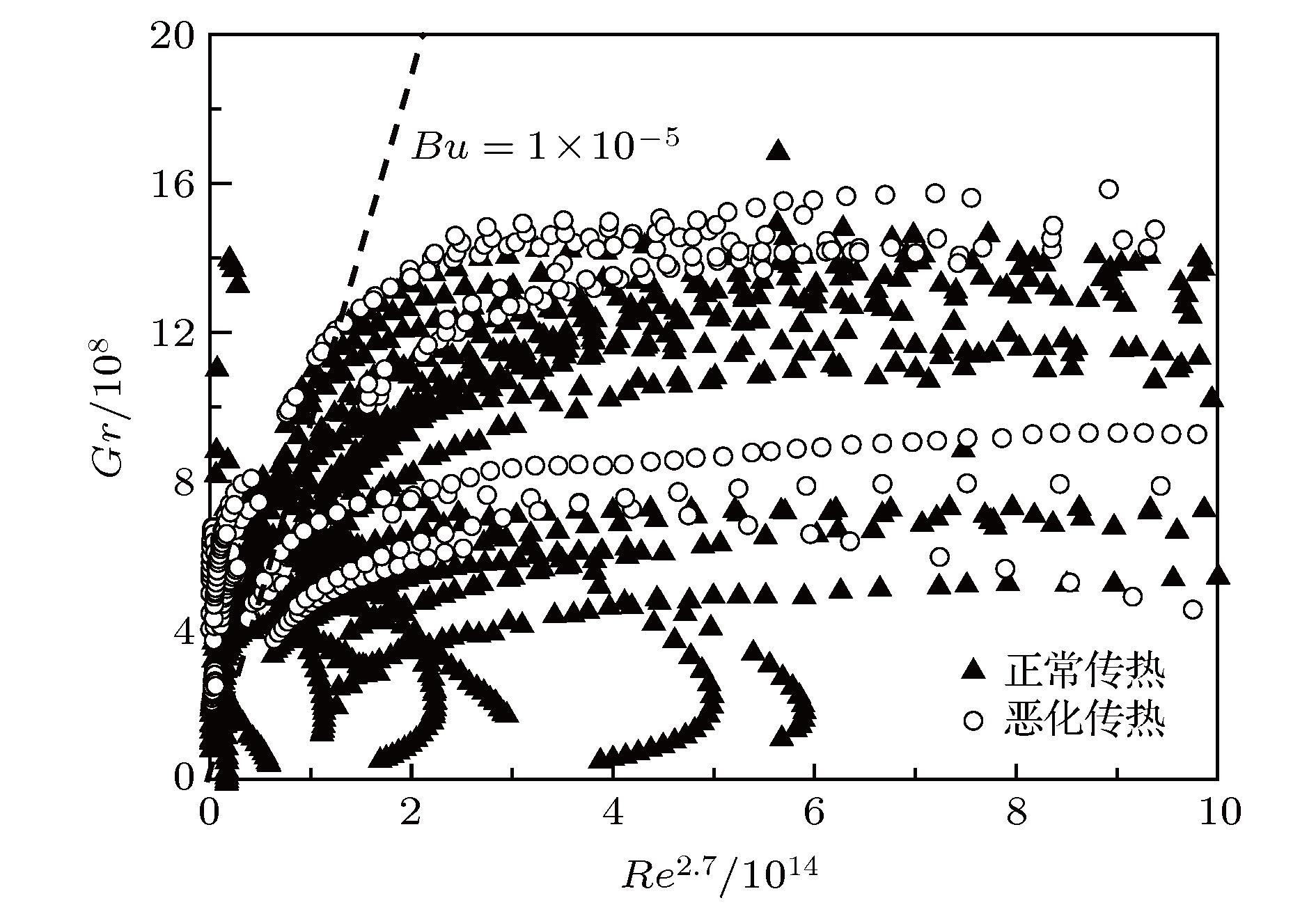
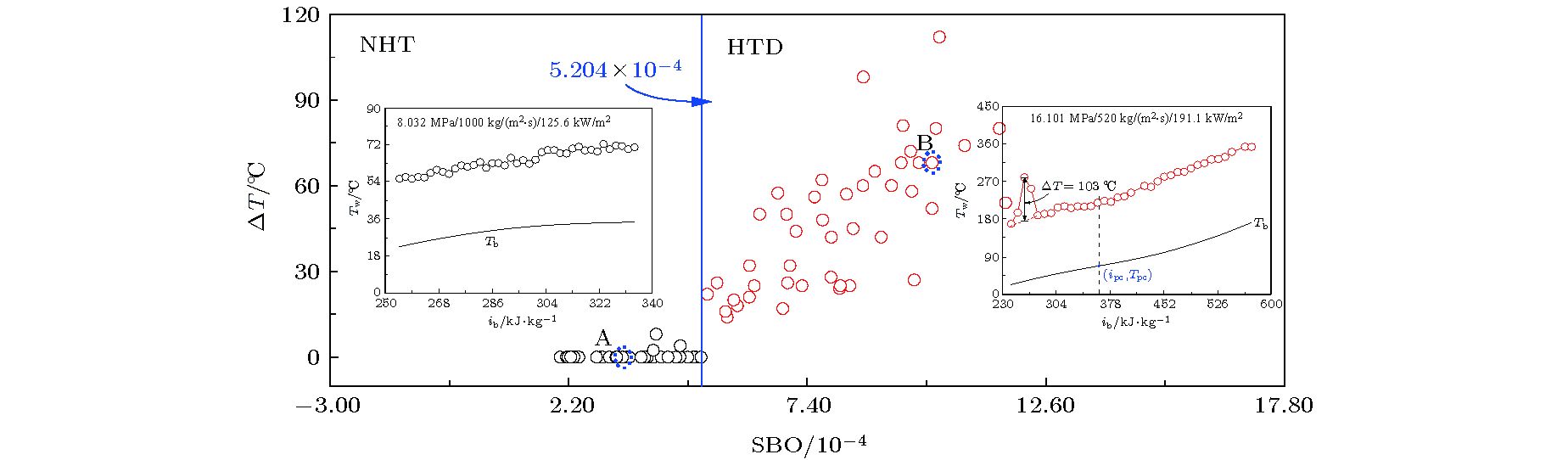
-
Based on the concept of single-phase fluid, the abnormal heat transfer behavior of supercritical fluid has been investigated for many years. However, there is no unified understanding of the mechanism of its flow and heat transfer. In this paper, we first review the reported effects of buoyancy and acceleration on supercritical fluids, and then study the effects of buoyancy and acceleration on the flow structure and heat transfer for the upward vertically flowing of supercritical CO2 fluid in a tube theoretically and experimentally. The results show that there is no conclusive experimental evidence that the abnormal heat transfer behavior of supercritical fluid is directly related to buoyancy and flow acceleration, and the existing criteria for estimating buoyancy and acceleration effect are based on the constant physical fluid and a lot of assumptions, as a result, different conclusions are obtained, though the same prediction method is used. Finally, we investigate the heat transfer deterioration of supercritical fluids based on the pseudo-boiling theory, and the proposed supercritical-boiling-number distinguishes the normal heat transfer deterioration from heat transfer deterioration of supercritical fluid. Our work paves a new way to understanding the supercritical fluid flow and heat transfer mechanism. The supercritical-boiling-number is important for establishing the optimum operating conditions for the supercritical fluid power cycle used in different technologies.
-
Keywords:
- supercritical carbon dioxide /
- heat transfer deterioration /
- buoyancy /
- acceleration /
- pseudo-boiling
[1] Crespi F, Gavagnin G, Sánchez, David, Martinez, Gonzalo S 2017 Appl. Energy 195 152
Google Scholar
[2] Xu J L, Sun E H, Li M J, Liu H, Zhu B G 2018 Energy 157 227
Google Scholar
[3] Ehsan M M, Guan Z, Klimenko A Y 2018 Renewable Sustainale Energy Rev. 92 658
Google Scholar
[4] Shiralkar, B S, Griffith P 1969 J. Heat Transfer 91 27
Google Scholar
[5] Bourke P J, Pulling D J, Gill L E, Denton, W H 1970 Int. J. Heat Mass Transfer 13 1339
Google Scholar
[6] Bae Y Y 2011 Nucl. Eng. Des. 241 3164
Google Scholar
[7] Brassington D J, Cairns D N H 1977 Int. J. Heat Mass Transfer 20 207
Google Scholar
[8] Hall W B, Jackson J D 1978 Advances in Heat Transfer 7 1
Google Scholar
[9] Mceligot D M, Coon C W, Perkins H C 1970 Int. J. Heat Mass Transfer 13 431
Google Scholar
[10] Liu S H, Huang Y P, Liu G X, Wang J F, Leung L K 2017 Int. J. Heat Mass Transfer 106 1144
Google Scholar
[11] Huang D, Wu Z, Sunden B, Li W 2016 Appl. Energy 162 494
Google Scholar
[12] Dang G X, Zhong F Q, Chen L H, Chang X Y 2013 Sci. China Technol. Sci. 56 416
Google Scholar
[13] Bruch A, Bontemps A, Colasson S 2009 Int. J. Heat Mass Transfer 52 2589
Google Scholar
[14] Liao S M, Zhao T S 2002 Int. J. Heat Mass Transfer 45 5025
Google Scholar
[15] Kim D E 2011 Int. J. Heat Fluid Flow 32 176
Google Scholar
[16] 徐肖肖, 吴杨杨, 刘朝, 王开正, 叶建 2015 64 054401
Google Scholar
Xu X X, Wu Y Y, Liu C, Wang K Z, Ye J 2015 Acta Phys. Sin. 64 054401
Google Scholar
[17] Kurganov V A, Kaptilnyi A G 1993 Int. J. Heat Mass Transfer 36 3383
Google Scholar
[18] Simeoni G G, Bryk T, Gorelli F A Krisch M, Ruocco G, Santoro M, Scopigno T 2010 Nat. Phys. 6 503
Google Scholar
[19] Gorelli F A, Bryk T, Krisch M, Ruocco G, Santoro M, Scopigno T 2013 Sci. Rep. 3 120
Google Scholar
[20] Banuti D T 2015 J. Supercrit. Fluids 98 12
Google Scholar
[21] Zhang Q, Li H X, Lei X L, Zhang J, Kong X F 2018 Int. J. Heat Mass Transfer 127 674
Google Scholar
[22] Kandlikar S G 2004 J. Heat Transfer 126 8
Google Scholar
-
图 1 实验系统图
Figure 1. Experiment setup.
图 2 实验段
Figure 2. Vertically positioned test tube.
图 3 圆管中简单的二维流动几何模型
Figure 3. Vertically positioned test tube.
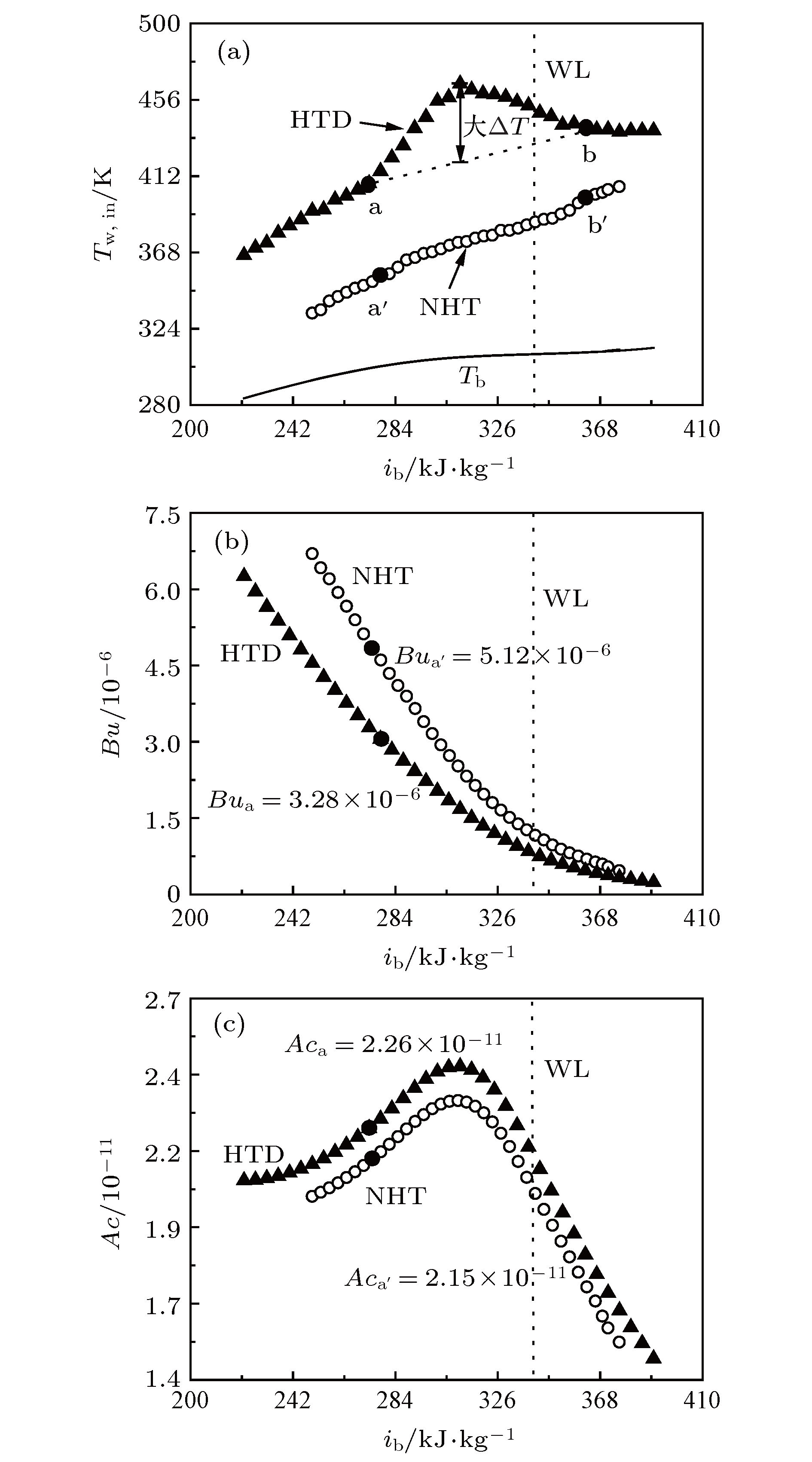
图 4 局部壁温Tw,in, Bu, Ac随主流焓值ib的分布关系 (a) P = 8.220 MPa, G = 200 kg/(m2·s), qw = 60 kW/m2, (b) P = 8.220 MPa, G = 520 kg/(m2·s), qw = 42 kW/m2
Figure 4. Local inner wall (Tw, in), Bu, Ac distributions with bulk fluid enthalpy (ib): (a) P = 8.220 MPa, G = 200 kg/(m2·s), qw = 60 kW/m2, (b) P = 8.220 MPa, G = 520 kg/(m2·s), qw = 42 kW/m2.
图 6 不同质量流速下(a)局部壁温Tw,in, (b) Bu, (c) Ac随主流焓值ib的分布(NHT, 正常传热; HTD, 恶化传热)
Figure 6. (a) Local inner wall Tw, in, (b) Bu, (c) Ac distributions with bulk fluid enthalpy ib (NHT, normal heat transfer; HTD, heat transfer deterioration).
图 5 局部壁温Tw,in, Bu, Ac随主流焓值ib的分布关系 (a) P = 8.220 MPa, G = 700 kg/(m2·s), qw = 245 kW/m2, (b) P = 8.220 MPa, G = 1000 kg/(m2·s), qw = 245 kW/m2
Figure 5. Local inner wall (Tw, in), Bu, Ac distributions with bulk fluid enthalpy (ib): (a) P = 8.220 MPa, G = 700 kg/(m2·s), qw = 245 kW/m2, (b) P = 8.220 MPa, G = 1000 kg/(m2·s), qw = 245 kW/m2.
图 7 不同质量流速下的Gr和Re2.7分布关系
Figure 7. Gr and Re2.7 distribution at different mass flow rates
图 8 基于拟沸腾理论的超临界流体径向膨胀模型
Figure 8. Radial expansion model of supercritical fluids based on pseudo-boiling.
图 9 超临界沸腾数区分两个传热机制
Figure 9. Supercritical boiling number distinguishes the two regimes of heat transfer.
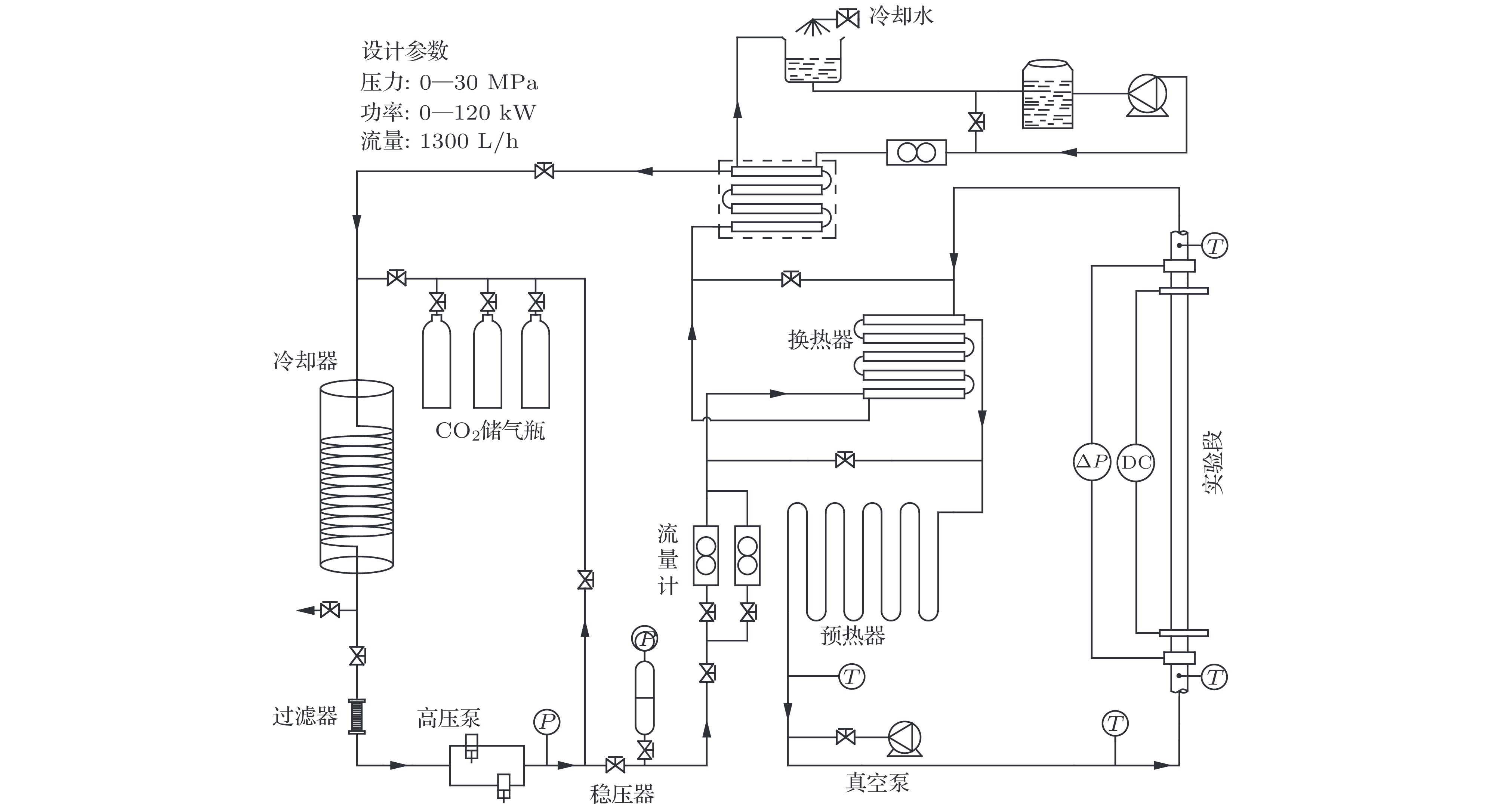
表 1 测量仪器的精度和范围
Table 1. Accuracies and ranges of measuring instruments.
参数 范围 不确定度 压力p/MPa 7.510—25.231 ± 1.42% 进口温度 Tin/℃ 5—70 ± 0.75% 出口温度 Tout/℃ 25—500 ± 0.75% 外壁面温度 Tw,o/℃ 30—450 ± 0.75% 质量流速 G/kg·m–2·s–1 488—2000 ± 2.05% 热流密度qw/kW·m–2 30—400.36 ± 8.06% 
DownLoad: CSV
-
[1] Crespi F, Gavagnin G, Sánchez, David, Martinez, Gonzalo S 2017 Appl. Energy 195 152
Google Scholar
[2] Xu J L, Sun E H, Li M J, Liu H, Zhu B G 2018 Energy 157 227
Google Scholar
[3] Ehsan M M, Guan Z, Klimenko A Y 2018 Renewable Sustainale Energy Rev. 92 658
Google Scholar
[4] Shiralkar, B S, Griffith P 1969 J. Heat Transfer 91 27
Google Scholar
[5] Bourke P J, Pulling D J, Gill L E, Denton, W H 1970 Int. J. Heat Mass Transfer 13 1339
Google Scholar
[6] Bae Y Y 2011 Nucl. Eng. Des. 241 3164
Google Scholar
[7] Brassington D J, Cairns D N H 1977 Int. J. Heat Mass Transfer 20 207
Google Scholar
[8] Hall W B, Jackson J D 1978 Advances in Heat Transfer 7 1
Google Scholar
[9] Mceligot D M, Coon C W, Perkins H C 1970 Int. J. Heat Mass Transfer 13 431
Google Scholar
[10] Liu S H, Huang Y P, Liu G X, Wang J F, Leung L K 2017 Int. J. Heat Mass Transfer 106 1144
Google Scholar
[11] Huang D, Wu Z, Sunden B, Li W 2016 Appl. Energy 162 494
Google Scholar
[12] Dang G X, Zhong F Q, Chen L H, Chang X Y 2013 Sci. China Technol. Sci. 56 416
Google Scholar
[13] Bruch A, Bontemps A, Colasson S 2009 Int. J. Heat Mass Transfer 52 2589
Google Scholar
[14] Liao S M, Zhao T S 2002 Int. J. Heat Mass Transfer 45 5025
Google Scholar
[15] Kim D E 2011 Int. J. Heat Fluid Flow 32 176
Google Scholar
[16] 徐肖肖, 吴杨杨, 刘朝, 王开正, 叶建 2015 64 054401
Google Scholar
Xu X X, Wu Y Y, Liu C, Wang K Z, Ye J 2015 Acta Phys. Sin. 64 054401
Google Scholar
[17] Kurganov V A, Kaptilnyi A G 1993 Int. J. Heat Mass Transfer 36 3383
Google Scholar
[18] Simeoni G G, Bryk T, Gorelli F A Krisch M, Ruocco G, Santoro M, Scopigno T 2010 Nat. Phys. 6 503
Google Scholar
[19] Gorelli F A, Bryk T, Krisch M, Ruocco G, Santoro M, Scopigno T 2013 Sci. Rep. 3 120
Google Scholar
[20] Banuti D T 2015 J. Supercrit. Fluids 98 12
Google Scholar
[21] Zhang Q, Li H X, Lei X L, Zhang J, Kong X F 2018 Int. J. Heat Mass Transfer 127 674
Google Scholar
[22] Kandlikar S G 2004 J. Heat Transfer 126 8
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 9294
- PDF Downloads: 169
- Cited By: 0
