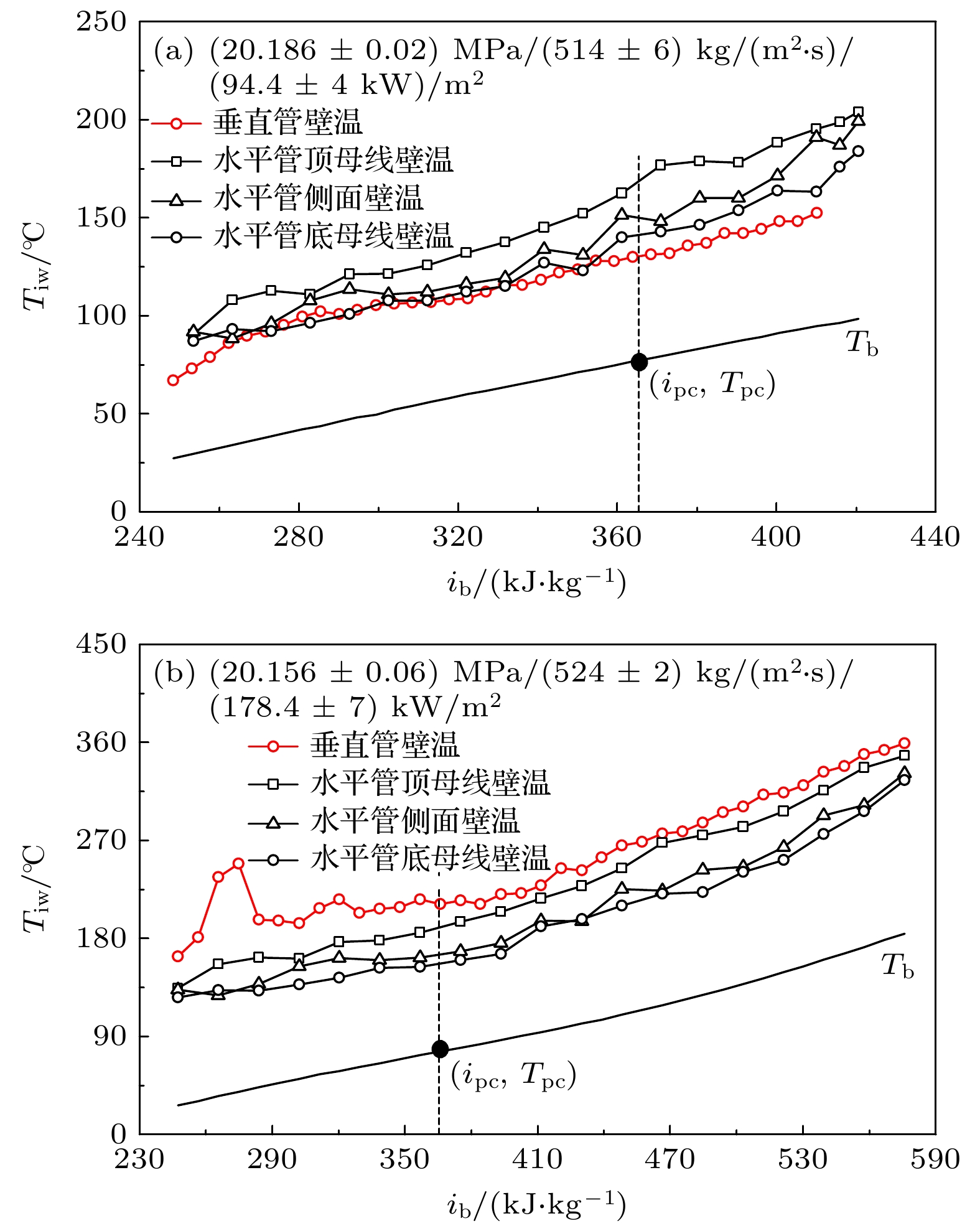
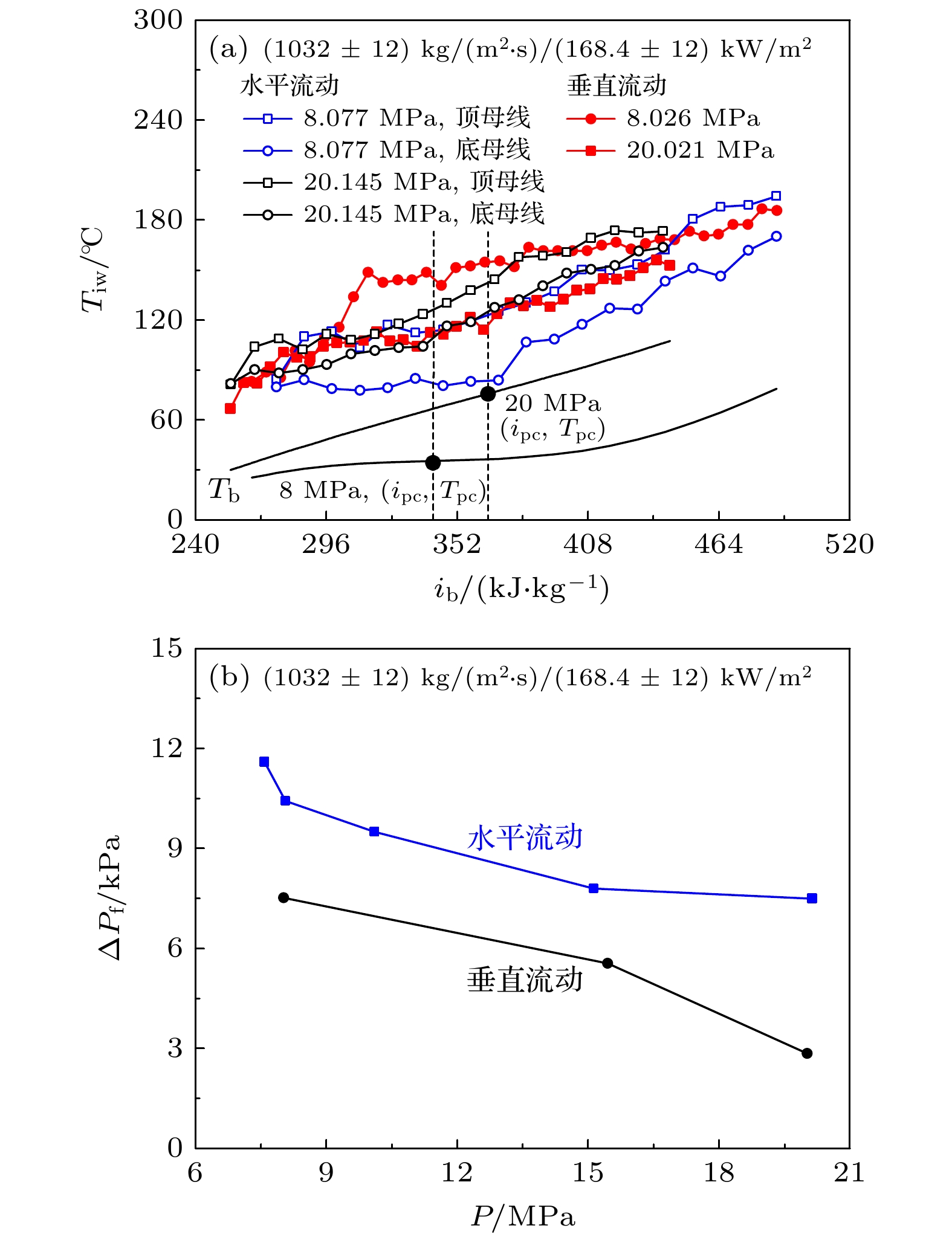
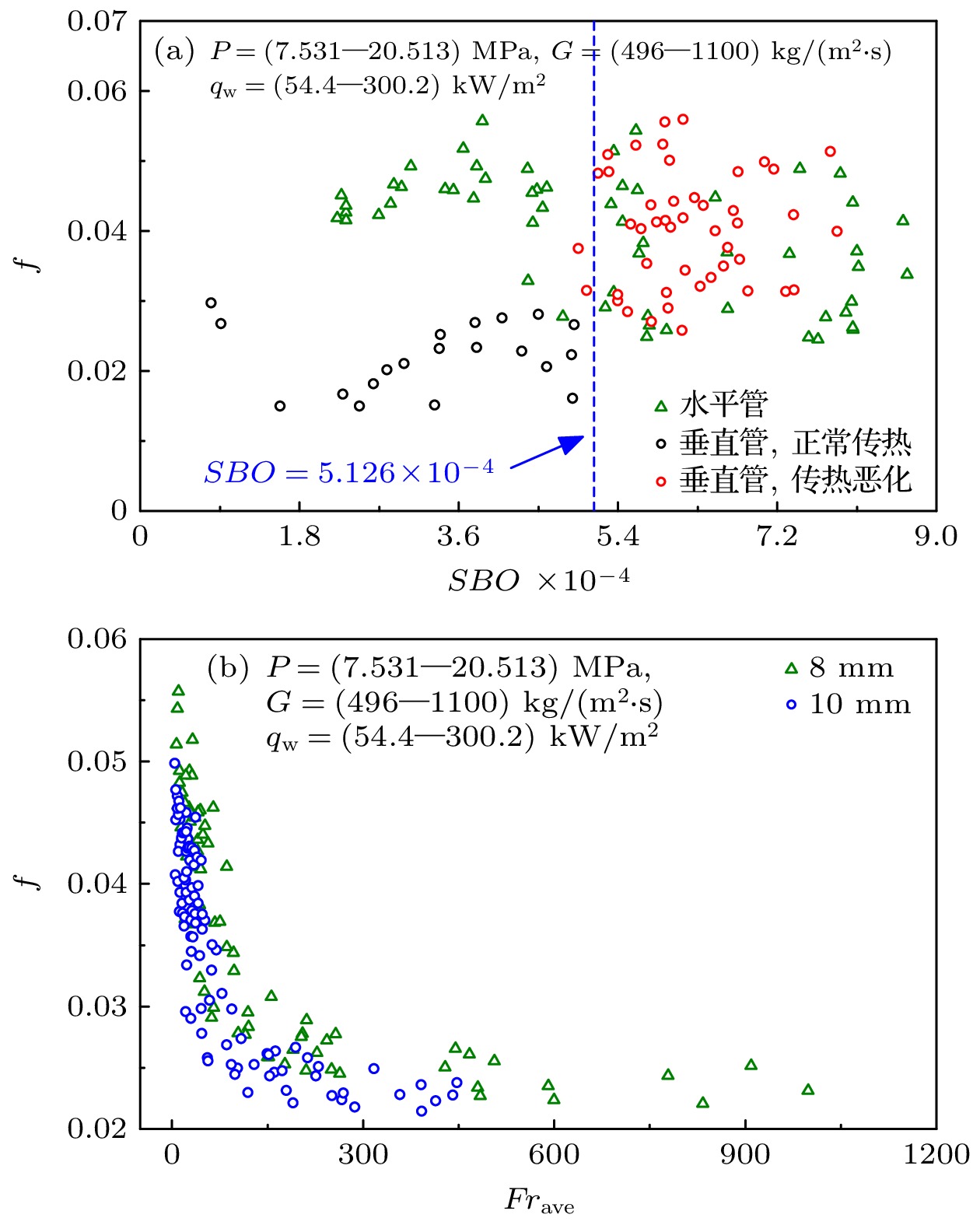
-
本文基于拟沸腾理论研究了超临界二氧化碳(sCO2)在水平和垂直向上管中的流动和传热特性差异. 比较了不同质量流量、热流密度和压力下水平管与垂直向上管的流动和换热特性差异. 与以往超临界流体的经典单相流体假设不同, 本文引入拟沸腾理论来处理sCO2在两管中的流动和传热, 将超临界流体视为多相结构, 包括近壁区的类气层和管芯中的类液流体. 结果发现, 传热方面, 在正常传热模式下垂直向上管内壁温和水平管底母线内壁温基本一致. 当垂直向上管发生传热恶化时, 垂直向上管的壁温峰值会随着超临界沸腾数(SBO)的增大超过对应焓值位置的水平管顶母线内壁温. 垂直向上管中SBO区分了正常传热和传热恶化. 而在水平管中, 当弗劳得数小于100时, SBO主导顶底壁面最大壁温差. 相比于垂直向上管, 相同压力下的超临界流体在水平管内发生传热恶化需要更高的热流密度和质量流量的比值. 流动方面, 引起垂直向上管压降斜率增高的机理是孔口收缩效应. 主导水平管压降变化的机理是分层效应, 并用弗劳得数在水平管中顶底壁温差异与压降之间建立联系.This work is devoted to investigating the difference in flow and heat transfer characteristics between vertical upward flow and horizontal flow of supercritical carbon dioxide (
$\rm sCO_2$ ) based on the pseudo-boiling theory and the experimental parameters: mass flux G = 496–1100 kg/m2s, heat flux qw = 54.4–300.2 kW/m2, and pressure P = 7.531–20.513 MPa. The differences in flow and heat transfer characteristics between horizontal upward tube and vertical upward tube are compared at different mass fluxes, heat fluxes and pressures fully. Finally, unlike the classical treatment of flow and heat transfer for supercritical fluid, single-phase fluid assumption is abandoned, instead, the pseudo-boiling theory is introduced to deal with the flow transfer and heat transfer of$\rm sCO_2 $ in the two tubes. Supercritical fluid is regarded as a multiphase structure in this work, including a vapor-like layer near the wall and a liquid-like fluid in tube core. The results are indicated below. 1) In terms of heat transfer, the inner-wall temperature of the vertical upward tube and the bottom generatrix of horizontal tube are basically the same under normal heat transfer mode. When the heat transfer deterioration occurs in the vertical upward tube, larger supercritical boiling number (SBO) will cause the wall temperature peak of the vertical upward tube to be much higher than the wall temperature at top generatrix of the horizontal tube at the corresponding enthalpy. The SBO (SBO = 5.126×10–4) distinguishes between normal heat transfer deterioration and heat transfer deterioration in the vertical upward tube. In the horizontal tubes, SBO dominates the maximum wall temperature difference between the top generatrix and the bottom generatrix. Comparing with vertical upward tubes, higher qw/G is required for the heat transfer deterioration of supercritical fluid in the horizontal tubes under the same pressure. 2) In terms of flow, the increase in slope of pressure drop in the vertical upward tube is due to the orifice contraction effect. The mechanism that dominates the variation of pressure drop in the horizontal tube is the flow stratification effect, and we show that Froude number Frave can be the similarity criterion number to connect the temperature difference between the top and bottom generatrix of horizontal tube and the pressure drop. The analysis suggests that mechanisms governing horizontal flow and vertical flow of$\rm sCO_2 $ are different in heat transfer deterioration mode. For the vertical flow, the SBO plays a leading role, while for the horizontal flow, the Fr plays an indispensable role.-
Keywords:
- supercritical carbon dioxide /
- flow heat transfer /
- pseudo-boiling /
- heat transfer deterioration
[1] Duffey R, Pioro I, Zhou X, Zirn U, Kuran S, Khartabil H, Naidin M 2008 Proceedings of the 16th International Conference on Nuclear Engineering Orlando, FL, USA, May 11–15, 2008 p9
[2] Cheng X, Schulenberg T 2001 Karlsruhe Research Centre of Technology and Environment (Karlsruhe, Germany) p12
[3] Martinez A, Duchateau J L, Mardion G B, Gauthier A, Rousset B 1994 Cryogenics 34 591
[4] Dadashev M, Stepanov G 2000 Chem. Technol. Fuels Oils 36 8
Google Scholar
[5] Yamada T, Haraguchi N, Hihara E, Wang J 2005 Therm. Sci. Eng. 13 93
[6] Fu Y C, Huang H R, Wen J, Xu G Q, Zhao W 2017 Int. J. Heat Mass Transfer 112 814
Google Scholar
[7] Zhang Q, Li H X, Kong X F, Liu J L, Lei X L 2018 Int. J. Heat Mass Transfer 122 469
Google Scholar
[8] Zhang H S, Xu J L, Zhu X J, Xie J, Li M J, Zhu B G 2021 Appl. Therm. Eng. 182 116078
Google Scholar
[9] Zhang C, Hao B, Cheng L, Xu J, Wang Q 2023 Heat Transfer Eng. 1
[10] Pu H, Li N, Dong M, Shang Y, Du H, Hou C, Zhang J 2023 Int. J. Therm. Sci. 184 107992
Google Scholar
[11] Xu Y, Yi Z M 2023 Energy 262 125474
Google Scholar
[12] Yu S, Li H, Lei X, Feng Y, Zhang Y, He H, Wang T 2013 Exp. Therm Fluid Sci. 50 213
Google Scholar
[13] Huang D, Wu Z, Sunden B, Li W 2016 Appl. Energy 162 494
Google Scholar
[14] Yamagata K, Nishikawa K, Hasegawa S, Fujii T, Yoshida S 1972 Int. J. Heat Mass Transfer. 15 2575
Google Scholar
[15] Lei Y C, Xu B, Chen Z Q 2021 Int. J. Heat Mass Transfer. 181 121792
Google Scholar
[16] Lei X L, Li H X, Zhang W Q, Dinh N T, Guo Y M, Yu S Q 2017 Appl. Therm. Eng. 113 609
Google Scholar
[17] Hall W B, Jackson J D 1978 Adv. in Heat Transfer. 7 1
[18] 张海松, 朱鑫杰, 朱兵国, 徐进良, 刘欢 2020 69 064401
Google Scholar
Zhang H S, Zhu X J, Zhu B G, Xu J L, Liu H 2020 Acta Phys. Sin. 69 064401
Google Scholar
[19] Brassington D, Cairns D 1977 Int. J. Heat Mass Transfer 20 207
Google Scholar
[20] Simeoni G, Bryk T, Gorelli F, Krisch M, Ruocco G, Santoro M, Scopigno T 2010 Nat. Phys. 6 503
Google Scholar
[21] Xu J L, Wang Y, Ma X J 2021 Phys. Rev. E 104 014142
Google Scholar
[22] 何孝天, 徐进良, 程怡玮 2023 72 057801
Google Scholar
He X T, Xu J L, Cheng Y W 2023 Acta Phys. Sin. 72 057801
Google Scholar
[23] Wang Q Y, Ma X J, Xu J L, Li M J, Wang Y 2021 Int. J. Heat Mass Transfer 181 121875
Google Scholar
[24] Zhu B J, Xu J L, Wu X M, Xie J, Li M J 2019 Int. J. Therm. Sci. 136 254
Google Scholar
[25] Kim T H, Kwon J G, Kim M H, Park H S 2018 Exp. Therm Fluid Sci. 92 222
Google Scholar
[26] Xu J, Chen T K 1998 Heat Transfer Eng. 19 45
Google Scholar
[27] Yin F, Chen T K, Li H X 2006 Heat Transfer Eng. 27 44
[28] Jiang P X, Zhang Y, Shi R F 2008 Int. J. Heat Mass Transfer 51 3052
Google Scholar
[29] Chu X, Laurien E 2016 J. Supercrit. Fluids 116 172
Google Scholar
[30] 闫晨帅, 徐进良 2020 69 044401
Google Scholar
Yan C S, Xu J L 2020 Acta Phys. Sin. 69 044401
Google Scholar
[31] Tian R, Xu Y T, Shi L, Song P P, Wei M S 2020 Energy 205 118061
Google Scholar
[32] Xing F, Xu J L, Xie J, Liu H, Wang Z X, Ma X L 2015 Int. J. Multiphase Flow 71 98
Google Scholar
[33] Kandlikar S 1990 J. Heat Transfer 112 219
Google Scholar
[34] Lu C, Kong R, Qiao S, Larimer J, Kim S, Bajorek S, Tien K, Hoxie C 2018 Nucl. Eng. Des. 332 147
Google Scholar
[35] 程亮元, 王清洋, 王庆华, 徐进良 2023 中国电机工程学报 43 6718
Cheng L Y, Wang Q Y, Wang Q H, Xu J L 2023 Proceed. CSEE 43 6718
[36] Fan Y H, Tang G H, Sheng Q, Li X L, Yang D L 2023 Energy 262 125470
Google Scholar
[37] Solov'Ev A V, Preobrazhenskii E I, Semenov P A 1967 Int. Chem. Engng 7 59
[38] Kumar A, Hardik B 2022 Appl. Therm. Eng. 201 117822
Google Scholar
[39] Saisorn S, Wongpromma P, Wongwises S 2018 Int. J. Multiphase Flow 101 97
Google Scholar
-
图 1 实验段及测点布置 (a) 水平管; (b) 垂直向上管
Fig. 1. Test tubes and the measuring point disposition: (a) Horizontal tube; (b) vertical upward tube.
图 2 水平管和垂直向上管内流动内壁温分布 (a) qw/G = 0.18 kJ/kg; (b) qw/G = 0.34 kJ/kg
Fig. 2. Inner-wall temperature in horizontal and vertical upward flow: (a) qw/G = 0.18 kJ/kg; (b) qw/G = 0.34 kJ/kg.
图 3 不同热流密度下水平管和垂直向上管换热和流动特性比较 (a)内壁温分布; (b)阻力压降
Fig. 3. Comparison of the heat transfer and flow characteristics in horizontal and vertical upward tubes at different heat fluxes: (a) Inner-wall temperature distribution; (b) friction pressure drop.
图 4 不同质量流量下水平管和垂直向上管换热和流动特性比较 (a)内壁温分布; (b)阻力压降
Fig. 4. Comparison of the heat transfer and flow characteristics in horizontal and vertical upward tubes at different mass fluxes: (a) Inner-wall temperature distribution; (b) friction pressure drop.
图 5 不同压力下水平管和垂直向上管换热和流动特性比较 (a) 内壁温分布; (b) 阻力压降
Fig. 5. Comparison of the heat transfer and flow characteristics in horizontal and vertical upward tubes at different pressures: (a) Inner-wall temperature distribution; (b) friction pressure drop.
图 6 水平管和垂直向上管摩擦因子比较 (a) 垂直向上管摩擦因子取决于SBO; (b)水平管摩擦因子取决于Frave
Fig. 6. Comparison of friction pressure drop in horizontal and vertical upward tubes: (a) f of vertical upward tube depending on SBO; (b) f of horizontal tube depending on Frave.
图 7 SBO和Frave对水平管最大上下壁温差的影响 (a) 三维图; (b) 等温线图
Fig. 7. Effect of SBO and Frave on the maximum temperature difference between top generatrix and bottom generatrix: (a) Three-dimensional figure; (b) isotherm figure.
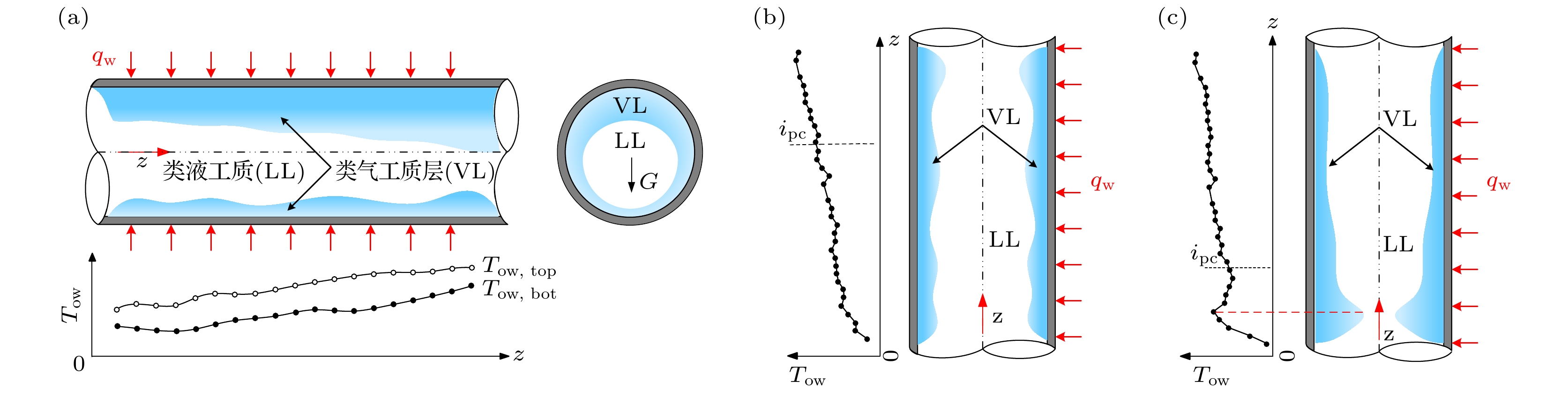
图 8 水平管和垂直向上管流动传热机理图 (a) 水平管; (b) 正常传热模式的垂直向上管; (c) 传热恶化模式的垂直向上管
Fig. 8. Schematics showing the mechanisms for heat transfer and flow characteristics in horizontal and vertical upward tubes: (a) Horizontal tube; (b) vertical upward tube in normal heat transfer mode; (c) vertical upward tube in heat transfer deterioration mode.
-
[1] Duffey R, Pioro I, Zhou X, Zirn U, Kuran S, Khartabil H, Naidin M 2008 Proceedings of the 16th International Conference on Nuclear Engineering Orlando, FL, USA, May 11–15, 2008 p9
[2] Cheng X, Schulenberg T 2001 Karlsruhe Research Centre of Technology and Environment (Karlsruhe, Germany) p12
[3] Martinez A, Duchateau J L, Mardion G B, Gauthier A, Rousset B 1994 Cryogenics 34 591
[4] Dadashev M, Stepanov G 2000 Chem. Technol. Fuels Oils 36 8
Google Scholar
[5] Yamada T, Haraguchi N, Hihara E, Wang J 2005 Therm. Sci. Eng. 13 93
[6] Fu Y C, Huang H R, Wen J, Xu G Q, Zhao W 2017 Int. J. Heat Mass Transfer 112 814
Google Scholar
[7] Zhang Q, Li H X, Kong X F, Liu J L, Lei X L 2018 Int. J. Heat Mass Transfer 122 469
Google Scholar
[8] Zhang H S, Xu J L, Zhu X J, Xie J, Li M J, Zhu B G 2021 Appl. Therm. Eng. 182 116078
Google Scholar
[9] Zhang C, Hao B, Cheng L, Xu J, Wang Q 2023 Heat Transfer Eng. 1
[10] Pu H, Li N, Dong M, Shang Y, Du H, Hou C, Zhang J 2023 Int. J. Therm. Sci. 184 107992
Google Scholar
[11] Xu Y, Yi Z M 2023 Energy 262 125474
Google Scholar
[12] Yu S, Li H, Lei X, Feng Y, Zhang Y, He H, Wang T 2013 Exp. Therm Fluid Sci. 50 213
Google Scholar
[13] Huang D, Wu Z, Sunden B, Li W 2016 Appl. Energy 162 494
Google Scholar
[14] Yamagata K, Nishikawa K, Hasegawa S, Fujii T, Yoshida S 1972 Int. J. Heat Mass Transfer. 15 2575
Google Scholar
[15] Lei Y C, Xu B, Chen Z Q 2021 Int. J. Heat Mass Transfer. 181 121792
Google Scholar
[16] Lei X L, Li H X, Zhang W Q, Dinh N T, Guo Y M, Yu S Q 2017 Appl. Therm. Eng. 113 609
Google Scholar
[17] Hall W B, Jackson J D 1978 Adv. in Heat Transfer. 7 1
[18] 张海松, 朱鑫杰, 朱兵国, 徐进良, 刘欢 2020 69 064401
Google Scholar
Zhang H S, Zhu X J, Zhu B G, Xu J L, Liu H 2020 Acta Phys. Sin. 69 064401
Google Scholar
[19] Brassington D, Cairns D 1977 Int. J. Heat Mass Transfer 20 207
Google Scholar
[20] Simeoni G, Bryk T, Gorelli F, Krisch M, Ruocco G, Santoro M, Scopigno T 2010 Nat. Phys. 6 503
Google Scholar
[21] Xu J L, Wang Y, Ma X J 2021 Phys. Rev. E 104 014142
Google Scholar
[22] 何孝天, 徐进良, 程怡玮 2023 72 057801
Google Scholar
He X T, Xu J L, Cheng Y W 2023 Acta Phys. Sin. 72 057801
Google Scholar
[23] Wang Q Y, Ma X J, Xu J L, Li M J, Wang Y 2021 Int. J. Heat Mass Transfer 181 121875
Google Scholar
[24] Zhu B J, Xu J L, Wu X M, Xie J, Li M J 2019 Int. J. Therm. Sci. 136 254
Google Scholar
[25] Kim T H, Kwon J G, Kim M H, Park H S 2018 Exp. Therm Fluid Sci. 92 222
Google Scholar
[26] Xu J, Chen T K 1998 Heat Transfer Eng. 19 45
Google Scholar
[27] Yin F, Chen T K, Li H X 2006 Heat Transfer Eng. 27 44
[28] Jiang P X, Zhang Y, Shi R F 2008 Int. J. Heat Mass Transfer 51 3052
Google Scholar
[29] Chu X, Laurien E 2016 J. Supercrit. Fluids 116 172
Google Scholar
[30] 闫晨帅, 徐进良 2020 69 044401
Google Scholar
Yan C S, Xu J L 2020 Acta Phys. Sin. 69 044401
Google Scholar
[31] Tian R, Xu Y T, Shi L, Song P P, Wei M S 2020 Energy 205 118061
Google Scholar
[32] Xing F, Xu J L, Xie J, Liu H, Wang Z X, Ma X L 2015 Int. J. Multiphase Flow 71 98
Google Scholar
[33] Kandlikar S 1990 J. Heat Transfer 112 219
Google Scholar
[34] Lu C, Kong R, Qiao S, Larimer J, Kim S, Bajorek S, Tien K, Hoxie C 2018 Nucl. Eng. Des. 332 147
Google Scholar
[35] 程亮元, 王清洋, 王庆华, 徐进良 2023 中国电机工程学报 43 6718
Cheng L Y, Wang Q Y, Wang Q H, Xu J L 2023 Proceed. CSEE 43 6718
[36] Fan Y H, Tang G H, Sheng Q, Li X L, Yang D L 2023 Energy 262 125470
Google Scholar
[37] Solov'Ev A V, Preobrazhenskii E I, Semenov P A 1967 Int. Chem. Engng 7 59
[38] Kumar A, Hardik B 2022 Appl. Therm. Eng. 201 117822
Google Scholar
[39] Saisorn S, Wongpromma P, Wongwises S 2018 Int. J. Multiphase Flow 101 97
Google Scholar
下载:
计量
- 文章访问数: 2076
- PDF下载量: 32
- 被引次数: 0
