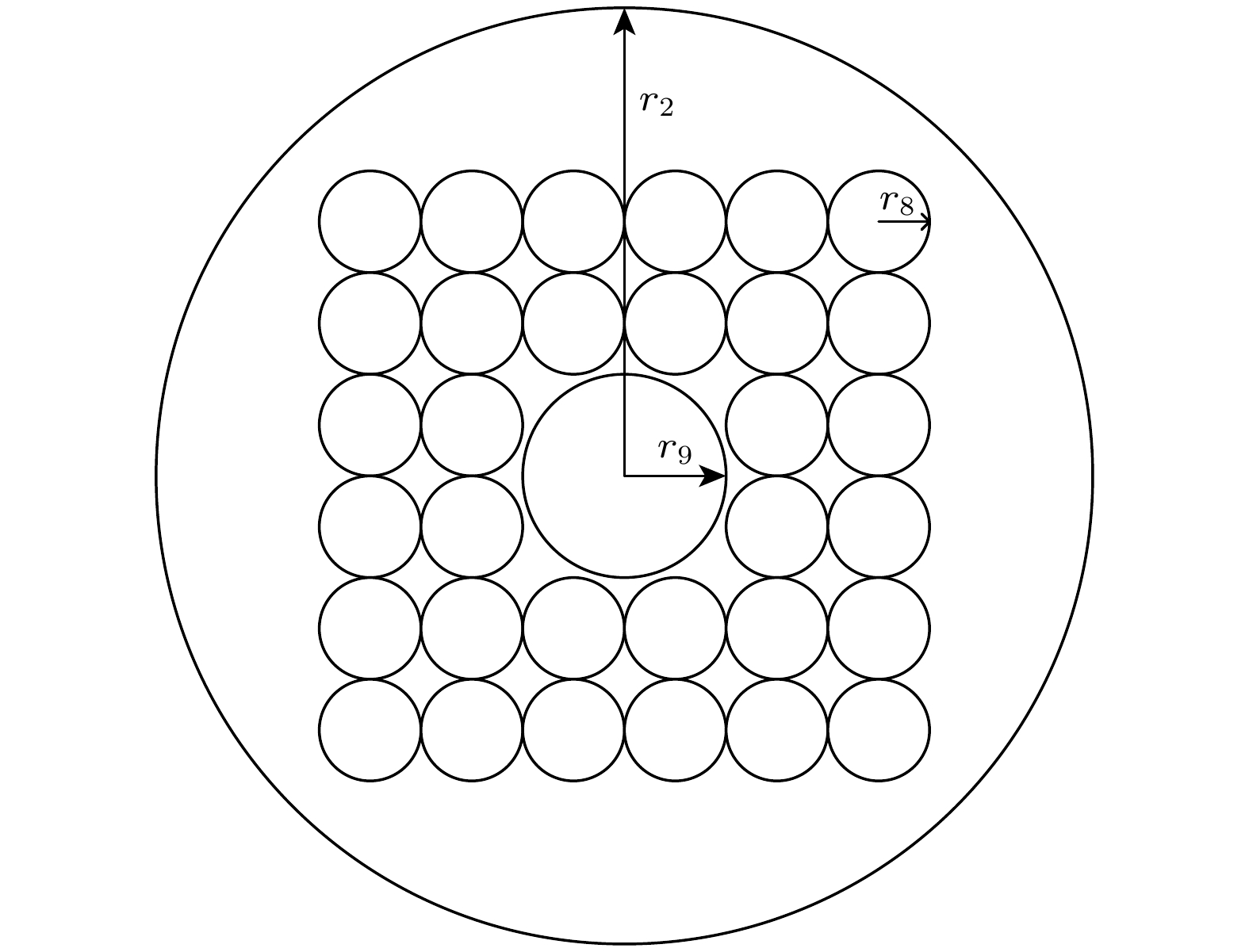
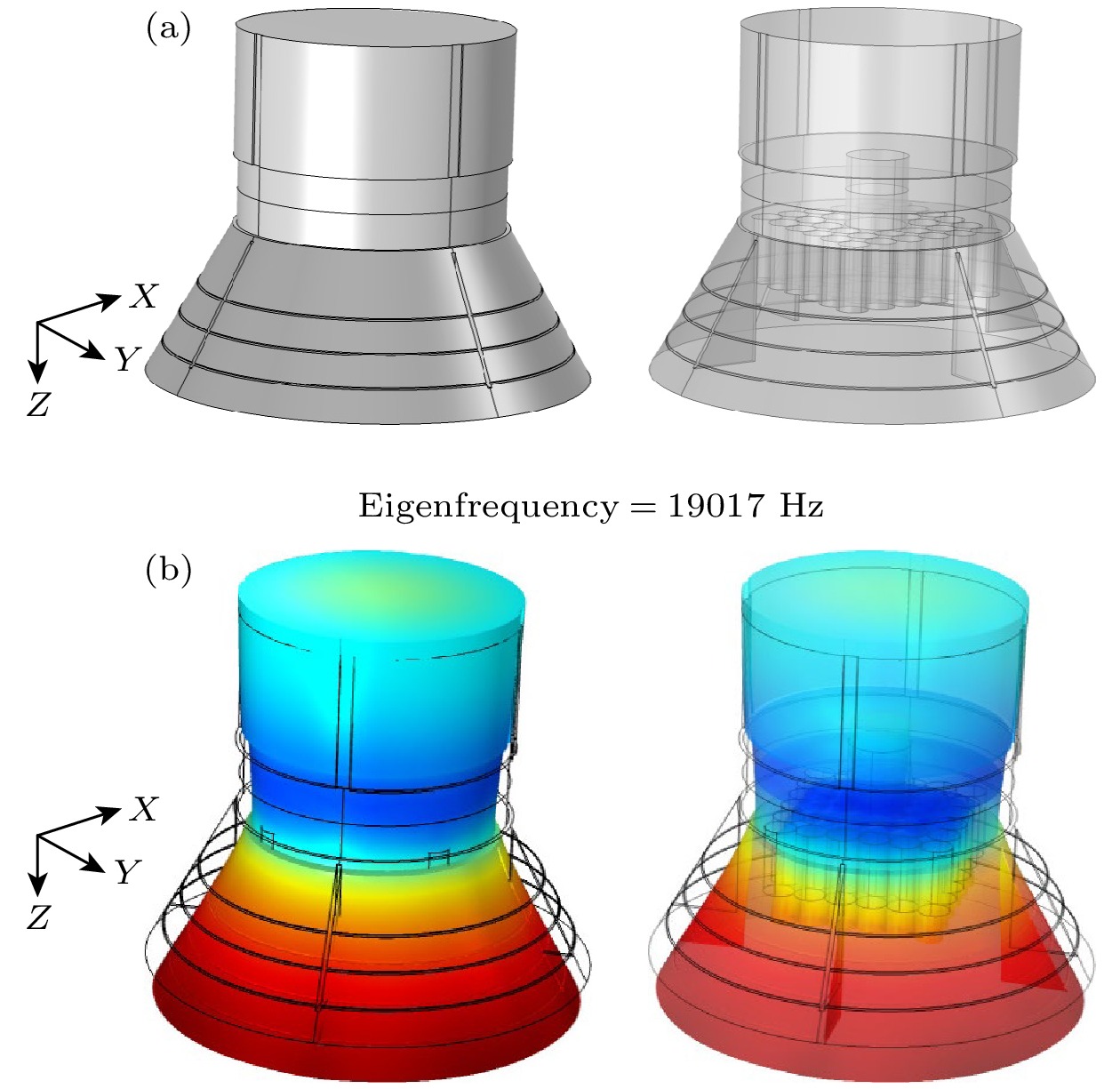
-
Researches have shown that a reasonably designed phononic crystal defect structure in high-power piezoelectric ultrasonic transducers can effectively suppress stray vibration modes. However, when the size of the transducer is large, the improvement of the displacement amplitude of the radiation surface of the transducer device by the phononic crystal defect structure is still not so ideal. How to effectively suppress harmful vibrations while ensuring the operational efficiency of transducers and enhancing the displacement amplitude of their radiating surfaces has always been a challenging problem in the field of power ultrasonics that needs to be solved urgently. Researches have found that acoustic surface structures can achieve unidirectional energy transmission, effectively reduce energy loss, and enhance the efficiency of energy transmission. Based on this, the high-power piezoelectric ultrasonic transducers with surface and defect regulation are investigated in this work. By designing reasonable defects and acoustic surface structures in the transducer, strong localization effects of sound waves can be excited to achieve acoustic anomalous transmission, significantly increasing the longitudinal radiated sound power of the transducer. At the same time, a data analysis technique is used to analyze the influence of material composition and geometric parameters of acoustic surface structure and defect structure on the performance of transducers, and a performance prediction model is established for high-power piezoelectric ultrasonic transducers, ultimately achieving optimized design of transducers. In this study. a new theory and method are systematically proposed for optimizing the design of high-power piezoelectric ultrasonic transducers quantitatively. Simulation and experimental results show that the innovative design capability and intelligent level of high-power piezoelectric ultrasonic transducers can be improved, making the vibration mode of the transducer more singular in high-power application environments, and thus significantly improving the displacement amplitude and amplitude distribution uniformity of the transducer radiation surface. -
Keywords:
- acoustic surface and defect control /
- high-power piezoelectric ultrasonic transducer /
- localization effect /
- performance prediction
-
图 1 大功率压电超声换能器的结构和振型图 (a) 结构图; (b)振型图; (c) 辐射面位移分布图
Figure 1. Structure and vibration mode diagram of high-power piezoelectric ultrasonic transducer: (a) Structural diagram; (b) vibration mode diagram; (c) displacement distribution diagram of radiation surface.
图 2 未优化换能器的辐射面位移振幅
Figure 2. Radiation surface displacement amplitude of unoptimized transducer.
图 3 优化后的后盖板的结构示意图
Figure 3. Structural schematic diagram of optimized rear cover plate.
图 4 优化后的前盖板的结构示意图
Figure 4. Structural schematic diagram of optimized front cover plate.
图 5 优化后的前盖板的结构示意图(俯视图)
Figure 5. Structural schematic diagram of optimized front cover plate (top view).
图 6 优化后的前盖板的结构示意图(侧视图)
Figure 6. Structural schematic diagram of optimized front cover plate (side view).
图 7 表面与缺陷调控型大功率压电超声换能器的结构模型图和振型图 (a) 换能器结构模型图; (b)振型图
Figure 7. Structural model diagram and vibration mode diagram of surface and defect controlled high-power piezoelectric ultrasonic transducer: (a) Structural model diagram of transducer; (b) vibration mode diagram.
图 8 优化后的换能器和未优化的换能器的辐射面位移振幅对比图
Figure 8. Comparison of displacement amplitude of radiation surface between optimized and unoptimized transducers.
图 9 三种换能器性能对比图 (a) 辐射面位移振幅和分布均匀度对比图; (b) 总辐射功率对比图
Figure 9. Comparison chart of three types of transducer performance: (a) Comparison diagram of displacement amplitude and distribution uniformity of radiation surface; (b) comparison chart of total radiated power.
图 10 不同的属性参数对纵向谐振频率f的影响.
Figure 10. Influences of different attributes parameters on the longitudinal resonance frequency f.
图 12 不同的属性参数对辐射面位移振幅分布Du的影响
Figure 12. Influences of different attribute parameters on the amplitude distribution Du of radiation surface displacement.
图 13 纵向谐振频率f的仿真值和预测值的对比及相对误差
Figure 13. Comparison and relative error between simulated and predicted values of longitudinal resonant frequency f.
图 15 辐射面位移振幅分布Du的仿真值和预测值的对比及相对误差
Figure 15. Comparison and relative error between simulated and predicted values of Du.
图 11 不同的属性参数对辐射面位移振幅平均值Aave的影响
Figure 11. Influences of different attribute parameters on the average displacement amplitude Aave of the radiation surface.
图 14 辐射面位移振幅平均值Aave的对比及相对误差
Figure 14. Comparison and relative error of average amplitude Aave of radiation surface displacement.
图 16 换能器实物图
Figure 16. Physical images of transducers.
图 17 精密阻抗分析仪的测量图.
Figure 17. Measurement diagram of precision impedance analyzer.
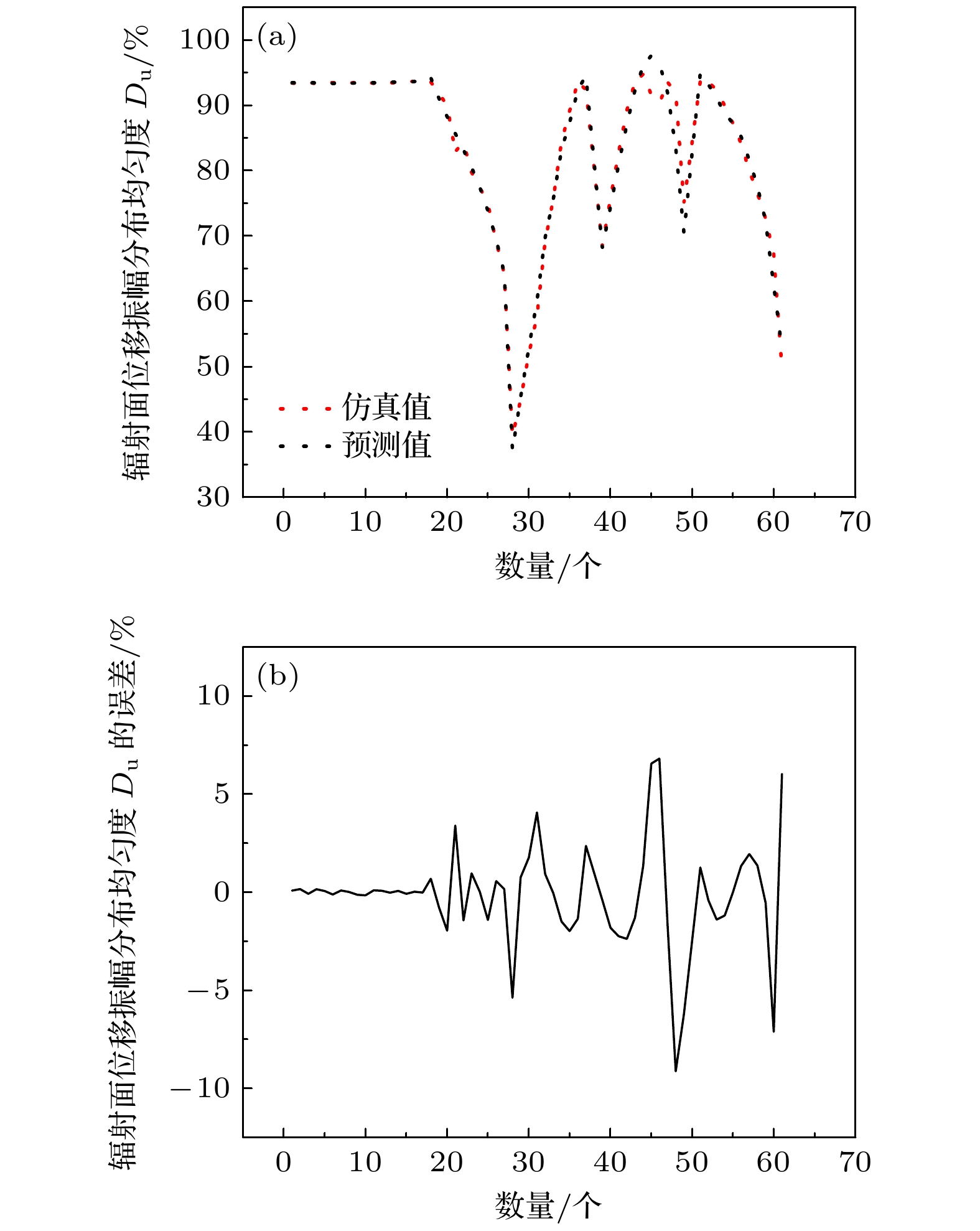
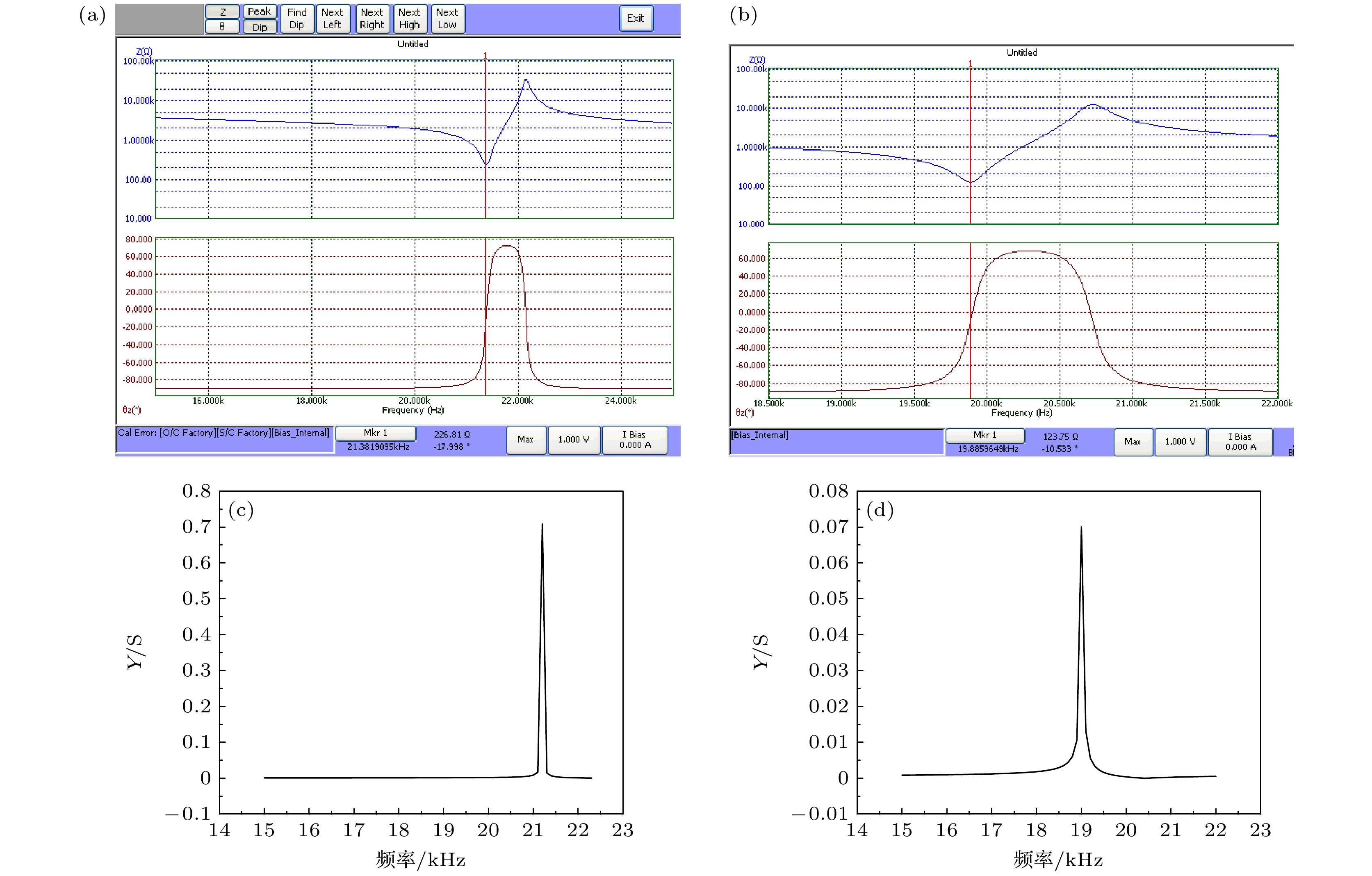
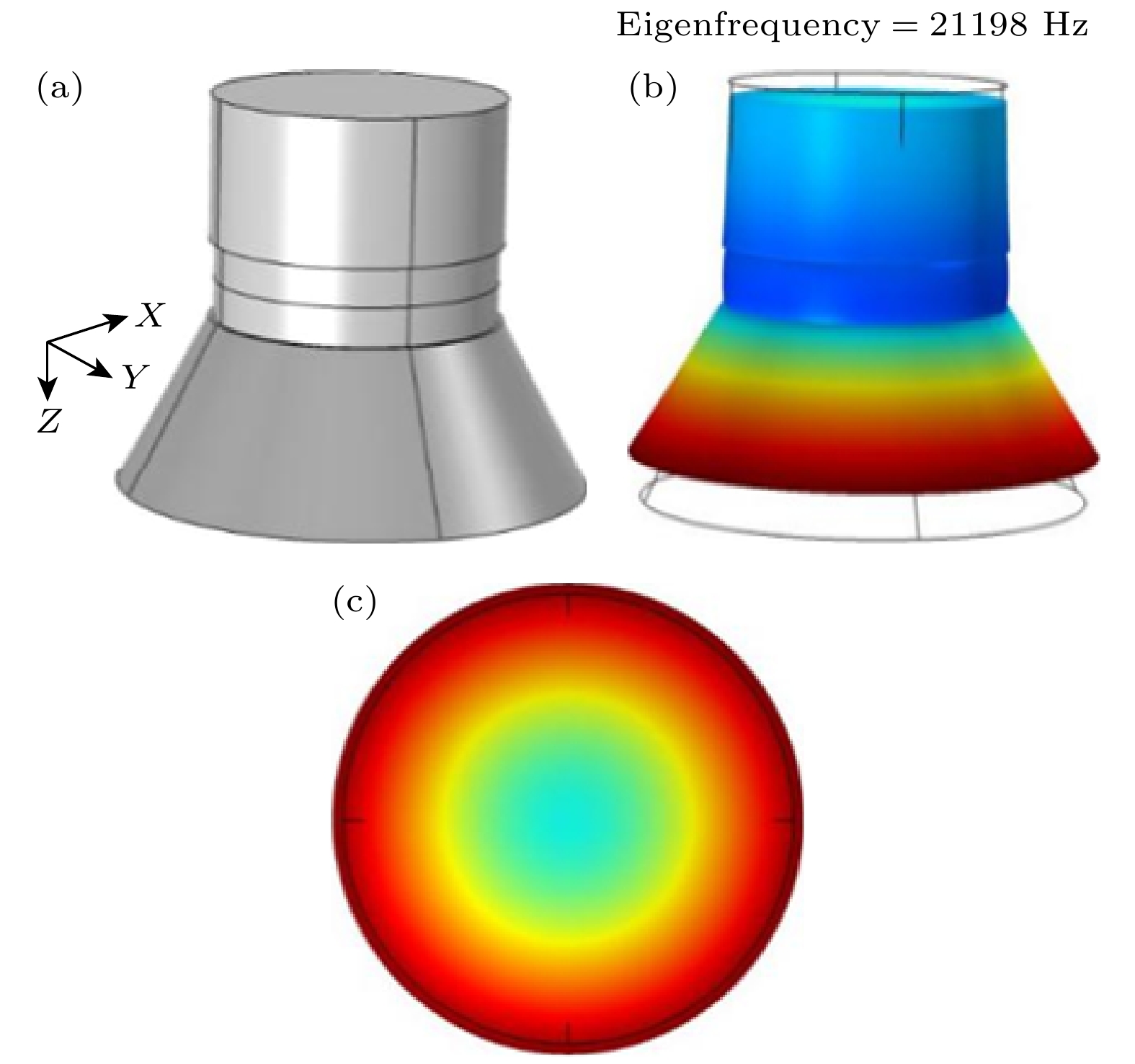
图 18 输入电阻抗与谐振频率的测量 (a) 未优化的换能器的测量结果; (b) 表面与缺陷调控型换能器的测量结果; (c) 未优化的换能器的仿真导纳曲线图; (d)表面与缺陷调控型换能器的仿真导纳曲线图
Figure 18. Measurement of input impedance and resonant frequency: (a) Measurement results of an unoptimized transducer; (b) measurement results of surface and defect controlled transducers; (c) simulation admittance curve of unoptimized transducer; (d) simulation admittance curve of surface and defect controlled transducers.
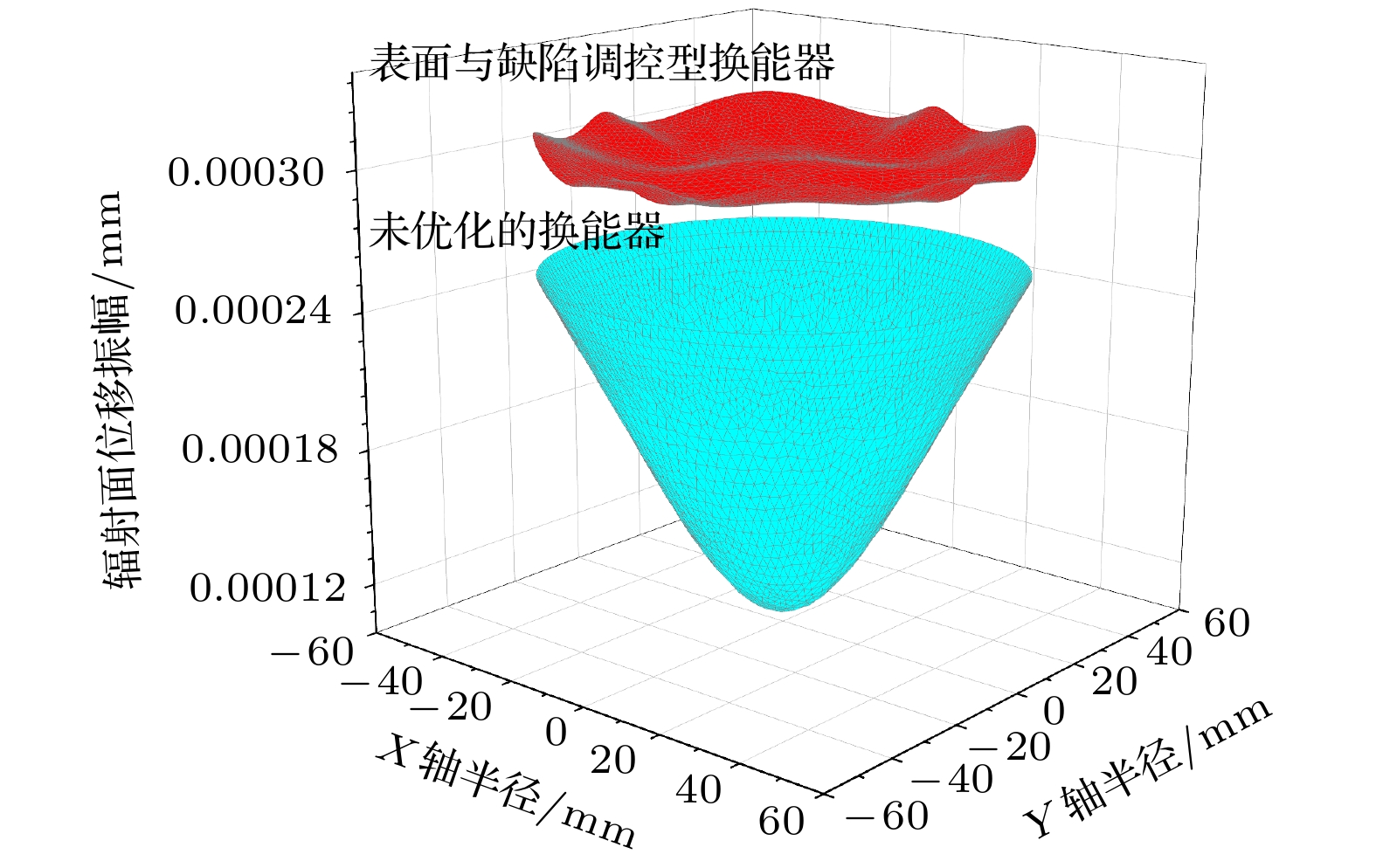
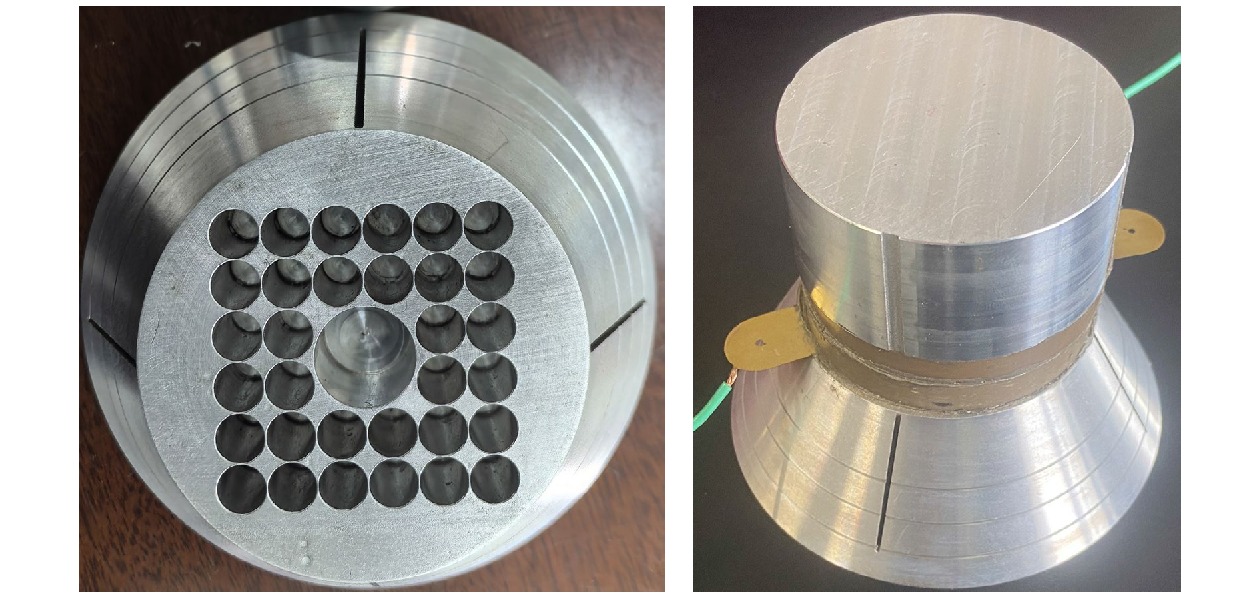
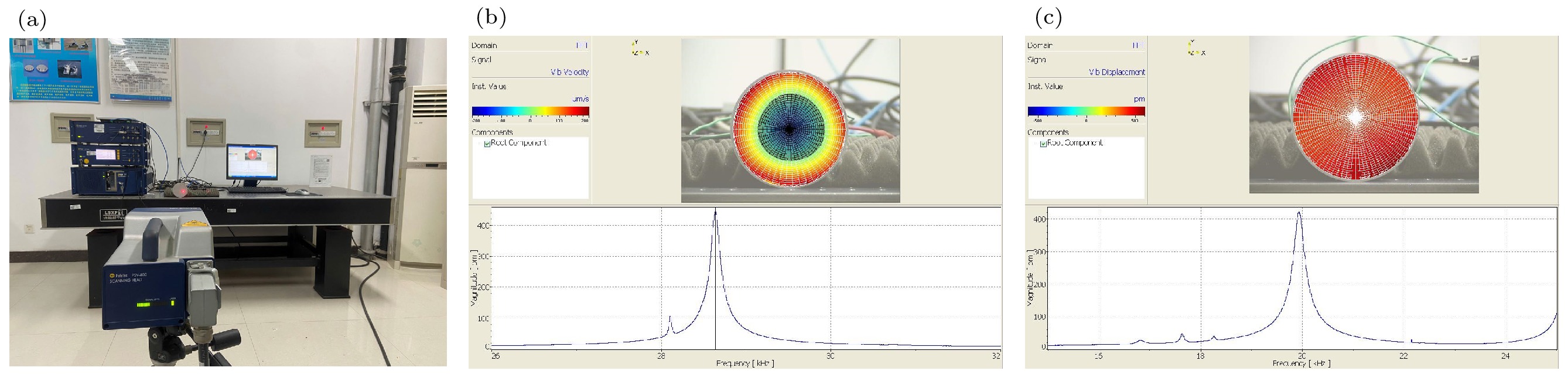
图 19 换能器辐射面位移振幅分布的实验测量 (a) 测量过程; (b) 测量得到的未优化换能器的表面数据; (c) 测量得到的优化后的换能器的表面数据
Figure 19. Experimental measurement of displacement amplitude distribution of transducer radiation surface: (a) Measurement process; (b) surface data of unoptimized transducers obtained through measurement; (c) the surface data of the optimized transducer obtained through measurement.
表 1 压电超声换能器的材料和几何结构参数
Table 1. Materials and geometric structure parameters of piezoelectric ultrasonic transducer.
部件 材料属性 形状 半径/mm 半径/mm 高/mm 后盖板 AISI 4340 钢 等截面圆柱 31 31 30 压电陶瓷圆环(两片) PZT-4压电陶瓷 等截面圆环 7(内径) 30(外径) 8 前盖板 6063-T83铝 圆台 31(上底) 50(下底) 35 
DownLoad: CSV
表 2 三种换能器性能对比表
Table 2. Performance comparison table of three transducers.
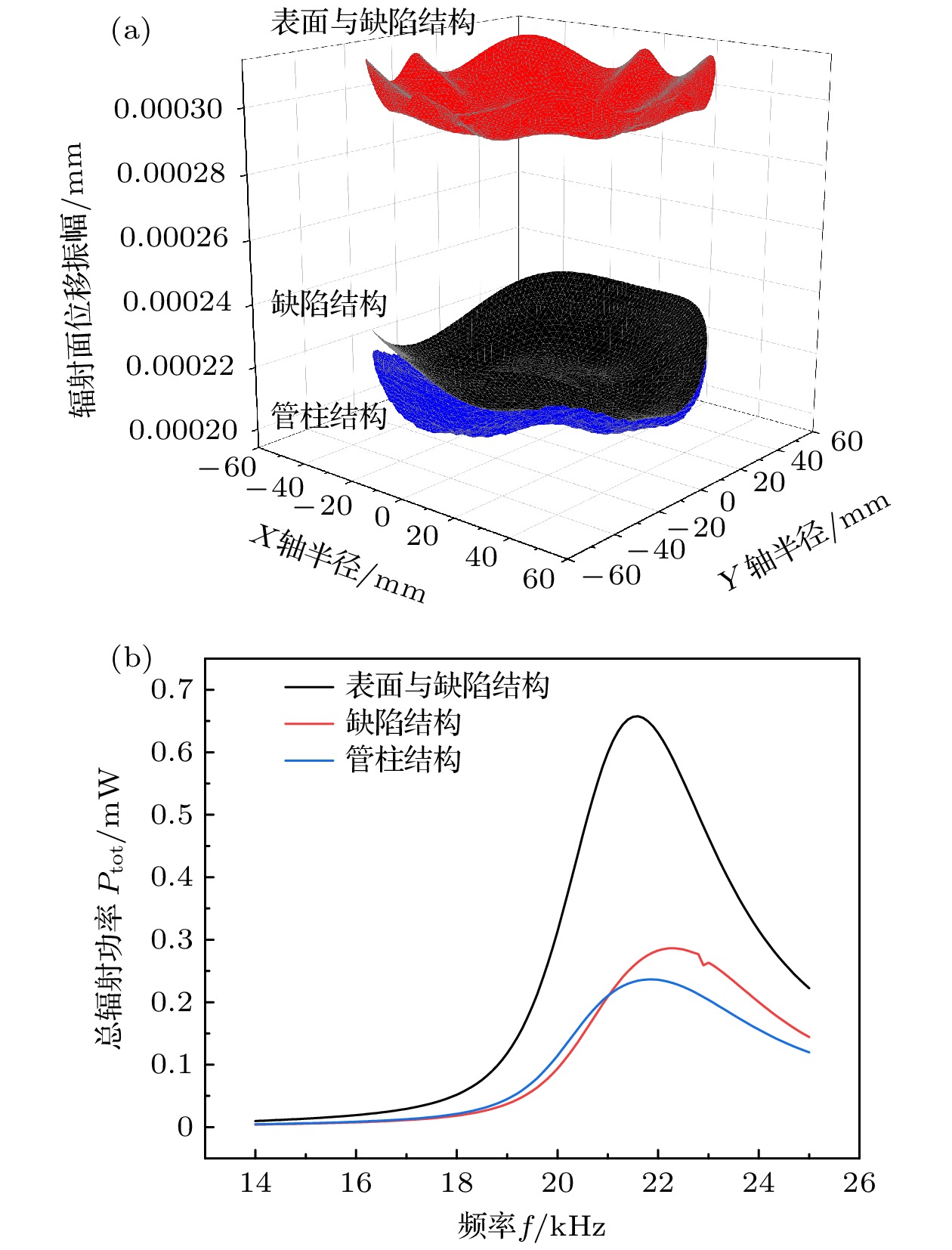
换能器 位移振幅Aave/mm 分布均匀度Du
/%总辐射功率
/mW缺陷结构 0.2180 × 10–3 87.73 0.04818 管柱结构 0.2109 × 10–3 90.56 0.04904 表面与缺陷结构 0.3015 × 10–3 93.56 0.1181 比值(表面与缺陷/缺陷) 1.384 1.066 2.450 比值(表面与缺陷/管柱) 1.430 1.033 2.407
DownLoad: CSV
表 3 纵向谐振频率f的预测模型(单位Hz)
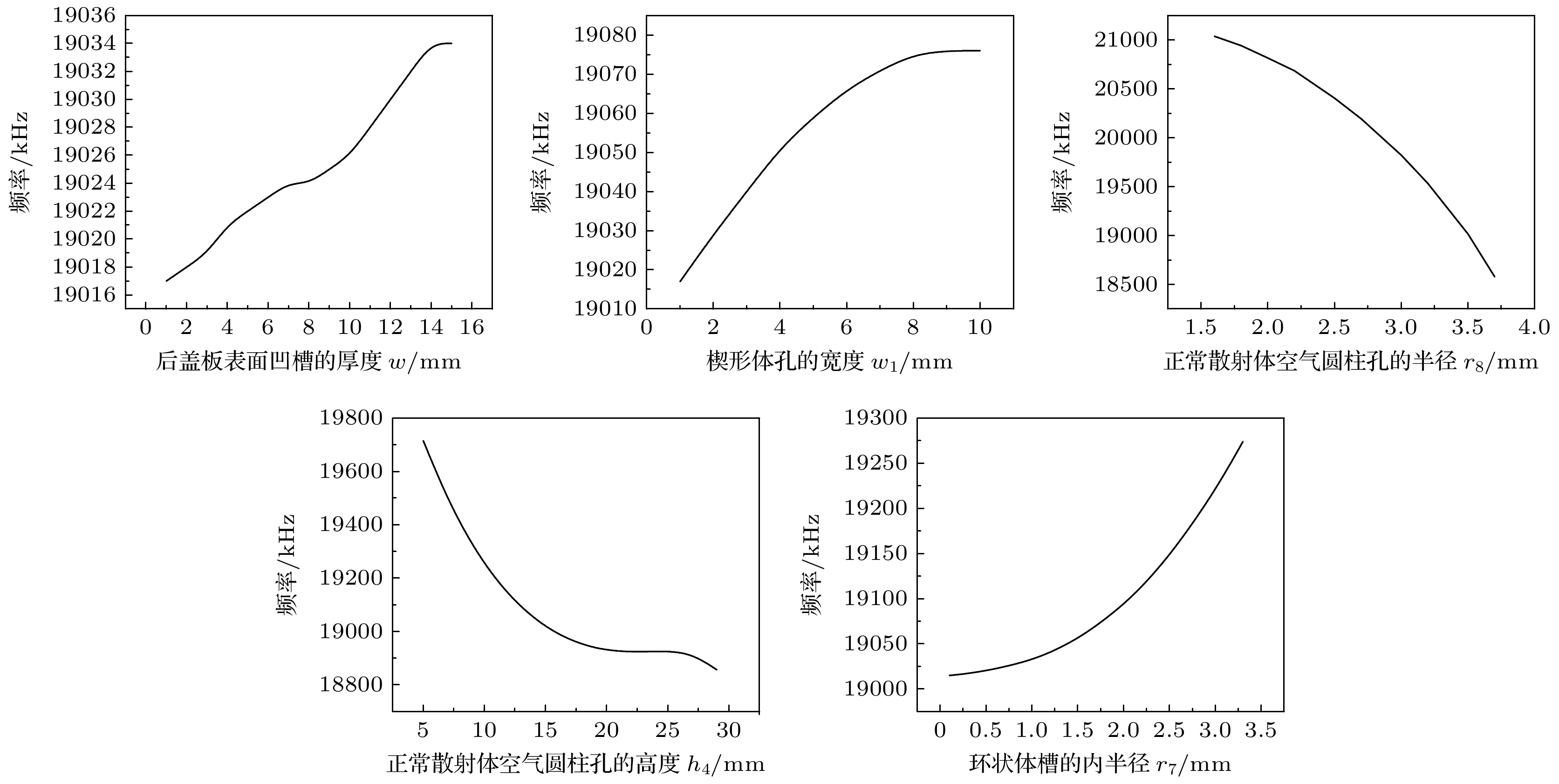
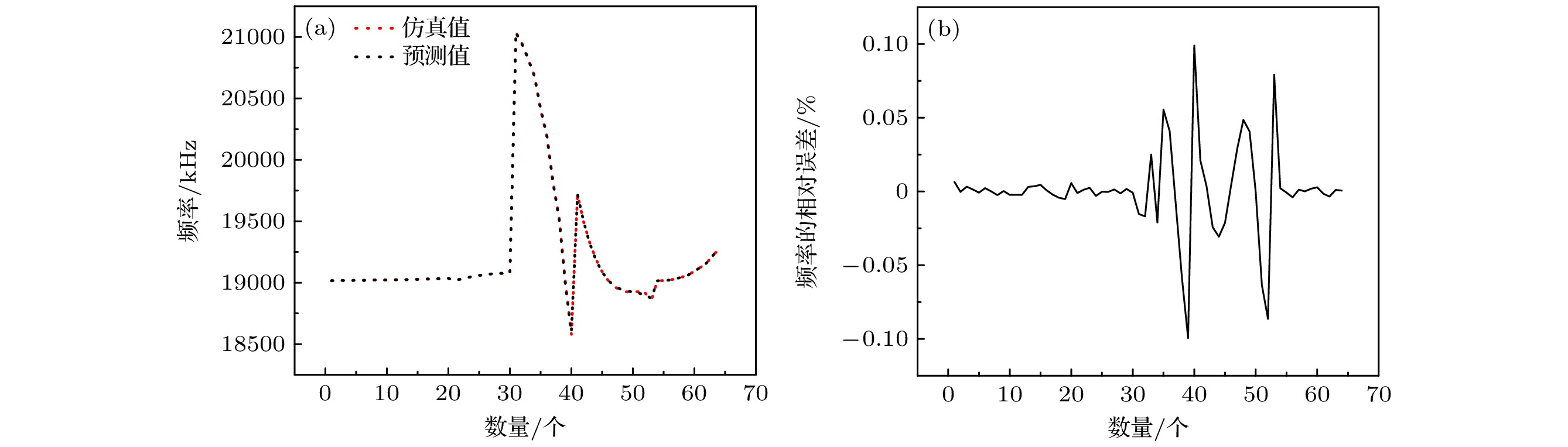
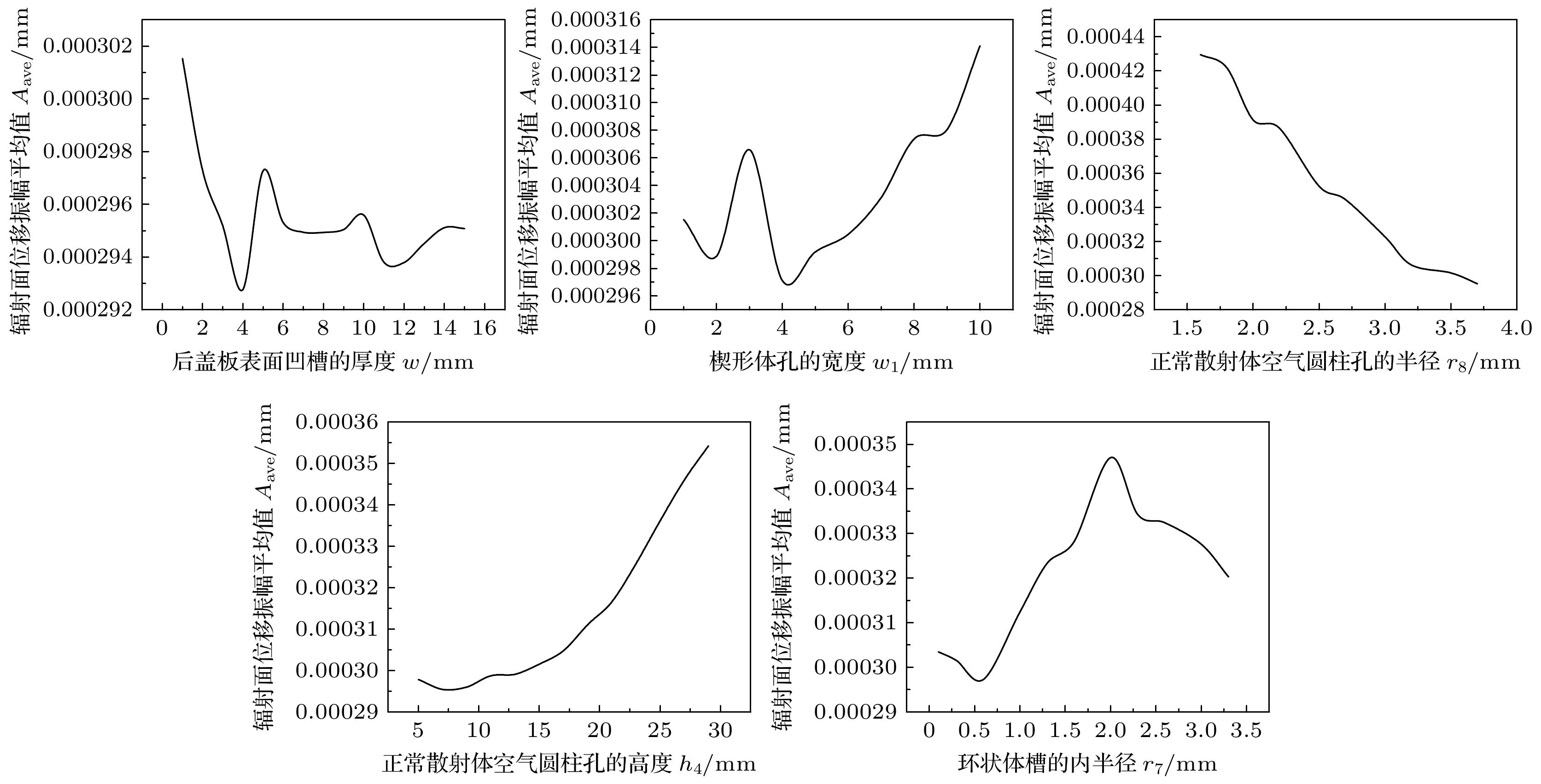
Table 3. Predictive model for longitudinal resonant frequency f of slot structures (Unit: Hz).
A B C D 后盖板表面凹槽厚度w/mm 19015.485 1.544 –0.09065 0.004986 楔形体孔的宽度w1 /mm 19003.333 13.94 –0.4575 –0.02117 正常散射体空气圆柱孔半径r8/mm 21299.902 –36.52 0.000 –50.71 空气圆柱孔的高度h4/mm 20530.792 –202.5 8.669 –0.1261 环状体槽的内半径r7/mm 19015.083 2.116 11.49 3.530
DownLoad: CSV
表 4 辐射面位移振幅平均值Aave的预测模型
Table 4. Prediction model for the average displacement amplitude Aave of the radiation surface.
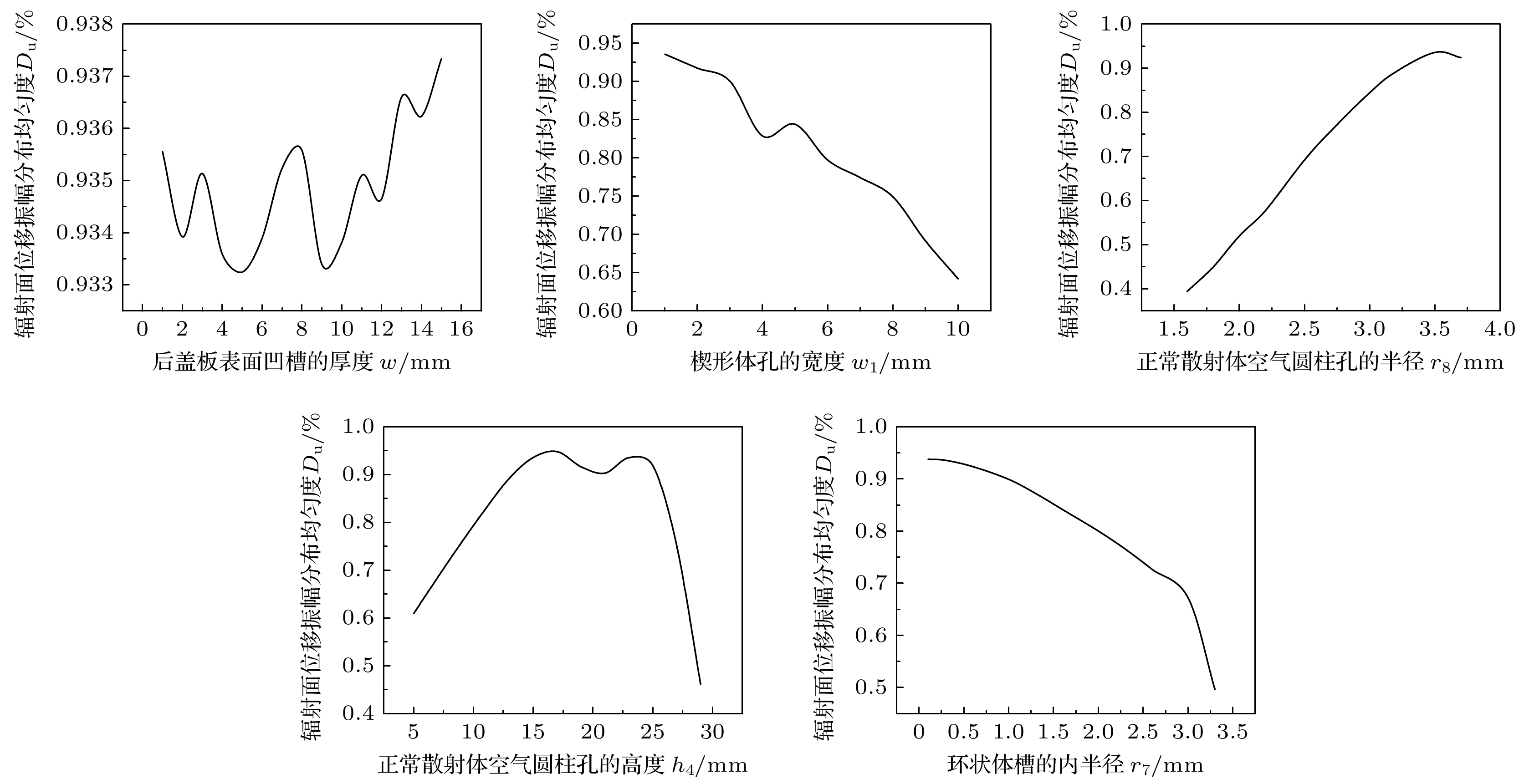
A B C D 后盖板表面凹槽厚度w/ mm 0.0002939 6.991×10–6 0.000 0.000 楔形体孔的宽度w1/mm 3.048×10–4 –2.717×10–6 3.576×10–7 0.000 正常散射体空气圆柱孔半径r8/mm 6.102×10–4 –1.155×10–4 0.000 2.187×10–6 空气圆柱孔的高度h4/mm 3.063×10–4 –2.397×10–6 1.375×10–7 1.373×10–10 环状体槽的内半径r7/mm 3.005×10–4 –4.852×10–6 2.407×10–5 –6.396×10–6
DownLoad: CSV
表 5 辐射面位移振幅分布均匀度Du的预测模型
Table 5. Prediction model for the uniformity of displacement amplitude distribution Du on the radiation surface.
A B C D 后盖板表面凹槽厚度w/mm 0.9355 –0.0004441 3.130×10–5 4.060×10–7 楔形体孔的宽度w1/mm 0.9798 –0.04139 0.003935 –0.0003165 正常散射体空气圆柱孔半径r8/mm –0.3622 0.4834 0.000 –0.009487 空气圆柱孔的高度h4/mm 0.5726 –0.004406 0.003738 –0.0001257 环状体槽的内半径r7/mm 0.9600 –0.1010 0.05795 –0.01953
DownLoad: CSV
-
[1] Lin S Y 2004 J. Acoust. Soc. Am. 115 182
Google Scholar
[2] Liu S Q, Yao Y 2008 J. Mecha. Engi. 44 239
[3] Chen C, Dong Y L, Wang S, Hu L Q, Lin S Y 2022 J. Acoust. Soc. Am. 151 2712
Google Scholar
[4] 许龙, 王威震, 龚涛, 李果, 赵伦, 周光平, 梁召峰 2024 振动与冲击 43 287
Xu L, Wang W Z, Gong T, Li G, Zhao L, Zhou G P, Liang Z F 2024 J. Vibration Shock 43 287
[5] Wu Z Z, Zhang Z, Wu D G, Chen Y H, Hu F, Guo C X, Tang L J 2024 Actuators 13 177
Google Scholar
[6] Wang S, Lin S Y 2021 Ultrasonics 111 106299
Google Scholar
[7] He Z J, Jia H, Qiu C Y, Peng S S, Mei X F, Cai F Y, Peng P, Ke Manzhu Liu Z Y. 2010 Phys. Revi. Lett. 105 074301
Google Scholar
[8] Christensen J, Fernandez-Dominguez A I, De Leon-Perez F, Martin-Moreno L, Garcia-Vidal F J 2007 Nat. Phys. 3 851
Google Scholar
[9] 冯俊瑾 2023 博士学位论文 (成都: 电子科技大学)
Feng J J 2023 Ph. D. Dissertation (Chengdu: University of Electronic Science and Technology of China
[10] Zhou Y, Lu M H, Liang F, Xu N, Chen Y F, Zhu Y Y, Zhu S N, Ming N B 2010 Phys. Revi. Lett. 104 164301
Google Scholar
[11] Liu F., Cai F., Ding, Y., Liu Z. 2008 Appl. Phys. Lett. 92 103504
Google Scholar
[12] 卢一铭, 曹东兴, 申永军, 陈许敏 2021 力学学报 53 1114
Google Scholar
Lu Y M, Cao D X, Shen Y J, Chen X M 2021 Chin. J. Theo. Appl. Mech. 53 1114
Google Scholar
[13] 孙伟彬, 王婷, 孙小伟, 康太凤, 谭自豪, 刘子江 2019 68 234206
Google Scholar
Sun W B, Wang T, Sun X W, Kang T F, Tan Z H, Liu Z J 2019 Acta Phys. Sin. 68 234206
Google Scholar
[14] 朱兴一, 钟盛, 叶安珂, 邓富文, 吴睿, 俞悟周 2014 人工晶体学报 43 2852
Google Scholar
Sun W B, Wang T, Sun X W, Kang T F, Tan Z H, Liu Z J 2014 J. Synt. Crys. 43 2852
Google Scholar
[15] 侯丽娜, 侯志林, 傅秀军 2014 63 034305
Google Scholar
Hou L N, Hou Z L, Fu X J 2014 Acta Phys. Sin. 63 034305
Google Scholar
[16] 高东宝, 曾新吾 2014 国防科技大学学报 35 129
Gao D B, Zeng X W J 2013 J. Nati. Univ. Defen. Tech. 35 129
[17] 林基艳, 林书玉, 徐洁, 王升, 钟兴华 2023 中国科学: 物理学 力学 天文学 53 254311
Google Scholar
Lin J Y, Lin S Y, Xu J, Wang S, Zhong X H 2023 Sci. Sin. Phys. Mech. As. 53 254311
Google Scholar
[18] 林基艳, 林书玉 2023 72 094301
Google Scholar
Lin J Y, Lin S Y 2023 Acta Phys. Sin. 72 094301
Google Scholar
[19] 孙琢刚 2023 博士学位论文 (秦皇岛市: 燕山大学)
Sun Z G 2023 Ph. D. Dissertation (Qinhuangdao: Yanshan University
[20] 刘婷 2022 博士学位论文 (哈尔滨: 哈尔滨工程大学)
Liu T 2022 Ph. D. Dissertation (Harbin: Harbin Engineering University
[21] 李雪锋 2011 硕士学位论文 (南京: 南京大学)
Li X F 2011 M. S. Thesis (Nanjing: Nanjing University
[22] 张蒙 2019 硕士学位论文 (沈阳: 沈阳航空航天大学)
Zhang M 2019 M. S. Thesis (Shenyang: Shenyang University of Aeronautics and Astronautics
[23] 郭梦萍 2019 硕士学位论文 (南京: 南京理工大学)
Guo M P 2019 M. S. Thesis (Nanjing: Nanjing University of Science and Technology
[24] 谢素君 2017 硕士学位论文 (吉首: 吉首大学)
Xie S J 2017 M. S. Thesis (Jishou: Jishou University
[25] 毛宇宸, 沈建东, 刘涛, 杨嘉倩, 豆涵杰, 张汪洋, 牟笑静 2024 压电与声光 46 617
Mao Y C, Shen J D, Liu T, Yang J Q, Dou H J, Zhang W Y, Mou X J 2024 Piez. & Acous. 46 617
[26] 郭万里 2022 硕士学位论文 (天津: 河北工业大学)
Guo W L 2022 M. S. Thesis (Tianjin: Hebei University of Technology
DownLoad:
Metrics
- Abstract views: 28
- PDF Downloads: 0
- Cited By: 0
