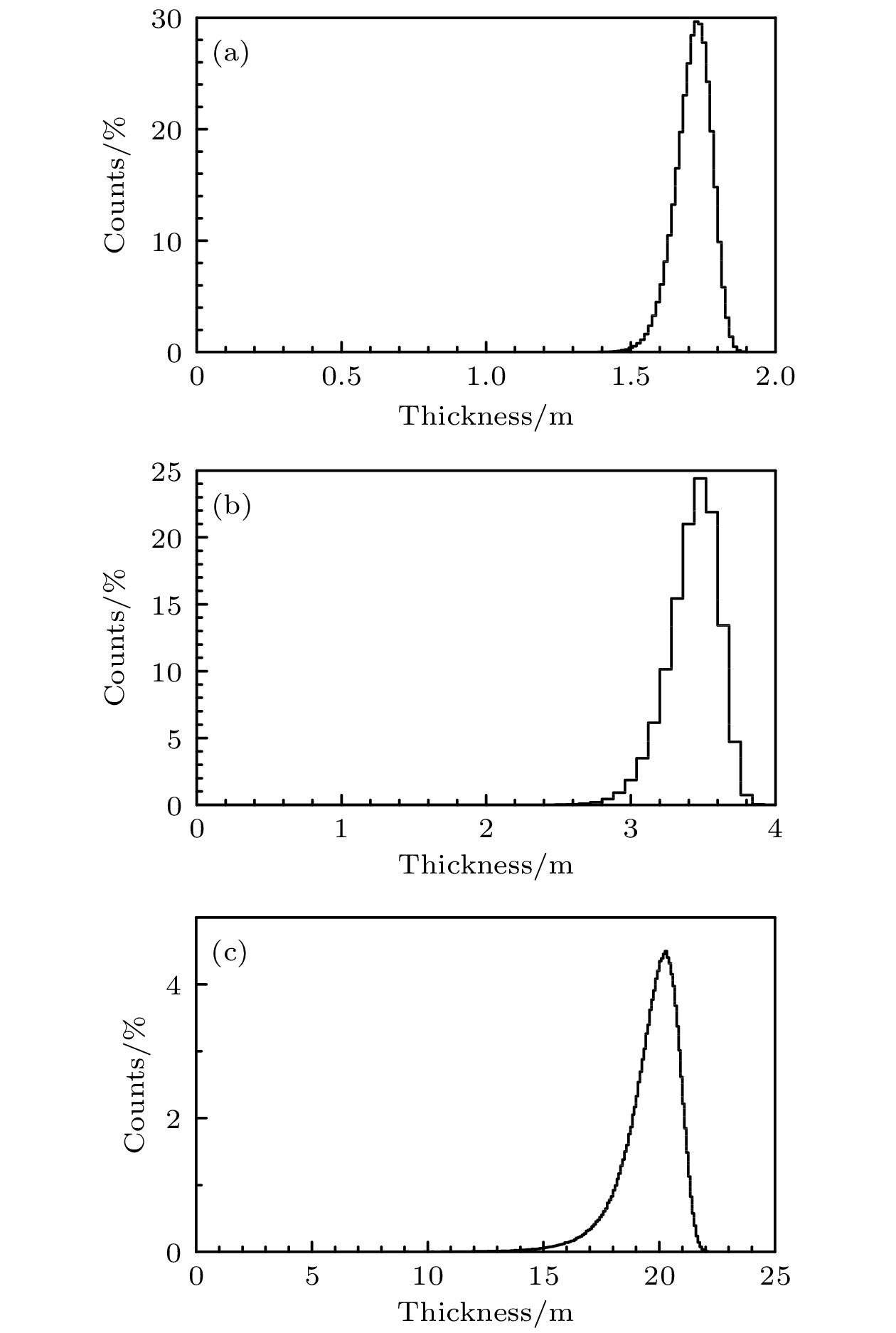
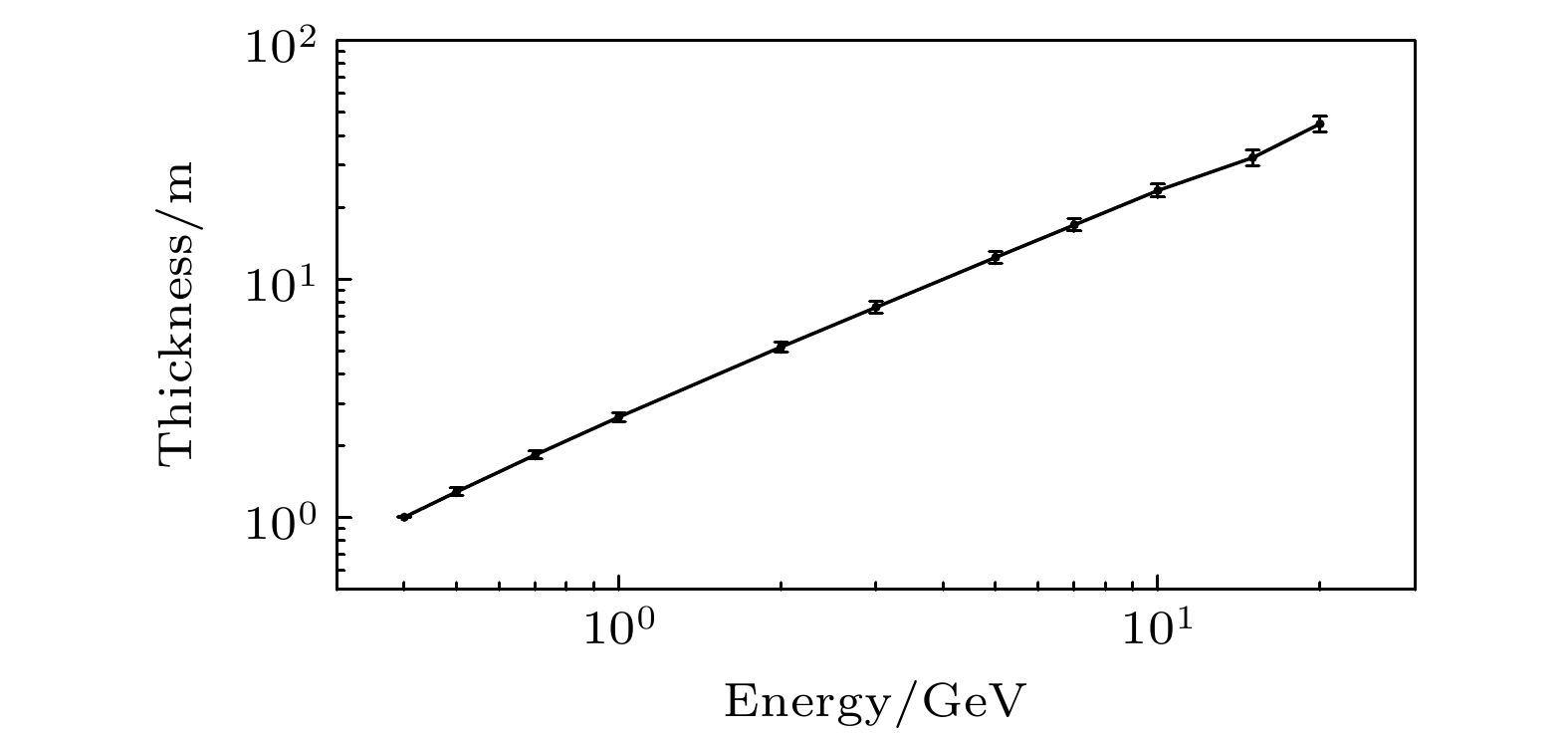
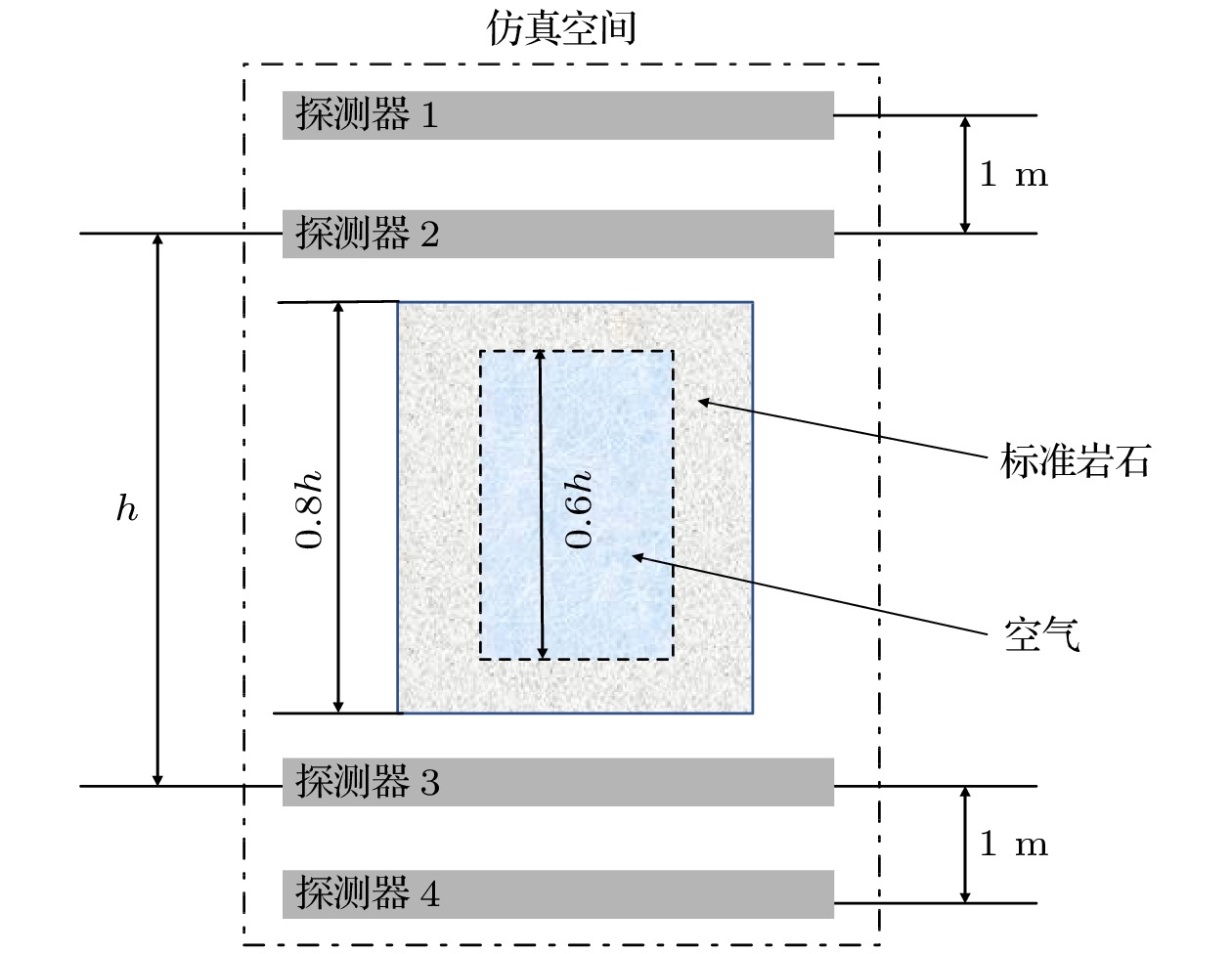
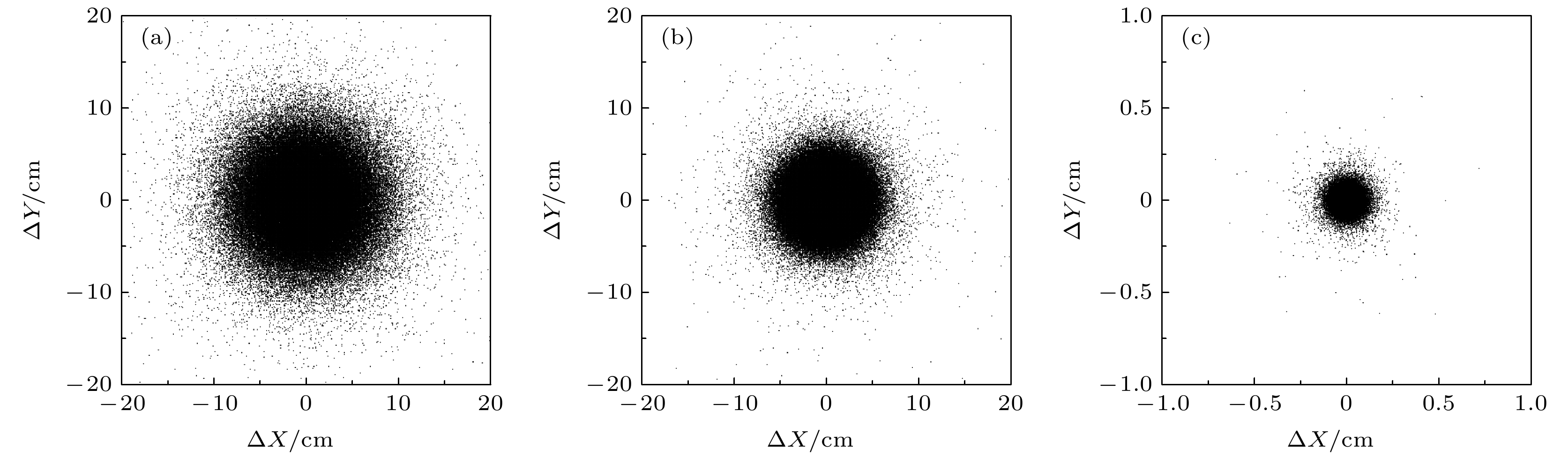
-
The muon transmission imaging method is a non-destructive detection imaging method and can obtain the internal density structure of the target object by analyzing the flux change of cosmic ray muon before and after passing through the target object. This method assumes that muon travels along a straight line in a lowatomic number material. However, the multiple Coulomb scattering causes the muon deviate from the straight line to a certain extent when penetrating the material, which may have a certain influence on the accuracy of muon transmission imaging. This study uses the Geant4 software package to carry out Monte Carlo simulation of muon transmission imaging. Object models with multiple density structures and several meters are used to analyze the effect of multiple scattering on the accuracy of transmission imaging. In the experiment, we set up a model in which muons of different energy vertically pass through a rock with a certain thickness, we can intuitively see the influence of scattering on the penetration path of muons. By setting up rock-water block models with thickness in a range of 0.8, 2.4 and 4.0 m, the effect of Coulomb scattering on the transmission imaging of small-scale material muons is analyzed. The results suggest that the muon transmission imaging method can well restore the geometric shape and spatial distribution characteristics of density anomalies for objects with several-meter scale for standard rock materials with a scale of several meters. However, the flux deviation caused by multiple Coulomb scattering on the muons in the near-vertical direction can reach 5%, and up to 13% in the boundary areas of the standard rock and air. We limit the scattering angle of the muon, and select the muon with a scattering angle of less than or equal to 1° for imaging. The results of transmission imaging by using the selected muon have improved. The image does not have the illusion of an abnormally increased flux around the model caused by scattering, but the muon flux in the model area is reduced even more, thereby affecting the accuracy of restoring the absolute density of an object using flux differences. Therefore, the effects of multiple Coulomb scattering should be considered for recovering more accurate absolute density in small-scale muon transmission imaging study.
-
Keywords:
- multiple Coulomb scattering /
- cosmic ray muons /
- density structure /
- imaging accuracy /
- transmission imaging
[1] Gaisser T K 1990 Cosmic Rays and Particles Physics (Cambridge: Cambridge University Press) p126
[2] Lesparre, Gibert N D, Marteau J, Komorowski J C, Nicollin F, Coutant O 2012 Geophys. J. Inter. 190 1008
Google Scholar
[3] Zhang Z X, Enqvist T, Holma M, Kuusiniemi P 2020 Rock Mech. Rock Eng. 53 4893
Google Scholar
[4] Hou L, Huo Y, Zuo W, Yao Q, Yang J, Zhang Q 2021 Nucl. Eng. Technol. 53 208
Google Scholar
[5] Gilboy W B, Jenneson P M, Simons S J R, Stanley S J, Rhodes D 2007 Nucl. Instrum. Meth. A 580 785
Google Scholar
[6] Borozdin K, Hogan G, Morris C, Priedhorsky W C, Saunders A, Schultz L J, Teasdale M E 2003 Nature 422 277
[7] Schultz L J, Borozdin K N, Gomez J J, Hogan G E, McGill J A, Morris C L, Priedhorsky W C, Saunders A, Teasdale M E 2004 Nucl. Instrum. Meth. A 519 687
Google Scholar
[8] Liu Y Y, Chen Z Q, Zhao Z R, Zhang L, Wang Z T 2009 Tsinghua Sci. Technol. 14 313
Google Scholar
[9] Schultz L J, Blanpied G S, Borozdin K N, Fraser A M, Hengartner N W, Klimenko A V, Morris C L, Orum C, Sossong M J 2007 IEEE Trans. Image Process. 16 1985
Google Scholar
[10] Wang G, Schultz L J, Qi J 2009 IEEE Trans. Image Process. 18 1080
Google Scholar
[11] Gary B, Sankaran K, Dustin D, Craig M, Isabelle B, Michael S, Shawn M K, Beth N 2015 Nucl. Instrum. Meth. A 784 352
Google Scholar
[12] George E P 1955 Commonw. Eng. 1955 455
[13] Alvarez L W, Anderson J A, Bedwei F E, Burkhard J, Fakhry A, Girgis A, Goneid A, Hassan F, Iverson D, Lynch G, Miligy Z, Moussa AH, Sharkawi M, Yazolino L 1970 Science 167 832
Google Scholar
[14] Morishima K, Kuno M, Nishio A, et al. 2017 Nature 552 386
Google Scholar
[15] Han R, Yu Q, Li Z W, Li J T, Cheng Y P, Liao B, Jiang L X, Ni S D, Liu T F, Wang Z 2020 J. Instrum. 15 06019
Google Scholar
[16] Li, J T, Li Z W, Han R, Cheng Y P, Mao X, Yu L, Feng X, Liu B, Jiang L, Ouyang X P 2022 J. Instrum. 17 0502
Google Scholar
[17] Cheng Y P, Han R, Li Z W, Li J T, Mao X, Dou W Q, Feng X Z, Ouyang X P, Liao B, Liu F, Huang L 2022 Nucl. Sci. Tech. 33 88
Google Scholar
[18] 苏宁, 刘圆圆, 王力, 程建平 2022 71 064201
Google Scholar
Su N, Liu Y Y, Wang L, Cheng J P 2022 Acta Phys. Sin. 71 064201
Google Scholar
[19] 何伟波 2019 博士学位论文 (合肥: 中国科学技术大学)
He W B 2019 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[20] Kume N, Miyadera H, Morris C L, Bacon J, Borozdin K N, Durham J M, Fuzita K, Guardincerri E, Izumi M, Nakayama K, Saltus M, Sugita T, Takakura K, Yoshioka K 2016 J. Instrum. 11 09008
Google Scholar
[21] Borozdin K, Greene S, Lukić Z, Milner E, Miyadera H, Morris C, Perry J 2012 Phys. Rev. Lett. 109 152501
Google Scholar
[22] Takamatsu K, Takegami H, Ito C, Suzuki K, Ohnuma H, Hino R, Okumura T 2015 Ann. Nucl. Energy 78 166
Google Scholar
[23] Bethe H A 1953 Phys Rev. 89 1256
Google Scholar
[24] Case C T, Battle E L 1968 Phys. Rev. 169 201
Google Scholar
[25] Hagmann C, Lange D, Wright D 2007 IEEE Nuclear Science Symposium Conference Record Hawaii, United States October 26–November 3, 2007 p1143
[26] Groom D E, Mokhov N V, Striganov S I 2001 Atom. Data Nucl. Data 78 183
Google Scholar
-
图 1 缪子穿透物体的路径
Figure 1. Path of the muon through the object.
图 2 CRY随机抽样产生缪子的能量分布
Figure 2. Energy distribution of muons generated by random sampling of CRY.
图 3 散射模块验证模型(缪子为单能点源, 垂直穿过待测物体)
Figure 3. Verification model of scattering module (A single energy muon at a point source, passing vertically through the object to be measured).
图 4 单能缪子竖直穿透的标准岩石厚度分布 (a) 0.5 GeV; (b) 1.0 GeV; (c) 10.0 GeV
Figure 4. Standard rock thickness distribution for vertical penetration of muons with different energies: (a) 0.5 GeV; (b) 1.0 GeV; (c) 10.0 GeV.
图 5 不同能量缪子能竖直穿透的标准岩石的平均厚度 (误差棒给出1σ误差)
Figure 5. Average thickness of standard rocks penetrated vertically by muons of different energies (Error bar is 1σ).
图 6 多重散射特征分析模型 (100个1.5 GeV的缪子, 垂直穿过3 m厚度的标准岩石(红色线条), 蓝色为缪子径迹)
Figure 6. Multiple scattering feature analysis model (100 muonsof 1.5 GeV vertically passes through a standard rock with a thickness of 3 m (red line), the blue is the muon track).
图 7 岩石-空腔模型透射成像模拟
Figure 7. Rock-cavity model transmission imaging simulation.
图 8 点源缪子竖直穿过1 m厚标准岩石的出射位置分布 (a) 0.7 GeV; (b) 1.0 GeV; (c) 3.0 GeV
Figure 8. Distribution of emission positions of point source muons vertically passing through 1 m thick standard rock: (a) 0.7 GeV; (b) 1.0 GeV; (c) 3.0 GeV
图 9 单能点源缪子穿过不同厚度的标准岩石的多重库仑散射影响 (a) 路径平面平均偏移长度(误差棒给出0.5σ误差); (b) 累计散射角
Figure 9. Multiple Coulomb scattering effects of single-energy point source muons passing through standard rocks with different thicknesses: (a) Average offset length of the path plane (the error bar is 0.5σ); (b) cumulative scattering angle.
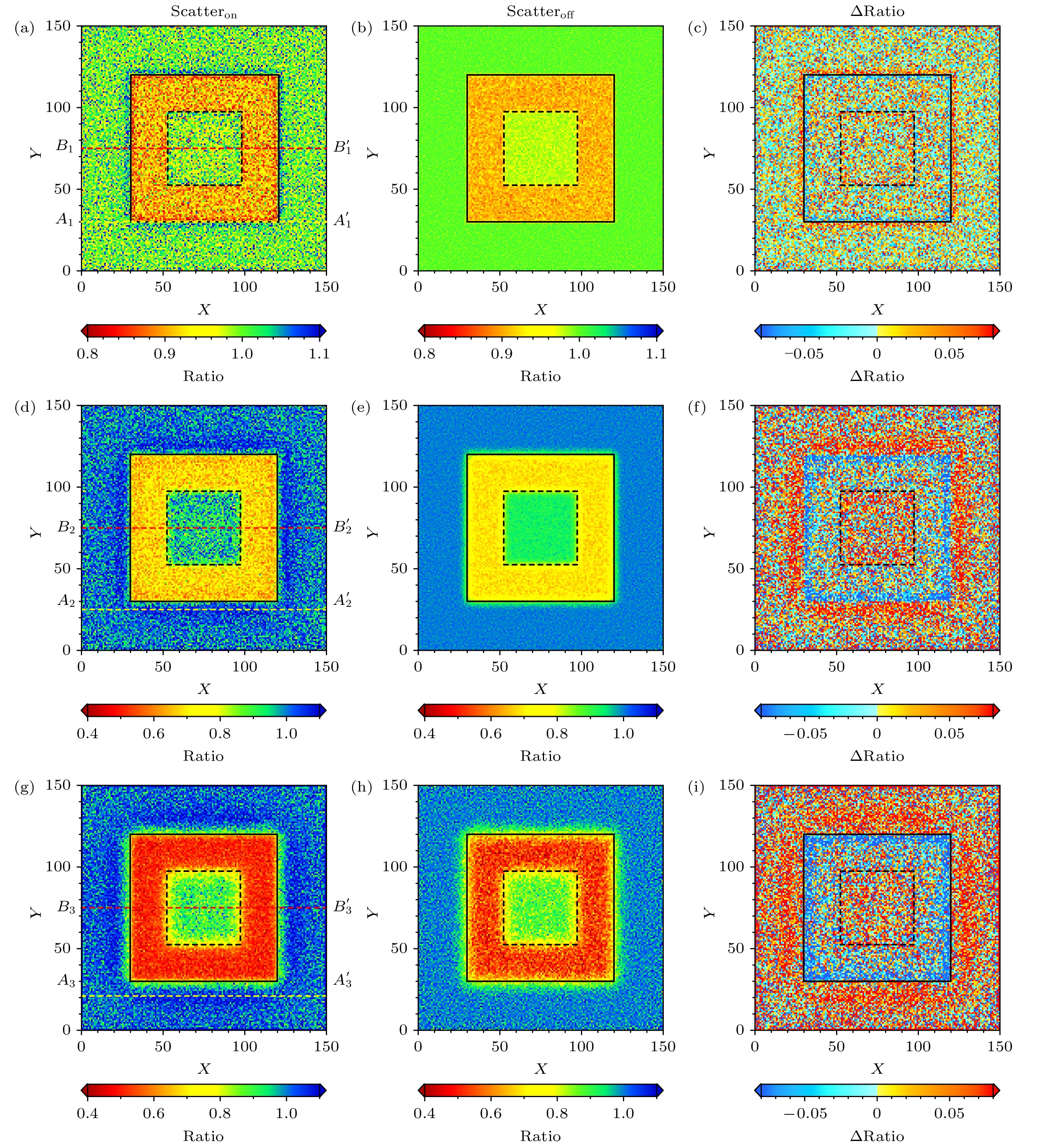
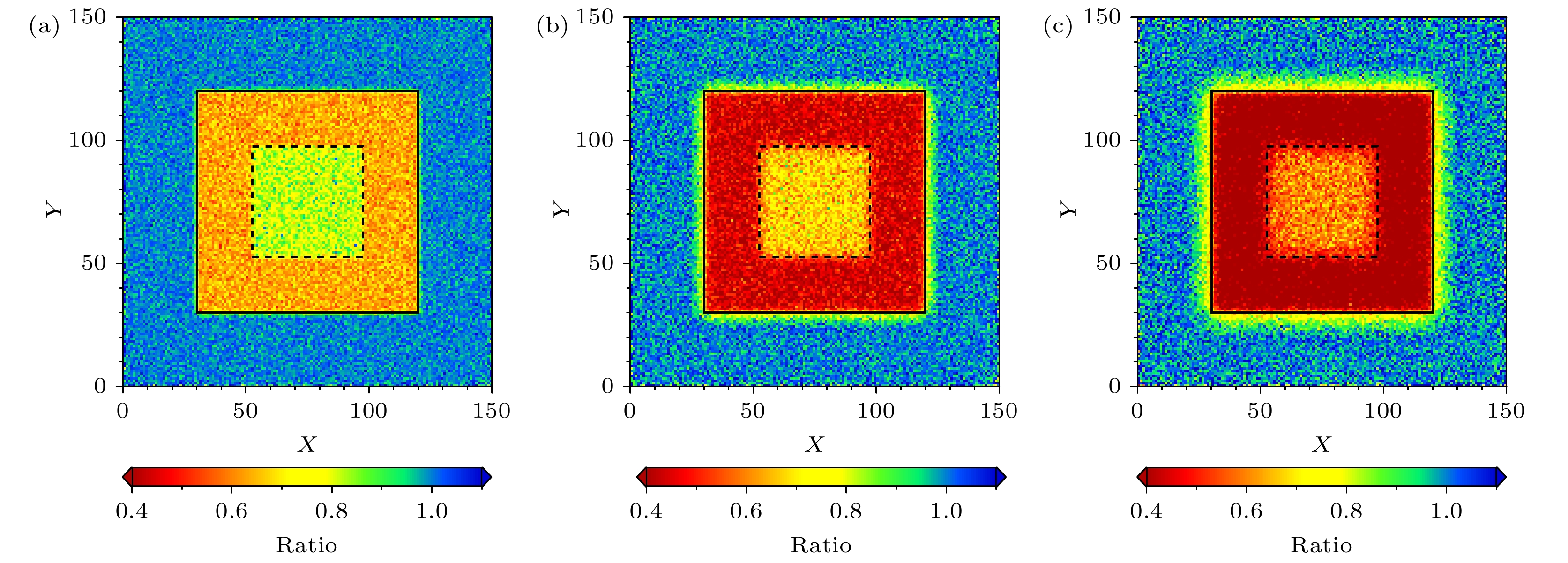
图 10 缪子穿过不同厚度模型前后各像素上计数的比值(俯视图) (a), (d), (g) 0.8 m; (b), (e), (h) 2.4 m; (c), (f), (i) 4.0 m (黑色实线为岩石模型的外边界, 黑色虚线为岩石与空腔的分界)
Figure 10. The ratio of the counts on each pixel before and after the muons pass through the models with different thicknesses (top view): (a), (d), (g) 0.8 m; (b), (e), (h) 2.4 m; (c), (f), (i) 4.0 m (Solid black line is the outer boundary of the rock model, the dashed black line is the boundary between the rock and the cavity).
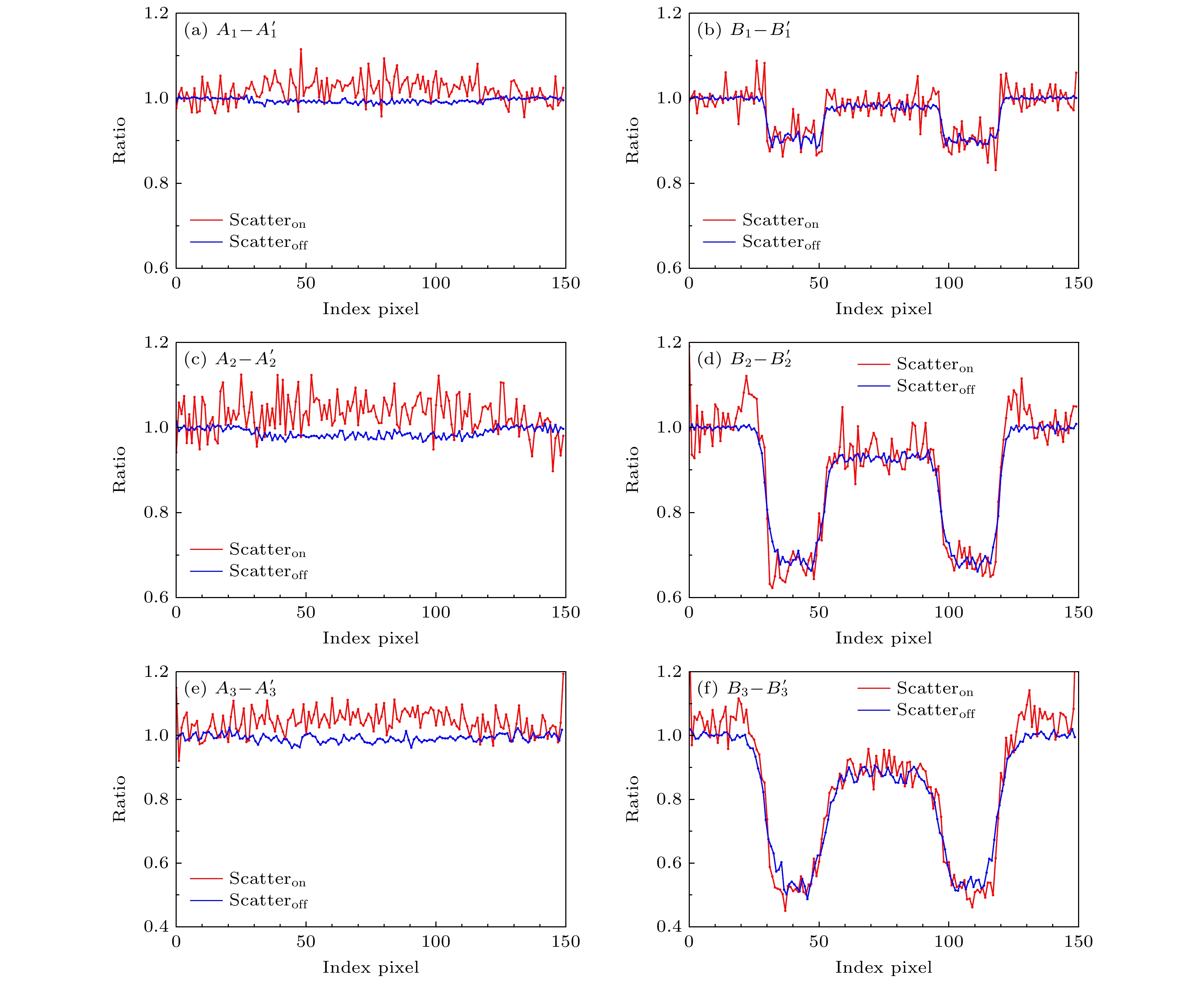
图 11 不同厚度模型切线经过像素的计数比值分布 (a), (d) 0.8 m; (b), (e) 2.4 m; (c), (f) 4.0 m (A-A' 线在岩石和外边界附近, B-B' 切线横穿了岩石-空腔模型 (图10 (a)—(c)))
Figure 11. Distribution of count ratios of the tangent lines passing through pixels for different thickness models: (a), (d) 0.8 m; (b), (e) 2.4 m; (c), (f) 4.0 m (A-A' line is near the rock and the outer boundary, and B-B' tangent traverses the rock-cavity model (Fig. 10 (a)-(c))).
图 12 经过缪子散射角筛选后不同厚度模型缪子透射成像结果(俯视图) (a) 0.8 m; (b) 2.4 m; (c) 4.0 m
Figure 12. Transmission imaging results of the model of different thicknesses after the screening of the muon scattering angle (top view): (a) 0.8 m; (b) 2.4 m; (c) 4.0 m.
表 1 3 GeV的缪子穿过10 cm厚不同材料的散射角
Table 1. Multiple scattering for 3 GeV muons passing through 10 cm of various materials.
材料 X0/cm 理论θ0/mrad 模拟θ0/mrad 相对偏差/% Al 8.99 4.80 4.43 –7.8 Fe 1.80 11.4 10.6 –7.0 Cu 1.48 12.6 12.6 0 U 0.31 29.3 31.7 8.2 
DownLoad: CSV
-
[1] Gaisser T K 1990 Cosmic Rays and Particles Physics (Cambridge: Cambridge University Press) p126
[2] Lesparre, Gibert N D, Marteau J, Komorowski J C, Nicollin F, Coutant O 2012 Geophys. J. Inter. 190 1008
Google Scholar
[3] Zhang Z X, Enqvist T, Holma M, Kuusiniemi P 2020 Rock Mech. Rock Eng. 53 4893
Google Scholar
[4] Hou L, Huo Y, Zuo W, Yao Q, Yang J, Zhang Q 2021 Nucl. Eng. Technol. 53 208
Google Scholar
[5] Gilboy W B, Jenneson P M, Simons S J R, Stanley S J, Rhodes D 2007 Nucl. Instrum. Meth. A 580 785
Google Scholar
[6] Borozdin K, Hogan G, Morris C, Priedhorsky W C, Saunders A, Schultz L J, Teasdale M E 2003 Nature 422 277
[7] Schultz L J, Borozdin K N, Gomez J J, Hogan G E, McGill J A, Morris C L, Priedhorsky W C, Saunders A, Teasdale M E 2004 Nucl. Instrum. Meth. A 519 687
Google Scholar
[8] Liu Y Y, Chen Z Q, Zhao Z R, Zhang L, Wang Z T 2009 Tsinghua Sci. Technol. 14 313
Google Scholar
[9] Schultz L J, Blanpied G S, Borozdin K N, Fraser A M, Hengartner N W, Klimenko A V, Morris C L, Orum C, Sossong M J 2007 IEEE Trans. Image Process. 16 1985
Google Scholar
[10] Wang G, Schultz L J, Qi J 2009 IEEE Trans. Image Process. 18 1080
Google Scholar
[11] Gary B, Sankaran K, Dustin D, Craig M, Isabelle B, Michael S, Shawn M K, Beth N 2015 Nucl. Instrum. Meth. A 784 352
Google Scholar
[12] George E P 1955 Commonw. Eng. 1955 455
[13] Alvarez L W, Anderson J A, Bedwei F E, Burkhard J, Fakhry A, Girgis A, Goneid A, Hassan F, Iverson D, Lynch G, Miligy Z, Moussa AH, Sharkawi M, Yazolino L 1970 Science 167 832
Google Scholar
[14] Morishima K, Kuno M, Nishio A, et al. 2017 Nature 552 386
Google Scholar
[15] Han R, Yu Q, Li Z W, Li J T, Cheng Y P, Liao B, Jiang L X, Ni S D, Liu T F, Wang Z 2020 J. Instrum. 15 06019
Google Scholar
[16] Li, J T, Li Z W, Han R, Cheng Y P, Mao X, Yu L, Feng X, Liu B, Jiang L, Ouyang X P 2022 J. Instrum. 17 0502
Google Scholar
[17] Cheng Y P, Han R, Li Z W, Li J T, Mao X, Dou W Q, Feng X Z, Ouyang X P, Liao B, Liu F, Huang L 2022 Nucl. Sci. Tech. 33 88
Google Scholar
[18] 苏宁, 刘圆圆, 王力, 程建平 2022 71 064201
Google Scholar
Su N, Liu Y Y, Wang L, Cheng J P 2022 Acta Phys. Sin. 71 064201
Google Scholar
[19] 何伟波 2019 博士学位论文 (合肥: 中国科学技术大学)
He W B 2019 Ph. D. Dissertation (Hefei: University of Science and Technology of China) (in Chinese)
[20] Kume N, Miyadera H, Morris C L, Bacon J, Borozdin K N, Durham J M, Fuzita K, Guardincerri E, Izumi M, Nakayama K, Saltus M, Sugita T, Takakura K, Yoshioka K 2016 J. Instrum. 11 09008
Google Scholar
[21] Borozdin K, Greene S, Lukić Z, Milner E, Miyadera H, Morris C, Perry J 2012 Phys. Rev. Lett. 109 152501
Google Scholar
[22] Takamatsu K, Takegami H, Ito C, Suzuki K, Ohnuma H, Hino R, Okumura T 2015 Ann. Nucl. Energy 78 166
Google Scholar
[23] Bethe H A 1953 Phys Rev. 89 1256
Google Scholar
[24] Case C T, Battle E L 1968 Phys. Rev. 169 201
Google Scholar
[25] Hagmann C, Lange D, Wright D 2007 IEEE Nuclear Science Symposium Conference Record Hawaii, United States October 26–November 3, 2007 p1143
[26] Groom D E, Mokhov N V, Striganov S I 2001 Atom. Data Nucl. Data 78 183
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4239
- PDF Downloads: 106
- Cited By: 0
