-
Owing to the inhomogeneity of the refractive index inside the sample (e.g. biological tissue) or on the surface of the sample(e.g. ground glass), light will be strongly scattered when it propagates through the sample. Therefore, we can hardly obtain the information about the objects behind the scattering medium, except for only a complex speckle pattern. To date, many approaches to realize focusing and imaging through scattering medium have been put forward. The traditional method mainly utilizes ballistic photons for imaging through scattering medium. Since the ballistic light is attenuated exponentially with the increase of depth of propagation in the scattering medium, the reconstruction from the speckle formed by scattered light is more conducive to practicability such as deep biomedical imaging. Typically, the wavefront shaping, optical transmission matrix and speckle correlation techniques which can successfully recover hidden object from the speckle, are valuable in biomedical imaging field. However, both optical transmission matrix and wavefront shaping rely on the coherence of light waves. The physical model of speckle correlation imaging is limited by the similarity of the point spread function of the imaging system. Thus, it is restrictive to achieve imaging through random scattering medium with broadband light illumination by using the current techniques. In this paper, we present a broadband scattering imaging method based on common-mode rejection of polarization characteristic. In order to solve the problem that current scattering imaging methods are limited by the spectral width of the light source illumination, the polarization characteristic of the speckle field is explored in depth. We qualitatively analyze the difference in polarization information between the hidden object and the background noise in the speckle field. Notably, owing to the differences among autocorrelation functions of the speckle field intensity with different rotate angles of polarization, we can obtain two images where the object information contained in the speckle field and the background noise are dominant. Specifically, two speckle patterns are selected according to the maximum value and minimum value of the peak-to-correlation energy of the different speckles’ intensity autocorrelation. Afterwards, the serious background noise caused by the broadband light illumination is significantly suppressed by using polarization speckle difference imaging, and then the hidden object is reconstructed, with basic phase retrieval algorithm combined. Comparison with conventional speckle correlation imaging technique, the value of peak signal-to-noise ratio and structural similarity index of reconstructions through using the proposed method are improved significantly, and the fitting curves are stabilized. Emphatically, the background noise item is physically handled by developing a novel physical imaging model. Furthermore, the proposed method is highly efficient and universal to recover different types of the hidden objects with better quality under broadband light illumination. Therefore, the proposed method has more potential applications in scattering imaging and biomedical imaging. -
Keywords:
- broadband scattering imaging /
- polarization /
- difference imaging
[1] Goodman J W 2007 Speckle phenomena in optics: theory and applications (Englewood: Roberts & Company) pp1–6
[2] Popoff S M, Lerosey G, Carminati R, Fink M, Boccara A C, Gigan S 2010 Phys. Rev. Lett. 104 100601
Google Scholar
[3] Popoff S M, Lerosey G, Fink M, Boccara A C, Gigan S 2010 Nat. Commun. 1 1
[4] Liu J T, Wang J N, Li W, Sun X Y, Zhu L, Guo C F, Shao X P 2018 IEEE Photonics J. 10 6900811
[5] Bertolotti J, van Putten E G, Blum C, Lagendijk A, Vos W L, Mosk A P 2012 Nature 491 232
Google Scholar
[6] Wang G, Liu J T, Sun X Y, He S F, Guo C F, Wu Y X, Shao X P 2020 Opt. Commun. 463 125361
Google Scholar
[7] He H X, Guan Y F, Zhou J Y 2013 Opt. Express 21 12539
Google Scholar
[8] Horstmeyer R, Ruan H, Yang C 2015 Nat. Photonics 9 563
Google Scholar
[9] Wan L, Chen Z, Huang H, Pu J X 2016 Appl. Phys. B 122 1
[10] Xu X Q, Xie X S, He H X, Zhuang H C, Zhou J Y, Thendiyammal A, Mosk A P 2017 Opt. Express 25 32829
[11] Liu J T, Li W, Wu Y X, He S F, Xia M R, Liang W H, Fan Z Z, Song Y F, Shao X P 2021 Laser & Optoelectronics Progress 58 1
[12] Katz O, Heidmann P, Fink M, Gigan S 2014 Nat. Photonics 8 784
Google Scholar
[13] Feng S C, Kane C, A. Lee P, Stone A 1988 Phys. Rev. Lett. 61 834
Google Scholar
[14] Freund I, Rosenbluh M, Feng S C 1988 Phys. Rev. Lett. 61 2328
Google Scholar
[15] 代伟佳 2015 硕士学位论文 (西安: 西安电子科技大学)
Dai W J 2015 M. S. Thesis (Xi’an: Xidian University) (in Chinese)
[16] Wu P F, Liang Z, Zhao X, Su L, Song L P, 2017 Appl. Opt. 56 3335
Google Scholar
[17] 李慧娟 2017 硕士学位论文 (西安: 西安电子科技大学)
Li H J 2017 M. S. Thesis (Xi’an: Xidian University) (in Chinese)
[18] Li H J, Wu T F, Liu J T, Gong C M, Shao X P 2016 Appl. Opt. 55 9731
Google Scholar
[19] Guo C F, Liu J T, Wu T F, Zhu L, Shao X P 2018 Appl. Opt. 57 905
Google Scholar
[20] Fienup J R 1978 Opt. Lett. 3 27
Google Scholar
[21] Goodman J W 2007 Speckle Phenomena in Optics: Theory and Applications (Englewood: Roberts & Company) pp40–42
[22] Kadambi A, Taamazyan V, Shi B X, Raskar R 2017 Int. J. Comput. Vision 125 34
Google Scholar
[23] 刘宾, 赵鹏翔, 赵霞, 罗悦, 张立超 2020 69 184202
Google Scholar
Liu B, Zhao P X, Zhao X, Luo Y, Zhang L C 2020 Acta Phys. Sin. 69 184202
Google Scholar
[24] Liu F, Wei Y, Han P L, Yang K, Bai L, Shao X P 2019 Opt. Express 27 3629
Google Scholar
[25] Tyo J S 1998 J. Opt. Soc. Am. A 15 359
[26] 吴腾飞 2017 博士学位论文 (西安: 西安电子科技大学)
Wu T F 2017 Ph. D. Dissertation (Xi’an: Xidian University) (in Chinese)
[27] Kumar B V K V, Shi W, Hendrix C 1990 Opt. Lett. 15 807
Google Scholar
[28] 韩平丽, 刘飞, 张广, 陶禹, 邵晓鹏 2018 67 054202
Google Scholar
Han P L, Liu F, Zhang G, Tao Y, Shao X P 2018 Acta Phys. Sin. 67 054202
Google Scholar
[29] Fienup J R 1982 Appl. Opt. 21 2758
Google Scholar
[30] 干红平, 张涛, 花燚, 舒君, 何立军 2021 70 038402
Google Scholar
Gan H P, Zhang T, Hua Y, Shu J, He L J 2021 Acta Phys. Sin. 70 038402
Google Scholar
[31] Wang Z, Bovik A C, Sheikh H R, Simoncelli E P 2004 IEEE Trans. Image Process. 13 600
Google Scholar
-
图 1 基于宽谱光源照明的透过散射介质成像原理示意图
Figure 1. Schematic of imaging through scattering medium with broadband light illumination.
图 2 宽谱照明下探测器前有无窄带滤波器的散斑对比图 (a)探测器前有窄带滤波器; (b)探测器前无窄带滤波器; (c)窄谱照明散斑图像局部放大结果; (d)宽谱照明散斑图像局部放大结果
Figure 2. Speckle patterns with or without narrowband filter in front of the detector under broadband light illumination: (a) With narrowband filter; (b) without narrowband filter; (c) zoomed-in view of the region of interest in speckle image with narrowband light illumination; (d) zoomed-in view of the region of interest in speckle image with broadband light illumination.
图 3 宽谱散斑光场的偏振特性分析 (a)基于宽谱光源照明的偏振散射成像系统; (b)不同偏振方位角宽谱散斑图像的均值强度分布曲线
Figure 3. Polarization characteristics analysis of broadband speckle field: (a) Polarization scattering imaging system with broadband light illumination; (b) the fitting curve between different rotated angles of polarizer and the mean intensity of broadband speckle.
图 4 宽谱散斑光场的偏振度归一化强度分布图
Figure 4. The normalized intensity distribution of the degree of polarization of broadband speckle.
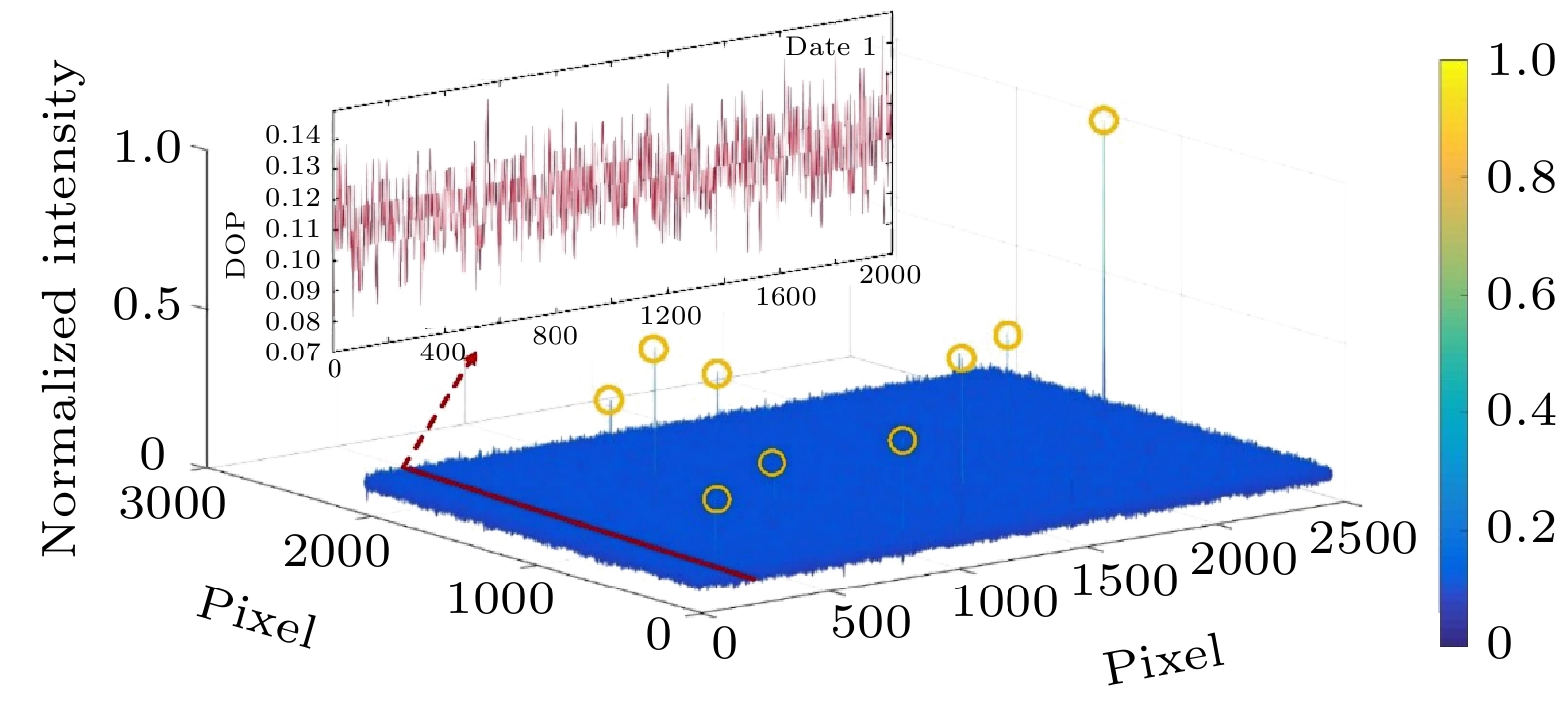
图 5 不同偏振方位角散斑图像中目标和背景的强度变化 (a)目标与背景的强度分布曲线(O_1和O_2分别表示图(b)中表征目标信息的绿色和红色区域散斑颗粒强度分布, B为图(b)中表征背景信息的蓝色区域散斑颗粒强度分布); (b)不同偏振方位角散斑图像 (P1, P2, P3, ⋅⋅⋅, Pn表示探测器前偏振片在不同旋转方位角采集的散斑图样)
Figure 5. The intensity of the object and background as a function of different rotated angles of polarizer: (a) The fitting curves (O_1 and O_2 respectively represent the object information intensity distribution of speckle particles in the green and red regions of the figure (b), and B represents the background information intensity distribution of the speckle particles in the blue region of the figure (b)); (b) speckles with different rotated angles of polarizer (P1, P2, P3, ⋅⋅⋅, Pn represent the speckle patterns obtained by the polarizer in front of the detector at different rotated angles).
图 6 不同偏振调制的宽谱散斑自相关信息对比图 (a)散斑场的自相关; (b)自相关函数中心归一化强度分布曲线
Figure 6. Autocorrelation images of broadband speckles under two different rotated angles of polarizer: (a) The autocorrelation images of speckles; (b) the normalized intensity curves of the center of autocorrelation images.
图 7 不同偏振方位角散斑自相关函数的PCE曲线
Figure 7. PCE curve of speckle autocorrelation functions with different rotated angles of polarizer.
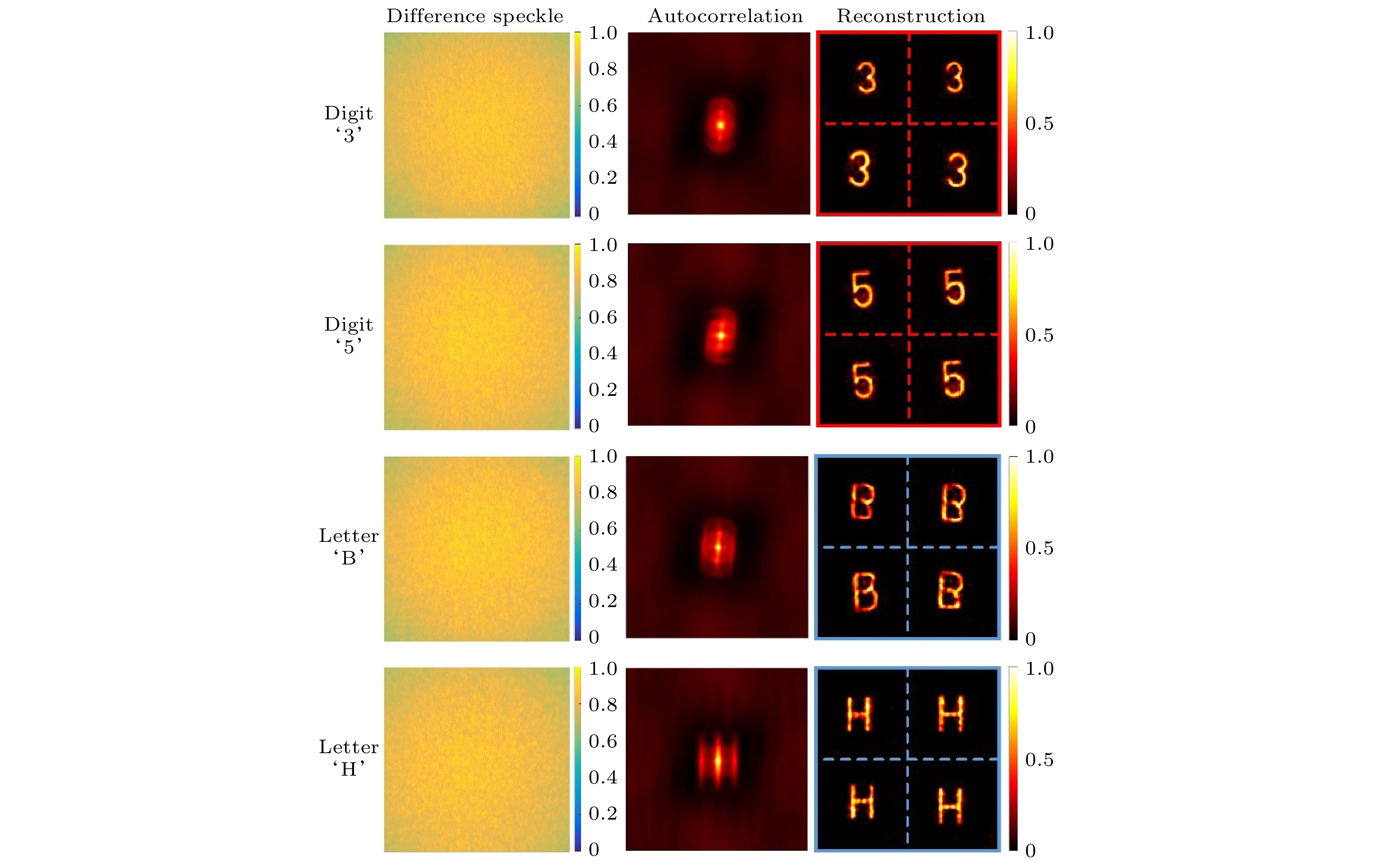
图 8 三种处理方法的重建结果对比图 (a)散斑原图重建; (b)传统的散斑相关散射成像方法; (c)基于散斑光场偏振共模抑制性的宽谱散射成像方法
Figure 8. Reconstruction images of three different methods: (a) Original speckle reconstruction; (b) traditional speckle correlation imaging method with filter; (c) broadband scattering imaging method based on common-mode rejection of polarization characteristic.
图 9 传统的预处理散射成像与本文所述宽谱散射成像方法重建结果的分析对比曲线 (a) PSNR对比曲线; (b) SSIM对比曲线
Figure 9. The PSNR and SSIM curves of reconstructions using traditional scattering imaging method and the proposed method: (a) PSNR curves; (b) SSIM curves.
图 10 不同目标在本文所述宽谱散射成像方法的实验结果
Figure 10. Reconstruction images of different objects using the proposed method.
-
[1] Goodman J W 2007 Speckle phenomena in optics: theory and applications (Englewood: Roberts & Company) pp1–6
[2] Popoff S M, Lerosey G, Carminati R, Fink M, Boccara A C, Gigan S 2010 Phys. Rev. Lett. 104 100601
Google Scholar
[3] Popoff S M, Lerosey G, Fink M, Boccara A C, Gigan S 2010 Nat. Commun. 1 1
[4] Liu J T, Wang J N, Li W, Sun X Y, Zhu L, Guo C F, Shao X P 2018 IEEE Photonics J. 10 6900811
[5] Bertolotti J, van Putten E G, Blum C, Lagendijk A, Vos W L, Mosk A P 2012 Nature 491 232
Google Scholar
[6] Wang G, Liu J T, Sun X Y, He S F, Guo C F, Wu Y X, Shao X P 2020 Opt. Commun. 463 125361
Google Scholar
[7] He H X, Guan Y F, Zhou J Y 2013 Opt. Express 21 12539
Google Scholar
[8] Horstmeyer R, Ruan H, Yang C 2015 Nat. Photonics 9 563
Google Scholar
[9] Wan L, Chen Z, Huang H, Pu J X 2016 Appl. Phys. B 122 1
[10] Xu X Q, Xie X S, He H X, Zhuang H C, Zhou J Y, Thendiyammal A, Mosk A P 2017 Opt. Express 25 32829
[11] Liu J T, Li W, Wu Y X, He S F, Xia M R, Liang W H, Fan Z Z, Song Y F, Shao X P 2021 Laser & Optoelectronics Progress 58 1
[12] Katz O, Heidmann P, Fink M, Gigan S 2014 Nat. Photonics 8 784
Google Scholar
[13] Feng S C, Kane C, A. Lee P, Stone A 1988 Phys. Rev. Lett. 61 834
Google Scholar
[14] Freund I, Rosenbluh M, Feng S C 1988 Phys. Rev. Lett. 61 2328
Google Scholar
[15] 代伟佳 2015 硕士学位论文 (西安: 西安电子科技大学)
Dai W J 2015 M. S. Thesis (Xi’an: Xidian University) (in Chinese)
[16] Wu P F, Liang Z, Zhao X, Su L, Song L P, 2017 Appl. Opt. 56 3335
Google Scholar
[17] 李慧娟 2017 硕士学位论文 (西安: 西安电子科技大学)
Li H J 2017 M. S. Thesis (Xi’an: Xidian University) (in Chinese)
[18] Li H J, Wu T F, Liu J T, Gong C M, Shao X P 2016 Appl. Opt. 55 9731
Google Scholar
[19] Guo C F, Liu J T, Wu T F, Zhu L, Shao X P 2018 Appl. Opt. 57 905
Google Scholar
[20] Fienup J R 1978 Opt. Lett. 3 27
Google Scholar
[21] Goodman J W 2007 Speckle Phenomena in Optics: Theory and Applications (Englewood: Roberts & Company) pp40–42
[22] Kadambi A, Taamazyan V, Shi B X, Raskar R 2017 Int. J. Comput. Vision 125 34
Google Scholar
[23] 刘宾, 赵鹏翔, 赵霞, 罗悦, 张立超 2020 69 184202
Google Scholar
Liu B, Zhao P X, Zhao X, Luo Y, Zhang L C 2020 Acta Phys. Sin. 69 184202
Google Scholar
[24] Liu F, Wei Y, Han P L, Yang K, Bai L, Shao X P 2019 Opt. Express 27 3629
Google Scholar
[25] Tyo J S 1998 J. Opt. Soc. Am. A 15 359
[26] 吴腾飞 2017 博士学位论文 (西安: 西安电子科技大学)
Wu T F 2017 Ph. D. Dissertation (Xi’an: Xidian University) (in Chinese)
[27] Kumar B V K V, Shi W, Hendrix C 1990 Opt. Lett. 15 807
Google Scholar
[28] 韩平丽, 刘飞, 张广, 陶禹, 邵晓鹏 2018 67 054202
Google Scholar
Han P L, Liu F, Zhang G, Tao Y, Shao X P 2018 Acta Phys. Sin. 67 054202
Google Scholar
[29] Fienup J R 1982 Appl. Opt. 21 2758
Google Scholar
[30] 干红平, 张涛, 花燚, 舒君, 何立军 2021 70 038402
Google Scholar
Gan H P, Zhang T, Hua Y, Shu J, He L J 2021 Acta Phys. Sin. 70 038402
Google Scholar
[31] Wang Z, Bovik A C, Sheikh H R, Simoncelli E P 2004 IEEE Trans. Image Process. 13 600
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 8516
- PDF Downloads: 195
- Cited By: 0
