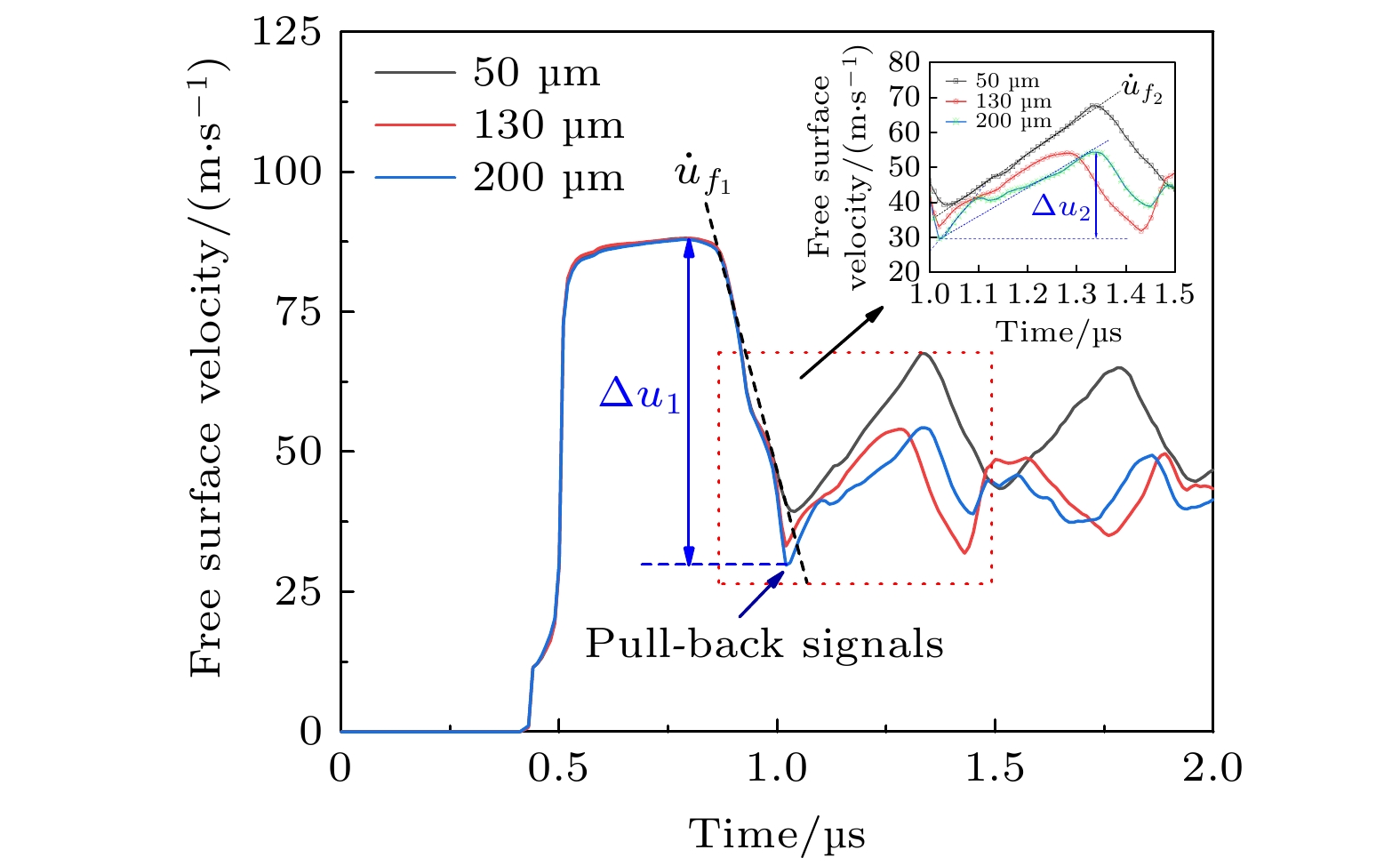
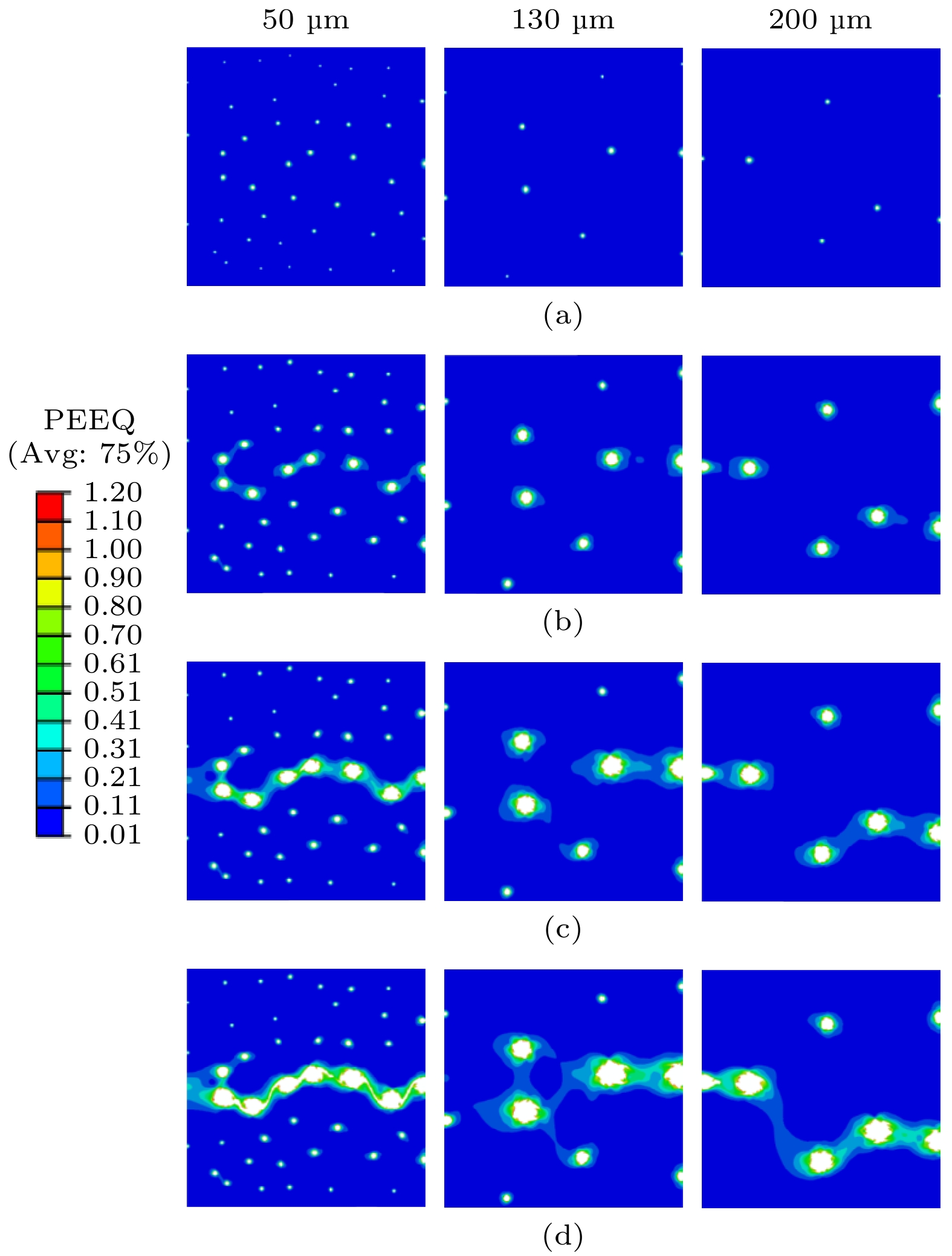
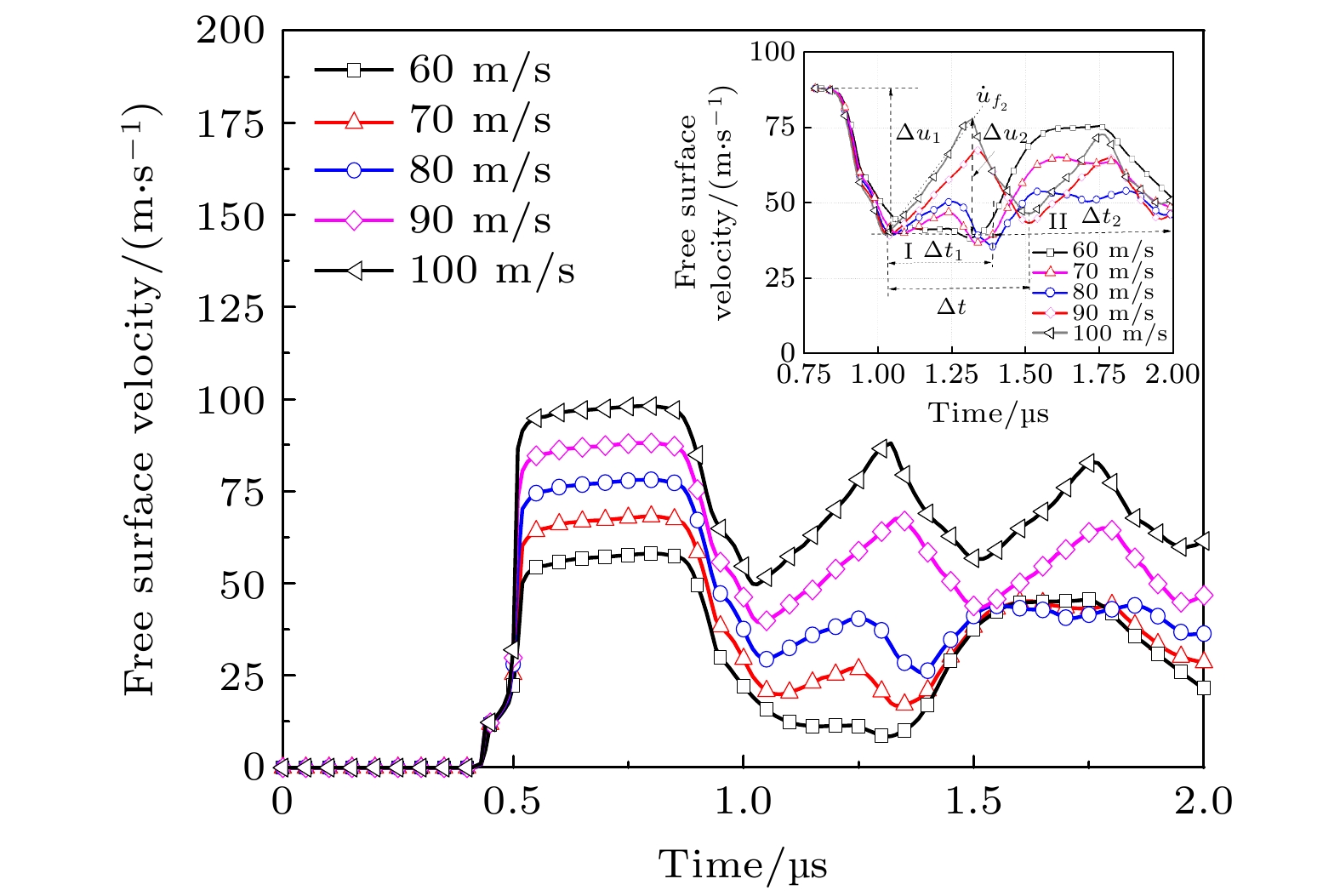
-
High-purity (HP) copper targets with grain sizes of 50, 130 and 200 μm are constructed by using the Voronoi method. Damage nucleation points are randomly prefabricated at the grain boundaries. A two-dimensional axisymmetric finite element model is established to simulate the spallation experiment of HP copper target. The effects of grain size and loading stress on the macro- mechanical response and meso-damage evolution of HP copper spallation are studied and compared with the relevant experimental results. Based on the analysis of free surface velocity profiles, the effects of grain size on the location of pull back velocity rebound point, velocity rebound slope and velocity rebound amplitude are revealed. It is demonstrated that the spalling strength corresponds to the peak value of tensile stress in the damage zone, which essentially represents the critical stress of micro damage nucleation or early growth. Based on the characteristic analysis of damage evolution nephogram, the evolution process of localized plastic strain field around the micro-voids in the growth and coalescence process is reproduced, and the strong dependence of micro-void coalescence behavior on grain size is clarified. The loading stress amplitude has little effect on the location of pull back velocity rebound point, but has a significant effect on the growth and coalescence behavior of micro-voids. The slope and amplitude of pull back velocity rebound increase with loading stress increasing, which is consistent with the relevant experimental result. With the increase of the loading stress, the micro-voids grow from independent growth to coalescence, thus forming spalling surface. The physical process of damage evolution determines the wave oscillation characteristics after the pull-back rebound point. The numerical simulation results reproduce the physical process of damage evolution and its influence on the macroscopic mechanical response, which is of great significance for further understanding spall damage evolution mechanism and theoretical model construction.
-
Keywords:
- spall fracture /
- grain size /
- numerical simulation /
- damage evolution
[1] Antoun T H, Seaman L, Curran D R, Kanel G I, Razorenov S V, Utkin A V 2003 Spall Fracture (New York: Springer-Verlag)
[2] Koller D D, Hixson R S 2005 J. Appl. Phys. 98 103518
Google Scholar
[3] 彭辉, 李平, 裴晓阳, 贺红亮, 程和平, 祁美兰 2014 63 196202
Google Scholar
Peng H, Li P, Pei X Y, He H L, Cheng H P, Qi M L 2014 Acta Phys. Sin. 63 196202
Google Scholar
[4] Johnson J N, Gray G T, Bourne N K 1999 J. Appl. Phys. 86 4892
Google Scholar
[5] Kanel G, Razorenov S, Bogatch A, Utkin A, Grady D 1997 Int. J. Impact. Eng. 20 467
Google Scholar
[6] Escobedo J P, Dennis-Koller D, Ceretta E K, Patterson B M, Bronkhorst C A, Hanson BL, Tonks D, Lebensohn R A 2011 J. Appl. Phys. 110 033513
Google Scholar
[7] Wang Y G, Qi M L, He H L, Wang L L 2014 Mech. Mater. 69 270
Google Scholar
[8] 裴晓阳, 彭辉, 贺红亮, 李平 2015 64 054601
Google Scholar
Pei X Y, Peng H, He H L, Li P 2015 Acta Phys. Sin. 64 054601
Google Scholar
[9] Lieberman E J, Lebensohn R A, Menasche D B, Bronkhorst C A, Rollett A D 2016 Acta Mater. 116 270
Google Scholar
[10] Turley W D, Fensin S J, Hixson R S, Jones R D, La Lone M B, Stevens G D, Thomas S A, Veeser L R 2018 J. Appl. Phys. 123 055102
Google Scholar
[11] Johnson J N 1981 J. Appl. Phys. 52 2812
Google Scholar
[12] Moninari A, Wright TW 2005 J. Mech. Phys. Solids 53 1476
Google Scholar
[13] Lubarda V A, Schneider M S, Kalantar D H, Remington B R, Meyers M A 2004 Acta Mater. 52 1397
Google Scholar
[14] Jacques N, Czarnota C, Mercier S, Molinari A 2015 Int. J. Fract. 162 159
[15] Wilkerson J W, Ramesh K T 2016 J. Mech. Phys. Solids 86 94
Google Scholar
[16] Wilkerson J W 2017 Int J. Plast. 95 21
Google Scholar
[17] Zurek A K, Thissell W R, Johnson J N, Tonks D L, Hixson R 1996 J. Mater. Process. Technol. 60 261
Google Scholar
[18] Wang Y G, He H L, Wang L L 2013 Mech. Mater. 56 131
Google Scholar
[19] Seppälä ET, Belak J, Rudd R E 2004 Phys. Rev. B 69 134101
Google Scholar
[20] Lou S N, Germann T C, Tonks D L 2009 J. Appl. Phys. 106 123518
Google Scholar
[21] 邓小良, 祝文军, 宋振飞, 贺红亮, 经福谦 2009 58 4772
Google Scholar
Deng X L, Zhu W J, Song Z F, He H L, Jing F Q 2009 Acta Phys. Sin. 58 4772
Google Scholar
[22] Becker R, LeBlanc M M, Cazamias J U 2007 J. Appl. Phys. 102 093512
Google Scholar
[23] Becker R 2017 Int. J. Fract. 208 5
Google Scholar
[24] Becker R, Callaghan K 2018 Int. J. Fract. 209 235
Google Scholar
[25] Trivedi P B, Asay J R, Gupta Y M, Field D P 2007 J. Appl. Phys. 102 083513
Google Scholar
[26] Schwartz A J, Cazamias J U, Fiske P S, Minich R W 2002 AIP Conf. Proc. 620 491
Google Scholar
[27] Chen T, Jiang Z X, Peng H, He H L, Wang L L, Wang Y G 2015 Strain 51 190
Google Scholar
[28] 张凤国, 周洪强 2013 62 164601
Google Scholar
Zhang F G, Zhou H Q 2013 Acta Phys. Sin. 62 164601
Google Scholar
[29] Johnson G R, Cook W H 1983 Eng. Fract. Mech. 21 541
[30] Novikov S A 1967 J. Appl. Meth. Tech. Phys. 3 109
[31] Chen D N, Yu Y Y, Yin Z H, Wang H R, Liu G Q 2005 Int. J. Impact Eng. 31 811
Google Scholar
[32] Kanel G I 2010 Int. J. Fract. 163 173
Google Scholar
[33] Seppälä ET, Belak J, Rudd R E 2004 Phys. Rev. Lett. 93 245503
Google Scholar
[34] 王永刚, 胡剑东, 祁美兰, 贺红亮 2011 60 126201
Google Scholar
Wang Y G, Hu J D, Qi M L, He H L 2011 Acta Phys. Sin. 60 126201
Google Scholar
-
图 1 三种晶粒尺寸高纯铜靶体层裂实验的几何建模
Figure 1. Geometric modeling of high purity copper sample with three grain sizes.
图 2 计算得到自由面速度时程曲线与实验结果的对比
Figure 2. Comparison of simulated free surface velocity profile with the experimental result.
图 3 不同晶粒度高纯铜靶体的自由面速度时程曲线及局部放大曲线
Figure 3. Free surface velocity profiles of HP copper with different grain sizes.
图 4 不同晶粒度高纯铜损伤区典型单元的应力时程曲线
Figure 4. Stress profiles of elements in the damage zone of HP copper with different grain sizes.
图 5 层裂实验中X-t波系图
Figure 5. Schematic diagram of X- t wave interactions in spallation experiment.
图 6 不同时刻下不同晶粒度高纯铜的细观损伤演化云图
Figure 6. Mesoscopic damage distribution of HP copper with different grain sizes at different times.
图 7 不同晶粒度高纯铜内部损伤度时程曲线
Figure 7. Damage degree evolution profiles of HP copper with different grain sizes.
图 8 不同撞击速度下高纯铜自由面速度时程曲线及局部放大
Figure 8. Free surface velocity profiles of HP copper at different impact velocities.
图 9 不同撞击速度下高纯铜损伤区单元应力时程曲线
Figure 9. Stress profiles of damaged elements in HP copper spallation experiment.
图 10 不同撞击速度下高纯铜细观损伤演化物理过程 (a) V = 60 m/s; (b) V = 70 m/s; (c) V = 80 m/s; (d) V = 90 m/s; (e) V = 100 m/s
Figure 10. Microscopic damage evolution process of high-purity copper under different impact velocities: (a)V = 60 m/s; (b) V = 70 m/s; (c) V = 80 m/s; (d) V = 90 m/s; (e) V = 100 m/s.
ρ/(kg·m–3) A/MPa B/MPa C m n $ {\dot{\varepsilon }}_{0} $ Troom Tmelt 8910 90 292 0.025 1.09 0.31 1 300 1356 $ {c}_{0} $/(m·s–1) s $ {\varGamma }_{0} $ $ G/\mathrm{G}\mathrm{P}\mathrm{a} $ d1 d2 d3 d4 d5 3910 1.51 2 47 1.08 4.89 3.03 0.014 1.12 
DownLoad: CSV
-
[1] Antoun T H, Seaman L, Curran D R, Kanel G I, Razorenov S V, Utkin A V 2003 Spall Fracture (New York: Springer-Verlag)
[2] Koller D D, Hixson R S 2005 J. Appl. Phys. 98 103518
Google Scholar
[3] 彭辉, 李平, 裴晓阳, 贺红亮, 程和平, 祁美兰 2014 63 196202
Google Scholar
Peng H, Li P, Pei X Y, He H L, Cheng H P, Qi M L 2014 Acta Phys. Sin. 63 196202
Google Scholar
[4] Johnson J N, Gray G T, Bourne N K 1999 J. Appl. Phys. 86 4892
Google Scholar
[5] Kanel G, Razorenov S, Bogatch A, Utkin A, Grady D 1997 Int. J. Impact. Eng. 20 467
Google Scholar
[6] Escobedo J P, Dennis-Koller D, Ceretta E K, Patterson B M, Bronkhorst C A, Hanson BL, Tonks D, Lebensohn R A 2011 J. Appl. Phys. 110 033513
Google Scholar
[7] Wang Y G, Qi M L, He H L, Wang L L 2014 Mech. Mater. 69 270
Google Scholar
[8] 裴晓阳, 彭辉, 贺红亮, 李平 2015 64 054601
Google Scholar
Pei X Y, Peng H, He H L, Li P 2015 Acta Phys. Sin. 64 054601
Google Scholar
[9] Lieberman E J, Lebensohn R A, Menasche D B, Bronkhorst C A, Rollett A D 2016 Acta Mater. 116 270
Google Scholar
[10] Turley W D, Fensin S J, Hixson R S, Jones R D, La Lone M B, Stevens G D, Thomas S A, Veeser L R 2018 J. Appl. Phys. 123 055102
Google Scholar
[11] Johnson J N 1981 J. Appl. Phys. 52 2812
Google Scholar
[12] Moninari A, Wright TW 2005 J. Mech. Phys. Solids 53 1476
Google Scholar
[13] Lubarda V A, Schneider M S, Kalantar D H, Remington B R, Meyers M A 2004 Acta Mater. 52 1397
Google Scholar
[14] Jacques N, Czarnota C, Mercier S, Molinari A 2015 Int. J. Fract. 162 159
[15] Wilkerson J W, Ramesh K T 2016 J. Mech. Phys. Solids 86 94
Google Scholar
[16] Wilkerson J W 2017 Int J. Plast. 95 21
Google Scholar
[17] Zurek A K, Thissell W R, Johnson J N, Tonks D L, Hixson R 1996 J. Mater. Process. Technol. 60 261
Google Scholar
[18] Wang Y G, He H L, Wang L L 2013 Mech. Mater. 56 131
Google Scholar
[19] Seppälä ET, Belak J, Rudd R E 2004 Phys. Rev. B 69 134101
Google Scholar
[20] Lou S N, Germann T C, Tonks D L 2009 J. Appl. Phys. 106 123518
Google Scholar
[21] 邓小良, 祝文军, 宋振飞, 贺红亮, 经福谦 2009 58 4772
Google Scholar
Deng X L, Zhu W J, Song Z F, He H L, Jing F Q 2009 Acta Phys. Sin. 58 4772
Google Scholar
[22] Becker R, LeBlanc M M, Cazamias J U 2007 J. Appl. Phys. 102 093512
Google Scholar
[23] Becker R 2017 Int. J. Fract. 208 5
Google Scholar
[24] Becker R, Callaghan K 2018 Int. J. Fract. 209 235
Google Scholar
[25] Trivedi P B, Asay J R, Gupta Y M, Field D P 2007 J. Appl. Phys. 102 083513
Google Scholar
[26] Schwartz A J, Cazamias J U, Fiske P S, Minich R W 2002 AIP Conf. Proc. 620 491
Google Scholar
[27] Chen T, Jiang Z X, Peng H, He H L, Wang L L, Wang Y G 2015 Strain 51 190
Google Scholar
[28] 张凤国, 周洪强 2013 62 164601
Google Scholar
Zhang F G, Zhou H Q 2013 Acta Phys. Sin. 62 164601
Google Scholar
[29] Johnson G R, Cook W H 1983 Eng. Fract. Mech. 21 541
[30] Novikov S A 1967 J. Appl. Meth. Tech. Phys. 3 109
[31] Chen D N, Yu Y Y, Yin Z H, Wang H R, Liu G Q 2005 Int. J. Impact Eng. 31 811
Google Scholar
[32] Kanel G I 2010 Int. J. Fract. 163 173
Google Scholar
[33] Seppälä ET, Belak J, Rudd R E 2004 Phys. Rev. Lett. 93 245503
Google Scholar
[34] 王永刚, 胡剑东, 祁美兰, 贺红亮 2011 60 126201
Google Scholar
Wang Y G, Hu J D, Qi M L, He H L 2011 Acta Phys. Sin. 60 126201
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5128
- PDF Downloads: 78
- Cited By: 0
