-
Molecular motor is a kind of protein macromolecule, which moves along the microfilament or microtubule in cell directionally and participates in all kinds of intracellular life activities fully. In order to study the directional motion of molecular motor, a series of ratchet models have been proposed. However, the potentials used in most of the ratchet models are smooth sawtooth potential or harmonic potential. Recently, the experimental studies show that intracellular impurities, spatial inhomogeneity or the folding process of protein can yield deviation from a smooth ratchet profile. This kind of deviation will roughen the known smooth potential. In fact, the roughness of potential is not only closely related to the properties of protein, but also has an important implication in transition rate. Therefore, the rough ratchet will be used to simulate the interaction between molecular motor and trajectory in this work. In addition, experimental researches show that there is a class of molecular motor that can move directionally without dragging load in organism. According to the theory presented by Wang and Oster (Wang H, Oster G 2002 Europhys. Lett. 57 134), the directional transport capability of this kind of motor can be investigated by means of Stokes efficiency. The higher the Stokes efficiency of the motor, the stronger the ability of the motor to use external input energy for directional motion. Here in this work, the overdamped Brownian transport of the two harmonically interacting particles is investigated, and the performance of transport is analyzed by studying the mean velocity and Stokes efficiency of the dimer induced by the introduction of roughness into the potential profile. The influences of the amplitude of perturbation, the wavenumber, the coupling strength and the free length of coupled Brownian particles on the directional transport performance are discussed in detail. According to the structure of ratchet, it is found that the roughness can either restrain or enhance the ratchet performance. It is shown that the appropriate amplitude and wavenumber of rough ratchet can promote the directional transport and enhance the Stokes efficiency of coupled Brownian particles. Moreover, one can distinguish between the optimal value of the coupling strength and free length that leads to a local maximum current. In addition, the directional transport of rough ratchet can be reversed by modulating the suitable coupling strength and free length. The conclusions obtained in this paper can provide theoretical guidance for understanding the motion behavior of molecular motor in experiment, and can also provide experimental inspiration for developing the nanometer machines and realizing the particle separation technology. -
Keywords:
- roughness /
- center-of-mass mean velocity /
- Stokes efficiency /
- current reversal
[1] Xie P 2010 Int. J. Biol. Sci. 6 665
[2] 黎明, 欧阳钟灿, 舒咬根 2016 18 188702
Google Scholar
Li M, Ouyang Z C, Shu Y G 2016 Acta Phys. Sin. 18 188702
Google Scholar
[3] 郭晓强 2019 自然杂志 41 56
Guo X Q 2019 Chin. J. Nat. 41 56
[4] Allen R D, Metuzals J, Tasahi I, Brady S T, Gilbert S P 1982 Science 218 1127
Google Scholar
[5] Vale R D, Schnapp B J, Reese T S, Sheetz M P 1985 Cell 40 449
Google Scholar
[6] Vale R D, Reese T S, Sheetz M P 1985 Cell 42 39
Google Scholar
[7] Dey K K, Zhao X, Tansi B M, Mendez-Ortiz W J, Cordova-Figueroa U M, Golestanian R, Sen A 2015 Nano Lett. 15 8311
Google Scholar
[8] Nara Y, Niemi H, Steinheimer J, Stöcker H 2017 Phys. Lett. B 769 543
[9] Mateos J L, Arzola A V, Volke-Seplveda K 2011 Phys. Rev. Lett. 106 168104
Google Scholar
[10] Minucci S, Pelicci P G 2006 Nat. Rev. Cancer 6 38
Google Scholar
[11] Palmigiano A, Santaniello F, Cerutti A, Penkov D, Pu-rushothama D 2018 Sci. Rep. 8 3198
Google Scholar
[12] Linke H 2002 Appl. Phys. A 75 167
Google Scholar
[13] Van den Heuvel M G L, Dekker C 2007 Science 317 333
Google Scholar
[14] Zhang H W, Wen S T, Zhang H T, Li Y X, Chen G R 2012 Chin. Phys. B 21 078701
Google Scholar
[15] Doering C R 1995 Nuovo Cimento 17 685
Google Scholar
[16] Astumian R D, Bier M 1994 Phys. Rev. Lett. 72 1766
Google Scholar
[17] Gao T F, Chen J C 2009 J. Phys. A: Math. Theor. 42 065002
Google Scholar
[18] Krishnan R, Chacko J, Sahoo M, Jayannavar A M 2006 J. Stat. Mech.: Theory Exp. 2006 P06017
[19] Wang H Y, Bao J D 2013 Physica A 392 4850
[20] Li P C, Chen H B, Fan H, Shen W M, Zheng Z G 2017 J. Phys. A: Math. Theor. 50 475003
Google Scholar
[21] 延明月, 张旭, 刘晨昊, 黄仁忠, 高天附, 郑志刚 2018 67 190501
Google Scholar
Yan M Y, Zhang X, Liu C H, Huang R Z, Gao T F, Zheng Z G 2018 Acta Phys. Sin. 67 190501
Google Scholar
[22] Camargo S, Anteneodo C 2018 Physica A 495 114
Google Scholar
[23] Frauenfelder H, Sligar S G, Wolynes P G 1991 Science 254 1598
Google Scholar
[24] Frauenfelder H, Wolynes P G, Austin R H 1999 Rev. Mod. Phys. 71 S419
Google Scholar
[25] Zwanzig R 1988 Proc. Natl. Acad. Sci. U.S.A. 85 2029
Google Scholar
[26] Marchesoni F 1997 Phys. Rev. E 56 2492
Google Scholar
[27] Parrondo J M R, Cisneros B J D 2002 Physics A 75 179
[28] Wang H, Oster G 2002 Europhys. Lett. 57 134
Google Scholar
[29] Sekimoto K 1997 J. Phys. Soc. Jpn. 66 1234
Google Scholar
[30] Ai B Q, Xie H Z, Liao H Y, Liu L G 2006 J. Stat. Mech.: Theory Exp. 2006 P09016
[31] Gehlen S V, Evstigneev M, Reimann P 2009 Phys. Rev. E 79 031114
Google Scholar
[32] Ai B Q, Zhong W R 2011 Phys. Rev. E 83 051106
Google Scholar
-
图 1 (a) 粗糙势
$U\left( x \right)$ 随扰动振幅$\varepsilon $ 的变化, 其中扰动波数$H \!=\! 5$ ; (b) 粗糙势$U\left( x \right)$ 随扰动波数H的变化, 其中扰动振幅$\varepsilon \!=\! 0.1$ Figure 1. (a) Diagram of the rough potential
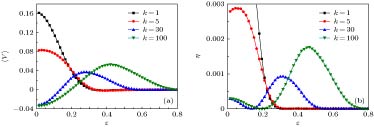
$U\left( x \right)$ varying with the amplitude of perturbation$\varepsilon $ , where perturbed wavenumber$H = 5$ ; (b) diagram of the rough potential$U\left( x \right)$ varying with perturbed wavenumber H, where the perturbation amplitude$\varepsilon = 0.1$ .
图 2 不同耦合强度下, (a) 质心平均速度
$\left\langle V \right\rangle $ 、(b) 斯托克斯效率$\eta $ 随粗糙势扰动振幅$\varepsilon $ 的变化($a = 0.5$ ,$A = 3$ ,$H = 5$ )Figure 2. Curves of (a) the center-of-mass velocity
$\left\langle V \right\rangle $ and (b) the Stokes efficiency$\eta $ varying with perturbation amplitude$\varepsilon $ for different coupling strength k, where$a = 0.5$ ,$A = 3$ ,$H = 5$ .
图 3 不同扰动波数H下 (a) 质心平均速度
$\left\langle V \right\rangle $ ; (b) 斯托克斯效率$\eta $ 随耦合强度k的变化曲线, 其中$a = 0.2$ ,$A = 3$ ,$\varepsilon = 0.1$ Figure 3. Curves of (a) the center-of-mass velocity
$\left\langle V \right\rangle $ ; (b) the Stokes efficiency$\eta $ varying with coupling strength k for different perturbed wavenumber H, where$a = 0.2$ ,$A = 3$ ,$\varepsilon = 0.1$ .
图 4 不同噪声强度下, (a) 质心平均速度速度
$\left\langle V \right\rangle $ 、(b) 斯托克斯效率$\eta $ 随扰动波数H的变化($a \!=\! 0.2$ ,$k \!=\! 10$ ,$A \!=\! 3$ ,$\varepsilon \!=\! 0.1$ )Figure 4. Curves of (a) the center-of-mass velocity
$\left\langle V \right\rangle $ and (b) the Stokes efficiency$\eta $ varying with perturbed wavenumber H for different noise intensity D, where$a = 0.2$ ,$k = 10$ ,$A = 3$ ,$\varepsilon = 0.1$ .
图 5 不同耦合强度下, (a) 质心平均速度
$\left\langle V \right\rangle $ 、(b) 斯托克斯效率$\eta $ 随外力振幅A的变化($a = 0.2$ ,$H = 5$ ,$\varepsilon = 0.1$ )Figure 5. Curves of (a) the center-of-mass velocity
$\left\langle V \right\rangle $ and (b) the Stokes efficiency$\eta $ varying with amplitude A for different coupling strength k, where$a = 0.2$ ,$H = 5$ ,$\varepsilon = 0.1$ .
图 6 (a) 质心平均速度
$\left\langle V \right\rangle $ 随耦合自由长度a的变化($A = 3$ ,$\varepsilon = 0.1$ ,$H = 5$ ,$k = 30$ ,$D = 0.1$ ); (b) 质心平均速度$\left\langle V \right\rangle $ 随耦合自由长度a及耦合强度k的变化($A = 3$ ,$\varepsilon = 0.1$ ,$H = 5$ ,$D = 0.1$ )Figure 6. Curves of (a) the center-of-mass velocity
$\left\langle V \right\rangle $ varying with free length a, where$A = 3$ ,$k = 30$ ,$\varepsilon = 0.1$ ,$D = 0.1$ ; the curves of (b)$\left\langle V \right\rangle $ varying with coupling strength k and free length a, where$A = 3$ ,$\varepsilon = 0.1$ ,$k = 30$ ,$D = 0.1$ . -
[1] Xie P 2010 Int. J. Biol. Sci. 6 665
[2] 黎明, 欧阳钟灿, 舒咬根 2016 18 188702
Google Scholar
Li M, Ouyang Z C, Shu Y G 2016 Acta Phys. Sin. 18 188702
Google Scholar
[3] 郭晓强 2019 自然杂志 41 56
Guo X Q 2019 Chin. J. Nat. 41 56
[4] Allen R D, Metuzals J, Tasahi I, Brady S T, Gilbert S P 1982 Science 218 1127
Google Scholar
[5] Vale R D, Schnapp B J, Reese T S, Sheetz M P 1985 Cell 40 449
Google Scholar
[6] Vale R D, Reese T S, Sheetz M P 1985 Cell 42 39
Google Scholar
[7] Dey K K, Zhao X, Tansi B M, Mendez-Ortiz W J, Cordova-Figueroa U M, Golestanian R, Sen A 2015 Nano Lett. 15 8311
Google Scholar
[8] Nara Y, Niemi H, Steinheimer J, Stöcker H 2017 Phys. Lett. B 769 543
[9] Mateos J L, Arzola A V, Volke-Seplveda K 2011 Phys. Rev. Lett. 106 168104
Google Scholar
[10] Minucci S, Pelicci P G 2006 Nat. Rev. Cancer 6 38
Google Scholar
[11] Palmigiano A, Santaniello F, Cerutti A, Penkov D, Pu-rushothama D 2018 Sci. Rep. 8 3198
Google Scholar
[12] Linke H 2002 Appl. Phys. A 75 167
Google Scholar
[13] Van den Heuvel M G L, Dekker C 2007 Science 317 333
Google Scholar
[14] Zhang H W, Wen S T, Zhang H T, Li Y X, Chen G R 2012 Chin. Phys. B 21 078701
Google Scholar
[15] Doering C R 1995 Nuovo Cimento 17 685
Google Scholar
[16] Astumian R D, Bier M 1994 Phys. Rev. Lett. 72 1766
Google Scholar
[17] Gao T F, Chen J C 2009 J. Phys. A: Math. Theor. 42 065002
Google Scholar
[18] Krishnan R, Chacko J, Sahoo M, Jayannavar A M 2006 J. Stat. Mech.: Theory Exp. 2006 P06017
[19] Wang H Y, Bao J D 2013 Physica A 392 4850
[20] Li P C, Chen H B, Fan H, Shen W M, Zheng Z G 2017 J. Phys. A: Math. Theor. 50 475003
Google Scholar
[21] 延明月, 张旭, 刘晨昊, 黄仁忠, 高天附, 郑志刚 2018 67 190501
Google Scholar
Yan M Y, Zhang X, Liu C H, Huang R Z, Gao T F, Zheng Z G 2018 Acta Phys. Sin. 67 190501
Google Scholar
[22] Camargo S, Anteneodo C 2018 Physica A 495 114
Google Scholar
[23] Frauenfelder H, Sligar S G, Wolynes P G 1991 Science 254 1598
Google Scholar
[24] Frauenfelder H, Wolynes P G, Austin R H 1999 Rev. Mod. Phys. 71 S419
Google Scholar
[25] Zwanzig R 1988 Proc. Natl. Acad. Sci. U.S.A. 85 2029
Google Scholar
[26] Marchesoni F 1997 Phys. Rev. E 56 2492
Google Scholar
[27] Parrondo J M R, Cisneros B J D 2002 Physics A 75 179
[28] Wang H, Oster G 2002 Europhys. Lett. 57 134
Google Scholar
[29] Sekimoto K 1997 J. Phys. Soc. Jpn. 66 1234
Google Scholar
[30] Ai B Q, Xie H Z, Liao H Y, Liu L G 2006 J. Stat. Mech.: Theory Exp. 2006 P09016
[31] Gehlen S V, Evstigneev M, Reimann P 2009 Phys. Rev. E 79 031114
Google Scholar
[32] Ai B Q, Zhong W R 2011 Phys. Rev. E 83 051106
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 7626
- PDF Downloads: 76
- Cited By: 0
