-
本文研究了耦合布朗粒子在空间非均匀摩擦环境下的定向输运问题, 并进一步讨论了摩擦系数振幅、空间相位差等因素对耦合粒子质心平均速度及能量转化效率的影响. 研究发现, 耦合粒子的质心平均速度和能量转化效率随摩擦系数振幅的变化都能呈现多峰结构. 这一结果表明摩擦阻尼并不总是阻碍布朗粒子的定向运动, 一定条件下合适的摩擦环境还能多次增强耦合布朗棘轮的输运性能. 此外, 在小摩擦系数振幅条件下通过改变外力振幅、外势不对称度、空间相位差还能诱导摩擦棘轮流反转的产生. 本文所得结论不仅有助于理解摩擦环境中耦合布朗粒子的输运性能, 还可为微观纳米机器的设计与粒子的筛选分离技术提供理论指导.
Biomolecular motor is a kind of protein macromolecule widely existing in cells. It can convert the chemical energy contained in ATP molecules into mechanical motion, and then continuously provide power for the material transport process. In order to further study the directional transport of molecular motors, the Brownian ratchet model is established based on Brownian motion theory. However, most of the considerations in previous studies are devoted to the motion of Brownian particles under the condition of unit friction damping. In order to further study the influence of medium damping on the directional motion of Brownian particles, our group further study the directional transport of Brownian ratchets in different damping environments, and find that the suitable friction damping coefficient ratio can increase the center-of-mass mean velocity of the coupled Brownian particle. It should be pointed out that the above studies of Brownian ratchets consider the motion of Brownian particles under the condition of uniform spatial friction. In fact, the cell environment in organism is very complex, and the concentration and impurities in the cell change all the time. The medium damping of molecular motor is not always fixed, so choosing the space non-uniform friction condition to study the directional motion of coupled Brownian particles under different damping environments can better understand the directional transport characteristics of friction ratchets. In addition, other point of interest in the research of biomolecular motors is the high efficiency of energy conversion. Experimental results show that the energy conversion efficiencies of most molecular motors are more than 70%, and the efficiencies of some motors are even close to 100%. However, by comparing the experimental results with the theoreticalstudies, it can be found that the efficiency calculated by ratchet model is much lower than that measured in experiment. Therefore, in this paper, the directional motion of coupled Brownian particles in the space non-uniform friction environment is studied in depth, and the energy conversion efficiency of Brownian particles is further discussed. The results show that the center-of-mass mean velocity varying with the amplitude of the friction coefficient presents a multi-peak structure. This conclusion shows that friction damping does not always hinder the directional motion of coupled particles, and the frictional environment under certain conditions can also enhance the directional transport of coupled Brownian particles. At the same time, the change of the energy conversion efficiency of friction ratchets is similar to that of the center-of-mass mean velocity, which means that the proper friction damping can also enhance the transport performance of the friction ratchets. In addition, under the condition of small friction amplitude, the flow reversal of friction ratchet can be induced by external force amplitude, external potential asymmetry and spatial phase difference. The conclusions obtained in this paper can not only help people understand the directional transport performance of coupled particles in a spatially non-uniform friction environment, but also provide theoretical inspiration for particle separation and screening technology and the design of artificial nanomachines. -
Keywords:
- friction ratchets /
- center-of-mass mean velocity /
- energy conversion efficiency /
- friction coefficient amplitude
[1] 舒咬根, 欧阳钟灿 2007 物理 36 735
Google Scholar
Shu Y G, Ouyang Z C 2007 Physics 36 735
Google Scholar
[2] Xie P 2010 Int. J. Biol. Sci. 6 665
[3] 郭晓强 2019 自然杂志 41 56
Google Scholar
Guo X Q 2019 Chin. J. Nat. 41 56
Google Scholar
[4] Ross J L 2012 P. Natl. Acad. Sci. Usa. 109 5911
Google Scholar
[5] Nara Y, Niemi H, Steinheimer J, Stöcker H 2017 Phys. Lett. B 769 543
Google Scholar
[6] Mateos J L, Arzola A V, Volke-Seplveda K 2011 Phys. Rev. Lett. 106 168104
Google Scholar
[7] Palmigiano A, Santaniello F, Cerutti A, Penkov D, Purushothama D 2018 Sci. Rep. 8 3198
Google Scholar
[8] Linke H 2002 Appl. Phys. A 75 167
Google Scholar
[9] Van den Heuvel M G L, Dekker C 2007 Science 317 333
Google Scholar
[10] Zhang H W, Wen S T, Zhang H T, Li Y X, Chen G R 2012 Chin. Phys. B 21 078701
Google Scholar
[11] Doering C R 1995 Nuovo Cimento 17 685
Google Scholar
[12] Astumian R D, Bier M 1994 Phys. Rev. Lett. 2 1766
[13] Gao T F, Chen J C 2009 J. Phys. A: Math. Theor. 42 065002
Google Scholar
[14] Dinis L, Quintero R N 2015 Phys. Rev. E 91 032920
Google Scholar
[15] Li P C, Chen H B, Fan H, Shen W M, Zheng Z G 2017 J. Phys. A:Math. Theor. 50 475003
Google Scholar
[16] 延明月, 张旭, 刘晨昊, 黄仁忠, 高天附, 郑志刚 2018 67 190501
Google Scholar
Yan M Y, Zhang X, Liu C H, Huang R Z, Gao T F, Zheng Z G 2018 Acta Phys. Sin. 67 190501
Google Scholar
[17] 张旭, 曹佳慧, 艾保全, 高天附, 郑志刚 2020 69 100503
Google Scholar
Zhang X, Cao J H, Ai B Q, Gao T F, Zheng Z G 2020 Acta Phys. Sin. 69 100503
Google Scholar
[18] Gehlen S V, Evstigneev M, Reimann P 2009 Phys. Rev. E 79 031114
Google Scholar
[19] Lombardo J, Broadwater D, Collins R, Cebe K, Brady R, Harrison S 2019 Hum. Pathol. 86 129
Google Scholar
[20] Toyoshima H 1951 J. Japan. Forest. Soc. 33 203
[21] Toyoshima H 1951 J. Japan. Forest. Soc. 33 83
[22] 黎明, 欧阳钟灿, 舒咬根 2016 65 188702
Google Scholar
Li M, Ouyang Z C, Shu Y G 2016 Acta Phys. Sin. 65 188702
Google Scholar
[23] Sumithra K, Sintes T 2001 Physica A 297 1
[24] Kharkongor D, Reenbohn W L, Mahato Mangal C 2018 J. Stat. Mech. 3 033209
[25] Kharkongor D, Reenbohn W L, Mahato Mangal C 2016 Phys. Rev. E 94 022148
Google Scholar
[26] Camargo S, Anteneodo C 2018 Physica A 495 114
Google Scholar
[27] Sekimoto K 1997 J. Phys. Soc. Jpn. 66 1234
Google Scholar
-
图 1 不同外力振幅
$ A $ 下质心平均速度$ \left\langle v \right\rangle $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线, 其中$\varDelta = 1$ ,$\theta = 0.75{\text{π }}$ Fig. 1. The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ under different external force amplitude$ A $ , where$\varDelta = 1$ ,$\theta = 0.75{\text{π }}$ .
图 2 (a) 不同外势不对称度
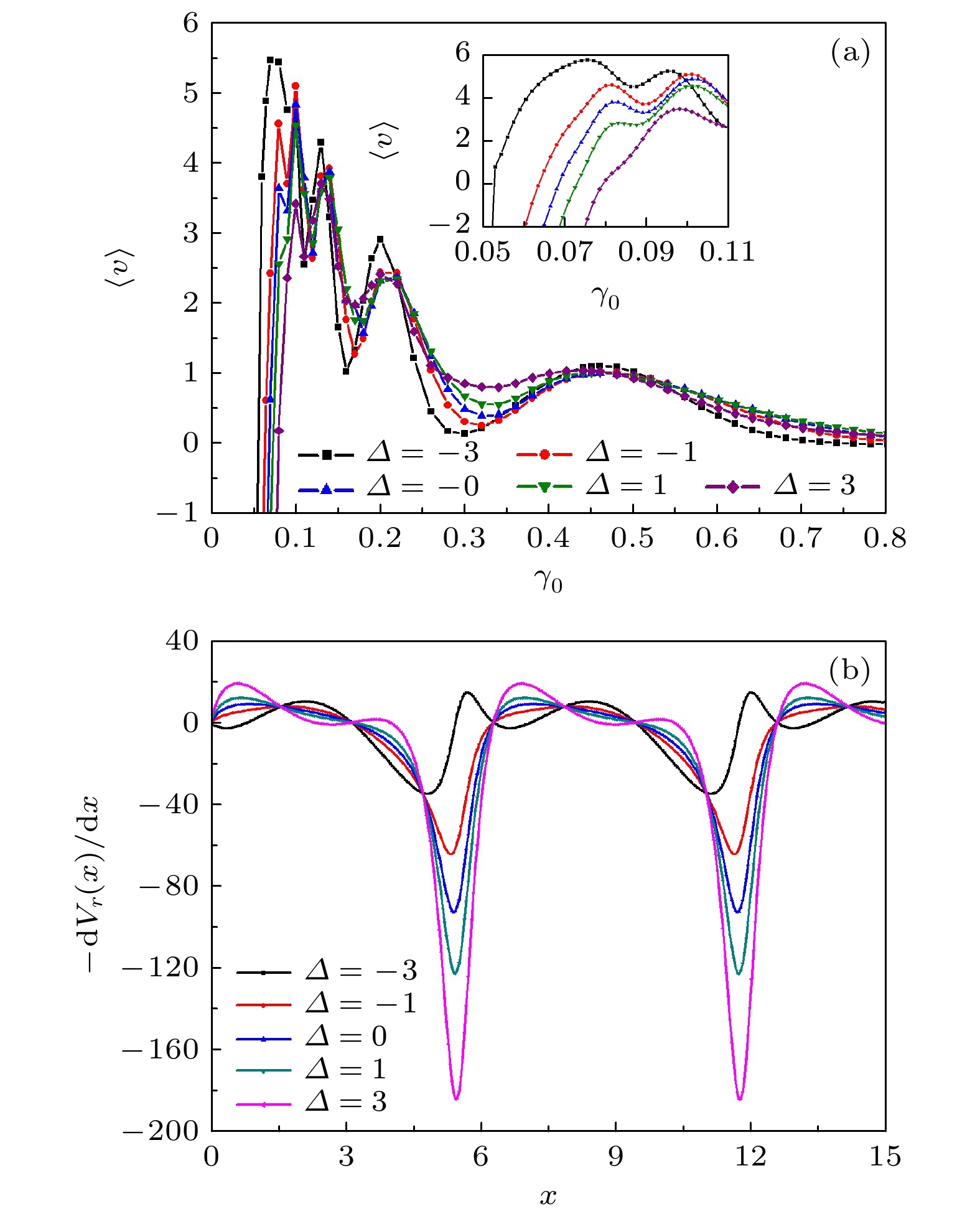
$\varDelta$ 下质心平均速度$ \left\langle v \right\rangle $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线; (b) 外势作用力$- {\text{d}}{V_r} \left( x \right)/{\text{d}}x$ 随外势不对称度$\varDelta$ 变化的曲线, 其中$ A = 4 $ ,$\theta = 0.75{\text{π }}$ Fig. 2. (a) The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ under different asymmetric parameter$\varDelta$ ; (b) the curves of the external potential force$ - {\text{d}}{V_r}\left( x \right)/{\text{d}}x$ varying with asymmetric parameter$\varDelta$ , where$ A = 4 $ ,$\theta = 0.75{\text{π }}$ .
图 3 不同空间相位差
$ \theta $ 下质心平均速度$ \left\langle v \right\rangle $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线, 其中$ A = 4 $ ,$\varDelta = 1$ Fig. 3. The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ under different spatial phase difference$ \theta $ , where$ A = 4 $ ,$\varDelta = 1$ .
图 4 不同外力振幅下能量转化效率
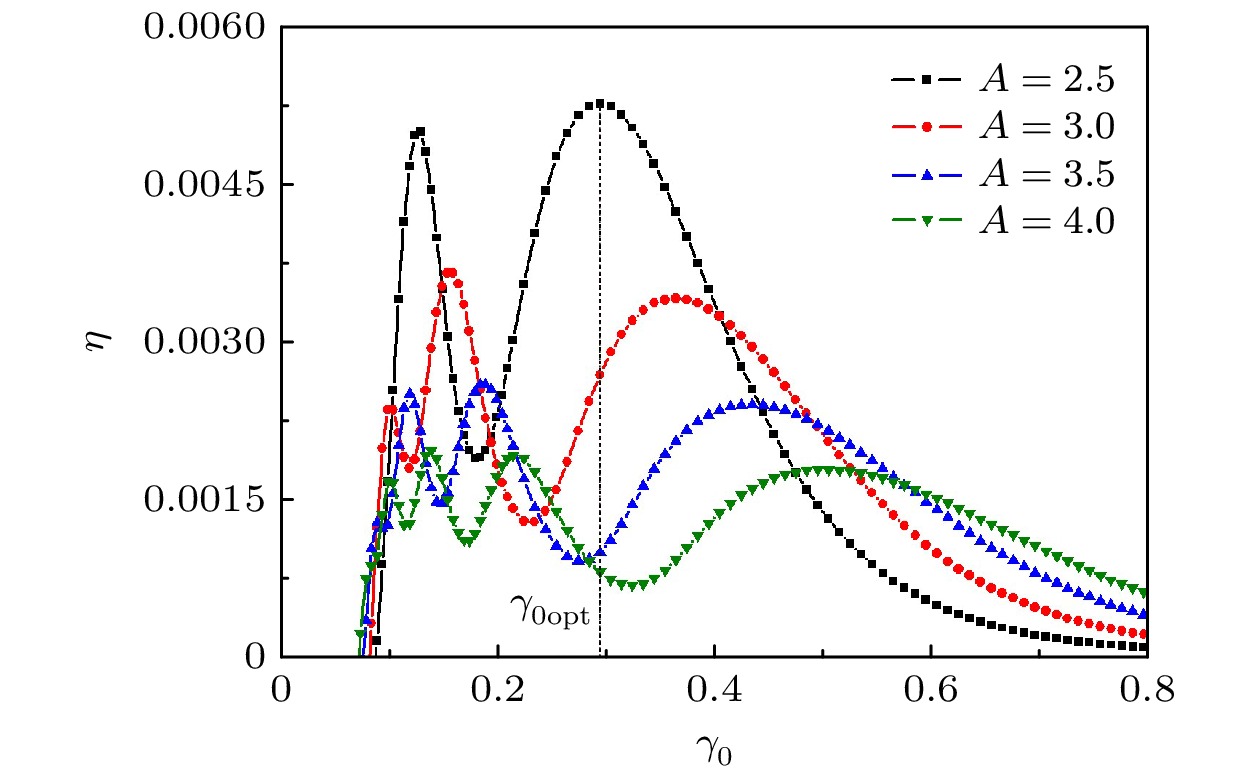
$ \eta $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线, 其中$\varDelta = 1= 1$ ,$\theta = 0.75{\text{π }}$ Fig. 4. The curves of the energy conversion efficiency
$ \eta $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ under different external force amplitudes A, where$\varDelta = 1= 1$ ,$\theta = 0.75{\text{π }}$ .
图 5 不同外势不对称度
$\varDelta$ 下能量转化效率$ \eta $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线, 其中$ A = 4 $ ,$\theta = 0.75{\text{π }}$ Fig. 5. The curves of the energy conversion efficiency
$ \eta $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ under different external asymmetric parameter$\varDelta$ , where$ A = 4 $ ,$\theta = 0.75{\text{π }}$ .
图 6
$\theta = 0.75{\text{π }}$ 时能量转化效率$ \eta $ 随摩擦系数振幅$ {\gamma _0} $ 的变化曲线, 其中$ A = 4 $ ,$\varDelta = 1$ Fig. 6. The curve of the energy conversion efficiency
$ \eta $ varying with the amplitude of the friction coefficient$ {\gamma _0} $ , where$\theta = 0.75{\text{π }}$ ,$ A = 4 $ ,$\varDelta = 1$ .
图 7 质心平均速度
$ \left\langle v \right\rangle $ 随外力振幅$ A $ 的变化曲线, 其中$\varDelta = 1$ ,$\theta = 0.75{\text{π }}$ Fig. 7. The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with the amplitude of the external force$ A $ , where$\varDelta = 1$ ,$\theta = 0.75{\text{π }}$ .
图 8 质心平均速度
$ \left\langle v \right\rangle $ 随外势不对称度$\varDelta$ 的变化曲线, 其中$ A = 4 $ ,$ {\gamma _0} = 0.08 $ Fig. 8. The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with asymmetric parameter$\varDelta$ , where$ A = 4 $ ,$ {\gamma _0} = 0.08 $ .
图 9 质心平均速度
$ \left\langle v \right\rangle $ 随空间相位差$ \theta $ 的变化曲线, 其中$\varDelta = 1$ ,$ {\gamma _0} = 0.08 $ Fig. 9. The curves of the center-of-mass mean velocity
$ \left\langle v \right\rangle $ varying with spatial phase difference$ \theta $ , where$\varDelta = 1$ ,$ {\gamma _0} = 0.08 $ . -
[1] 舒咬根, 欧阳钟灿 2007 物理 36 735
Google Scholar
Shu Y G, Ouyang Z C 2007 Physics 36 735
Google Scholar
[2] Xie P 2010 Int. J. Biol. Sci. 6 665
[3] 郭晓强 2019 自然杂志 41 56
Google Scholar
Guo X Q 2019 Chin. J. Nat. 41 56
Google Scholar
[4] Ross J L 2012 P. Natl. Acad. Sci. Usa. 109 5911
Google Scholar
[5] Nara Y, Niemi H, Steinheimer J, Stöcker H 2017 Phys. Lett. B 769 543
Google Scholar
[6] Mateos J L, Arzola A V, Volke-Seplveda K 2011 Phys. Rev. Lett. 106 168104
Google Scholar
[7] Palmigiano A, Santaniello F, Cerutti A, Penkov D, Purushothama D 2018 Sci. Rep. 8 3198
Google Scholar
[8] Linke H 2002 Appl. Phys. A 75 167
Google Scholar
[9] Van den Heuvel M G L, Dekker C 2007 Science 317 333
Google Scholar
[10] Zhang H W, Wen S T, Zhang H T, Li Y X, Chen G R 2012 Chin. Phys. B 21 078701
Google Scholar
[11] Doering C R 1995 Nuovo Cimento 17 685
Google Scholar
[12] Astumian R D, Bier M 1994 Phys. Rev. Lett. 2 1766
[13] Gao T F, Chen J C 2009 J. Phys. A: Math. Theor. 42 065002
Google Scholar
[14] Dinis L, Quintero R N 2015 Phys. Rev. E 91 032920
Google Scholar
[15] Li P C, Chen H B, Fan H, Shen W M, Zheng Z G 2017 J. Phys. A:Math. Theor. 50 475003
Google Scholar
[16] 延明月, 张旭, 刘晨昊, 黄仁忠, 高天附, 郑志刚 2018 67 190501
Google Scholar
Yan M Y, Zhang X, Liu C H, Huang R Z, Gao T F, Zheng Z G 2018 Acta Phys. Sin. 67 190501
Google Scholar
[17] 张旭, 曹佳慧, 艾保全, 高天附, 郑志刚 2020 69 100503
Google Scholar
Zhang X, Cao J H, Ai B Q, Gao T F, Zheng Z G 2020 Acta Phys. Sin. 69 100503
Google Scholar
[18] Gehlen S V, Evstigneev M, Reimann P 2009 Phys. Rev. E 79 031114
Google Scholar
[19] Lombardo J, Broadwater D, Collins R, Cebe K, Brady R, Harrison S 2019 Hum. Pathol. 86 129
Google Scholar
[20] Toyoshima H 1951 J. Japan. Forest. Soc. 33 203
[21] Toyoshima H 1951 J. Japan. Forest. Soc. 33 83
[22] 黎明, 欧阳钟灿, 舒咬根 2016 65 188702
Google Scholar
Li M, Ouyang Z C, Shu Y G 2016 Acta Phys. Sin. 65 188702
Google Scholar
[23] Sumithra K, Sintes T 2001 Physica A 297 1
[24] Kharkongor D, Reenbohn W L, Mahato Mangal C 2018 J. Stat. Mech. 3 033209
[25] Kharkongor D, Reenbohn W L, Mahato Mangal C 2016 Phys. Rev. E 94 022148
Google Scholar
[26] Camargo S, Anteneodo C 2018 Physica A 495 114
Google Scholar
[27] Sekimoto K 1997 J. Phys. Soc. Jpn. 66 1234
Google Scholar
下载:
计量
- 文章访问数: 3711
- PDF下载量: 51
- 被引次数: 0
