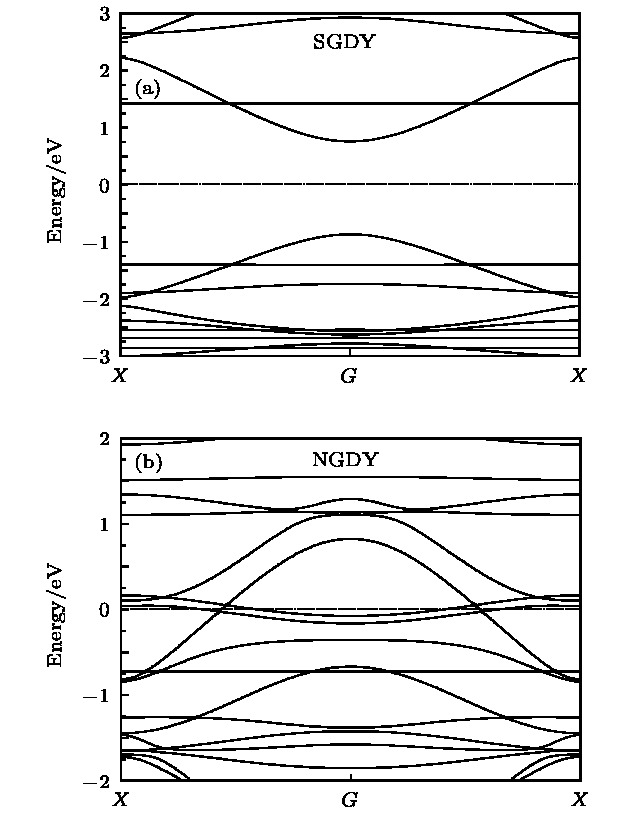
-
Resonant tunneling transistors have received wide attention because of their ability to reduce the complexity of circuits, and promise to be an efficient candidate in ultra-high speed and ultra-high frequency applications. The chemical compatibility between graphene and graphdiyne implies that they can be combined into various configurations to fulfill ultra-high frequency nanotransistor. In the present paper, two novel resonant tunneling transistors based on graphene/graphdiyne/graphene double-heterojunction are theoretically developed to model two new kinds of bipolar devices with two representative graphdiyne nanoribbons. The electronic structures of two pristine graphdiyne nanoribbons are investigated by performing the first-principles calculations with all-electron relativistic numerical-orbit scheme as implemented in Dmol3 code. The electronic transport properties including quantum conductance (transmission spectrum) and electrical current varying with bias-voltage for each of the designed graphdiyne nanoribbon transistors are calculated in combination with non-equilibrium Green function formalism. The calculated electronic transmission and current-voltage characteristics of these transistors demonstrate that the current is dominantly determined by resonant tunneling transition and can be effectively controlled by gate electric field thereby representing the favorable negative-differential-conductivity, which is the qualified attribute of ultra-high frequency nanotransistor. It follows from the I-Ub variations explained by electronic transmission spectra that quantum resonance tunneling can occur in the proposed star-like graphdiyne (SGDY) and net-like graphdiyne (NGDY) nanoribbon transistors, with the resonance condition limited to a narrow bias-voltage range, leading to a characteristic resonant peak in I-Ub curve, which means the strong negative differential conductivity. Under a gate voltage of 4 V, when the bias-voltage rises up to 0.6 V (0.7 V), the Fermi level of source electrode aligns identically to the quantized level of SGDY (NGDY) nanoribbon channel, causing electron resonance tunneling as illustrated by the considerable transmission peak in bias window; once the source Fermi level deviates from the quantized level of SGDY (NGDY) channels at higher bias-voltage, the resonance tunneling transforms into ordinary electron tunneling, which results in the disappearing of the substantial transmission peak in bias window and the rapid declining of current. The designed SGDY and NGDY nanotransistors will achieve high-level negative differential conductivity with the peak-to-valley current ratio approaching to 4.5 and 6.0 respectively, which can be expected to be applied to quantum transmission nanoelectronic devices.
-
Keywords:
- graphene /
- graphdiyne /
- nanoribbon heterostructure /
- resonant tunneling transistor
[1] Pi S, Lin P, Xia Q 2016 Nanotechnology 27 464004
Google Scholar
[2] Lawrence T C, Vashishtha V, Shifren L, Gujja A, Sinha S, Cline B, Ramamurthy C, Yeric G 2016 Microelect. J. 53 105
Google Scholar
[3] Guo Y G, Wang F Q, Wang Q 2017 Appl. Phys. Lett. 111 073503
Google Scholar
[4] Punniyakoti S, Sivakumarasamy R, Vaurette F, Joseph P, Nishiguchi K, Fujiwara A, Clement N 2017 Adv. Mater. Interf. 4 1601155
Google Scholar
[5] Murugesan A 2014 Int. J. Innovative Sci. Eng. Tech. 1 264
[6] Akbar F, Kolahduz M, Larimian S, Radamson H H 2015 J. Mater. Sci. Mater. Elect. 26 4347
Google Scholar
[7] Park J S, Choi H J 2015 Phys. Rev. B 92 045402
Google Scholar
[8] Mihnev M T, Wang F, Liu G, Rothwell S, Cohen P I, Feldman L C, Conrad E H, Norris T B 2015 Appl. Phys. Lett. 107 173107
Google Scholar
[9] Li G, Li Y, Liu H, Guo Y, Li Y, Zhu D 2010 Chem. Commun. 46 3256
Google Scholar
[10] Cranford S W, Buehler M J 2011 Carbon 49 4111
Google Scholar
[11] Kang J, Li J, Wu F, Li S S, Xia J B 2011 J. Phys. Chem. C 115 20466
Google Scholar
[12] Long M, Tang L, Wang D, Li Y, Shuai Z 2011 ACS Nano 5 2593
Google Scholar
[13] Zhou J, Lü K, Wang Q, Chen X S, Sun Q, Jena P 2011 J. Chem. Phys. 134 174701
Google Scholar
[14] Capasso F, Kiehl R A 1985 J. Appl. Phys. 58 1366
Google Scholar
[15] Teong H, Lam K T, Khalid S B, Liang G 2009 J. Appl. Phys. 105 084317
Google Scholar
[16] Britnell L, Gorbachev R V, Geim A K, Ponomarenko L A, Mishchenko A, Greenaway M T, Fromhold T M, Novoselov K S, Eaves L 2013 Nat. Commun. 4 1794
Google Scholar
[17] Mishchenko A, Tu J S, Cao Y, Gorbachev R V, Wallbank J R, Greenaway M T, Morozov V E, Morozov S V, Zhu M J, Wong S L, Withers F, Woods C R, Kim Y J, Watanabe K, Taniguchi T, Vdovin E E, Makarovsky O, Fromhold T M, FaI’ko V I, Geim A K, Eaves L, Novoselov K S 2014 Nat. Nanotech. 9 808
Google Scholar
[18] Özçelik V O, Durgun E, Ciraci S 2015 J. Phys. Chem. C 119 13248
Google Scholar
[19] Chowdhury S, Chattaraj S, Biswas D 2015 J. Semicond. 36 044001
Google Scholar
[20] Allis D G, Prokhorova D, Korter T M 2006 J. Phys. Chem. A 110 1951
Google Scholar
[21] Niehaus T A, Rohlfing M, Della Sala F, Di Carlo A, Frauenheim T 2005 Phys. Rev. A 71 022508
Google Scholar
[22] Pecchia A, Penazzi G, Salvucci L, Di Carlo A 2008 New J. Phys. 10 065022
Google Scholar
[23] Chantis A N, Christensen N E, Svane A, Cardona M 2010 Phys. Rev. B 81 205205
Google Scholar
[24] Perdew J P, Ruzsinszky A, Csonka G I, Vydrov O A, Scuseria G E, Constantin L A, Zhou X L, Burke K 2008 Phys. Rev. Lett. 100 136406
Google Scholar
[25] Weinert M, Davenport J W 1992 Phys. Rev. B 45 13709
Google Scholar
[26] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[27] Tkatchenko A, Scheffler M 2009 Phys. Rev. Lett. 102 073005
Google Scholar
[28] Ghanbari Shohany B, RoknabadiM R, Kompany A 2016 Commun. Theor. Phys. 65 99
Google Scholar
[29] Dong J, Li H, Li L 2013 NPG Asia Mater. 5 e56
Google Scholar
-
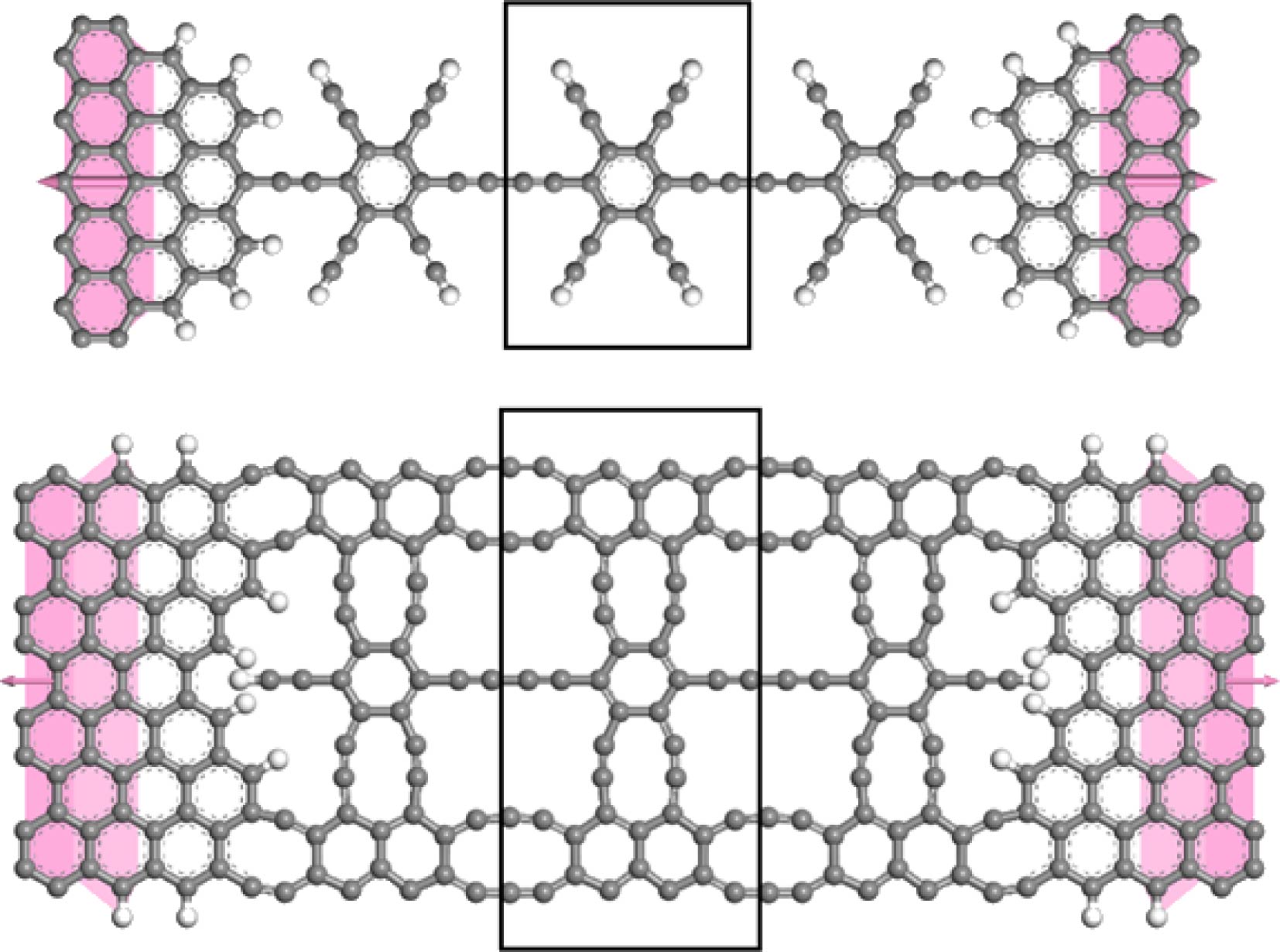
图 1 SGDY (上图)和NGDY (下图)纳米带两端连接石墨烯纳米带半无限电极(源极和漏极粉色区域)构建的双极器件模型, 石墨炔尖端和石墨烯边缘碳原子由氢原子钝化, 黑色框架表示周期性单胞
Figure 1. Schematic bipolar device models constructed with the SGDY (above panel) or NGDY (below panel) as center scattering region and the graphene nanoribbons as semi-infinite electrodes (source and drain in pink areas). The apex and edge carbon atoms are passivated by hydrogen atoms, and black frames indicate periodic unit cells.
图 2 SGDY (a)和NGDY (b)纳米带的电子能带结构, 以费米能级(水平虚线)为能量参考零点
Figure 2. Electronic energy band structure of SGDY (a) and NGDY (b) nanoribbons with Fermi energy level as reference energy zero (horizontal dashed line).
图 3 在SGDY/石墨烯异质结纳米带双极器件的垂直方向施加栅极电压构建的晶体管电子输运计算模型, 粉色区域表示电极, 灰色、白色、红色、黄色和粉色小球分别代表碳、氢、氧、硅和铝原子
Figure 3. Electron transport calculation in transistor model of bipolar devices with the SGDY/graphene nanoribbons heterostructure as the center scattering region and semi-infinite electrodes (source and drain) respectively under the gate voltage in vertical direction. The pink areas indicate electrodes, and the gray, white, red, yellow and pink spheres represent carbon, hydrogen, oxygen, silicon and aluminium atoms respectively.
图 4 SGDY和NGDY纳米带晶体管的漏极电流随偏置电压的变化 (a) Ug = 0 V; (b) Ug = 4 V
Figure 4. Drain current of SGDY and NGDY nanoribbon transistors varying with bias voltage under (a) Ug = 0 V and (b) Ug = 4 V
图 5 SGDY纳米带晶体管在偏置电压0—1.0 V范围内的电子透射谱(栅极电压Ug = 4 V)
Figure 5. Electron transmission spectra of SGDY nanoribbon transistors in the bias voltage range of 0−1.0 V under gate voltage Ug = 4 V.
表 1 使用Dmol3程序的计算方法和参数设置
Table 1. Scheme and parameter setting up in calculationswith Dmol3 program.
电子态描述及求解方法 计算方案 参数设置 交换相关泛函 GGA PBEsol[24] 电子与原子实相互作用(core treatment) 全电子相对论(all electron relativistic) 数值基组 双数值极化(DNP) 轨道截至(orbital cutoff) Global 5.0 Å SCF 容忍度 1 × 10–6 Ha/原子 (1 Ha = 27.2 eV) 多极展开 八极 密度混合 电荷和自旋混合幅度分别为0.2和0.5 轨道占据热拖尾(smearing)[25] 0.001 Ha 布里渊区积分k点取样(电子结构) Monkhorst-Pack格点[26] 1 × 1 × 25 计算范德瓦耳斯相互作用 DFT交换-相关泛函色散校正[27] 布里渊区积分k点取样(电子输运) 均匀间隔格点 间隔0.02/Å 泊松求解法和泊松边界条件(电子输运) 器件侧面缓冲长度 7.5 Å 泊松网格最大格点间距 0.5 Å 电极界面边界条件 Dirichlet 非电极界面边界条件 Neumann 电极边界区缓冲长度 3 Å 
DownLoad: CSV
表 2 不同纳米带晶体管在不同栅极电压下的PVR
Table 2. PVR for nanoribbon transistors fabricated with different materials under different gate voltage.
DownLoad: CSV
-
[1] Pi S, Lin P, Xia Q 2016 Nanotechnology 27 464004
Google Scholar
[2] Lawrence T C, Vashishtha V, Shifren L, Gujja A, Sinha S, Cline B, Ramamurthy C, Yeric G 2016 Microelect. J. 53 105
Google Scholar
[3] Guo Y G, Wang F Q, Wang Q 2017 Appl. Phys. Lett. 111 073503
Google Scholar
[4] Punniyakoti S, Sivakumarasamy R, Vaurette F, Joseph P, Nishiguchi K, Fujiwara A, Clement N 2017 Adv. Mater. Interf. 4 1601155
Google Scholar
[5] Murugesan A 2014 Int. J. Innovative Sci. Eng. Tech. 1 264
[6] Akbar F, Kolahduz M, Larimian S, Radamson H H 2015 J. Mater. Sci. Mater. Elect. 26 4347
Google Scholar
[7] Park J S, Choi H J 2015 Phys. Rev. B 92 045402
Google Scholar
[8] Mihnev M T, Wang F, Liu G, Rothwell S, Cohen P I, Feldman L C, Conrad E H, Norris T B 2015 Appl. Phys. Lett. 107 173107
Google Scholar
[9] Li G, Li Y, Liu H, Guo Y, Li Y, Zhu D 2010 Chem. Commun. 46 3256
Google Scholar
[10] Cranford S W, Buehler M J 2011 Carbon 49 4111
Google Scholar
[11] Kang J, Li J, Wu F, Li S S, Xia J B 2011 J. Phys. Chem. C 115 20466
Google Scholar
[12] Long M, Tang L, Wang D, Li Y, Shuai Z 2011 ACS Nano 5 2593
Google Scholar
[13] Zhou J, Lü K, Wang Q, Chen X S, Sun Q, Jena P 2011 J. Chem. Phys. 134 174701
Google Scholar
[14] Capasso F, Kiehl R A 1985 J. Appl. Phys. 58 1366
Google Scholar
[15] Teong H, Lam K T, Khalid S B, Liang G 2009 J. Appl. Phys. 105 084317
Google Scholar
[16] Britnell L, Gorbachev R V, Geim A K, Ponomarenko L A, Mishchenko A, Greenaway M T, Fromhold T M, Novoselov K S, Eaves L 2013 Nat. Commun. 4 1794
Google Scholar
[17] Mishchenko A, Tu J S, Cao Y, Gorbachev R V, Wallbank J R, Greenaway M T, Morozov V E, Morozov S V, Zhu M J, Wong S L, Withers F, Woods C R, Kim Y J, Watanabe K, Taniguchi T, Vdovin E E, Makarovsky O, Fromhold T M, FaI’ko V I, Geim A K, Eaves L, Novoselov K S 2014 Nat. Nanotech. 9 808
Google Scholar
[18] Özçelik V O, Durgun E, Ciraci S 2015 J. Phys. Chem. C 119 13248
Google Scholar
[19] Chowdhury S, Chattaraj S, Biswas D 2015 J. Semicond. 36 044001
Google Scholar
[20] Allis D G, Prokhorova D, Korter T M 2006 J. Phys. Chem. A 110 1951
Google Scholar
[21] Niehaus T A, Rohlfing M, Della Sala F, Di Carlo A, Frauenheim T 2005 Phys. Rev. A 71 022508
Google Scholar
[22] Pecchia A, Penazzi G, Salvucci L, Di Carlo A 2008 New J. Phys. 10 065022
Google Scholar
[23] Chantis A N, Christensen N E, Svane A, Cardona M 2010 Phys. Rev. B 81 205205
Google Scholar
[24] Perdew J P, Ruzsinszky A, Csonka G I, Vydrov O A, Scuseria G E, Constantin L A, Zhou X L, Burke K 2008 Phys. Rev. Lett. 100 136406
Google Scholar
[25] Weinert M, Davenport J W 1992 Phys. Rev. B 45 13709
Google Scholar
[26] Monkhorst H J, Pack J D 1976 Phys. Rev. B 13 5188
Google Scholar
[27] Tkatchenko A, Scheffler M 2009 Phys. Rev. Lett. 102 073005
Google Scholar
[28] Ghanbari Shohany B, RoknabadiM R, Kompany A 2016 Commun. Theor. Phys. 65 99
Google Scholar
[29] Dong J, Li H, Li L 2013 NPG Asia Mater. 5 e56
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 10963
- PDF Downloads: 149
- Cited By: 0
