-
Diatomic molecule BeC has a complex electronic structure with a large number of low-lying excited states that are all strongly bound electronic states. Thus, the BeC molecule has the abundant spectral information. In this work, the potential energy curves and wavefunctions of
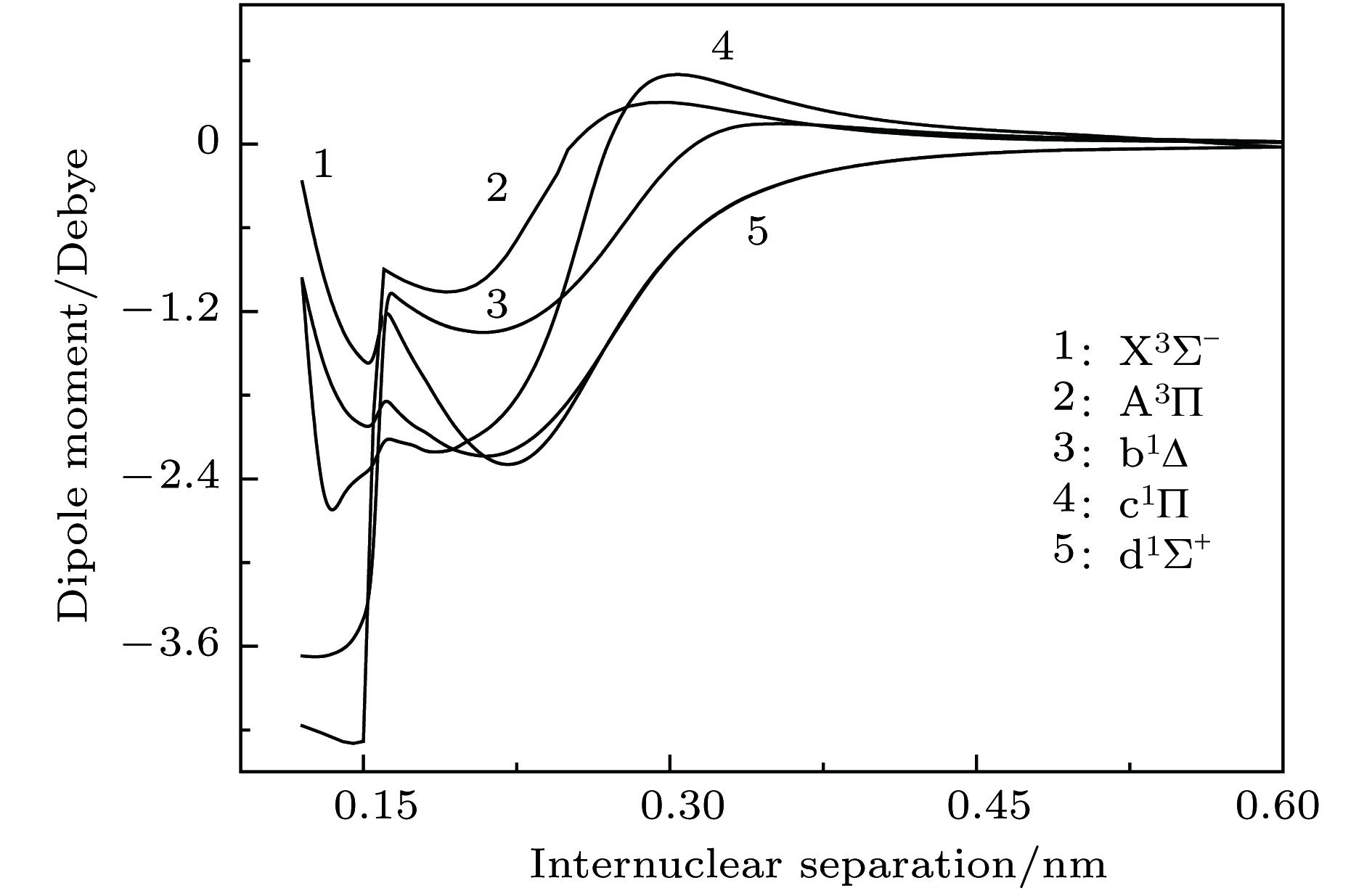
$ {{\rm{X}}^3} {{\text{Σ}} ^ - } $ ,${\rm{A}}^3 {\text{Π}}$ ,$ {{\rm{b}}^1} {{\text{Δ}} } $ ,${{\rm{c}}^1} {\text{Π}}$ and$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ states of the BeC molecule are calculated by using the internally contracted multi-reference configuration interaction (MRCI) approach, which is based on the use of a dynamically weighted complete active space self-consistent field (DW-CASSCF) procedure. To improve the reliability and accuracy of calculation, the scalar relativistic corrections and the extrapolation of potential energy to the complete basis set limit are taken into account. On the basis of the calculated potential energy curves and wavefunctions, the spectroscopic constants (Te, Re,${\omega _{\rm{e}}}$ ,${\omega _{\rm{e}}}{x_{\rm{e}}}$ ,${\omega _{\rm{e}}}{y_{\rm{e}}}$ , Be,${\alpha _{\rm{e}}}$ , and De) and permanent dipole moments of those states are determined, the results of which are in good agreement with the existing available experimental and theoretical values. The obtained permanent dipole moments indicate that the electrons transfer from Be to C and the polarity for molecule is$ {\rm{B}}{{\rm{e}}^{{\text{δ}} + }}{{\rm{C}}^{{\text{δ}} - }}$ . The transition properties of the spin-allowed${\rm{A}}^3 {\text{Π}}$ −$ {{\rm{X}}^3} {{\text{Σ}} ^ - } $ ,${{\rm{c}}^1} {\text{Π}}$ −$ {{\rm{b}}^1} {{\text{Δ}} } $ ,${{\rm{c}}^1} {\text{Π}}$ −$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ transitions are predicted, including the transition dipole moments, Franck-Condon factors, and radiative lifetimes. The radiative lifetimes for the${\rm{A}}^3 {\text{Π}}$ −$ {{\rm{X}}^3} {{\text{Σ}} ^ - } $ transitions are predicated to be at a$ {{\text{µ}}\rm{ s}}$ level, and the good agreement with previous theoretical values is found. Radiative lifetimes for${{\rm{c}}^1} {\text{Π}}$ −$ {{\rm{b}}^1} {{\text{Δ}} } $ and${{\rm{c}}^1} {\text{Π}}$ −$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ transitions are also evaluated at the levels of$ {{\text{µ}}\rm{ s}}$ and ms, respectively. The PEC for the ground state is fitted into accurate analytical potential energy functions by using the extended-Rydberg potential function.-
Keywords:
- dynamically weighted /
- spectroscopic constants /
- transition properties /
- BeC
[1] Borodin D, Doerner R, Nishijima D, Kirschner A, Kreter A, Matveev D, Galonska A, Philipps V 2011 J. Nucl. Mater. 415 219
Google Scholar
[2] Conn R W, Doerner R P, Won J 1997 Fusion Eng. Des. 37 481
[3] Chen M D, Li X B, Yang J, Zhang Q E, Au C T 2006 Int. J. Mass Spectrom. 253 30
Google Scholar
[4] Chen M D, Li X B, Yang J, Zhang Q E 2006 J. Phys. Chem. A 110 4502
[5] Zhang C J 2006 J. Mol. Struc.: Theochem. 759 201
Google Scholar
[6] Barker B J, Antonov I O, Merritt J M, Bondybey V E, Heaven M C, Dawes R 2012 J. Chem. Phys. 137 214313
Google Scholar
[7] Wright J S, Kolbuszewski M 1993 J. Chem. Phys. 98 9725
Google Scholar
[8] Borin A C, Ornellas F R 1993 J. Chem. Phys. 98 8761
Google Scholar
[9] da Silva C O, Teixeira F E C, Azevedo, J A T, Da Silva E C, Nascimento M A C 1996 Int. J. Quant. Chem. 60 433
Google Scholar
[10] Pelegrini M, Roberto-Neto O, Ornellas F R, Machado F B C 2004 Chem. Phys. Lett. 383 143
Google Scholar
[11] Wells N, Lane I C 2011 Phys. Chem. Chem. Phys. 13 19036
Google Scholar
[12] Koch W, Frenking G, Gauss J, Cremer D, Sawaryn A, Schleyer P V R 1986 J. Am. Chem. Soc. 108 5732
Google Scholar
[13] Fioressi S E, Binning Jr R C, Bacelo D E 2014 Chem. Phys. 443 76
Google Scholar
[14] Patrick A D, Williams P, Blaisten-Barojas E 2007 J. Mol. Struc.: Theochem. 824 39
Google Scholar
[15] Ghouri M M, Yareeda L, Mainardi D S 2007 J. Phys. Chem. A 111 13133
Google Scholar
[16] Midda S, Das A K 2004 J. Mol. Spectrosc. 224 1
Google Scholar
[17] Borin A C, Ornellas F R 1995 Chem. Phys. 190 43
Google Scholar
[18] Borin A C, Ornellas F R A 1995 J. Mol. Struc.: Theochem 335 107
Google Scholar
[19] Teberekidis V I, Kerkines I S K, Carsky P, Tsipis C A, Mavridis A 2005 Int. J. Quant. Chem. 102 762
Google Scholar
[20] Deskevich M P, Nesbitt D J, Werner H J 2004 J. Chem. Phys. 120 7281
Google Scholar
[21] Dawes R, Jasper A W, Tao C, Richmond C, Mukarakate C, Kable S H, Reid S A 2010 J. Phys. Chem. Lett. 1 641
Google Scholar
[22] Werner H J, Knowles P J 1988 J. Chem. Phys. 89 5803
Google Scholar
[23] Knowles P J, Werner H J 1988 Chem. Phys. Lett. 145 514
Google Scholar
[24] Werner H J, Knowles P, Knizia G, Manby F R, Schütz M, Celani P, Korona T, Lindh R, Mitrushenkov A, Rauhut G 2010 Molpro Version 2010.1: A Package of ab initio Programs
[25] Peterson K A, Wilson A K, Woon D E, Dunning Jr T H 1997 Theor. Chem. Acc. 97 251
Google Scholar
[26] Woon D E, Dunning Jr T H 1995 J. Chem. Phys. 103 4572
Google Scholar
[27] Prascher B P, Woon D E, Peterson K A, Dunning T H, Wilson A K 2011 Theor. Chem. Acc. 128 69
Google Scholar
[28] Karton A, Martin J M L. 2006 Theor. Chem. Acc. 115 330
Google Scholar
[29] Halkier A, Helgaker T, Jørgesen P, Klopper W, Koch H, Olsen J, Wilson A K 1998 Chem. Phys. Lett. 286 243
Google Scholar
[30] Nakajima T, Hirao K 2000 J. Chem. Phys. 113 7786
Google Scholar
[31] Fedorov D G, Nakajima T, Hirao K 2003 J. Chem. Phys. 118 4970
Google Scholar
[32] Paulovic J, Nakajima T, Hirao K, Lindh R, Malmqvist P Å 2003 J. Chem. Phys. 119 798
Google Scholar
[33] NIST, Atomic Spectra Database, see http://www.nist.gov/pml/data/asd.cfm (2012)
[34] Haris K, Kramida A, 2017 Astrophys. J. Suppl. S. 233 16
Google Scholar
[35] LeRoy R J 2010 LEVEL 8.0: A Computer Program for Solving the Radial Schrödinger Equation for Bound and Quasibound Levels, Chemical Physics: Research Report CP-663 (Ontario: University of Waterloo)
[36] Herzberg G. 1950 Molecular Spectra and Molecular Structure I. Spectra of Diatomic Molecules(New York: Van Nostrand Reinhold) p658
[37] Murrel J N, Carter S, Farantos S C, Huxley P, Varandas A J C 1984 Molecular Potential Energy Functions (Wiley: Chichester, U. K.) p9
[38] 7D-Soft High Technology, Inc. 1st Opt User Manual (V.6.0) Beijing, People’s Republic of China, 2014
-
图 1 BeC分子5个电子态的势能曲线(1 Hartree = 2625.4984 kJ/mol)
Figure 1. Potential energy curves of five states of BeC molecule (1 Hartree = 2625.4984 kJ/mol).
图 2 BeC分子5个电子态的偶极矩(1 Debye = 3.336 × 10–30 C·m)
Figure 2. Dipole moments of five electronic states of BeC(1 Debye = 3.336 × 10–30 C·m).
图 3 BeC分子基态的Mulliken电荷分布
Figure 3. Mulliken population for the ground state BeC molecule.
图 4 跃迁偶极距曲线
Figure 4. Transition dipole moment curves.
表 1 BeC分子6个态的离解极限关系
Table 1. Dissociation relationship of six electronic states of BeC molecule.
原子态 $ {\text{Λ}}{\text{-}}{\rm S}$态 相对能量/cm–1 本文 (无Q) 本文 (+Q) 实验值[34] Be(1Sg)+C(3Pg) $ {{\rm{X}}^3} {{\text{Σ}} ^ - } $, ${\rm{A}}^3 {\text{Π}}$ 0 0 Be(1Sg)+C(1Dg) $ {{\rm{b}}^1} {{\text{Δ}} } $, ${{\rm{c}}^1} {\text{Π}}$, $ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ 10124.12 10169.70 10192.66 Be(1Sg)+C(1Sg) ${2^1}{{\text{Σ}} ^ + } $ 21679.58 21581.62 21648.03 
DownLoad: CSV
表 2 BeC分子
$ {{\rm{X}}^3}{\text{Σ}} $ ,${{\rm{A}}^3}{\text{Π}}$ ,$ {{\rm{b}}^1} {{\text{Δ}} } $ ,${{\rm{c}}^1} {\text{Π}}$ 和$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ 等5个态的光谱参数Table 2. Spectroscopic constants of the five states of BeC molecule.
Te/cm–1 Re/nm ${\omega _{\rm{e}}}$/cm–1 $ {\omega _{\rm{e}}}{x_{\rm{e}}} $/cm–1 102${\omega _{\rm{e}}}$уe/cm–1 Be/cm–1 $ {10^3}{\alpha _{\rm{e}}}$/cm–1 De/eV ${{{\rm{X}}^3}{\text{Σ}}^- } $ 0 0.1673 918.08 7.350 17.87 1.1783 15.644 2.1873 Cal. [6] 0 0.1661 937.9 9.6 — 1.19 — — Cal. [7] 0 0.1693 905 — — — — 2.04 Cal. [8] 0 0.1667 951 8.42 — 1.183 — 2.39 Cal. [10] 0 0.1683 925 11.25 — — — 2.14 Cal. [19] 0 0.1680 — — — — — 2.04 ${{\rm{A}}^3}{\text{Π}}$ 8916.35 0.1771 772.74 8.692 199.89 1.0518 28.367 1.0777 Cal. [7] 9033.41 0.1799 — — — — — 0.92 Cal. [8] 9466 0.1756 764 14.69 — 1.0752 — 1.16 Cal. [10] 8961 0.1791 874 26.26 — — — 1.03 $ {{\rm{b}}^1}{\text{Δ}} $ 7823.39 0.1675 933.50 8.301 7.56 1.1714 16.700 2.4408 Cal. [7] 8872.10 0.1693 904 — — — — 2.27 Cal. [8] 8732 0.1668 956 7.6 — 1.1757 — 2.63 $ {{\rm{c}}^1}{\text{Π}} $ 10909.21 0.1760 834.80 7.138 9.20 1.0551 14.365 2.0933 Cal. [7] 11291.76 0.1778 818 — — — — 1.97 Cal. [8] 11618 0.1758 847 6.94 — 1.0612 — 2.24 $ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ 12139.14 0.16698 936.21 17.553 126.98 1.1764 16.000 1.9060 Cal. [7] 12582.24 0.1693 905 — — — — 1.81 Cal. [8] 13579 0.167 955 7.3 — 1.1732 — 2.02 注: Cal. 为理论计算值.
DownLoad: CSV
表 3
${{\rm{A}}^3}{\text{Π}}$ —$ {{\rm{X}}^3} {{\text{Σ}} ^ - } $ ,${{\rm{c}}^1}{\text{Π}}$ —$ {{\rm{b}}^1} {{\text{Δ}} } $ ,$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ —${{\rm{c}}^1}{\text{Π}}$ 跃迁的弗兰克-康登因子Table 3. Franck-condon factor for
${{\rm{A}}^3}{\text{Π}}$ −$ {{\rm{X}}^3} {{\text{Σ}} ^ - } $ ,${{\rm{c}}^1}{\text{Π}}$ −$ {{\rm{b}}^1}{{\text{Δ}} } $ ,$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ −${{\rm{c}}^1}{\text{Π}}$ transitions.$\nu '$—$ \nu ''$ FC $\nu '$—$ \nu ''$ FC $\nu '$—$ \nu ''$ FC $\nu '$—$ \nu ''$ FC $\nu '$—$ \nu ''$ FC $\nu '$— $ \nu ''$ FC ${\rm{A}}^3 {\text{Π}}$—${\rm{X}}^3 {\text{Σ}}^-$ 0—0 0.5439 0—1 0.3653 1—0 0.3049 1—2 0.4143 1—3 0.1670 2—0 0.1080 2—1 0.2660 2—3 0.3601 2—4 0.2276 3—2 0.1654 3—4 0.2908 3—5 0.2691 4—2 0.1763 4—5 0.2255 4—6 0.2993 5—3 0.1561 5—6 0.1639 5—7 0.3216 6—3 0.1070 6—4 0.1273 6—6 0.1339 6—7 0.1052 6—8 0.3348 6—9 0.1183 7—4 0.1095 7—5 0.1000 7—7 0.1618 7—9 0.3342 7—10 0.1531 ${{\rm{c}}^1}{\text{Π}}$ —${{\rm{b}}^1}{\text{Δ}}$ 0—0 0.6237 0—1 0.3153 1—0 0.2701 1—1 0.1740 1—2 0.3999 1—3 0.1368 2—1 0.2689 2—3 0.3470 2—4 0.2322 3—1 0.1518 3—2 0.1803 3—4 0.2122 3—5 0.2964 4—2 0.1894 4—4 0.1027 4—5 0.1045 4—6 0.3042 4—7 0.1238 5—3 0.1634 5—5 0.1435 5—7 0.3138 6—3 0.1236 6—4 0.1268 6—6 0.1567 6—8 0.2968 7—4 0.1289 7—7 0.1495 7—9 0.2690 8—5 0.1219 8—8 0.1212 8—10 0.2380 9—11 0.2025 10—12 0.1699 ${{\rm{d}}^1}{\text{Σ}}$—${{\rm{c}}^1}{\text{Π}}$ 0—0 0.6164 0—1 0.2717 1—0 0.3232 1—1 0.1693 1—2 0.2617 2—1 0.4016 2—3 0.1699 2—4 0.1850 3—1 0.3901 3—2 0.3513 3—5 0.1510 4—2 0.2362 4—3 0.2156 4—4 0.1073 4—6 0.1068 5—3 0.3014 5—4 0.1011 5—5 0.1501 6—4 0.3109 6—6 0.1613 7—4 0.1292 7—5 0.3175 7—7 0.1442 8—5 0.1801 8—6 0.2945 8—8 0.1054 9—6 0.2192 9—7 0.2612 10—7 0.2563 10—8 0.2265
DownLoad: CSV
表 4
${\rm{A}}^3 {\text{Π}}$ ,$ {{\rm{b}}^1} {{\text{Δ}} } $ ,${{\rm{c}}^1}{\text{Π}}$ 和$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ 态几个振动能级的辐射寿命Table 4. Radiative lifetime of the vibrational energy levels for
${\rm{A}}^3 {\text{Π}}$ ,$ {{\rm{b}}^1} {{\text{Δ}} } $ ,${{\rm{c}}^1} {\text{Π}}$ and$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ states.跃迁 辐射寿命$/{{\text{μ}}{\rm{ s}}}$ $\nu '$ = 0 $\nu '$ = 1 $\nu '$ = 2 $\nu '$ = 3 $\nu '$ = 4 $\nu '$ = 5 $\nu '$ = 6 $\nu '$ = 7 ${\rm{A}}^3 {\text{Π}}$—${{\rm{X}}^3}{{\text{Σ}} ^ - }$ 14.66 14.64 14.46 13.82 12.99 12.16 11.65 11.31 Cal.[10] 14.0 14.5 15.6 ${{\rm{c}}^1} {\text{Π}}$—$ {{\rm{b}}^1} {{\text{Δ}} } $ 191.10 166.59 161.38 159.48 151.88 176.09 197.32 239.85 ${{\rm{c}}^1} {\text{Π}}$—$ {{\rm{d}}^1}{{\text{Σ}} ^ + } $ 2730.00 1010.00 630.00 460.00 380.00 330 280.00 230.00
DownLoad: CSV
表 5 BeC分子基态解析势能函数参数值
Table 5. The values for the analytic parameters for the ground state of BeC molecule.
参数 C1 C2 C3 C4 C5 参数值 4.584720688 6.825173375 4.979780662 2.012372055 1.900852427 参数 C6 C7 C8 C9 C10 参数值 –5.415648763 –6.033517345 12.82526525 –6.086434893 0.939009741
DownLoad: CSV
-
[1] Borodin D, Doerner R, Nishijima D, Kirschner A, Kreter A, Matveev D, Galonska A, Philipps V 2011 J. Nucl. Mater. 415 219
Google Scholar
[2] Conn R W, Doerner R P, Won J 1997 Fusion Eng. Des. 37 481
[3] Chen M D, Li X B, Yang J, Zhang Q E, Au C T 2006 Int. J. Mass Spectrom. 253 30
Google Scholar
[4] Chen M D, Li X B, Yang J, Zhang Q E 2006 J. Phys. Chem. A 110 4502
[5] Zhang C J 2006 J. Mol. Struc.: Theochem. 759 201
Google Scholar
[6] Barker B J, Antonov I O, Merritt J M, Bondybey V E, Heaven M C, Dawes R 2012 J. Chem. Phys. 137 214313
Google Scholar
[7] Wright J S, Kolbuszewski M 1993 J. Chem. Phys. 98 9725
Google Scholar
[8] Borin A C, Ornellas F R 1993 J. Chem. Phys. 98 8761
Google Scholar
[9] da Silva C O, Teixeira F E C, Azevedo, J A T, Da Silva E C, Nascimento M A C 1996 Int. J. Quant. Chem. 60 433
Google Scholar
[10] Pelegrini M, Roberto-Neto O, Ornellas F R, Machado F B C 2004 Chem. Phys. Lett. 383 143
Google Scholar
[11] Wells N, Lane I C 2011 Phys. Chem. Chem. Phys. 13 19036
Google Scholar
[12] Koch W, Frenking G, Gauss J, Cremer D, Sawaryn A, Schleyer P V R 1986 J. Am. Chem. Soc. 108 5732
Google Scholar
[13] Fioressi S E, Binning Jr R C, Bacelo D E 2014 Chem. Phys. 443 76
Google Scholar
[14] Patrick A D, Williams P, Blaisten-Barojas E 2007 J. Mol. Struc.: Theochem. 824 39
Google Scholar
[15] Ghouri M M, Yareeda L, Mainardi D S 2007 J. Phys. Chem. A 111 13133
Google Scholar
[16] Midda S, Das A K 2004 J. Mol. Spectrosc. 224 1
Google Scholar
[17] Borin A C, Ornellas F R 1995 Chem. Phys. 190 43
Google Scholar
[18] Borin A C, Ornellas F R A 1995 J. Mol. Struc.: Theochem 335 107
Google Scholar
[19] Teberekidis V I, Kerkines I S K, Carsky P, Tsipis C A, Mavridis A 2005 Int. J. Quant. Chem. 102 762
Google Scholar
[20] Deskevich M P, Nesbitt D J, Werner H J 2004 J. Chem. Phys. 120 7281
Google Scholar
[21] Dawes R, Jasper A W, Tao C, Richmond C, Mukarakate C, Kable S H, Reid S A 2010 J. Phys. Chem. Lett. 1 641
Google Scholar
[22] Werner H J, Knowles P J 1988 J. Chem. Phys. 89 5803
Google Scholar
[23] Knowles P J, Werner H J 1988 Chem. Phys. Lett. 145 514
Google Scholar
[24] Werner H J, Knowles P, Knizia G, Manby F R, Schütz M, Celani P, Korona T, Lindh R, Mitrushenkov A, Rauhut G 2010 Molpro Version 2010.1: A Package of ab initio Programs
[25] Peterson K A, Wilson A K, Woon D E, Dunning Jr T H 1997 Theor. Chem. Acc. 97 251
Google Scholar
[26] Woon D E, Dunning Jr T H 1995 J. Chem. Phys. 103 4572
Google Scholar
[27] Prascher B P, Woon D E, Peterson K A, Dunning T H, Wilson A K 2011 Theor. Chem. Acc. 128 69
Google Scholar
[28] Karton A, Martin J M L. 2006 Theor. Chem. Acc. 115 330
Google Scholar
[29] Halkier A, Helgaker T, Jørgesen P, Klopper W, Koch H, Olsen J, Wilson A K 1998 Chem. Phys. Lett. 286 243
Google Scholar
[30] Nakajima T, Hirao K 2000 J. Chem. Phys. 113 7786
Google Scholar
[31] Fedorov D G, Nakajima T, Hirao K 2003 J. Chem. Phys. 118 4970
Google Scholar
[32] Paulovic J, Nakajima T, Hirao K, Lindh R, Malmqvist P Å 2003 J. Chem. Phys. 119 798
Google Scholar
[33] NIST, Atomic Spectra Database, see http://www.nist.gov/pml/data/asd.cfm (2012)
[34] Haris K, Kramida A, 2017 Astrophys. J. Suppl. S. 233 16
Google Scholar
[35] LeRoy R J 2010 LEVEL 8.0: A Computer Program for Solving the Radial Schrödinger Equation for Bound and Quasibound Levels, Chemical Physics: Research Report CP-663 (Ontario: University of Waterloo)
[36] Herzberg G. 1950 Molecular Spectra and Molecular Structure I. Spectra of Diatomic Molecules(New York: Van Nostrand Reinhold) p658
[37] Murrel J N, Carter S, Farantos S C, Huxley P, Varandas A J C 1984 Molecular Potential Energy Functions (Wiley: Chichester, U. K.) p9
[38] 7D-Soft High Technology, Inc. 1st Opt User Manual (V.6.0) Beijing, People’s Republic of China, 2014
DownLoad:
Catalog
Metrics
- Abstract views: 9195
- PDF Downloads: 68
- Cited By: 0
