Three-dimensional spatial orbital angular momentum holography

Jia Yi-Cheng; Zhang Fu-Rong; Zhang Jing-Feng; Kong Ling-Jun; Zhang Xiang-Dong

doi:10.7498/aps.73.20231822

Abstract

The degree of freedom of orbital angular momentum of light has been used as a new information carrier in optical holographic information processing technology. However, current research on orbital angular momentum holography mainly focuses on two-dimensional orbital angular momentum holography, where the reconstructed two-dimensional holographic image is located in a certain plane in three-dimensional space. How to further implement three-dimensional spatial orbital angular momentum holographic technology and use it to increase the information capacity of holographic communication is still a blank. Here, we implement three-dimensional spatial orbital angular momentum holographic technology based on the degrees of freedom of orbital angular momentum and the positional degrees of freedom of reconstructed two-dimensional images in three-dimensional space. In other words, in the three-dimensional spatial orbital angular momentum holography, the acquisition of the target object image requires not only the correct orbital angular momentum state used for decoding, but also the correct spatial position where the object’s image is detected. In addition, we further investigate the three-dimensional spatial orbit angular momentum holographic multiplexing technology and point out that this multiplexing technology can be used for information encryption. Compared with traditional two-dimensional orbital angular momentum holography, three-dimensional spatial orbital angular momentum holography uses an additional degree of freedom. Therefore, the encryption scheme based on three-dimensional spatial orbital angular momentum holographic technology can further improve the security level of information. Our simulation results and experimental results have verified the feasibility of three-dimensional spatial orbit angular momentum holographic technology and three-dimensional spatial orbit angular momentum holographic encryption technology.

Keywords:

Authors and contacts

Beijing Key Laboratory of Nanophotonics & Ultrafine Optoelectronic Systems, Key Laboratory of Advanced Optoelectronic Quantum Architecture and Measurements of Ministry of Education, School of Physics, Beijing Institute of Technology, Beijing 100081, China

Corresponding author: Kong Ling-Jun, konglj@bit.edu.cn

Funds: Project supported by the National Natural Science Foundation of China (Grant Nos. 12374324, 12004038).

FullText HTML : translate this paragraph

References

[1]	Gabor D 1948 Nature 161 777 Google Scholar
[2]	Blanche P A, Bablumian A, Voorakaranam R, et al. 2010 Nature 468 80 Google Scholar
[3]	Huang L, Chen X, Mühlenbernd H, et al. 2013 Nat. Commun. 4 2808 Google Scholar
[4]	Li X, Ren H, Chen X, et al. 2015 Nat. Commun. 6 6984 Google Scholar
[5]	Wakunami K, Hsieh P Y, Oi R, et al. 2016 Nat. Commun. 7 12954 Google Scholar
[6]	Pégard N C, Mardinly A R, Oldenburg I A, Sridharan S, Waller L, Adesnik H 2017 Nat. Commun. 8 1228 Google Scholar
[7]	Yu H, Lee K, Park J, Park Y 2017 Nat. Photon. 11 186 Google Scholar
[8]	Makey G, Yavuz Ö, Kesim D K, Turnalı A, Elahi P, Ilday S, Tokel O, Ilday F Ö 2019 Nat. Photonics 13 251 Google Scholar
[9]	Marquet P, Rappaz B, Magistretti P J, Cuche E, Emery Y, Colomb T, Depeursinge C 2005 Opt. Lett. 30 468 Google Scholar
[10]	Rosen J, Brooker G 2008 Nat. Photonics 2 190 Google Scholar
[11]	赵应春, 张秀英, 袁操今, 聂守平, 朱竹青, 王林, 李杨, 贡丽萍, 冯少彤 2014 63 224202 Google Scholar Zhao Y C, Zhang X Y, Yuan C J, Nie S P, Zhu Z Q, Wang L, Li Y, Gong L P, Feng S T 2014 Acta Phys. Sin. 63 224202 Google Scholar
[12]	Refregier P, Javidi B 1995 Opt. Lett. 20 767 Google Scholar
[13]	Chen W, Javidi B, Chen X 2014 Adv. Opt. Photonics 6 120 Google Scholar
[14]	王仁德, 张亚萍, 朱旭锋, 王帆, 李重光, 张永安, 许蔚 2019 68 114202 Google Scholar Wang R D, Zhang Y P, Zhu X F, Wang F, Li C G, Zhang Y A, Xu W 2019 Acta Phys. Sin. 68 114202 Google Scholar
[15]	席思星, 于娜娜, 王晓雷, 朱巧芬, 董昭, 王微, 刘秀红, 王华英 2019 68 110502 Google Scholar Xi S X, Yu N N, Wang X L, Zhu Q F, Dong Z, Wang W, Liu X H, Wang H Y 2019 Acta Phys. Sin. 68 110502 Google Scholar
[16]	Heanue J F, Bashaw M C, Hesselink L 1994 Science 265 749 Google Scholar
[17]	Larocque H, Sugic D, Mortimer D, Taylor A J, Fickler R, Boyd R W, Dennis M R, Karimi E 2018 Nat. Phys. 14 1079 Google Scholar
[18]	Wang S, Wang L, Zhang F, Kong L J 2022 Chin. Phys. Lett. 39 104101 Google Scholar
[19]	Guo X, Li P, Zhong J, Liu S, Wei B, Zhu W, Qi S, Cheng H, Zhao J 2020 Laser Photonics Rev. 14 1900366 Google Scholar
[20]	Kong L J, Zhang W, Li P, Guo X, Zhang J, Zhang F, Zhao J, Zhang X 2022 Nat. Commun. 13 2705 Google Scholar
[21]	Ozaki M, Kato J, Kawata S 2011 Science 332 218 Google Scholar
[22]	Li X, Chen L, Li Y, Zhang X, Pu M, Zhao Z, Ma X, Wang Y, Hong M, Luo X 2016 Sci. Adv. 2 e1601102 Google Scholar
[23]	Bao Y, Yu Y, Xu H, Guo C, Li J, Sun S, Zhou Z K, Qiu C W, Wang X H 2019 Light-Sci. Appl. 8 95 Google Scholar
[24]	Lim K T P, Liu H, Liu Y, Yang J K W 2019 Nat. Commun. 10 25 Google Scholar
[25]	Shen X A, Nguyen A D, Perry J W, Huestis D L, Kachru R 1997 Science 278 96 Google Scholar
[26]	Tikan A, Bielawski S, Szwaj C, Randoux S, Suret P 2018 Nat. Photonics 12 228 Google Scholar
[27]	Balthasar Mueller J P, Rubin N A, Devlin R C, Groever B, Capasso F 2017 Phys. Rev. Lett. 118 113901 Google Scholar
[28]	Zhao R, Sain B, Wei Q, Tang C, Li X, Weiss T, Huang L, Wang Y, Zentgraf T 2018 Light Sci. Appl. 7 95 Google Scholar
[29]	Defienne H, Ndagano B, Lyons A, Faccio D 2021 Nat. Phys. 17 591 Google Scholar
[30]	Wang J, Yang J Y, Fazal I M, et al. 2012 Nat. Photonics 6 488 Google Scholar
[31]	Huang H, Xie G, Yan Y, et al. 2014 Opt. Lett. 39 197 Google Scholar
[32]	Krenn M, Handsteiner J, Fink M, Fickler R, Ursin R, Malik M, Zeilinger A 2016 Proc. Natl. Acad. Sci. U. S. A. 113 13648 Google Scholar
[33]	Lavery M P J, Peuntinger C, Günthner K, et al. 2017 Sci. Adv. 3 e1700552 Google Scholar
[34]	Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545 Google Scholar
[35]	Mair A, Vaziri A, Weihs G, Zeilinger A 2001 Nature 412 313 Google Scholar
[36]	Kong L J, Li Y, Liu R, et al. 2019 Phys. Rev. A 100 023822 Google Scholar
[37]	Kong L J, Liu R, Qi W R, Wang Z X, Huang S Y, Wang Q, Tu C, Li Y, Wang H T 2019 Sci. Adv. 5 eaat9206 Google Scholar
[38]	Kong L J, Sun Y, Zhang F, Zhang J, Zhang X 2023 Phys. Rev. Lett. 130 053602 Google Scholar
[39]	Zhang Y, Agnew M, Roger T, Roux F S, Konrad T, Faccio D, Leach J, Forbes A 2017 Nat. Commun. 8 632 Google Scholar
[40]	Dada A C, Leach J, Buller G S, Padgett M J, Andersson E 2011 Nat. Phys. 7 677 Google Scholar
[41]	Ren H, Briere G, Fang X, Ni P, Sawant R, Héron S, Chenot S, Vézian S, Damilano B, Brändli V, Maier S A, Genevet P 2019 Nat. Commun. 10 2986 Google Scholar
[42]	Fang X, Ren H, Gu M 2020 Nat. Photonics 14 102 Google Scholar
[43]	Ren H, Fang X, Jang J, Bürger J, Rho J, Maier S A 2020 Nat. Nanotechnol. 15 948 Google Scholar
[44]	Zhou H, Sain B, Wang Y, Schlickriede C, Zhao R, Zhang X, Wei Q, Li X, Huang L, Zentgraf T 2020 ACS Nano 14 5553 Google Scholar
[45]	Fang X, Yang H, Yao W, Wang T, Zhang Y, Gu M, Xiao M 2021 Adv. Photonics 3 015001 Google Scholar
[46]	Zhu G, Bai Z, Chen J, Huang C, Wu L, Fu C, Wang Y 2021 Opt. Express 29 28452 Google Scholar
[47]	Wang F, Zhang X, Xiong R, Ma X, Jiang X 2022 Opt. Express 30 11110 Google Scholar
[48]	Shi Z, Wan Z, Zhan Z, Liu K, Liu Q, Fu X 2023 Nat. Commun. 14 1869 Google Scholar
[49]	Zhang F, Kong L J, Zhang Z, Zhang J, Zhang X 2023 Opt. Express 31 12922 Google Scholar

Cited By

图 1 用于实现三维空间OAM全息的全息图设计方案. 通过将目标图像与二维Dirac梳状采样阵列相乘即可获得能够保留OAM特征的全息图; 然后通过在保留OAM全息图上添加OAM态的螺旋相位分布, 生成具有OAM特征选择性的全息图; 最后, 使用FZP对OAM选择性全息图进行编码, 即可获得用于三维空间OAM全息的全息图. 这里, FZP的“焦距”($ {f_{{\text{FZP}}}} $)控制着重构出的全息图像在三维空间中的位置

Figure 1. Design of the holograms of three-dimensional (3D) spatial OAM holography. The OAM-preserved holograms are obtained by multiplying the target images with the two-dimensional Dirac comb sampling array. Then, the OAM-selective holograms are generated by adding a phase function of OAM modes onto the OAM-preserved hologram. 3D spatial OAM-selective holograms are obtained by encoding the OAM-selective holograms with the Fresnel zone plates (FZP). The positions of the reconstructed holographic images are controlled by the focal lengths of FZP ($ {f_{{\text{FZP}}}} $).

DownLoad: Full-Size Img PowerPoint

图 2 三维空间OAM全息的理论模拟结果　(a) 理论模拟中选用的6个目标图像; (b) 6个目标图像的三维空间OAM全息图; (c)三维空间OAM全息中, 6个图像重构的理论模拟结果.

Figure 2. Simulation results of the reconstructed images of 3D spatial OAM holography: (a) The six target images; (b) holograms of 3D spatial OAM holography of six target images; (c) simulation results of reconstructed images in 3D spatial OAM holography.

DownLoad: Full-Size Img PowerPoint

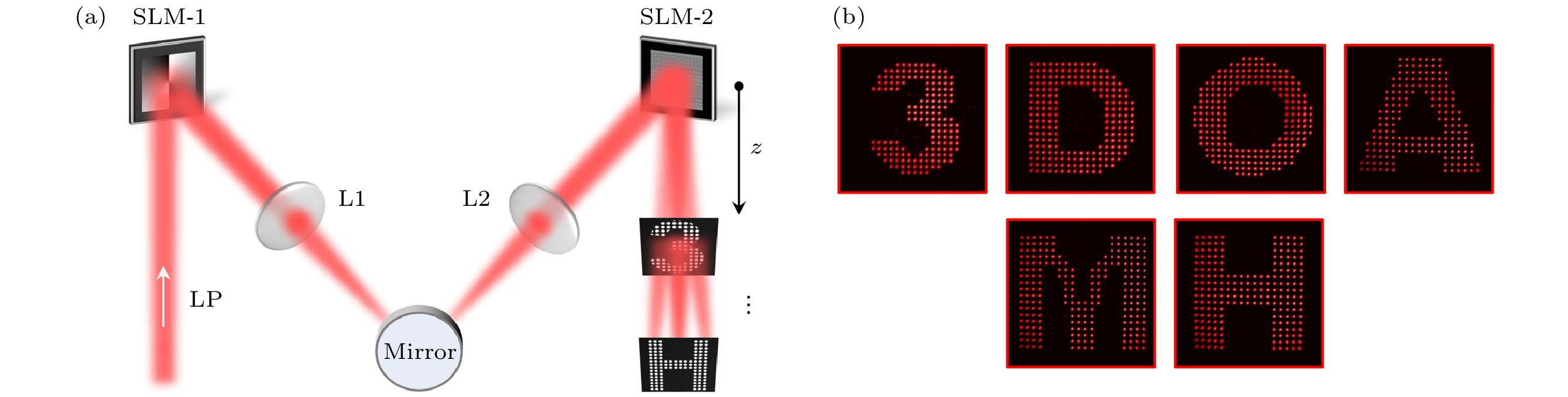
图 3 三维空间OAM全息的实验装置及其重构结果　(a) 实验装置. 波长633 nm、输出功率10 mW线偏振连续激光经过SLM-1调制后携带OAM态; 由透镜L1和L2组成的4f系统将SLM-1成像到SLM-2上; 三维空间 OAM全息的全息图加载于SLM-2上. 携带OAM态的光场经SLM-2衍射后, 在三维空间中重构出的目标物体的全息图像; (b) 与图2(c)中一一对应的三维空间OAM全息图像重构的实验结果

Figure 3. Experiments and results of 3D spatial OAM hologram reconstruction. (a) Experimental setup. The light source is a continuous-wave laser operating at 633 nm with an output power of ~10 mW. The incident light, after modulated by SLM-1, carries the desired OAM state. A 4f system consisted of lenses L1 and L2 images SLM-1 onto the SLM-2. The holographic images of objects will be reconstructed in 3D space after the light field carrying OAM state is diffracted by SLM-2. (b) The experimental results of 3D spatial OAM holographic image reconstruction, which corresponds to the simulation results in Fig. 2 (c).

DownLoad: Full-Size Img PowerPoint

图 4 三维空间OAM多路复用全息　(a) 三维空间OAM多路复用全息的全息图设计; (b) 三维空间OAM多路复用全息的重构的实验结果. 只有当入射光束具备正确的OAM态, 且在正确的全息图像重构平面内时, 才会出现清晰的图像. 这里, 每一行的三个实验数据已做了归一化处理

Figure 4. 3D spatial OAM holographic multiplexing technology. (a) Design of the holograms for 3D spatial OAM holographic multiplexing technology. (b) The experimental results for the 3D spatial OAM holographic multiplexing technology. Only when the incident beam has the correct OAM state and is in the correct holographic image reconstruction plane, will a clear image appear. Here, the three experimental data in each row have been normalized.

DownLoad: Full-Size Img PowerPoint

图 5 在三维空间OAM全息中, 全息重构图像的间距对串扰的影响. 第一行表示用于编码的三个FZP的“焦距”$ {f_{{\text{FZP}}}} $= z₁, $ {f_{{\text{FZP}}}} $= z₂和$ {f_{{\text{FZP}}}} $=z₃的大小. 在重构的结果中, 每一个重构图像的左上角的数字为该图像对应的参数$ R = {{{{\bar I}_{{\text{pigeon}}}}} \mathord{\left/ {\vphantom {{{{\bar I}_{{\text{pigeon}}}}} {{{\bar I}_{{\text{non - pigeon}}}}}}} \right. } {{{\bar I}_{{\text{non - pigeon}}}}}} $的值. $ {\bar I_{{\text{pigeon}}}} $和$ {\bar I_{{\text{non - pigeon}}}} $分别代表鸽子区域和非鸽子区域的强度分布的平均值

Figure 5. Influence of the distances among the holographic reconstructed images on crosstalk in 3D spatial OAM holography. The first line represents the values of the “focal length” of the three FZPs used for encoding, $ {f_{{\text{FZP}}}} $= z₁, $ {f_{{\text{FZP}}}} $= z₂, and $ {f_{{\text{FZP}}}} $= z₃. In the reconstructed results, the number in the upper left corner of each image represents its value of the parameter $ R = {{{{\bar I}_{{\text{pigeon}}}}} \mathord{\left/ {\vphantom {{{{\bar I}_{{\text{pigeon}}}}} {{{\bar I}_{{\text{non - pigeon}}}}}}} \right. } {{{\bar I}_{{\text{non - pigeon}}}}}} $, where among them, $ {\bar I_{{\text{pigeon}}}} $represents the average intensity value in the pigeon areas and $ {\bar I_{{\text{non - pigeon}}}} $ represents the average intensity distribution in non-pigeon areas.

DownLoad: Full-Size Img PowerPoint

map

[1]	Gabor D 1948 Nature 161 777 Google Scholar
[2]	Blanche P A, Bablumian A, Voorakaranam R, et al. 2010 Nature 468 80 Google Scholar
[3]	Huang L, Chen X, Mühlenbernd H, et al. 2013 Nat. Commun. 4 2808 Google Scholar
[4]	Li X, Ren H, Chen X, et al. 2015 Nat. Commun. 6 6984 Google Scholar
[5]	Wakunami K, Hsieh P Y, Oi R, et al. 2016 Nat. Commun. 7 12954 Google Scholar
[6]	Pégard N C, Mardinly A R, Oldenburg I A, Sridharan S, Waller L, Adesnik H 2017 Nat. Commun. 8 1228 Google Scholar
[7]	Yu H, Lee K, Park J, Park Y 2017 Nat. Photon. 11 186 Google Scholar
[8]	Makey G, Yavuz Ö, Kesim D K, Turnalı A, Elahi P, Ilday S, Tokel O, Ilday F Ö 2019 Nat. Photonics 13 251 Google Scholar
[9]	Marquet P, Rappaz B, Magistretti P J, Cuche E, Emery Y, Colomb T, Depeursinge C 2005 Opt. Lett. 30 468 Google Scholar
[10]	Rosen J, Brooker G 2008 Nat. Photonics 2 190 Google Scholar
[11]	赵应春, 张秀英, 袁操今, 聂守平, 朱竹青, 王林, 李杨, 贡丽萍, 冯少彤 2014 63 224202 Google Scholar Zhao Y C, Zhang X Y, Yuan C J, Nie S P, Zhu Z Q, Wang L, Li Y, Gong L P, Feng S T 2014 Acta Phys. Sin. 63 224202 Google Scholar
[12]	Refregier P, Javidi B 1995 Opt. Lett. 20 767 Google Scholar
[13]	Chen W, Javidi B, Chen X 2014 Adv. Opt. Photonics 6 120 Google Scholar
[14]	王仁德, 张亚萍, 朱旭锋, 王帆, 李重光, 张永安, 许蔚 2019 68 114202 Google Scholar Wang R D, Zhang Y P, Zhu X F, Wang F, Li C G, Zhang Y A, Xu W 2019 Acta Phys. Sin. 68 114202 Google Scholar
[15]	席思星, 于娜娜, 王晓雷, 朱巧芬, 董昭, 王微, 刘秀红, 王华英 2019 68 110502 Google Scholar Xi S X, Yu N N, Wang X L, Zhu Q F, Dong Z, Wang W, Liu X H, Wang H Y 2019 Acta Phys. Sin. 68 110502 Google Scholar
[16]	Heanue J F, Bashaw M C, Hesselink L 1994 Science 265 749 Google Scholar
[17]	Larocque H, Sugic D, Mortimer D, Taylor A J, Fickler R, Boyd R W, Dennis M R, Karimi E 2018 Nat. Phys. 14 1079 Google Scholar
[18]	Wang S, Wang L, Zhang F, Kong L J 2022 Chin. Phys. Lett. 39 104101 Google Scholar
[19]	Guo X, Li P, Zhong J, Liu S, Wei B, Zhu W, Qi S, Cheng H, Zhao J 2020 Laser Photonics Rev. 14 1900366 Google Scholar
[20]	Kong L J, Zhang W, Li P, Guo X, Zhang J, Zhang F, Zhao J, Zhang X 2022 Nat. Commun. 13 2705 Google Scholar
[21]	Ozaki M, Kato J, Kawata S 2011 Science 332 218 Google Scholar
[22]	Li X, Chen L, Li Y, Zhang X, Pu M, Zhao Z, Ma X, Wang Y, Hong M, Luo X 2016 Sci. Adv. 2 e1601102 Google Scholar
[23]	Bao Y, Yu Y, Xu H, Guo C, Li J, Sun S, Zhou Z K, Qiu C W, Wang X H 2019 Light-Sci. Appl. 8 95 Google Scholar
[24]	Lim K T P, Liu H, Liu Y, Yang J K W 2019 Nat. Commun. 10 25 Google Scholar
[25]	Shen X A, Nguyen A D, Perry J W, Huestis D L, Kachru R 1997 Science 278 96 Google Scholar
[26]	Tikan A, Bielawski S, Szwaj C, Randoux S, Suret P 2018 Nat. Photonics 12 228 Google Scholar
[27]	Balthasar Mueller J P, Rubin N A, Devlin R C, Groever B, Capasso F 2017 Phys. Rev. Lett. 118 113901 Google Scholar
[28]	Zhao R, Sain B, Wei Q, Tang C, Li X, Weiss T, Huang L, Wang Y, Zentgraf T 2018 Light Sci. Appl. 7 95 Google Scholar
[29]	Defienne H, Ndagano B, Lyons A, Faccio D 2021 Nat. Phys. 17 591 Google Scholar
[30]	Wang J, Yang J Y, Fazal I M, et al. 2012 Nat. Photonics 6 488 Google Scholar
[31]	Huang H, Xie G, Yan Y, et al. 2014 Opt. Lett. 39 197 Google Scholar
[32]	Krenn M, Handsteiner J, Fink M, Fickler R, Ursin R, Malik M, Zeilinger A 2016 Proc. Natl. Acad. Sci. U. S. A. 113 13648 Google Scholar
[33]	Lavery M P J, Peuntinger C, Günthner K, et al. 2017 Sci. Adv. 3 e1700552 Google Scholar
[34]	Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545 Google Scholar
[35]	Mair A, Vaziri A, Weihs G, Zeilinger A 2001 Nature 412 313 Google Scholar
[36]	Kong L J, Li Y, Liu R, et al. 2019 Phys. Rev. A 100 023822 Google Scholar
[37]	Kong L J, Liu R, Qi W R, Wang Z X, Huang S Y, Wang Q, Tu C, Li Y, Wang H T 2019 Sci. Adv. 5 eaat9206 Google Scholar
[38]	Kong L J, Sun Y, Zhang F, Zhang J, Zhang X 2023 Phys. Rev. Lett. 130 053602 Google Scholar
[39]	Zhang Y, Agnew M, Roger T, Roux F S, Konrad T, Faccio D, Leach J, Forbes A 2017 Nat. Commun. 8 632 Google Scholar
[40]	Dada A C, Leach J, Buller G S, Padgett M J, Andersson E 2011 Nat. Phys. 7 677 Google Scholar
[41]	Ren H, Briere G, Fang X, Ni P, Sawant R, Héron S, Chenot S, Vézian S, Damilano B, Brändli V, Maier S A, Genevet P 2019 Nat. Commun. 10 2986 Google Scholar
[42]	Fang X, Ren H, Gu M 2020 Nat. Photonics 14 102 Google Scholar
[43]	Ren H, Fang X, Jang J, Bürger J, Rho J, Maier S A 2020 Nat. Nanotechnol. 15 948 Google Scholar
[44]	Zhou H, Sain B, Wang Y, Schlickriede C, Zhao R, Zhang X, Wei Q, Li X, Huang L, Zentgraf T 2020 ACS Nano 14 5553 Google Scholar
[45]	Fang X, Yang H, Yao W, Wang T, Zhang Y, Gu M, Xiao M 2021 Adv. Photonics 3 015001 Google Scholar
[46]	Zhu G, Bai Z, Chen J, Huang C, Wu L, Fu C, Wang Y 2021 Opt. Express 29 28452 Google Scholar
[47]	Wang F, Zhang X, Xiong R, Ma X, Jiang X 2022 Opt. Express 30 11110 Google Scholar
[48]	Shi Z, Wan Z, Zhan Z, Liu K, Liu Q, Fu X 2023 Nat. Commun. 14 1869 Google Scholar
[49]	Zhang F, Kong L J, Zhang Z, Zhang J, Zhang X 2023 Opt. Express 31 12922 Google Scholar

[1]	Chen Bo, Liu Jin, Li Jun-Tao, Wang Xue-Hua. Research progress of integrated quantum light sources with orbital angular momentum. Acta Physica Sinica, 2024, 73(16): 164204. doi: 10.7498/aps.73.20240791
[2]	Zhang Zhuo, Zhang Jing-Feng, Kong Ling-Jun. Orbital angular momentum splitter of light based on beam displacer. Acta Physica Sinica, 2024, 73(7): 074201. doi: 10.7498/aps.73.20231874
[3]	Xu Meng-Min, Li Xiao-Qing, Tang Rong, Ji Xiao-Ling. Influence of wind-dominated thermal blooming on orbital angular momentum and phase singularity of dual-mode vortex beams. Acta Physica Sinica, 2023, 72(16): 164202. doi: 10.7498/aps.72.20230684
[4]	Wu Hang, Chen Liao, Shu Xue-Wen, Zhang Xin-Liang. Generation of all-fiber third-order orbital angular momentum modes based on femtosecond laser processing of long-period grating. Acta Physica Sinica, 2023, 72(4): 044201. doi: 10.7498/aps.72.20221928
[5]	Zhao Li-Juan, Jiang Huan-Qiu, Xu Zhi-Niu. Helically twisted double-cladding-three-core photonic crystal fiber for generation of orbital angular momentum. Acta Physica Sinica, 2023, 72(13): 134201. doi: 10.7498/aps.72.20222405
[6]	Yang Xin-Yu, Ye Hua-Peng, Li Pei-Yun, Liao He-Lin, Yuan Dong, Zhou Guo-Fu. Miniaturized optical vortex mode demultiplexer: Principle, fabrication, and applications. Acta Physica Sinica, 2023, 72(20): 204207. doi: 10.7498/aps.72.20231521
[7]	Liu Rui-Xi, Ma Lei. Effects of ocean turbulence on photon orbital angular momentum quantum communication. Acta Physica Sinica, 2022, 71(1): 010304. doi: 10.7498/aps.71.20211146
[8]	Gao Xi, Tang Li-Guang. Wideband and high efficiency orbital angular momentum generator based on bi-layer metasurface. Acta Physica Sinica, 2021, 70(3): 038101. doi: 10.7498/aps.70.20200975
[9]	Jiang Ji-Heng, Yu Shi-Xing, Kou Na, Ding Zhao, Zhang Zheng-Ping. Beam steering of orbital angular momentum vortex wave based on planar phased array. Acta Physica Sinica, 2021, 70(23): 238401. doi: 10.7498/aps.70.20211119
[10]	Cui Can, Wang Zhi, Li Qiang, Wu Chong-Qing, Wang Jian. Modulation of orbital angular momentum in long periodchirally-coupled-cores fiber. Acta Physica Sinica, 2019, 68(6): 064211. doi: 10.7498/aps.68.20182036
[11]	Fan Rong-Hua, Guo Bang-Hong, Guo Jian-Jun, Zhang Cheng-Xian, Zhang Wen-Jie, Du Ge. Entangled W state of multi degree of freedom system based on orbital angular momentum. Acta Physica Sinica, 2015, 64(14): 140301. doi: 10.7498/aps.64.140301
[12]	Ke Xi-Zheng, Chen Juan, Yang Yi-Ming. Study on orbital angular momentum of Laguerre-Gaussian beam in a slant-path atmospheric turbulence. Acta Physica Sinica, 2014, 63(15): 150301. doi: 10.7498/aps.63.150301
[13]	Qi Xiao-Qing, Gao Chun-Qing, Xin Jing-Tao, Zhang Ge. Experimental study of 8-bits information transmission system based on orbital angular momentum of light beams. Acta Physica Sinica, 2012, 61(17): 174204. doi: 10.7498/aps.61.174204
[14]	Li Tie, Chen Juan, Ke Xi-Zheng. Study of orbital angular momentum entangled photons entanglement in atmospheric channel. Acta Physica Sinica, 2012, 61(12): 124208. doi: 10.7498/aps.61.124208
[15]	Ke Xi-Zheng, Nu Ning, Yang Qin-Ling. Research of transmission characteristics of single-photon orbital angular momentum. Acta Physica Sinica, 2010, 59(9): 6159-6163. doi: 10.7498/aps.59.6159
[16]	Chen Xiao-Yi, Li Hai-Xia, Song Hong-Sheng, Teng Shu-Yun, Cheng Chuan-Fu, Liu Man. Measurement of orbital angular momentum of Laguerre-Gaussian beam by using phase vortices of interference fields. Acta Physica Sinica, 2010, 59(12): 8490-8498. doi: 10.7498/aps.59.8490
[17]	Lü Hong, Ke Xi-Zheng. Scattering of a beam with orbital angular momentum by a single sphere. Acta Physica Sinica, 2009, 58(12): 8302-8308. doi: 10.7498/aps.58.8302
[18]	Su Zhi-Kun, Wang Fa-Qiang, Lu Yi-Qun, Jin Rui-Bo, Liang Rui-Sheng, Liu Song-Hao. Study on quantum cryptography using orbital angular momentum states of photons. Acta Physica Sinica, 2008, 57(5): 3016-3021. doi: 10.7498/aps.57.3016
[19]	Gao Ming-Wei, Gao Chun-Qing, Lin Zhi-Feng. Generation of twisted stigmatic beam and transfer of orbital angular momentum during the beam transformation. Acta Physica Sinica, 2007, 56(4): 2184-2190. doi: 10.7498/aps.56.2184
[20]	Gao Ming-Wei, Gao Chun-Qing, He Xiao-Yan, Li Jia-Ze, Wei Guang-Hui. Rotation of particles by using the beamwith orbital angular momentum. Acta Physica Sinica, 2004, 53(2): 413-417. doi: 10.7498/aps.53.413

Catalog

Vol. 73, Issue 9 - 2024-05-05

Metrics

Abstract views: 2870
PDF Downloads: 278
Cited By: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

Search

Article

留言板