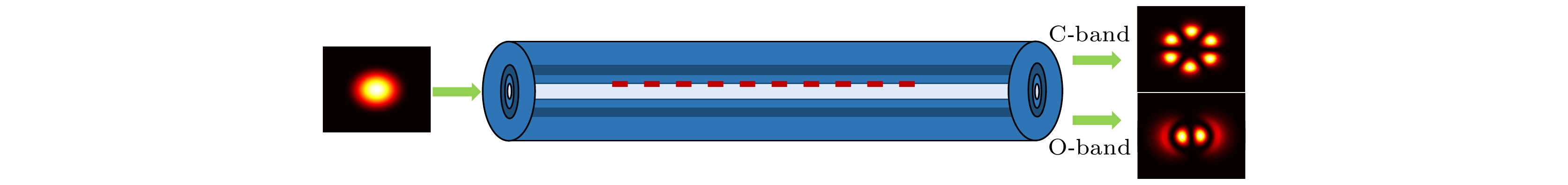
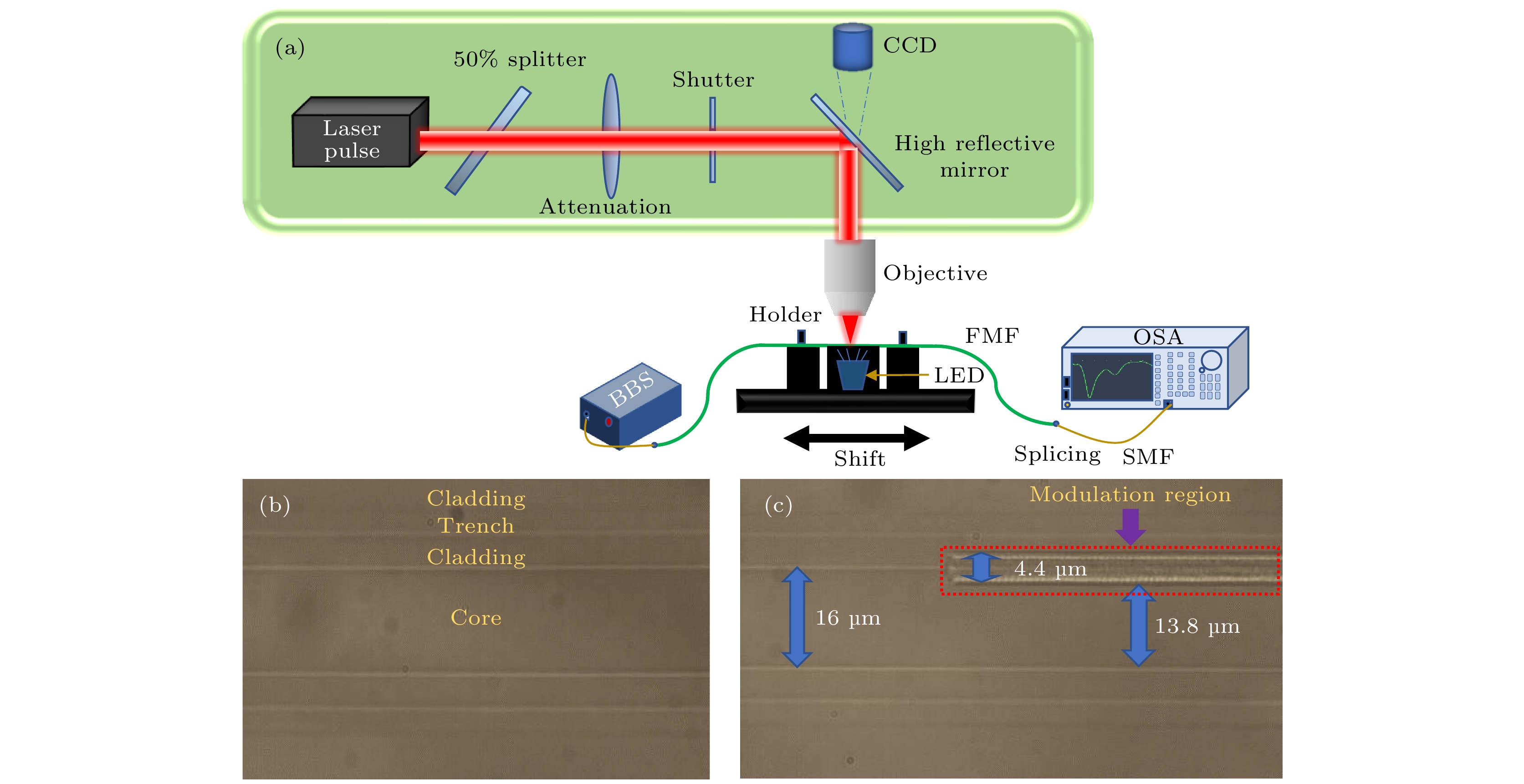
-
高效地产生相互正交的各阶轨道角动量(orbital angular momentum, OAM)模式具有重要的研究价值. 目前全光纤系统中高效地产生高阶轨道角动量模式的方法主要是基于二氧化碳激光器加工的长周期光纤光栅(long period fiber grating, LPFG). 然而产生高阶模式的光栅需要强的折射率调制与小的光栅周期, 因此二氧化碳激光器高的功率和大的聚焦光斑不利于其刻写的重复性、成功率和延展性. 为了解决这一问题, 本文首次提出并制作了基于飞秒激光加工的三阶OAM模式转换器, 在六模光纤上加工出了非对称的长周期光纤光栅, 实验结果表明其在1550 nm附近能将基模转换为三阶的角向线性偏振模式LP31模式, 模式转换效率为98%, 该模式可进一步被叠加转化为三阶OAM模式. 与此同时, 在1310 nm附近, 该光栅还能够产生角向一阶径向二阶的OAM模式. 本文证明了飞秒激光加工提供了一种可用于全光纤系统, 具有高重复刻写性的长周期光纤光栅来产生高阶OAM模式的思路.The generation of orbital angular momentum (OAM) modes is very important, for they have a variety of applications such as in optical tweezers, quantum optics, and optical communication systems. Particularly, how can high-order OAM modes be generated efficiently in fibers with the advantage of low cost and compatible with fiber system? The Traditional method for first order to third order OAM is based on long period fiber grating (LPFG) fabricated by carbon dioxide laser. However, high power and large focused spot of carbon dioxide laser are unfavorable for stable and repeatable generation of higher-order OAM, which needs the LPFG with small grating pitch. In order to solve this problem, a third-order OAM mode converter based on femtosecond microfabrication is proposed and fabricated for the first time. With the advantage of 4.4 μm focused spot size near the core, lower power and lower heat absorption efficiency, this method can be more stable and promising. Therefore, we first carry out the mode filed analysis and simulate the intensity and phase profiles of the superposed mode field in LP odd-even mode on different scales and phases patterns to obtain OAM mode. Second, we use the coupled-mode theory to analyze and simulate the transmission spectrum of LPFG, which guides the setting of the grating parameters such as the grating pitch, the depth of modulation and the length of the grating. By experimental verification, an asymmetric modulated long-period fiber grating with a pitch setting to 194 μm is fabricated on a six-mode fiber. The fundamental mode can be converted into the third-order angular linear polarization mode LP31 mode with 98% mode conversion efficiency near 1550 nm, and further converted into the OAM±3 modes by superposition of the odd and even LP31 mode with ±π/2 phase difference. At the same time, this fiber grating can also generate LP12 mode with 90% mode conversion efficiency near 1325 nm. Then we can take the same approach to transform LP12 mode into OAM modes with angular first-order as well as radial second-order. The experimental result is consistent with the simulation result. Thus, this scheme provides an idea for generating the high-order OAM modes in all-fiber systems by using only one grating with high repeatability.
-
Keywords:
- orbital angular momentum /
- fiber grating /
- fiber optics components /
- fiber optics communications
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Poynting J H 1909 Proc. R. Soc. London Ser. A 82 560
Google Scholar
[3] Bliokh K Y, Rodríguez-Fortuño F J, Nori F, Zayats A V 2015 Nat. Photonics 9 796
Google Scholar
[4] Vitullo D L, Leary C C, Gregg P, Smith R A, Reddy D V, Ramachandran S, Raymer M G 2017 Phys. Rev. Lett. 118 083601
Google Scholar
[5] Grier D 2003 Nature 424 810
Google Scholar
[6] Leach J, Jack B, Romero J, K Jha A, M Yao A, Frank-Arnold S, G Ireland D, W Boyd R, M Barnett S, J Padgett M 2010 Science 329 662
Google Scholar
[7] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y X, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488
Google Scholar
[8] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[9] Naidoo D, Roux F S, Dudley A, Litvin I, Piccirillo B, Marrucci L, Forbes A 2016 Nat. Photonics 10 327
Google Scholar
[10] Cao H, Gao S C, Zhang C, Wang J, He D Y, Liu B H, Guo G C 2020 Optica 7 232
Google Scholar
[11] Wen Y, Chremmos I, Chen Y, Zhu G, Zhang J, Zhu J, Zhang Y, Liu J, Yu S 2020 Optica 7 254
Google Scholar
[12] Beijersbergen M W, Coerwinkel R P C, Kristensen M, Woerdman J P 1994 Opt. Commun. 112 321
Google Scholar
[13] Marrucci L, Karimi E, Slussarenko S, Piccirillo B, Santamato E, Nagali E, Sciarrino, F 2011 J. Opt. 13 064001
Google Scholar
[14] Cai X, Wang J, Strain M J, Johnson-Morris B, Zhu J, Sorel M, O’brien J, Thompson M, Yu S 2012 Science 338 363
Google Scholar
[15] Zhao Z, Wang J, Li S, Willner A E 2013 Opt. Lett. 38 932
Google Scholar
[16] Chen Y, Fang Z X, Ren Y X, Gong L, Lu R D 2015 Appl. Opt. 54 8030
Google Scholar
[17] Fujisawa T, Saitoh K 2020 Photonics. Res. 8 1278
Google Scholar
[18] Ramachandran, S, Kristensen P 2013 Nanophotonics 2 455
Google Scholar
[19] Li S, Mo Q, Hu X, Du C, Wang J 2015 Opt. Lett. 40 4376
Google Scholar
[20] Zhang W, Wei K, Huang L, Mao D, Jiang B, Gao F, Zhao J 2016 Opt. Express 24 19278
Google Scholar
[21] Li Y, Jin L, Wu H, Gao S, Feng Y H, Li Z 2017 Photonics. J. 9 1
Google Scholar
[22] Han Y, Liu Y G, Wang Z, Huang W, Chen L, Zhang H W, Yang K 2018 Nanophotonics 7 287
Google Scholar
[23] Wu H, Gao S, Huang B, Feng Y, Huang X, Liu W, Li Z 2017 Opt. Lett. 42 5210
Google Scholar
[24] Detani T, Zhao H, Wang P, Suzuki T, Li H 2021 Opt. Lett. 46 949
Google Scholar
[25] Shao L, Liu S, Zhou M, Huang Z, Bao W, Bai Z, Wang Y 2021 Opt. Express 29 43371
Google Scholar
[26] He X, Tu J, Wu X, Gao S, Shen L, Hao C, Li Z 2020 Opt. Lett. 45 3621
Google Scholar
[27] Huang H, Milione G, Lavery M P J, Xie G, Ren Y, Cao Y, Ahmed N, Nguyen T A, Nolan D A, Li M, Tur M, Alfano R R, Willner A E 2015 Sci. Rep. 5 1
Google Scholar
[28] Han Y, Liu Y G, Huang W, Wang Z, Guo J, Luo M 24 2016 Opt. Express 17272
Google Scholar
[29] Anemogiannis E, Glytsis E N, Gaylord T K 2003 J. Lightwave Technol. 21 218
[30] Erdogan T 1997 J. Lightwave Technol. 15 1277
[31] Jin L, Jin W, Ju J, Wang Y 2010 J. Lightwave Technol. 28 1745
[32] Barshak E, Alexeyev C, Lapin B, Yavorsky M 2015 Phys. Rev. A 91 033833
Google Scholar
[33] Bernas M, Zolnacz K, Napiorkowski M, Statkiewicz G, Urbanczyk W 2021 Opt. Lett. 46 4446
[34] Pu G Q, Yi L L, Zhang L, Luo C, Li Z H, Hu W S 2020 Light Sci. Appl. 9 13
-
图 1 LP31奇偶模式以不同的比例和相位叠加后的模场的强度和相位图
Fig. 1. Intensity and phase profiles of the superposed mode field in LP31 odd-even mode with different scales and phases.
图 2 (a) 六模光纤横截面以及折射率分布; (b)—(g) 六模光纤中所支持传导的LP模式(LP01, LP11, LP21, LP02, LP31, LP12); (h) 六模光纤的色散曲线
Fig. 2. (a) Cross-section image and transverse refractive index distribution of the 6MF; (b)–(g) fiber-supported LP modes, LP01, LP11, LP21, LP02, LP31 and LP12; (h) mode dispersion curves of the 6MF.
图 3 基模耦合向不同的光纤导模的光栅周期随波长变化曲线
Fig. 3. The grating pitch of fundamental mode coupling to different fiber guide mode varies with wavelength.
图 4 基模通过光栅在不同波段转化为不同模式的示意图
Fig. 4. Schematic representation of a fundamental mode converted to a different mode by a grating at different wavebands.
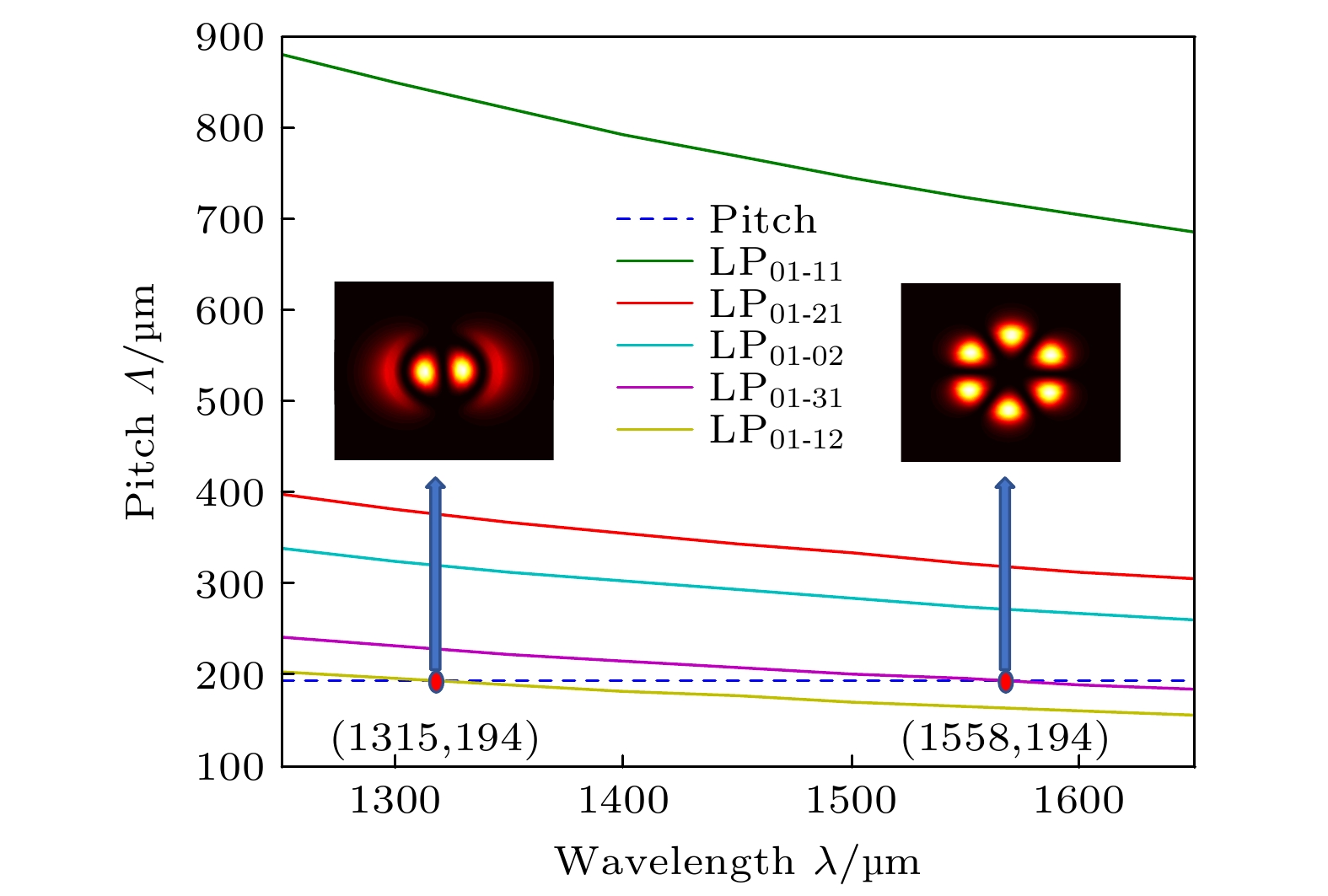
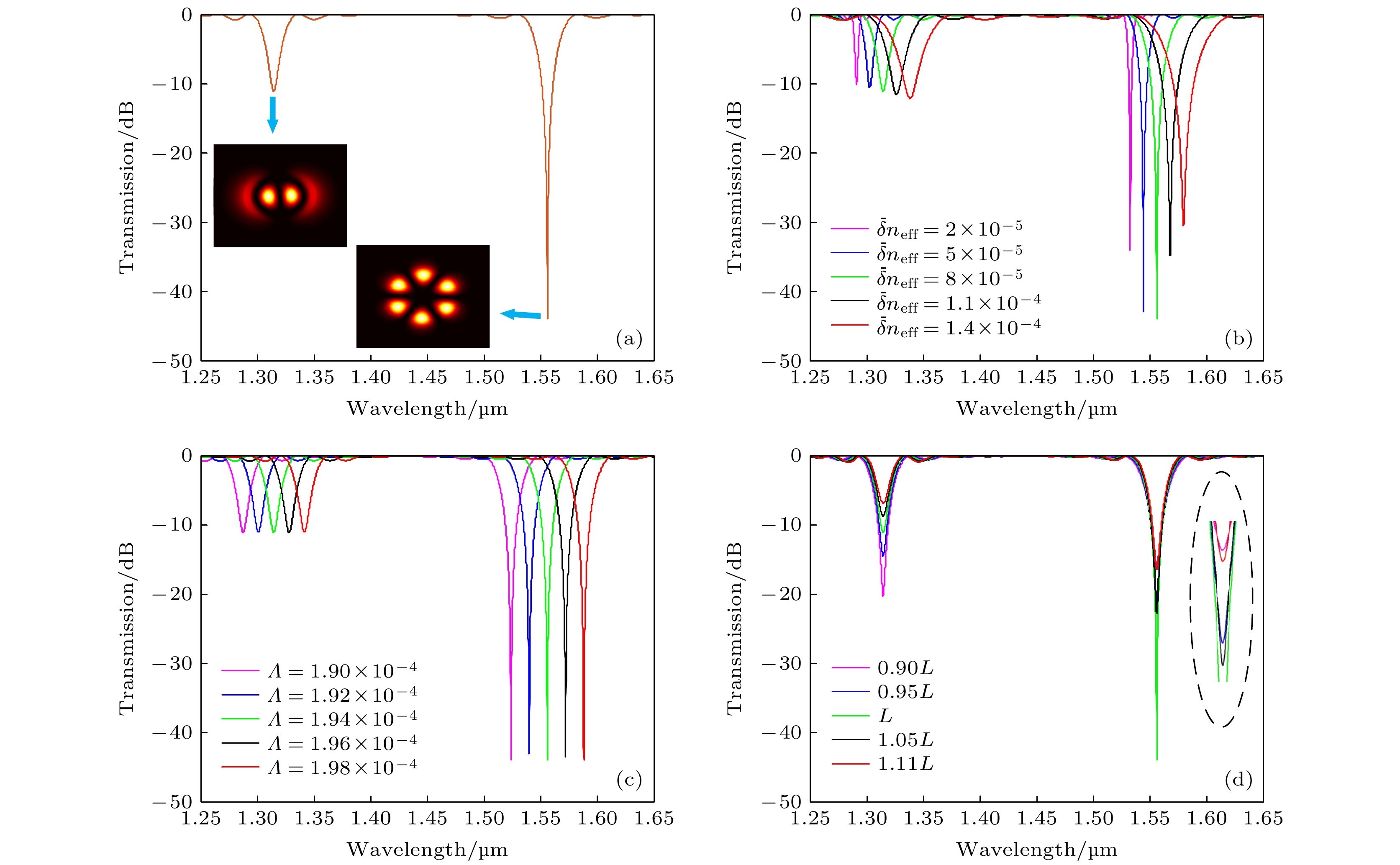
图 5 长周期光纤光栅透射谱 (a) 光栅谐振峰对应的模式; (b) 不同的调制深度对光谱的影响; (c) 不同周期对光谱的影响; (d)不同耦合长度对光谱的影响
Fig. 5. The transmission spectrum of long-period fiber grating: (a) The mode corresponding to the resonant peak of the grating; (b) the influence of different modulation depth on the spectrum; (c) the influence of different pitch on the spectrum; (d) the influence of different coupling length on the spectrum.
图 6 (a)飞秒激光器刻写长周期光纤光栅实验装置; (b)光栅刻写前光纤侧视图; (c)光栅刻写后折射率调制区域侧视图
Fig. 6. (a) Experimental setup of the fabricated LPFG by employing a femtosecond laser; (b) side view of the fiber before LPFG fabricated; (c) side view of the refractive indexation modulation region after LPFG fabricated.
图 7 (a) 六模长周期光纤光栅透射谱的测量以及光栅前后的模场; (b)不同刻写位置光栅透射谱的测量
Fig. 7. (a) Measured transmission spectrum of the 6 MF-LPFG and the mode profile before/after the LPFG; (b) measurement of transmission spectrum of grating at different writing positions.
图 8 三阶OAM模式的产生和验证装置, ATT: 光衰; Col: 准直镜; BS: 光分束镜
Fig. 8. Experimental setup for the generation and detection of the
${\text{OA}}{{\text{M}}_{ \pm 3}}$ , ATT: attenuator; Col: collimator; BS: beam splitter.
图 9 (a)(b)光栅未扭转时产生的LP31奇偶模式的模场; (c)(d)光栅经过扭转后产生的OAM±3模式的模场; (e)(f) OAM±3和参考高斯光干涉的图样
Fig. 9. (a) (b) The intensity profiles of the generated LP31 even-odd modes before twisting the grating; (c) (d) the intensity profiles of the generated OAM±3 modes after twisting the grating; (e) (f) their interference patterns with a reference Gaussian beam.
图 10 LP12模式和OAM±1, 2模式的强度和相位图以及两个模式之间的关系
Fig. 10. Intensity and phase profiles of the LP12and OAM±1, 2 modes, and the relationship between these modes.
图 11 (a)(b)光栅未扭转时产生的LP12奇偶模式的模场; (c)(d)光栅经过扭转后产生的OAM±1, 2模式的模场; (e)(f) OAM±1, 2和参考高斯光干涉的图样
Fig. 11. (a) (b) The intensity profiles of the generated LP12even-odd modes before twisting the grating; (c) (d) the intensity profiles of the generated OAM±1, 2 modes after twisting the grating; (e) (f ) their interference patterns with a reference Gaussian beam.
-
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Poynting J H 1909 Proc. R. Soc. London Ser. A 82 560
Google Scholar
[3] Bliokh K Y, Rodríguez-Fortuño F J, Nori F, Zayats A V 2015 Nat. Photonics 9 796
Google Scholar
[4] Vitullo D L, Leary C C, Gregg P, Smith R A, Reddy D V, Ramachandran S, Raymer M G 2017 Phys. Rev. Lett. 118 083601
Google Scholar
[5] Grier D 2003 Nature 424 810
Google Scholar
[6] Leach J, Jack B, Romero J, K Jha A, M Yao A, Frank-Arnold S, G Ireland D, W Boyd R, M Barnett S, J Padgett M 2010 Science 329 662
Google Scholar
[7] Wang J, Yang J Y, Fazal I M, Ahmed N, Yan Y, Huang H, Ren Y X, Yue Y, Dolinar S, Tur M, Willner A E 2012 Nat. Photonics 6 488
Google Scholar
[8] Bozinovic N, Yue Y, Ren Y, Tur M, Kristensen P, Huang H, Willner A E, Ramachandran S 2013 Science 340 1545
Google Scholar
[9] Naidoo D, Roux F S, Dudley A, Litvin I, Piccirillo B, Marrucci L, Forbes A 2016 Nat. Photonics 10 327
Google Scholar
[10] Cao H, Gao S C, Zhang C, Wang J, He D Y, Liu B H, Guo G C 2020 Optica 7 232
Google Scholar
[11] Wen Y, Chremmos I, Chen Y, Zhu G, Zhang J, Zhu J, Zhang Y, Liu J, Yu S 2020 Optica 7 254
Google Scholar
[12] Beijersbergen M W, Coerwinkel R P C, Kristensen M, Woerdman J P 1994 Opt. Commun. 112 321
Google Scholar
[13] Marrucci L, Karimi E, Slussarenko S, Piccirillo B, Santamato E, Nagali E, Sciarrino, F 2011 J. Opt. 13 064001
Google Scholar
[14] Cai X, Wang J, Strain M J, Johnson-Morris B, Zhu J, Sorel M, O’brien J, Thompson M, Yu S 2012 Science 338 363
Google Scholar
[15] Zhao Z, Wang J, Li S, Willner A E 2013 Opt. Lett. 38 932
Google Scholar
[16] Chen Y, Fang Z X, Ren Y X, Gong L, Lu R D 2015 Appl. Opt. 54 8030
Google Scholar
[17] Fujisawa T, Saitoh K 2020 Photonics. Res. 8 1278
Google Scholar
[18] Ramachandran, S, Kristensen P 2013 Nanophotonics 2 455
Google Scholar
[19] Li S, Mo Q, Hu X, Du C, Wang J 2015 Opt. Lett. 40 4376
Google Scholar
[20] Zhang W, Wei K, Huang L, Mao D, Jiang B, Gao F, Zhao J 2016 Opt. Express 24 19278
Google Scholar
[21] Li Y, Jin L, Wu H, Gao S, Feng Y H, Li Z 2017 Photonics. J. 9 1
Google Scholar
[22] Han Y, Liu Y G, Wang Z, Huang W, Chen L, Zhang H W, Yang K 2018 Nanophotonics 7 287
Google Scholar
[23] Wu H, Gao S, Huang B, Feng Y, Huang X, Liu W, Li Z 2017 Opt. Lett. 42 5210
Google Scholar
[24] Detani T, Zhao H, Wang P, Suzuki T, Li H 2021 Opt. Lett. 46 949
Google Scholar
[25] Shao L, Liu S, Zhou M, Huang Z, Bao W, Bai Z, Wang Y 2021 Opt. Express 29 43371
Google Scholar
[26] He X, Tu J, Wu X, Gao S, Shen L, Hao C, Li Z 2020 Opt. Lett. 45 3621
Google Scholar
[27] Huang H, Milione G, Lavery M P J, Xie G, Ren Y, Cao Y, Ahmed N, Nguyen T A, Nolan D A, Li M, Tur M, Alfano R R, Willner A E 2015 Sci. Rep. 5 1
Google Scholar
[28] Han Y, Liu Y G, Huang W, Wang Z, Guo J, Luo M 24 2016 Opt. Express 17272
Google Scholar
[29] Anemogiannis E, Glytsis E N, Gaylord T K 2003 J. Lightwave Technol. 21 218
[30] Erdogan T 1997 J. Lightwave Technol. 15 1277
[31] Jin L, Jin W, Ju J, Wang Y 2010 J. Lightwave Technol. 28 1745
[32] Barshak E, Alexeyev C, Lapin B, Yavorsky M 2015 Phys. Rev. A 91 033833
Google Scholar
[33] Bernas M, Zolnacz K, Napiorkowski M, Statkiewicz G, Urbanczyk W 2021 Opt. Lett. 46 4446
[34] Pu G Q, Yi L L, Zhang L, Luo C, Li Z H, Hu W S 2020 Light Sci. Appl. 9 13
下载:
计量
- 文章访问数: 4694
- PDF下载量: 151
- 被引次数: 0
