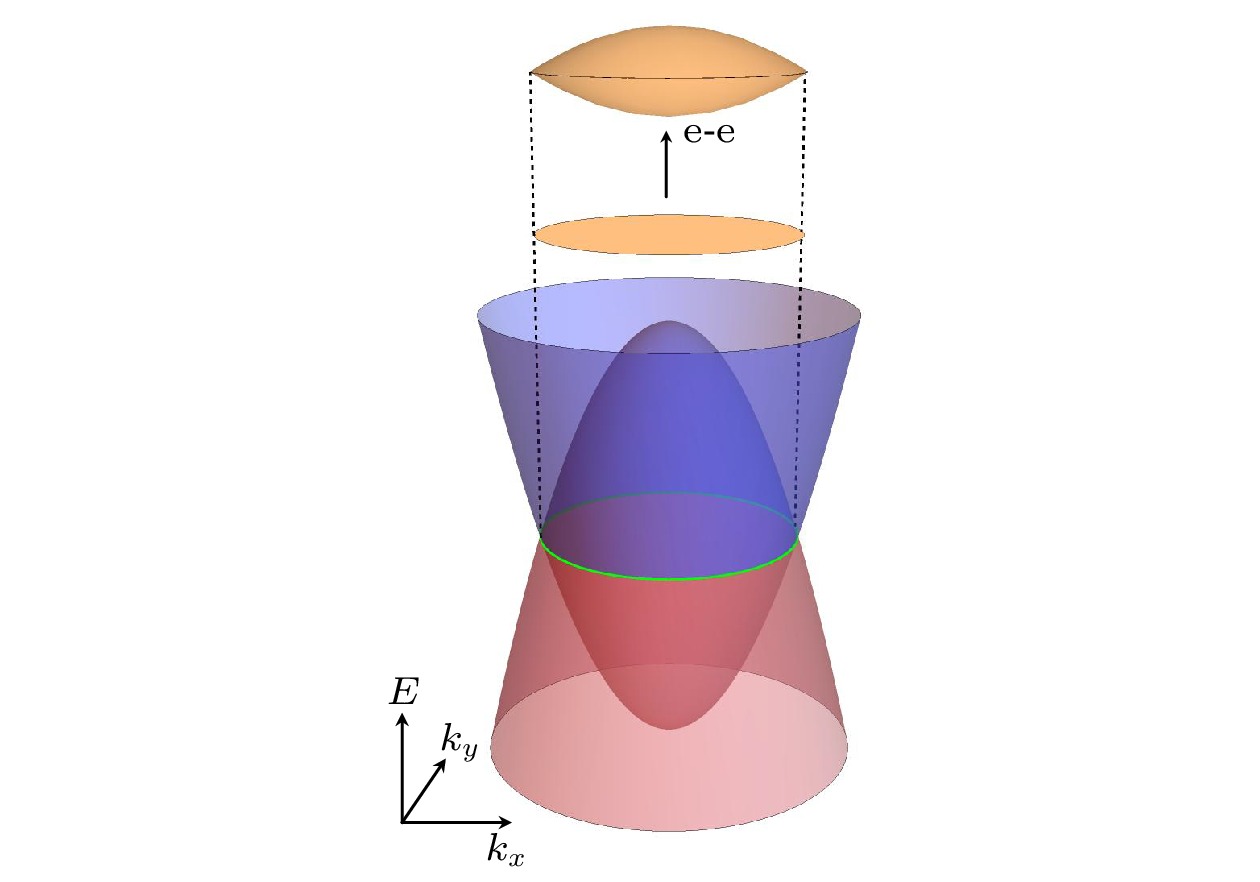
-
The topological nodal-line semimetal is characterized by the conduction band and valence band of electrons crossing along a one-dimensional line or closed loop in reciprocal space, with each nodal line carrying Π Berry phase. According to bulk-boundary correspondence, there exist drumheadlike surface states with weak dispersion at the boundary of system, surrounded by the projection of nodal loops onto the surface Brillouin zone. In most of nodal-line semimetals, the spin orbit coupling effect is weak, leading to the absence of a spin configuration for surface states under the single-particle picture. However, the featured weak dispersion of drumheadlike surface states enhances the electron-electron interaction effect, which triggers out ferromagnetic instability and causes spin splitting in the surface state. In this work, spin-dependent scattering caused by ferromagnetic surface states in spin-degenerate nodal-line semimetals is considered. It is found that both spin-splitting drumheadlike surface states can lead to resonant spin-flipped reflection. This physical process is reflected in a double-peak structure in the spin conductance spectrum. Specifically, we deal with the scattering problem induced by surface states in normal metal and nodal-line semimetal heterojunctions by using the scattering matrix and the Green’s functions theory, respectively, and obtain consistent conclusions. The result points out that spin-degenerate nodal-line semimetal surface states can still lead to spin-dependent transport, which provides a new perspective for the detection and potential application of spintronics in nodal-line semimetals.
[1] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[2] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[3] 初纯光, 王安琦, 廖志敏 2023 72 087401
Google Scholar
Chu C G, Wang A Q, Liao Z M 2023 Acta Phys. Sin. 72 087401
Google Scholar
[4] Klitzing K V, Dorda G, Pepper M 1980 Phys. Rev. Lett. 45 494
Google Scholar
[5] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[6] Halperin B I 1982 Phys. Rev. B 25 2185
Google Scholar
[7] 耿逸飞, 王铸宁, 马耀光, 高飞 2019 68 224101
Google Scholar
Geng Y F, Wang Z N, Ma Y G, Gao F 2019 Acta Phys. Sin. 68 224101
Google Scholar
[8] Xue S W, Wang M Y, Zhang S Y, Jia X, Zhou J H, Shi Y G, Zhu X T, Yao Y G, Guo J D 2021 Phys. Rev. Lett. 127 186802
Google Scholar
[9] Weng H M, Dai X, Fang Z 2016 J. Phys. Condens. Matter 28 303001
Google Scholar
[10] Murakami S 2007 New J. Phys. 9 356
Google Scholar
[11] Wan X G, Turner A M, Vishwanath A, Savrasov S Y 2011 Phys. Rev. B 83 205101
Google Scholar
[12] Weng H M, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029
Google Scholar
[13] Burkov A A, Hook M D, Balents L 2011 Phys. Rev. B 84 235126
Google Scholar
[14] Weng H M, Liang Y Y, Xu Q N, Fang Z, Dai X, Kawazoe Y 2015 Phys. Rev. B 92 045108
Google Scholar
[15] Bian G, Chang T R, Sankar R, Xv S Y, Zheng H, Neupert T, Chiu C K, Huang S M, Chang G Q, Belopolski I, Sanchez D S, Neupane M, Alidoust N, Liu C, Wang B K, Lee C C, Jeng H T, Zhang C L, Yuan Z J, Jia S, Bansil A, Chou F C, Lin H, Hasan M Z 2016 Nat. Commun. 7 10556
Google Scholar
[16] Schoop L M, Ali M N, Straber C, Andreas T, Varykhalov A, Marchenko D, Duppel V, Parkin S S, Lotsch B V, Ast C R 2016 Nat. Commun. 7 11696
Google Scholar
[17] Takane D, Wang Z W, Souma S, Nakayama K, Trang C X, Sato T, Takahashi T, Ando Y 2016 Phys. Rev. B 94 121108(R
Google Scholar
[18] Hu J, Tang Z J, Liu J Y, Liu X, Zhu Y L, Graf D, Myhro K, Tran S, Lau C N, Wei J, Mao Z Q 2016 Phys. Rev. Lett. 117 016602
Google Scholar
[19] Kumar N, Manna K, Qi Y P, Wu S C, Wang L, Yan B H, Felser C, Shekhar C 2017 Phys. Rev. B 95 121109(R
Google Scholar
[20] Chen W, Luo K, Li L, Zilberberg O 2018 Phys. Rev. Lett. 121 166802
Google Scholar
[21] Liu J P, Balents L 2017 Phys. Rev. B 95 075426
Google Scholar
[22] Song C Y, Liu L L, Cui S T, Gao J J, Song P B, Jin L, Zhao W J, Sun Z, Zhang X M, Zhao L, Luo X, Sun Y P, Shi Y G, Zhang H J, Liu G D, Zhou X J 2023 Phys. Rev. B 107 045142
Google Scholar
[23] Chan Y H, Chiu C K, Chou M Y, Schnyder A P 2016 Phys. Rev. B 93 205132
Google Scholar
[24] Ryu S, Hatsugai Y 2002 Phys. Rev. Lett. 89 077002
Google Scholar
[25] Hirayama M, Okugawa R, Miyake T, Murakami S 2017 Nat. Commun. 8 14022
Google Scholar
[26] Mahan G D 2000 Many-Particle Physics (New York: Kluwer Academic/Plenum Publishers) p414
[27] Chen W, Lado J L 2019 Phys. Rev. Lett. 122 016803
Google Scholar
[28] BenDaniel D J, Duke C B 1966 Phys. Rev. 152 683
Google Scholar
[29] Zulicke U, Schroll C 2002 Phys. Rev. Lett. 88 029701
Google Scholar
[30] Ryndyk D A 2016 Theory of Quantum Transport at Nanoscale: An Introduction (Springer International, Cham) p90
[31] Datta S 1995 Electronic Transport in Mesoscopic System (Cambridge: Cambridge University Press) P239
-
图 1 节线半金属鼓面态自旋劈裂示意图. 绿色实线表示节线环, 饼型圆盘表示劈裂后的鼓面态色散关系
Figure 1. Schematic diagram for the spin splitting of the drumheadlike surface states. The green solid line represents the nodal loop, and the pie-shaped disk represents the dispersion relationship of the spin-splitting drumheadlike surface states
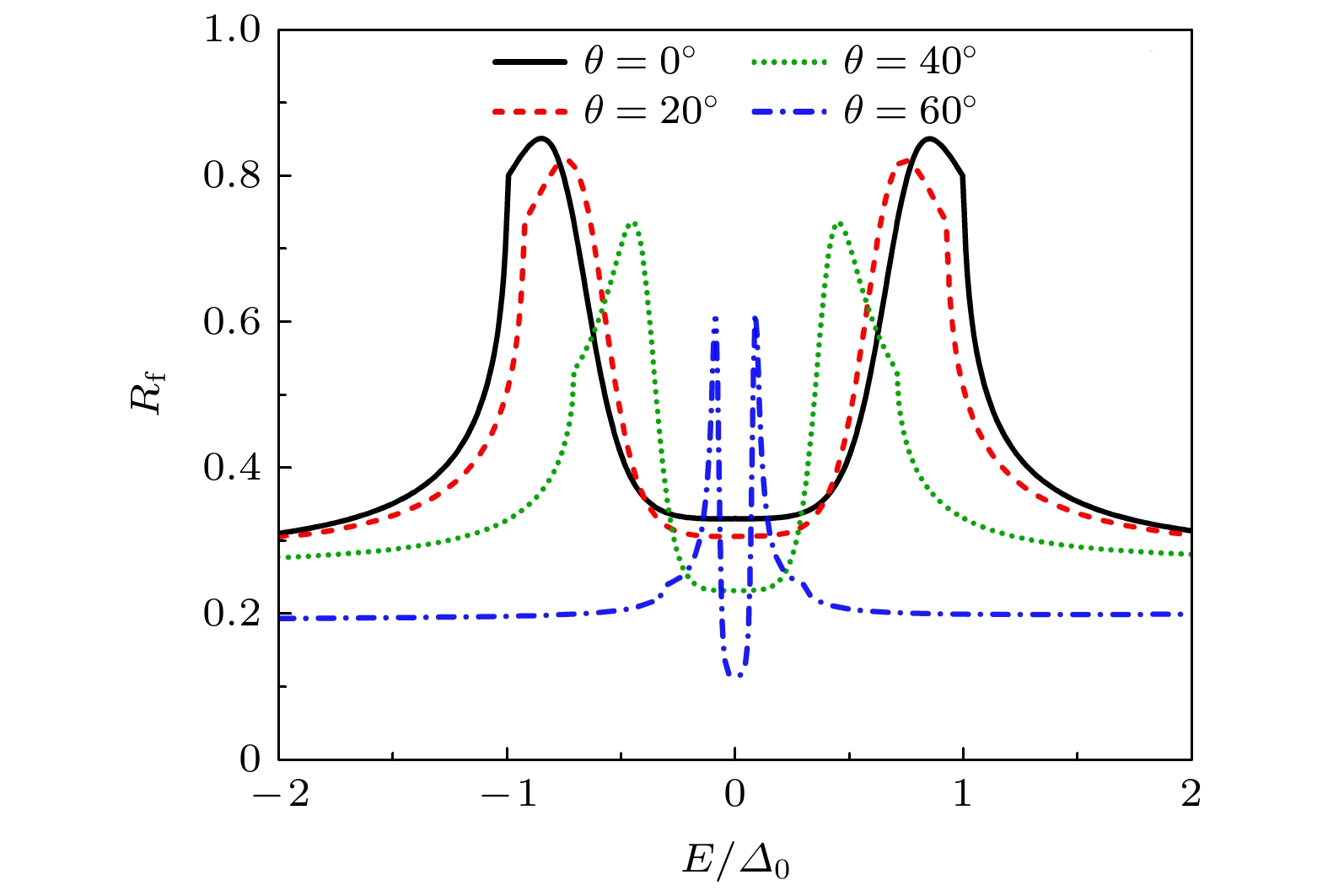
图 2 电子自旋翻转反射与能量的依赖关系. θ 为入射电子与z方向夹角,
$\varDelta_0=\varDelta({\boldsymbol{k}}_{/ /}=0)$ 是电子垂直入射时的有效能隙. 相关参数取$ B=C=1\; {\rm{eV}}{\cdot} {\rm{nm}}^2 $ ,$ \lambda=0.01\; {\rm{eV}}{\cdot} {\rm{nm}} $ ,$|{\boldsymbol{k}}_{\rm{F}}|=1.1 k_0=1.54\; {\rm{nm}}^{-1}$ ,$ D=3\; {\rm{eV}} $ Figure 2. Probability of electron spin-flipped reflection
$ R_{\rm{f }}$ vs. E for different angles of incidence θ (relative to z axis).$\varDelta_0$ is the effective energy gap when θ is zero. Relevant parameters take:$ B=C=1\; {\rm{eV}}{\cdot}{\rm{ nm}}^2 $ ,$ \lambda=0.01\; {\rm{eV}}{\cdot}{\rm{nm}} $ ,$ |{\boldsymbol{k}}_{\rm{F}}|= $ $ 1.1 k_0=1.54\;{\rm{ nm}}^{-1} $ ,$ D=3\;{\rm{ eV}} $ 
图 3 隧穿哈密顿量方法求得的自旋翻转反射系数. 相关参数取
$ E_-=-E_+=8\; {\rm{meV}} $ ,$ \varGamma=0.5 $ ,$ 3.0 $ ,$ 5.5\, {\rm{meV}} $ Figure 3. Probability of spin-flipped reflection solved by Green's function as a function of energy with the following parameters:
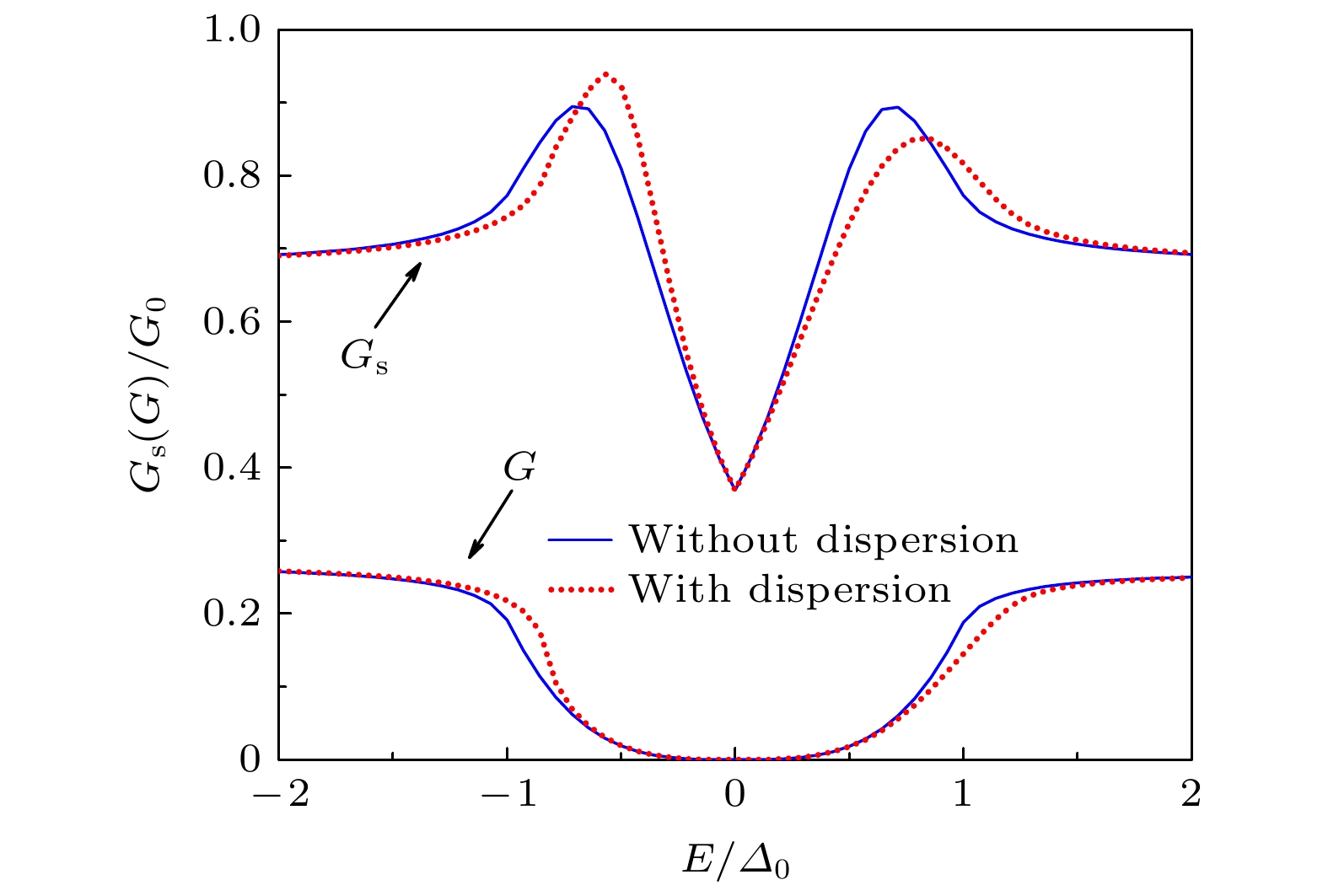
$ E_- = -E_+ = 8\; {\rm{meV}} $ ,$\varGamma = 0.5$ ,$ 3.0 $ ,$ 5.5\; {\rm{meV}} $ 
图 4 自旋电导、电荷电导与能量的依赖关系. 实线表示鼓面态无色散, 虚线表示鼓面态存在色散. 相关参数取
$ B=C= $ $ 1\; {\rm{eV}}{\cdot} {\rm{nm}}^2 $ ,$ \lambda=0.01\; {\rm{eV}}{\cdot}{\rm{ nm}} $ ,$ |{\boldsymbol{k}}_{\rm{F}}|=1.1 k_0=1.54\; {\rm{nm}}^{-1} $ ,$ D= $ $ 3\; {\rm{eV}} $ ,$ a=2\; {\rm{meV}}{\cdot} {\rm{nm}}^2 $ Figure 4. Spin (charge) conductance as a function of energy with (without) drumheadlike surface states dispersion. Relevant parameters take:
$ B=C=1\;{\rm{ eV}}{\cdot }{\rm{nm}}^2 $ ,$ \lambda=0.01\; {\rm{eV}}{\cdot }{\rm{nm}} $ ,$ |{\boldsymbol{k}}_{\rm{F}}|=1.1 k_0=1.54\; {\rm{nm}}^{-1} $ ,$ D=3\; {\rm{eV}} $ ,$ a=2\; {\rm{meV}}{\cdot} {\rm{nm}}^2 $ -
[1] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[2] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[3] 初纯光, 王安琦, 廖志敏 2023 72 087401
Google Scholar
Chu C G, Wang A Q, Liao Z M 2023 Acta Phys. Sin. 72 087401
Google Scholar
[4] Klitzing K V, Dorda G, Pepper M 1980 Phys. Rev. Lett. 45 494
Google Scholar
[5] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[6] Halperin B I 1982 Phys. Rev. B 25 2185
Google Scholar
[7] 耿逸飞, 王铸宁, 马耀光, 高飞 2019 68 224101
Google Scholar
Geng Y F, Wang Z N, Ma Y G, Gao F 2019 Acta Phys. Sin. 68 224101
Google Scholar
[8] Xue S W, Wang M Y, Zhang S Y, Jia X, Zhou J H, Shi Y G, Zhu X T, Yao Y G, Guo J D 2021 Phys. Rev. Lett. 127 186802
Google Scholar
[9] Weng H M, Dai X, Fang Z 2016 J. Phys. Condens. Matter 28 303001
Google Scholar
[10] Murakami S 2007 New J. Phys. 9 356
Google Scholar
[11] Wan X G, Turner A M, Vishwanath A, Savrasov S Y 2011 Phys. Rev. B 83 205101
Google Scholar
[12] Weng H M, Fang C, Fang Z, Bernevig B A, Dai X 2015 Phys. Rev. X 5 011029
Google Scholar
[13] Burkov A A, Hook M D, Balents L 2011 Phys. Rev. B 84 235126
Google Scholar
[14] Weng H M, Liang Y Y, Xu Q N, Fang Z, Dai X, Kawazoe Y 2015 Phys. Rev. B 92 045108
Google Scholar
[15] Bian G, Chang T R, Sankar R, Xv S Y, Zheng H, Neupert T, Chiu C K, Huang S M, Chang G Q, Belopolski I, Sanchez D S, Neupane M, Alidoust N, Liu C, Wang B K, Lee C C, Jeng H T, Zhang C L, Yuan Z J, Jia S, Bansil A, Chou F C, Lin H, Hasan M Z 2016 Nat. Commun. 7 10556
Google Scholar
[16] Schoop L M, Ali M N, Straber C, Andreas T, Varykhalov A, Marchenko D, Duppel V, Parkin S S, Lotsch B V, Ast C R 2016 Nat. Commun. 7 11696
Google Scholar
[17] Takane D, Wang Z W, Souma S, Nakayama K, Trang C X, Sato T, Takahashi T, Ando Y 2016 Phys. Rev. B 94 121108(R
Google Scholar
[18] Hu J, Tang Z J, Liu J Y, Liu X, Zhu Y L, Graf D, Myhro K, Tran S, Lau C N, Wei J, Mao Z Q 2016 Phys. Rev. Lett. 117 016602
Google Scholar
[19] Kumar N, Manna K, Qi Y P, Wu S C, Wang L, Yan B H, Felser C, Shekhar C 2017 Phys. Rev. B 95 121109(R
Google Scholar
[20] Chen W, Luo K, Li L, Zilberberg O 2018 Phys. Rev. Lett. 121 166802
Google Scholar
[21] Liu J P, Balents L 2017 Phys. Rev. B 95 075426
Google Scholar
[22] Song C Y, Liu L L, Cui S T, Gao J J, Song P B, Jin L, Zhao W J, Sun Z, Zhang X M, Zhao L, Luo X, Sun Y P, Shi Y G, Zhang H J, Liu G D, Zhou X J 2023 Phys. Rev. B 107 045142
Google Scholar
[23] Chan Y H, Chiu C K, Chou M Y, Schnyder A P 2016 Phys. Rev. B 93 205132
Google Scholar
[24] Ryu S, Hatsugai Y 2002 Phys. Rev. Lett. 89 077002
Google Scholar
[25] Hirayama M, Okugawa R, Miyake T, Murakami S 2017 Nat. Commun. 8 14022
Google Scholar
[26] Mahan G D 2000 Many-Particle Physics (New York: Kluwer Academic/Plenum Publishers) p414
[27] Chen W, Lado J L 2019 Phys. Rev. Lett. 122 016803
Google Scholar
[28] BenDaniel D J, Duke C B 1966 Phys. Rev. 152 683
Google Scholar
[29] Zulicke U, Schroll C 2002 Phys. Rev. Lett. 88 029701
Google Scholar
[30] Ryndyk D A 2016 Theory of Quantum Transport at Nanoscale: An Introduction (Springer International, Cham) p90
[31] Datta S 1995 Electronic Transport in Mesoscopic System (Cambridge: Cambridge University Press) P239
DownLoad:
Catalog
Metrics
- Abstract views: 2788
- PDF Downloads: 147
- Cited By: 0
