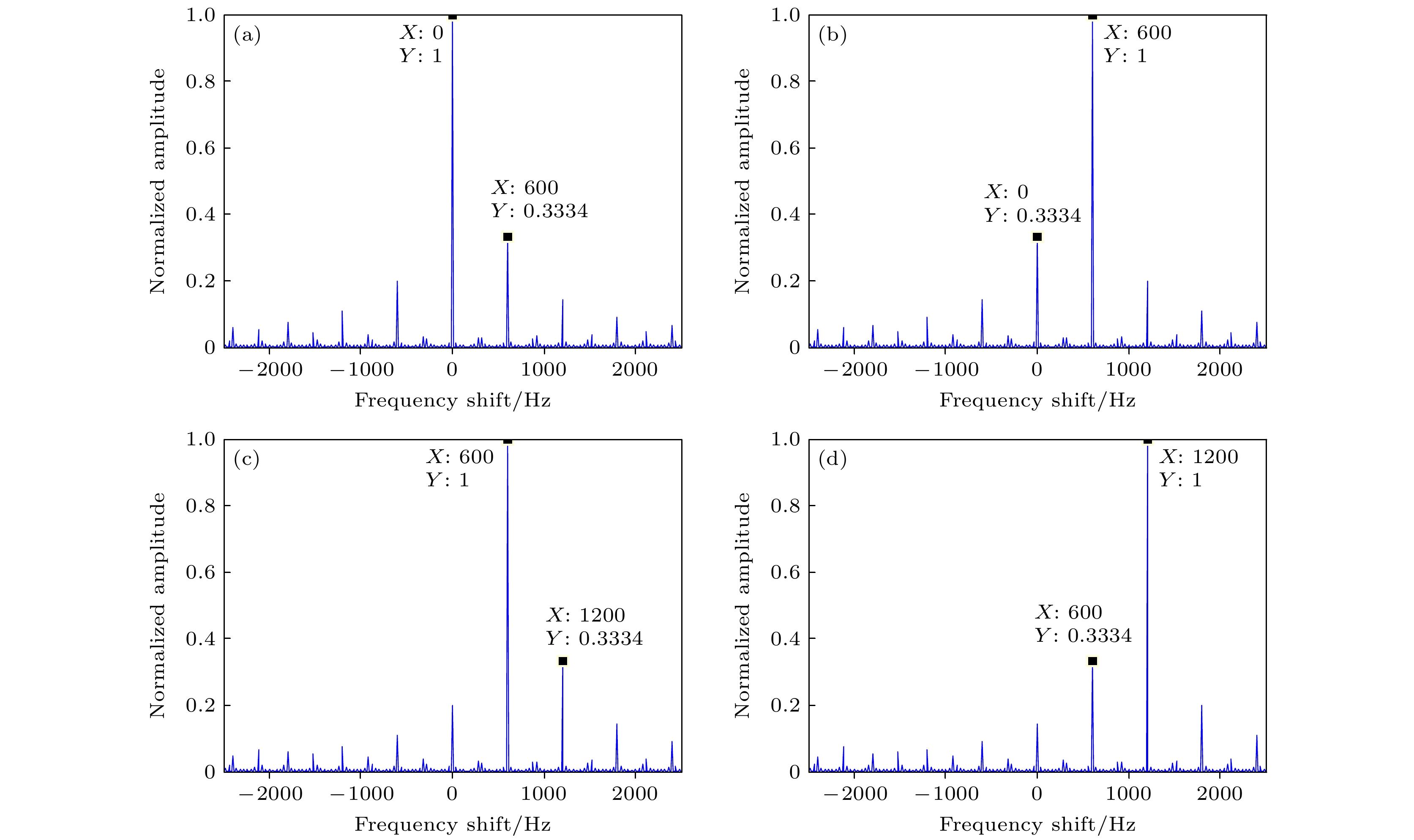
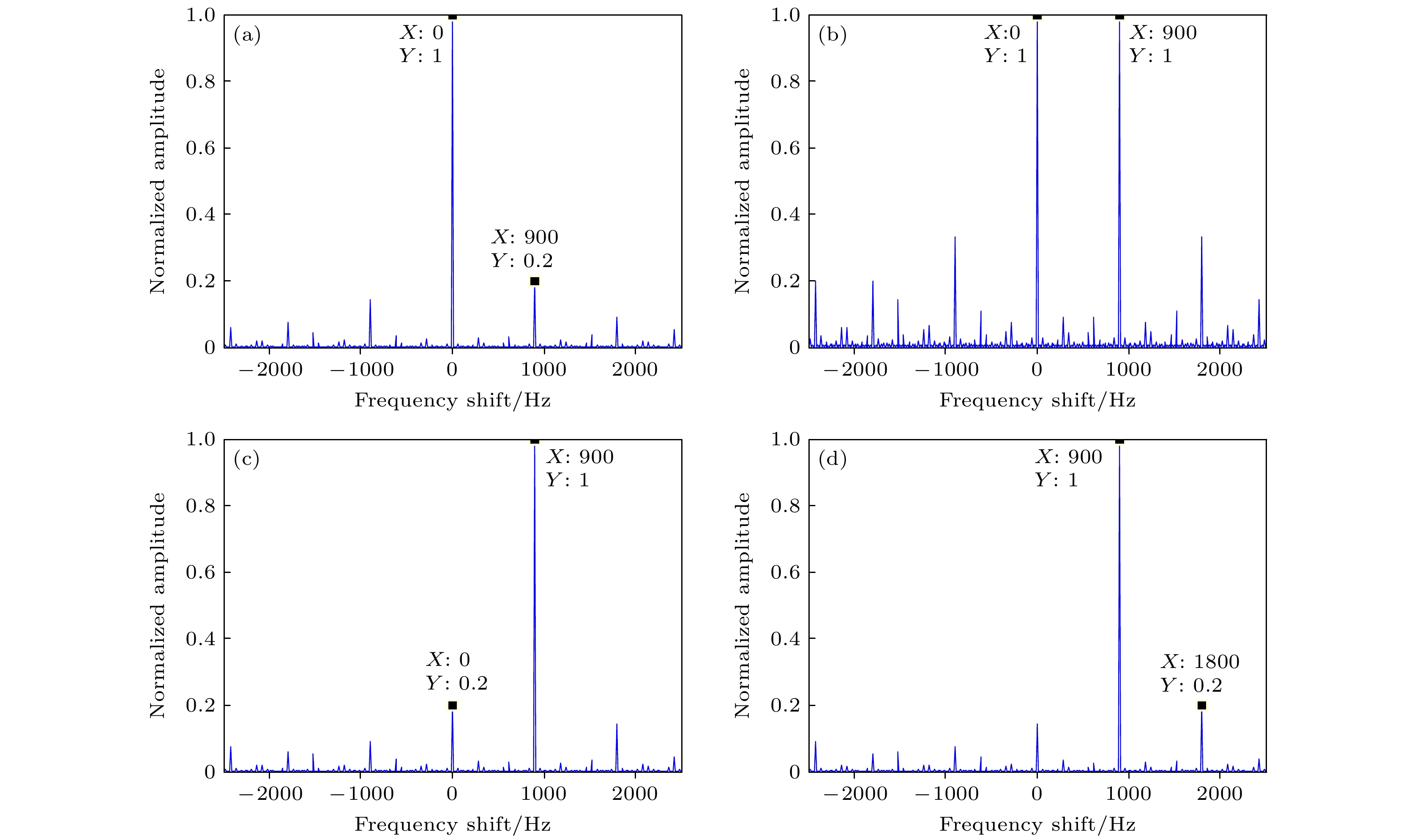
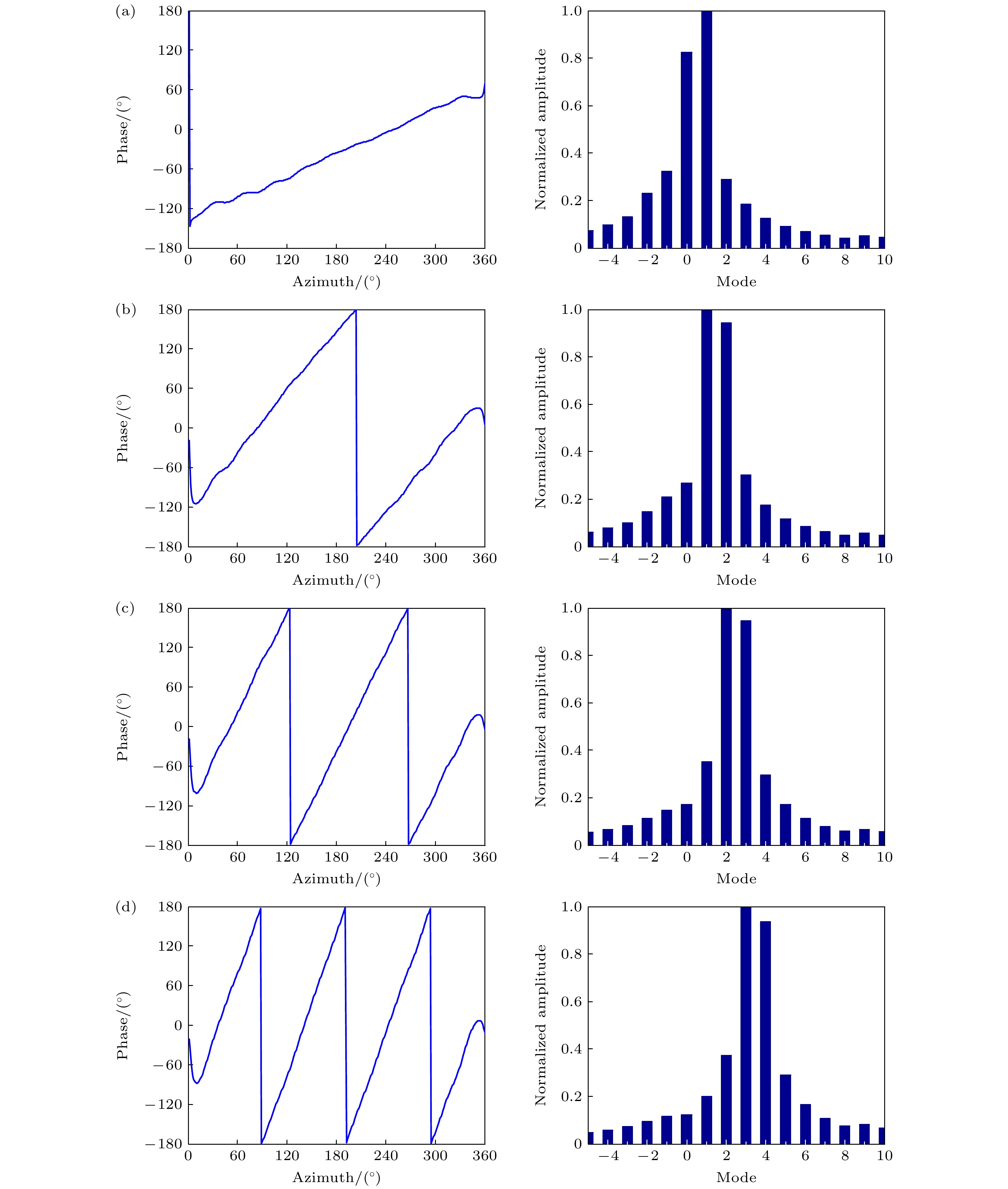
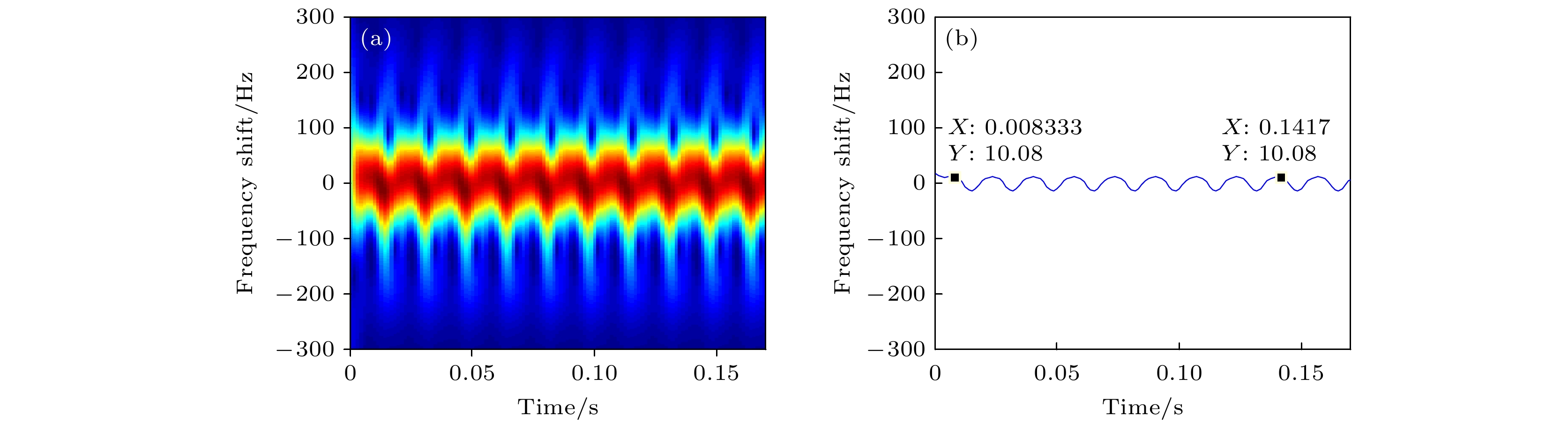
-
Rotational Doppler effect is an important phenomenon when the vortex electromagnetic wave carrying orbital angular momentum is used to detect a rotating target. Compared with the traditional plane wave case, rotational Doppler effect enables the vortex electromagnetic wave to detect the spin motion along the rotation axis of target. However, there are still some blind zones when the integer orbital angular momentum beams are used to detect specific spinning objects. To expand the application scope of detection scheme based on the rotational Doppler effect, according to the time-frequency analysis, in this paper we study the method of estimating the rotation speed of spinning objects under the normal incidence and oblique incidence of fractional orbital angular momentum beams. Firstly, based on the ideal scattering point model, the echo models are derived under the normal incidence and oblique incidence of integer orbital angular momentum beam and fractional orbital angular momentum beam, respectively, as well as theoretical time-frequency curves. Then taking the three-dimensional practical object for example, the echo under the incidence of fractional orbital angular momentum beams and its time-frequency graph are achieved by using the momentum method and short-time Fourier transform. The time-frequency ridge and its fluctuation period are extracted from the time-frequency graph, thereby estimating the spinning speed of target. The results show that the fractional orbital angular momentum beams can be used to estimate the rotation speed of spinning objects effectively, whether it is normal incidence or oblique incidence, thereby solving the problem about the detection blind zone of integer orbital angular momentum beams, and improving the applicability in detecting spin motion.
-
Keywords:
- rotational Doppler effect /
- fractional orbital angular momentum /
- oblique incidence /
- time-frequency analysis
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Courtial J, Dholakia K, Robertson D A, Allen L, Padgett M J 1998 Phys. Rev. Lett. 80 3217
Google Scholar
[3] Courtial J, Robertson D A, Dholakia K, Allen L, Padgett M J 1998 Phys. Rev. Lett. 81 4828
Google Scholar
[4] Mohammadi S M, Daldorff L K S, Bergman J E S, Karlsson R L, Thide B, Forozesh K, Carozzi T D, Isham B 2010 IEEE Trans. Antennas Propag. 58 565
Google Scholar
[5] Yin B H, He Z, Chen R S 2019 Appl. Comput. Electromagn. Soc. J. 34 1637
[6] Thide B, Then H, Sjoholm J, Palmer K, Bergman J, Carozzi, T D, Istomin Ya N, Ibragimov N H, Khamitova R 2007 Phys. Rev. Lett. 99 087701
Google Scholar
[7] Liu K, Cheng Y Q, Yang Z C, Wang H Q, Qin Y L, Li X 2015 IEEE Antennas Wirel. Propag. Lett. 14 711
Google Scholar
[8] Zhang C, Chen D, Jiang X F 2017 Sci. Rep. 7 15412
Google Scholar
[9] Zhao M Y, Gao X L, Xie M T, Zhai W S, Xu W J, Huang S G, Gu W Y 2016 Opt. Lett. 41 2549
Google Scholar
[10] Zheng J Y, Zheng S L, Shao Z L, Shao Z L, Zhang X M 2018 J. Appl. Phys. 124 164907
Google Scholar
[11] Gong T, Cheng Y Q, Li X, Chen D C 2018 IEEE Microwave Wireless Compon. Lett. 28 843
Google Scholar
[12] Zhou Z L, Cheng Y Q, Liu K, Wang H Q, Qin Y L 2019 IEEE Sens. Lett. 3 1
Google Scholar
[13] Yin B H, He Z, Chen R S 2022 IEEE Trans. Antennas Propag. 70 9971
Google Scholar
[14] Luo Y, Chen Y J, Zhu Y Z, Li W Y, Zhang Q 2019 IET Radar Sonar Navig. 14 2
Google Scholar
[15] 李瑞, 李开明, 张群, 梁佳, 罗迎 2021 电子与信息学报 43 547
Google Scholar
Li R, Li K M, Zhang Q, Liang J, Luo Y 2021 J. Electron. Inf. Technol. 43 547
Google Scholar
[16] 王煜, 刘康, 王建秋, 王宏强, 程永强 2021 雷达学报 10 740
Google Scholar
Wang Y, Liu K, Wang J Q, Wang H Q, Cheng Y Q 2021 J. Radars 10 740
Google Scholar
[17] Lavery M P J, Speirits F C, Barnett S M, Padgett M J 2013 Science 431 537
Google Scholar
[18] Zheng S L, Hui X N, Jin X F, Chi H, Zhang X M 2015 IEEE Trans. Antennas Propag. 63 1530
Google Scholar
[19] Berry M V 2004 J. Opt. A:Pure Appl. Opt. 6 259
Google Scholar
[20] Liu H Y, Wang Y, Wang J Q, Liu K, Wang H Q 2021 IEEE Antennas Wirel. Propag. Lett. 20 948
Google Scholar
-
图 1 波束轴指向目标旋转中心并垂直于旋转平面的理想入射情况
Figure 1. The ideal illumination with the beam axis pointing to the center of rotation and perpendicular to the plane of rotation.
图 2 DBoR散射中心分布情况
Figure 2. The distribution of scattering centers for the DBoR.
图 3 目标旋转平面的偏转
Figure 3. The deflection of the rotating plane.
图 4 回波频谱 (a) FFT频谱; (b) 理论时频曲线
Figure 4. The frequency spectrum of echo: (a) FFT spectrum; (b) theoretical time-frequency curve.
图 5 当
$N = 1$ 时的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 5. Echo frequency spectrums with
$N = 1$ : (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ .
图 6 当
$N = 2$ 时的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 6. Echo frequency spectrums with
$N = 2$ : (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ .
图 7 当
$N = 3$ 时的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 7. Echo frequency spectrums with
$N = 3$ and: (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ .
图 8 目标旋转平面偏转时的非理想入射情况
Figure 8. The unideal illumination with the deflection of the rotating plane.
图 9 当
$N = 3$ 时, 旋转平面偏转后的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 9. Echo frequency spectrums after the tilt of rotation plane with
$N = 3$ : (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ 
图 10 旋转平面偏转后模式1.5回波时频图及其局部放大
Figure 10. Echo frequency time-frequency graphs of mode 1.5 after the tilt of rotation plane and its local zoom
图 11 旋转平面偏转后模式2.5回波时频图及其局部放大
Figure 11. Echo frequency time-frequency graphs of mode 2.5 after the tilt of rotation plane and its local zoom
图 12 风速仪模型
Figure 12. The anemoscope model.
图 13 辐射电场的相位梯度以及模式谱 (a)
$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 13. Phase gradients and mode spectrums of the radiation electric field with: (a)
$l = 0.5$ (b)$l = 1.5$ (c)$l = 2.5$ (d)$l = 3.5$ .
图 14 当
$N = 3$ 时的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 14. Echo frequency spectrums with
$N = 3$ : (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ 
图 15 当
$N = 3$ 时, 旋转平面偏转后的回波频谱 (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ Figure 15. Echo frequency spectrums after the tilt of rotation plane with
$N = 3$ : (a)$l = 0.5$ ; (b)$l = 1.5$ ; (c)$l = 2.5$ ; (d)$l = 3.5$ 
图 16 模式0.5波束正入射目标时的时频图及时频曲线 (a) 时频图; (b) 时频曲线
Figure 16. Time-frequency graph and curve under the normal incidence of the beam with mode 0.5: (a) Time-frequency map; (b) time-frequency curve
图 17 模式2.5波束斜入射目标时(旋转平面绕Y轴倾斜5°)的时频图及时频曲线 (a) 时频图; (b) 时频曲线
Figure 17. Time-frequency graph and curve under the oblique incidence (the plane of rotation tilts around Y axis with 5°) of the beam with mode 2.5: (a) Time-frequency map; (b) time-frequency curve
-
[1] Allen L, Beijersbergen M W, Spreeuw R J C, Woerdman J P 1992 Phys. Rev. A 45 8185
Google Scholar
[2] Courtial J, Dholakia K, Robertson D A, Allen L, Padgett M J 1998 Phys. Rev. Lett. 80 3217
Google Scholar
[3] Courtial J, Robertson D A, Dholakia K, Allen L, Padgett M J 1998 Phys. Rev. Lett. 81 4828
Google Scholar
[4] Mohammadi S M, Daldorff L K S, Bergman J E S, Karlsson R L, Thide B, Forozesh K, Carozzi T D, Isham B 2010 IEEE Trans. Antennas Propag. 58 565
Google Scholar
[5] Yin B H, He Z, Chen R S 2019 Appl. Comput. Electromagn. Soc. J. 34 1637
[6] Thide B, Then H, Sjoholm J, Palmer K, Bergman J, Carozzi, T D, Istomin Ya N, Ibragimov N H, Khamitova R 2007 Phys. Rev. Lett. 99 087701
Google Scholar
[7] Liu K, Cheng Y Q, Yang Z C, Wang H Q, Qin Y L, Li X 2015 IEEE Antennas Wirel. Propag. Lett. 14 711
Google Scholar
[8] Zhang C, Chen D, Jiang X F 2017 Sci. Rep. 7 15412
Google Scholar
[9] Zhao M Y, Gao X L, Xie M T, Zhai W S, Xu W J, Huang S G, Gu W Y 2016 Opt. Lett. 41 2549
Google Scholar
[10] Zheng J Y, Zheng S L, Shao Z L, Shao Z L, Zhang X M 2018 J. Appl. Phys. 124 164907
Google Scholar
[11] Gong T, Cheng Y Q, Li X, Chen D C 2018 IEEE Microwave Wireless Compon. Lett. 28 843
Google Scholar
[12] Zhou Z L, Cheng Y Q, Liu K, Wang H Q, Qin Y L 2019 IEEE Sens. Lett. 3 1
Google Scholar
[13] Yin B H, He Z, Chen R S 2022 IEEE Trans. Antennas Propag. 70 9971
Google Scholar
[14] Luo Y, Chen Y J, Zhu Y Z, Li W Y, Zhang Q 2019 IET Radar Sonar Navig. 14 2
Google Scholar
[15] 李瑞, 李开明, 张群, 梁佳, 罗迎 2021 电子与信息学报 43 547
Google Scholar
Li R, Li K M, Zhang Q, Liang J, Luo Y 2021 J. Electron. Inf. Technol. 43 547
Google Scholar
[16] 王煜, 刘康, 王建秋, 王宏强, 程永强 2021 雷达学报 10 740
Google Scholar
Wang Y, Liu K, Wang J Q, Wang H Q, Cheng Y Q 2021 J. Radars 10 740
Google Scholar
[17] Lavery M P J, Speirits F C, Barnett S M, Padgett M J 2013 Science 431 537
Google Scholar
[18] Zheng S L, Hui X N, Jin X F, Chi H, Zhang X M 2015 IEEE Trans. Antennas Propag. 63 1530
Google Scholar
[19] Berry M V 2004 J. Opt. A:Pure Appl. Opt. 6 259
Google Scholar
[20] Liu H Y, Wang Y, Wang J Q, Liu K, Wang H Q 2021 IEEE Antennas Wirel. Propag. Lett. 20 948
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 3191
- PDF Downloads: 86
- Cited By: 0
