-
Parity-time (PT) symmetric is not a necessary condition for achieving a real spectrum and some studies about realizing real spectra in non-PT-symmetric systems with arbitrary gain–loss profiles have been presented recently. By tuning the free parameters in non-PT-symmetric potentials, phase transition could also be induced. Above phase transition point, discrete complex eigenvalues bifurcate out from continuous real eigenvalues in the interior of the continuous spectrum. In this work, we investgate the existence and stability of solitons in nonlocal nonlinear couplers with non-PT-symmetric complex potentials both below and above phase transition. There are several discrete eigenvalues in the linear spectra of the non-PT-symmetric system used here. With the square-operator iteration method, we find that different continuous families of solitions can bifurcate from different discrete linear eigenvalues. Moreover, linear-stability analysis collaborated with direct numerical propagation simulations demonstrates that the nonlocal solitions can be stable in a range of parameter values. we first address the cases below the phase transition. To be specific, when we fix the coupling coefficient and vary the degree of nonlocality, it’s found that fundamental solitons, dipole solitons, tripolar solitons, quadrupole solitons bifurcate from the largest,the second-largest, the third-largest and the fifth-largest discrete eigenvalue, respectively. These nonlocal solitons are all stable in the low power region. With an increase of the degree of nonlocality, the stability region shrinks for the fundamental solitons while it widens for the dipole and multiplole solitons. At the same time, the power of all the stable solitons increases with the increase of the degree of nonlocality. By varying the coupling coefficient, the arrangement of soliton families emerging in the discrete interval of the linear spectrum can be changed. For example, the dipole solitons bifurcate from the third-or fourth-largest discrete eigenvalue while the tripolar solitons bifurcate from the fifth largest discrete eigenvalue. Above phase transition,the fundamental solitons are unstable in the low and high power region but are stable in the moderate power region. The stability region shrinks with the increasing degree of nonlocality. We also find the family of dipole solitons bifurcates from the second-largest discrete eigenvalue, but all the dipole solitons are unstable. In addition, we find that the eigenvalues in linear-stability spectra of solitons emerge as conjugation pairs.
-
Keywords:
- non-parity-time-symmetry /
- nonlocal /
- nonlinear coupler /
- stability
[1] Jensen S M 1982 IEEE J. Quantum Electron. 18 1580
Google Scholar
[2] Trillo S, Wabnitz S, Wright E M, Stegeman G I 1988 Opt. Lett. 13 672
Google Scholar
[3] Abdullaev F K, Abrarov R M, Darmanyan S A 1989 Opt. Lett. 14 131
Google Scholar
[4] Paré C, Florjańczyk M 1990 Phys. Rev. A 41 6287
Google Scholar
[5] Mak W C K, Malomed B A, Chu P L 2004 Phys. Rev. E 69 066610
Google Scholar
[6] Herring G, Kevrekidis P G, Malomed B A, Carretero-González R, Frantzeskakis D J 2007 Phys. Rev. E 76 066606
Google Scholar
[7] Snyder A W, Mitchell D J 1997 Science 276 1538
Google Scholar
[8] Królikowski W, Bang O, Nikolov N I, Neshev D, Wyller J, Rasmussen J J, Edmundson D 2004 J. Opt. B: Quantum Semiclass. Opt. 6 S288
Google Scholar
[9] Peccianti M, Brzdakiewicz K A, Assanto G 2002 Opt. Lett. 27 1460
Google Scholar
[10] Pérez-García V M, Konotop V V, García-Ripoll J J 2000 Phys. Rev. E 62 4300
Google Scholar
[11] Bang O, Krolikowski W, Wyller J, Rasmussen J J 2002 Phys. Rev. E 66 046619
Google Scholar
[12] Ye F W, Dong L W, Hu B 2009 Opt. Lett. 34 584
Google Scholar
[13] Chen W, Wang Q, Shi J L, Shen M 2017 Opt. Commun. 403 22
Google Scholar
[14] Guo J L, Yang Z J, Song L M, Pang Z G 2020 Nonlinear Dynam. 101 1147
Google Scholar
[15] Guo J L, Yang Z J, Li X L, Zhang S M 2022 Chin. Phys. B 31 014203
Google Scholar
[16] Conti C, Peccianti M, Assanto G 2003 Phys. Rev. Lett. 91 073901
Google Scholar
[17] Peccianti M, Conti C, Assanto G 2005 Opt. Lett. 30 415
Google Scholar
[18] Rotschild C, Cohen O, Manela O, Segev M, Carmon T 2005 Phys. Rev. Lett. 95 213904
Google Scholar
[19] Królikowski W, Saffman M, Luther-Davies B, Denz C 1998 Phys. Rev. Lett. 80 3240
Google Scholar
[20] Mamaev A V, Zozulya A A, Mezentsev V K, Anderson D Z, Saffman M 1997 Phys. Rev. A 56 R1110
Google Scholar
[21] Wand M, Schindlmayr A, Meier T, Förstner J 2011 Phys. Status Solidi B 248 887
Google Scholar
[22] Dang Y L, Li H J, Lin J 2017 Nonlinear Dynam. 88 489
Google Scholar
[23] Gao Z J, Dang Y L, Lin J 2018 Opt. Commun. 426 302
Google Scholar
[24] 李森清, 张肖, 林机 2022 71 234207
Google Scholar
Li S Q, Zhang X, Lin J 2022 Acta Phys Sin. 71 234207
Google Scholar
[25] Wang Q, Mihalache D, Belic M, Zeng L W, Lin J 2023 Opt. Lett. 48 747
Google Scholar
[26] Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
Google Scholar
[27] Wang H, Wang J D 2011 Opt. Express 19 4030
Google Scholar
[28] Abdullaev F K, Kartashov Y K, Konotop V V, Zezyulin D A 2011 Phys. Rev. A 83 041805
Google Scholar
[29] Nixon S, Ge L J, Yang J K 2012 Phys. Rev. A 85 023822
Google Scholar
[30] Cannata F, Junker G, Trost J 1998 Phys. Lett. A 246 219
Google Scholar
[31] Miri M A, Heinrich M, Christodoulides D N 2013 Phys. Rev. A 87 043819
Google Scholar
[32] Tsoy E N, Allayarov I M, Abdullaev F K 2014 Opt. Lett. 39 4215
Google Scholar
[33] Konotop V V, Zezyulin D A 2014 Opt. Lett. 39 5535
Google Scholar
[34] Nixon S, Yang J K 2016 Stud. Appl. Math. 136 459
Google Scholar
[35] Yang J K, Nixon S 2016 Phys. Lett. A 380 3803
Google Scholar
[36] Zhu X, He Y J 2018 Opt. Express 26 26511
Google Scholar
[37] Zhu X, Peng X, Qiu Y L, Wang H C, He Y J 2020 New J. Phys. 22 033035
Google Scholar
[38] Yang J K 2021 Stud. Appl. Math. 147 4
Google Scholar
[39] Zhu X, Liao S W, Cai Z, Qiu Y L, He Y J 2021 Chaos Soliton.s Fract. 146 110837
Google Scholar
[40] Zhu X, Cai Z, Liu J L, Liao S W, He Y J 2022 Nonlinear Dynam. 108 2563
Google Scholar
-
图 1 (a)
$ \gamma=0.3 $ 对应的非PT对称复数势, 蓝色为实部, 红色为虚部; (b)$ \gamma=0.3 $ ,$ a_{1}=1 $ ,$ a_{2}=3 $ 对应的线性谱; (c)$ \gamma=-0.3 $ 对应的非PT对称复数势; (d)$ \gamma=-0.3 $ ,$ a_{1}=1 $ ,$ a_{2}=3 $ 对应的线性谱Figure 1. (a) Non-PT-symmetric complex potentials for
$ \gamma=0.3 $ (blue is the real part, and red is the imaginary part); (b) the linear spectrum at$ \gamma=0.3 $ ,$ a_{1}=1 $ ,$ a_{2}=3 $ ; (c) the non-PT-symmetric complex potentials for$ \gamma=-0.3 $ ; (d) the linear spectrum at$ \gamma=-0.3 $ ,$ a_{1}=1 $ ,$ a_{2}=3 $ .
图 2 (a), (b) 分别为u和v分量在
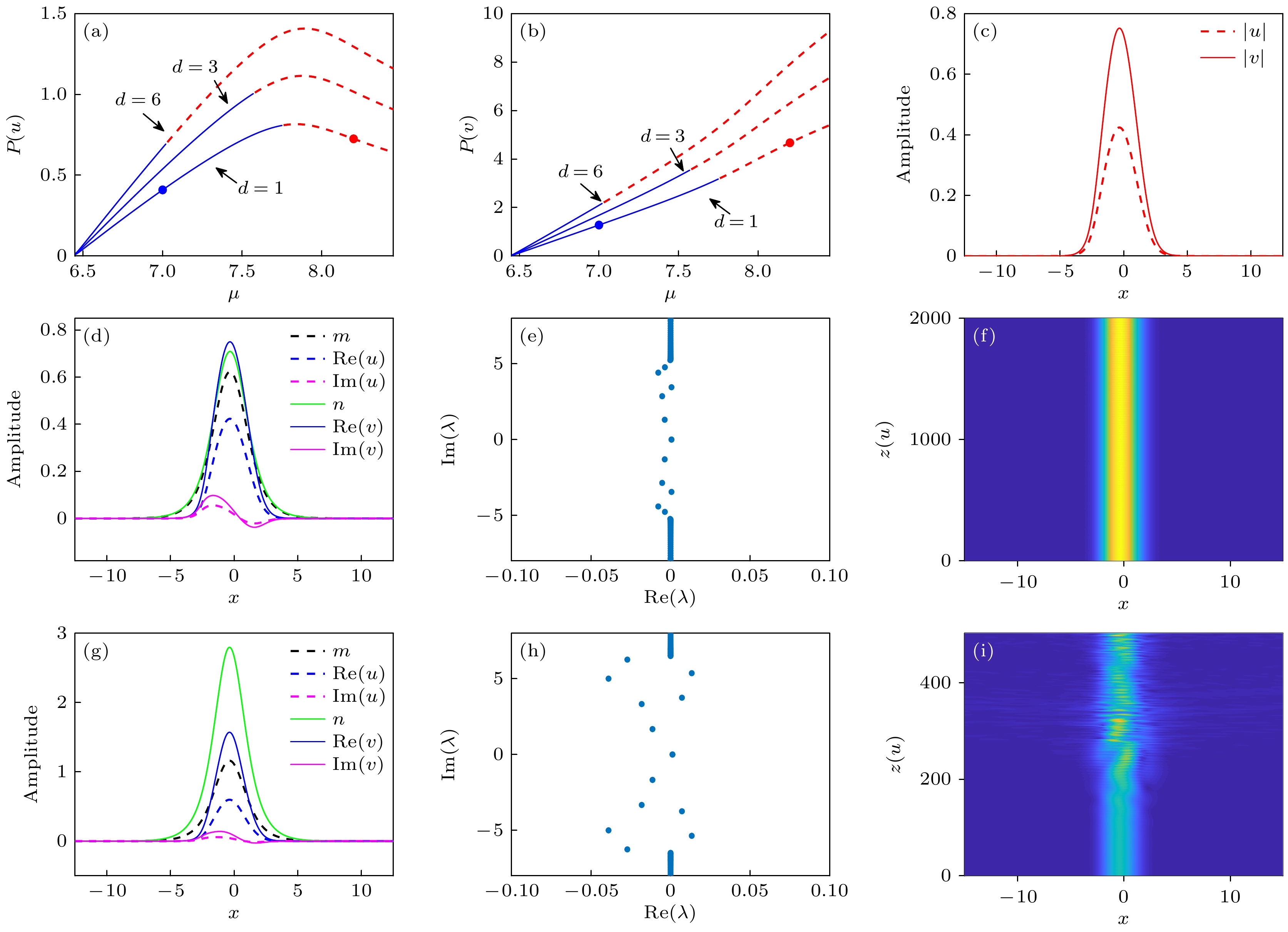
$ \mu > \mu_{1} $ 这一区间对应的功率曲线图, 蓝色实线是稳定区域, 红色虚线为不稳定区域; (c)蓝色加粗点$ \mu=7 $ 时两分量的模; (d)蓝色加粗点$ \mu=7 $ 时u, v, m, n的轮廓图; (e)$ \mu=7 $ 时的稳定性分析; (f)$ \mu=7 $ 时加了1%白噪声后u分量的传输; (g)—(i)分别为红色加粗点处$ \mu=8.2 $ 时对应的图. 其他参数的选取为$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ Figure 2. (a), (b) Power diagrams corresponding to the u and v components in the interval of
$ \mu > \mu_{1} $ , respectively (solid blue is the stable region, and dashed red line is the unstable region); (c) the modulus of u and v when$ \mu=7 $ (blue bold dot); (d) the contours of the soliton and refractive index at$ \mu=7 $ ; (e) stability analysis when$ \mu=7 $ ; (f) the stable propagation of the u component after adding 1% random noise when$ \mu=7 $ ; (g)–(i) the corresponding diagrams when$ \mu=8.2 $ (red bold dot), respectively. The other parameters are$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ .
图 3 (a), (b) 分别为u和v分量在
$ \mu_{2} < \mu < \mu_{1} $ 这一区间对应的功率曲线图; (c)蓝色加粗点$ \mu=5.4 $ ,$ d=6 $ 时两分量的模; (d)蓝色加粗点$ \mu=5.4 $ ,$ d=6 $ 时u, v, m, n的轮廓图; (e)$ \mu=5.4 $ ,$ d=6 $ 时的稳定性分析; (f)$ \mu=5.4 $ ,$ d=6 $ 时加了1%白噪声后u分量的传输; (g)—(i)分别为红色加粗点处$ \mu=5.8 $ ,$ d=1 $ 时对应的图. 其他参数的选取为$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ Figure 3. (a), (b) Power diagrams corresponding to the u and v components in the interval of
$ \mu_{2} < \mu < \mu_{1} $ ; (c) the modulus of u and v when$ \mu=5.4 $ and$ d=6 $ (blue bold dot); (d) the contours of the soliton and refractive index at$ \mu=5.4 $ and$ d=6 $ ; (e) stability analysis when$ \mu=5.4 $ and$ d=6 $ ; (f) the stable propagation of the u component after adding 1% random noise when$ \mu=5.4 $ and$ d=6 $ ; (g)–(i) the corresponding diagrams when$ \mu=5.8 $ and$ d=1 $ (red bold dot), respectively. The other parameters are$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ .
图 4 (a), (b) 分别为u和v分量在
$ \mu_{3} < \mu < \mu_{2} $ 这一区间对应的功率曲线图; (c)蓝色加粗点$ \mu=3.9 $ ,$ d=6 $ 时两分量的模; (d)蓝色加粗点$ \mu=3.9 $ ,$ d=6 $ 时u, v, m, n的轮廓图; (e)$ \mu=3.9 $ ,$ d=6 $ 时的稳定性分析; (f)$ \mu=3.9 $ ,$ d=6 $ 时加了1%白噪声后u分量的传输; (g)—(i)分别为红色加粗点处$ \mu=4.3 $ ,$ d=1 $ 时对应的图. 其他参数的选取为$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ Figure 4. (a), (b) Power diagrams corresponding to the u and v components in the interval of
$ \mu_{3} < \mu < \mu_{2} $ ; (c) the modulus of u and v when$ \mu=3.9 $ and$ d=6 $ (blue bold dot); (d) the contours of the soliton and refractive index at$ \mu=3.9 $ and$ d=6 $ ; (e) stability analysis when$ \mu=3.9 $ and$ d=6 $ ; (f) the stable propagation of the u component after adding 1% random noise when$ \mu=3.9 $ and$ d=6 $ ; (g)–(i) the corresponding diagrams when$ \mu=4.3 $ and$ d=1 $ (red bold dot), respectively. The other parameters are$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ .
图 5 (a), (b) 分别为u和v分量在
$ \mu_{4} < \mu < \mu_{3} $ 这一区间对应的功率曲线图; (c)蓝色加粗点$ \mu=3.1 $ ,$ d=6 $ 时两分量的模; (d)—(f)分别为在$ \mu=3.1 $ ,$ d=6 $ 时孤子和折射率的轮廓图, 稳定性分析以及加了1%白噪声的传输情况. 其他参数的选取为$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ Figure 5. (a), (b) Power diagrams corresponding to the u and v components in the interval of
$ \mu_{4} < \mu < \mu_{3} $ ; (c) the modulus of u and v when$ \mu=3.1 $ and$ d=6 $ (blue bold dot); (d)–(f) profile plots of soliton and refractive index, stability analysis, and transmission with 1% white noise for$ \mu=3.1 $ and$ d=6 $ , respectively. The other parameters are$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ .
图 6 (a)
$ \gamma=0.3 $ ,$ a_{1}=0.2 $ ,$ a_{2}=0.6 $ 参数下的线性谱; (b), (c) 分别为$ \mu=1.00 $ ,$ a_{1}=0.2 $ ,$ a_{2}=0.6 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ ,$ d=1 $ 参数下的u, v, m, n的轮廓图和u分量的传输图Figure 6. (a) Linear spectrum of µ at the parameter
$ \gamma=0.3 $ ,$ a_{1}=0.2 $ , and$ a_{2}=0.6 $ ; (b), (c) profile plots of soliton and refractive index and transmission with 1% white noise for$ \mu=1.00 $ ,$ a_{1}=0.2 $ ,$ a_{2}=0.6 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=0.3 $ ,$ d=1 $ , respectively.
图 7 (a), (b)分别为u和v分量在
$\mu > \mu_{1}''$ 这一区间对应的功率曲线图; (c)蓝色加粗点$ \mu=4.9 $ ,$ d=1 $ 时两分量的模; (d)蓝色加粗点$ \mu=4.9 $ ,$ d=1 $ 时的u, v, m, n; (e)$ \mu=4.9 $ ,$ d=1 $ 时的稳定性分析; (f)$ \mu=4.9 $ ,$ d=1 $ 时加了1%白噪声后u分量的传输; (g)—(i)分别为红色加粗点处$ \mu=4.2 $ ,$ d=1 $ 时对应的图; 其他参数的选取为$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=-0.3 $ Figure 7. (a), (b) The power diagrams corresponding to the u and v components in the interval of
$\mu > \mu_{1}''$ ; (c) ithe modulus of u and v when$ \mu=4.9 $ and$ d=1 $ (blue bold dot); (d) the contours of the soliton and refractive index at$ \mu=4.9 $ and$ d=1 $ ; (e) stability analysis when$ \mu=4.9 $ and$ d=1 $ ; (f) the stable propagation of the u component after adding 1% random noise when$ \mu=4.9 $ and$ d=1 $ ; (g)–(i) the corresponding diagrams when$ \mu=4.2 $ and$ d=1 $ (red bold dot), respectively. The other parameters are$ a_{1}=1 $ ,$ a_{2}=3 $ ,$ b_{1}=5.5 $ ,$ b_{2}=2 $ ,$ \gamma=-0.3 $ . -
[1] Jensen S M 1982 IEEE J. Quantum Electron. 18 1580
Google Scholar
[2] Trillo S, Wabnitz S, Wright E M, Stegeman G I 1988 Opt. Lett. 13 672
Google Scholar
[3] Abdullaev F K, Abrarov R M, Darmanyan S A 1989 Opt. Lett. 14 131
Google Scholar
[4] Paré C, Florjańczyk M 1990 Phys. Rev. A 41 6287
Google Scholar
[5] Mak W C K, Malomed B A, Chu P L 2004 Phys. Rev. E 69 066610
Google Scholar
[6] Herring G, Kevrekidis P G, Malomed B A, Carretero-González R, Frantzeskakis D J 2007 Phys. Rev. E 76 066606
Google Scholar
[7] Snyder A W, Mitchell D J 1997 Science 276 1538
Google Scholar
[8] Królikowski W, Bang O, Nikolov N I, Neshev D, Wyller J, Rasmussen J J, Edmundson D 2004 J. Opt. B: Quantum Semiclass. Opt. 6 S288
Google Scholar
[9] Peccianti M, Brzdakiewicz K A, Assanto G 2002 Opt. Lett. 27 1460
Google Scholar
[10] Pérez-García V M, Konotop V V, García-Ripoll J J 2000 Phys. Rev. E 62 4300
Google Scholar
[11] Bang O, Krolikowski W, Wyller J, Rasmussen J J 2002 Phys. Rev. E 66 046619
Google Scholar
[12] Ye F W, Dong L W, Hu B 2009 Opt. Lett. 34 584
Google Scholar
[13] Chen W, Wang Q, Shi J L, Shen M 2017 Opt. Commun. 403 22
Google Scholar
[14] Guo J L, Yang Z J, Song L M, Pang Z G 2020 Nonlinear Dynam. 101 1147
Google Scholar
[15] Guo J L, Yang Z J, Li X L, Zhang S M 2022 Chin. Phys. B 31 014203
Google Scholar
[16] Conti C, Peccianti M, Assanto G 2003 Phys. Rev. Lett. 91 073901
Google Scholar
[17] Peccianti M, Conti C, Assanto G 2005 Opt. Lett. 30 415
Google Scholar
[18] Rotschild C, Cohen O, Manela O, Segev M, Carmon T 2005 Phys. Rev. Lett. 95 213904
Google Scholar
[19] Królikowski W, Saffman M, Luther-Davies B, Denz C 1998 Phys. Rev. Lett. 80 3240
Google Scholar
[20] Mamaev A V, Zozulya A A, Mezentsev V K, Anderson D Z, Saffman M 1997 Phys. Rev. A 56 R1110
Google Scholar
[21] Wand M, Schindlmayr A, Meier T, Förstner J 2011 Phys. Status Solidi B 248 887
Google Scholar
[22] Dang Y L, Li H J, Lin J 2017 Nonlinear Dynam. 88 489
Google Scholar
[23] Gao Z J, Dang Y L, Lin J 2018 Opt. Commun. 426 302
Google Scholar
[24] 李森清, 张肖, 林机 2022 71 234207
Google Scholar
Li S Q, Zhang X, Lin J 2022 Acta Phys Sin. 71 234207
Google Scholar
[25] Wang Q, Mihalache D, Belic M, Zeng L W, Lin J 2023 Opt. Lett. 48 747
Google Scholar
[26] Bender C M, Boettcher S 1998 Phys. Rev. Lett. 80 5243
Google Scholar
[27] Wang H, Wang J D 2011 Opt. Express 19 4030
Google Scholar
[28] Abdullaev F K, Kartashov Y K, Konotop V V, Zezyulin D A 2011 Phys. Rev. A 83 041805
Google Scholar
[29] Nixon S, Ge L J, Yang J K 2012 Phys. Rev. A 85 023822
Google Scholar
[30] Cannata F, Junker G, Trost J 1998 Phys. Lett. A 246 219
Google Scholar
[31] Miri M A, Heinrich M, Christodoulides D N 2013 Phys. Rev. A 87 043819
Google Scholar
[32] Tsoy E N, Allayarov I M, Abdullaev F K 2014 Opt. Lett. 39 4215
Google Scholar
[33] Konotop V V, Zezyulin D A 2014 Opt. Lett. 39 5535
Google Scholar
[34] Nixon S, Yang J K 2016 Stud. Appl. Math. 136 459
Google Scholar
[35] Yang J K, Nixon S 2016 Phys. Lett. A 380 3803
Google Scholar
[36] Zhu X, He Y J 2018 Opt. Express 26 26511
Google Scholar
[37] Zhu X, Peng X, Qiu Y L, Wang H C, He Y J 2020 New J. Phys. 22 033035
Google Scholar
[38] Yang J K 2021 Stud. Appl. Math. 147 4
Google Scholar
[39] Zhu X, Liao S W, Cai Z, Qiu Y L, He Y J 2021 Chaos Soliton.s Fract. 146 110837
Google Scholar
[40] Zhu X, Cai Z, Liu J L, Liao S W, He Y J 2022 Nonlinear Dynam. 108 2563
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 2960
- PDF Downloads: 120
- Cited By: 0
