-
An appropriate weak value can be used to amplify weak physical parameters and improve the precision of parameter estimation in the process of quantum weak measurement. The increase of the precision of such a parameter estimation may originate from the entanglement in the system. This paper uses Fisher information to study the influence of the entanglement in the system and the entanglement between the system and the pointer on the estimation precision of the coupling parameters between the system and the pointer in the process of weak measurement. The entanglement of the entangled pure state of the GHZ-like state and the entangled mixed state affected by the depolarization noise and the influence of the entanglement between the system and the pointer on the parameter estimation are analyzed. The results show that the Fisher information quantity increases with the increase of the initial state entanglement degree of the system when the initial state of the system is an entangled pure state or an entangled mixed state affected by depolarization noise, and both the Fisher information quantity and the post-selection probability reach their maximum values when the initial and final state of the system are both the maximum entangled states; but the weaker the entanglement between the system and the pointer, the more the Fisher information obtained in the measurement will be and the higher the accuracy of parameter estimation. These research results show that the entanglement in the system will improve the precision of parameter estimation, while the entanglement between the system and the pointer will reduce the precision of parameter estimation.
-
Keywords:
- weak measurement /
- weak value /
- entanglement /
- Fisher information
[1] Aharonov Y, Albert D Z, Vaidman L 1988 Phys. Rev. Lett. 60 1351
Google Scholar
[2] Viza G I, Martínez-Rincón J, Alves G B, Jordan A N, Howell J C 2015 Phys. Rev. A 92 032127
Google Scholar
[3] Jozsa R 2007 Phys. Rev. A 76 044103
Google Scholar
[4] Dressel J 2015 Phys. Rev. A 91 032116
Google Scholar
[5] Dixon P B, Starling D J, Jordan A N, Howell J C 2009 Phys. Rev. Lett. 102 173601
Google Scholar
[6] Zhou L, Turek Y, Sun C P, Nori F 2013 Phys. Rev. A 88 053815
Google Scholar
[7] Starling D J, Dixon P B, Jordan A N, Howell J C 2010 Phys. Rev. A 82 063822
Google Scholar
[8] Magaña-Loaiza O S, Mirhosseini M, Rodenburg B, Boyd R W 2014 Phys. Rev. Lett. 112 200401
Google Scholar
[9] Viza G I, Martínez-Rincón J, Howland G A, Frostig H, Shomroni I, Dayan B, Howell J C 2013 Opt. Lett. 38 2949
Google Scholar
[10] Egan P, Stone J A 2012 Opt. Lett. 37 4991
Google Scholar
[11] Pati A K, Mukhopadhyay C, Chakraborty S, Ghosh S 2020 Phys. Rev. A 102 012204
Google Scholar
[12] Starling D J, Dixon P B, Williams N S, Jordan A N, Howell J C 2010 Phys. Rev. A 82 011802(R
Google Scholar
[13] Brunner N, Simon C 2010 Phys. Rev. Lett. 105 010405
Google Scholar
[14] Strübi G, Bruder C 2013 Phys. Rev. Lett. 110 083605
Google Scholar
[15] Xu X Y, Kedem Y, Sun K, Vaidman L, Li C F, Guo G C 2013 Phys. Rev. Lett. 111 033604
Google Scholar
[16] Zhang Z H, Chen G, Xu X Y, Tang J S, Zhang W H, Han Y J, Li C F, Guo G C 2016 Phys. Rev. A 94 053843
Google Scholar
[17] Liu W T, Martínez-Rincón J, Howell J C 2019 Phys. Rev. A 100 012125
Google Scholar
[18] Lyons K, Dressel J, Jordan A N, Howell J C, Kwiat P G 2015 Phys. Rev. Lett. 114 170801
Google Scholar
[19] Wang Y T, Tang J S, Hu G, Wang J, Yu S, Zhou Z Q, Cheng Z D, Xu J S, Fang S Z, Wu Q L, Li C F, Guo G C 2016 Phys. Rev. Lett. 117 230801
Google Scholar
[20] Pang S S, Dressel J, Brun T A 2014 Phys. Rev. Lett. 113 030401
Google Scholar
[21] Chen G S, Liu B H, Hu M J, Hu X M, Li C F, Guo G C, Zhang Y S 2019 Phys. Rev. A 99 032120
Google Scholar
[22] Zhang L J, Datta A, Walmsley L A 2015 Phys. Rev. Lett. 114 210801
Google Scholar
[23] Plenio M B 2005 Phys. Rev. Lett. 95 090503
Google Scholar
[24] Luo L, He Y, Liu X, Li Z X, Duan P, Zhang Z Y 2020 Opt. Express 28 6408
Google Scholar
-
图 1 n量子比特系统弱测量的原理图. 系统初始态为
$\left|S_{{\rm{i}}}\right\rangle$ , 探针初始态为$|T\rangle$ . 系统和探针之间的演化算符为$\hat {\boldsymbol {U}} $ . 演化后对系统做$\left| {{S}_{{\rm{f}}}} \right\rangle $ 的后选择. 探针末态为$\left|T_{{\rm{f}}}\right\rangle$ Figure 1. Schematic diagram of the weak measurement of the n qubit system. The initial state of the system is
$\left|S_{{\rm{i}}}\right\rangle$ , and the initial state of the pointer is$|T\rangle$ . The evolution operator is$ \hat {\boldsymbol {U}} $ between the system and the pointer. After the evolution, the system is selected to$\left| {{S}_{{\rm{f}}}} \right\rangle $ . The final state of the pointer is$\left|T_{{\rm{f}}}\right\rangle$ 
图 2 (a)后选择概率
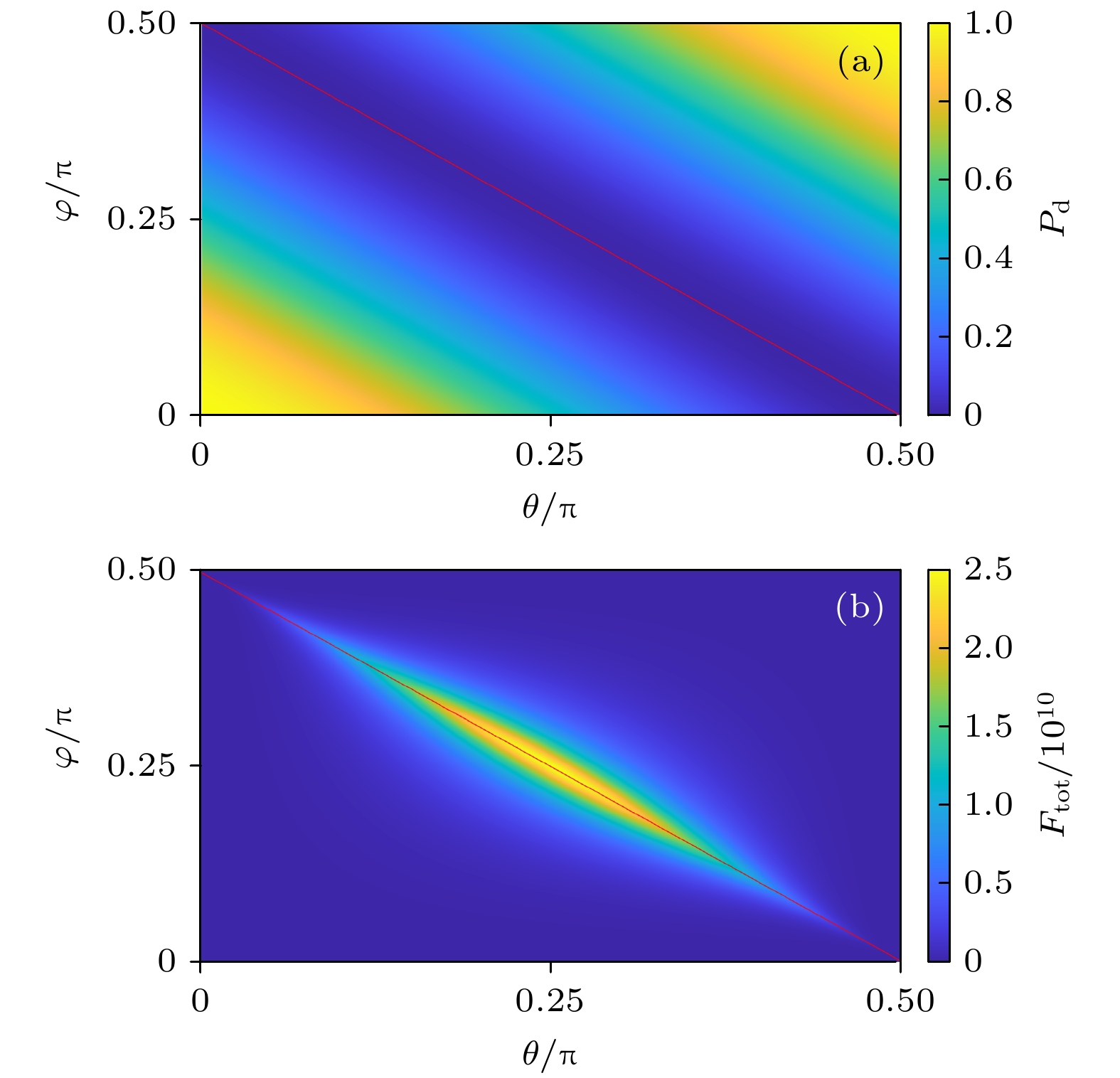
${{P}_{{\rm{d}}}}$ 随θ和φ的变化趋势; (b)总的Fisher信息${{F}_{{\rm{tot}}}}$ 随θ和φ的变化趋势. 图中红色的线表示$\theta +\varphi = {\pi }/{2}$ . 参数选取为$n=2$ ,$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = $ $ 0.01$ ,$g=0.88\;\rm{μ} {\rm{m}}$ Figure 2. (a) Post-selection probability
${{P}_{{\rm{d}}}}$ as a function of θ and φ; (b) total Fisher information${{F}_{{\rm{tot}}}}$ as a function of θ and φ. The red line represent$\theta +\varphi = {\pi }/{2}$ . Parameters selection:$n=2$ ,$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = $ $ 0.01$ ,$g=0.88\;\rm{μ} {\rm{m}}$ .
图 3 类GHZ态的纠缠纯态的纠缠度
$E_{{\rm{s}}}^{({\rm{p}})}$ 随参数θ的变化曲线, 绘制了$\theta =\{0, \pi /2\}$ 的范围Figure 3. Entanglement
$E_{{\rm{s}}}^{({\rm{p}})}$ of the entangled pure states of GHZ-like states as a function of the parameter$\theta$ . we have plotted the range of$\theta =\{0, \pi /2\}$ .
图 4 蓝色曲线表示后选择概率随纠缠度
$E_{{\rm{s}}}^{({\rm{p}})}$ 的变化曲线, 橙色曲线表示耦合参数g的Fisher信息随纠缠度$E_{{\rm{s}}}^{({\rm{p}})}$ 的变化曲线. 参数选取:$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = 0.01$ ,$g= $ $ 0.88\;\text{μ} {\rm{m}}$ ,$n=2$ .Figure 4. Blue curve represents the post-selection probability as a function of entanglement
$E_{{\rm{s}}}^{({\rm{p}})}$ , and the orange curve represents the Fisher information of the coupling parameter g as a function of entanglement$E_{{\rm{s}}}^{({\rm{p}})}$ . Parameter selection:$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = 0.01$ ,$g=0.88\;\text{μ} {\rm{m}}$ ,$n=2$ .
图 5
$n=2$ 时, 系统初态的纠缠度$E_{{\rm{s}}}^{({\rm{m}})}$ 随纯度参数Q的变化曲线Figure 5. Entanglement
$E_{{\rm{s}}}^{({\rm{m}})}$ of the initial state of the system as a function of the purity parameter Q. Parameter selection:$n=2$ .
图 6 蓝色曲线表示后选择概率随纠缠度
$E_{{\rm{s}}}^{({\rm{m}})}$ 的变化曲线, 橙色曲线表示参数g的Fisher信息随纠缠度$E_{{\rm{s}}}^{({\rm{m}})}$ 的变化曲线. 参数选取:$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = 0.01$ ,$g=0.88\;\rm{μ} {\rm{m}}$ Figure 6. Blue curve represents the post-selection probability as a function of entanglement
$E_{{\rm{s}}}^{({\rm{m}})}$ , and the orange curve represents the Fisher information of the parameter g as a function of entanglement$E_{{\rm{s}}}^{({\rm{m}})}$ . Parameter selection:$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = 0.01$ ,$g=0.88\;\rm {μ} {\rm{m}}$ .
图 7 浅蓝色曲线表示初态为纠缠态时参数g的Fisher信息随耦合参数g的变化曲线, 深蓝色曲线表示初态为直积态时参数g的Fisher信息随耦合参数g的变化曲线. 参数选取:
$n=2$ ,$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = 0.01$ Figure 7. Light blue curve represents Fisher information of the parameter g as a function of the coupling parameter g when the initial state is entangled, and dark blue curve represents Fisher information of the parameter g as a function of the coupling parameter g when the initial state is product state. Parameter selection:
$n=2$ ,$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}$ ,$\varepsilon = $ $ 0.01$ .
图 8 (a) 系统探针之间的纠缠度
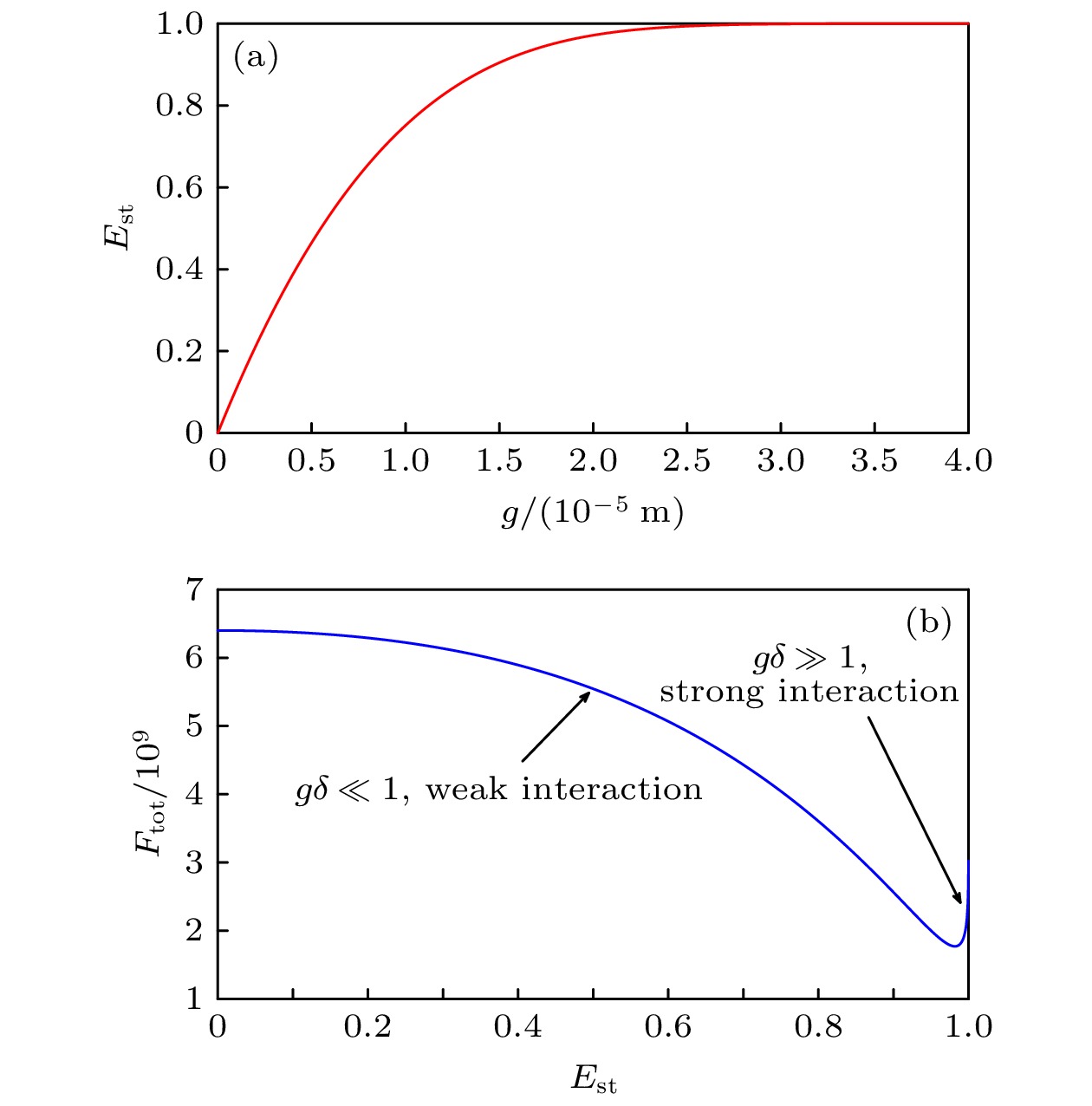
${{E}_{{\rm{st}}}}$ 随耦合参数g的变化曲线; (b)参数g的Fisher信息随系统探针之间的纠缠度${{E}_{{\rm{st}}}}$ 的变化曲线. 参数选取为$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}, \varepsilon = 0.01$ Figure 8. (a) Entanglement
${{E}_{{\rm{st}}}}$ between system and pointers as a function of the coupling parameter g; (b) the Fisher information of the parameter g as a function of the entanglement${{E}_{{\rm{st}}}}$ between system and pointers. Parameter selection:$\delta = 4\times {{10}^{4}}\;{{{\rm{m}}}^{-1}}, \varepsilon = 0.01$ .表 1 两种方案后选择概率和后选择后指针分布的比较
Table 1. Comparison of the post-selection probability and the post-selection pointer distribution of the two schemes
系统初态 后选择概率 后选择后指针分布 $\left| S_{\rm{i}}^{{\rm{m}}} \right\rangle $ ${ {P}_{\rm{d} } } = \dfrac{1}{2}\left( 1-{ {\text{e} }^{-2{ {g}^{2} }{ {n}^{2} }{ {\delta }^{2} } } }\cos \varepsilon \right)$ $\dfrac{1}{ { {P}_{\rm{d} } } }{ {\sin }^{2} }\Big(ngp+\dfrac{\varepsilon }{2}\Big)P(p)$ $\left| S_{\rm{i}}^{({\rm{P}})} \right\rangle $ $ { {P}_{\rm{d} } }^{\prime } = { {2}^{-n} }\left( 1- { {\text{e} }^{-2{ {g}^{2} }{ {n}^{2} }{ {\delta }^{2} } } } \cos \varepsilon\right) $ $\dfrac{1}{ { {2}^{n-1} }{ {P}_{\rm{d} } }^{\prime } }{ {\sin }^{2} }\Big(ngp+\dfrac{\varepsilon }{2}\Big)P(p)$ 
DownLoad: CSV
-
[1] Aharonov Y, Albert D Z, Vaidman L 1988 Phys. Rev. Lett. 60 1351
Google Scholar
[2] Viza G I, Martínez-Rincón J, Alves G B, Jordan A N, Howell J C 2015 Phys. Rev. A 92 032127
Google Scholar
[3] Jozsa R 2007 Phys. Rev. A 76 044103
Google Scholar
[4] Dressel J 2015 Phys. Rev. A 91 032116
Google Scholar
[5] Dixon P B, Starling D J, Jordan A N, Howell J C 2009 Phys. Rev. Lett. 102 173601
Google Scholar
[6] Zhou L, Turek Y, Sun C P, Nori F 2013 Phys. Rev. A 88 053815
Google Scholar
[7] Starling D J, Dixon P B, Jordan A N, Howell J C 2010 Phys. Rev. A 82 063822
Google Scholar
[8] Magaña-Loaiza O S, Mirhosseini M, Rodenburg B, Boyd R W 2014 Phys. Rev. Lett. 112 200401
Google Scholar
[9] Viza G I, Martínez-Rincón J, Howland G A, Frostig H, Shomroni I, Dayan B, Howell J C 2013 Opt. Lett. 38 2949
Google Scholar
[10] Egan P, Stone J A 2012 Opt. Lett. 37 4991
Google Scholar
[11] Pati A K, Mukhopadhyay C, Chakraborty S, Ghosh S 2020 Phys. Rev. A 102 012204
Google Scholar
[12] Starling D J, Dixon P B, Williams N S, Jordan A N, Howell J C 2010 Phys. Rev. A 82 011802(R
Google Scholar
[13] Brunner N, Simon C 2010 Phys. Rev. Lett. 105 010405
Google Scholar
[14] Strübi G, Bruder C 2013 Phys. Rev. Lett. 110 083605
Google Scholar
[15] Xu X Y, Kedem Y, Sun K, Vaidman L, Li C F, Guo G C 2013 Phys. Rev. Lett. 111 033604
Google Scholar
[16] Zhang Z H, Chen G, Xu X Y, Tang J S, Zhang W H, Han Y J, Li C F, Guo G C 2016 Phys. Rev. A 94 053843
Google Scholar
[17] Liu W T, Martínez-Rincón J, Howell J C 2019 Phys. Rev. A 100 012125
Google Scholar
[18] Lyons K, Dressel J, Jordan A N, Howell J C, Kwiat P G 2015 Phys. Rev. Lett. 114 170801
Google Scholar
[19] Wang Y T, Tang J S, Hu G, Wang J, Yu S, Zhou Z Q, Cheng Z D, Xu J S, Fang S Z, Wu Q L, Li C F, Guo G C 2016 Phys. Rev. Lett. 117 230801
Google Scholar
[20] Pang S S, Dressel J, Brun T A 2014 Phys. Rev. Lett. 113 030401
Google Scholar
[21] Chen G S, Liu B H, Hu M J, Hu X M, Li C F, Guo G C, Zhang Y S 2019 Phys. Rev. A 99 032120
Google Scholar
[22] Zhang L J, Datta A, Walmsley L A 2015 Phys. Rev. Lett. 114 210801
Google Scholar
[23] Plenio M B 2005 Phys. Rev. Lett. 95 090503
Google Scholar
[24] Luo L, He Y, Liu X, Li Z X, Duan P, Zhang Z Y 2020 Opt. Express 28 6408
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5586
- PDF Downloads: 153
- Cited By: 0
