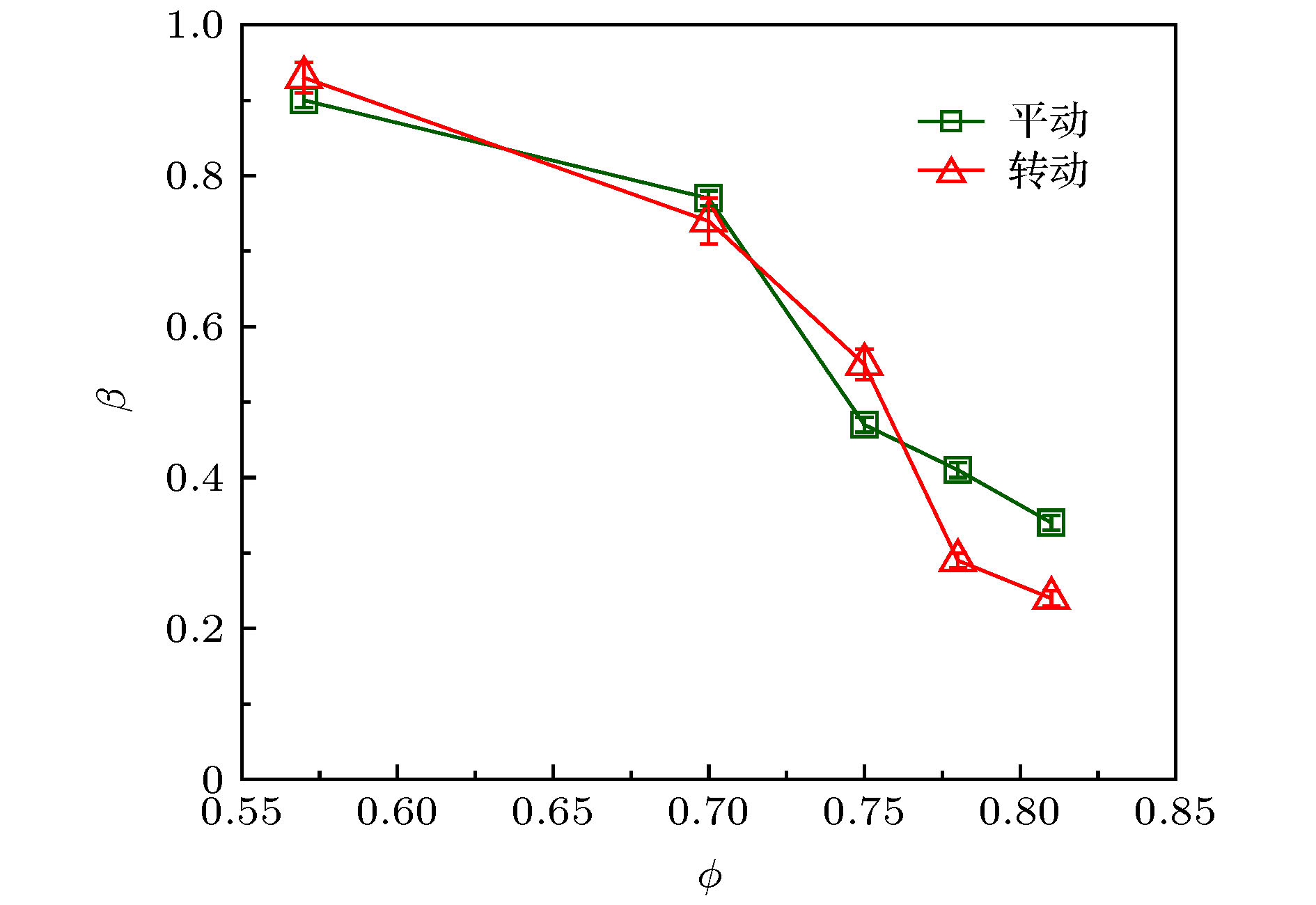
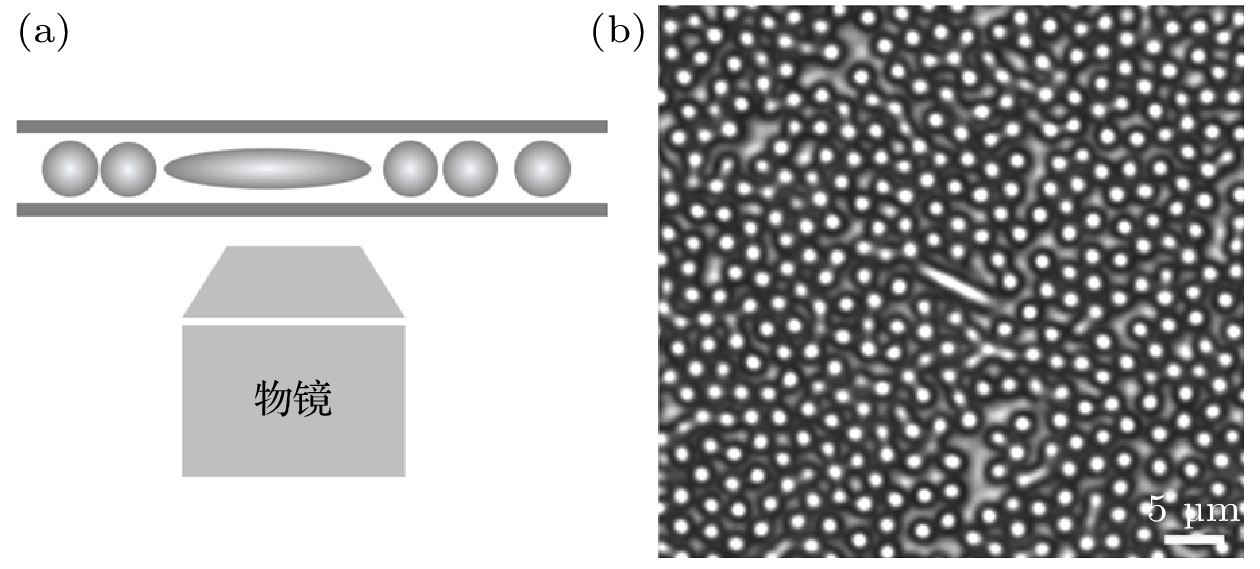
-
The diffusive transport in complex confined media is ubiquitous such as diffusions of micro- or nano-particles in glassy liquids and polymer solutions, protein diffusions under crowded conditions, and deliveries of drugs in the biological media. Therefore, the understanding of the diffusive transport arouses the great interest of researchers in the physics, materials science, and biology circles. Despite the fact that the shape of the colloidal particles acts as one of the important physical factors influencing their dynamic behaviors, the study of the anisotropic particles diffusing in confined media is still lacking. In this work, we propose a simple experimental model to investigate the confined diffusion of shape-anisotropic particles. The diffusion of an ellipsoid at different area fractions (ϕ) of colloidal spheres is investigated through video microscopy. At low ϕ, ellipsoid exhibits a random trajectory and free diffusion in translational and rotational degree of freedom; while at high ϕ, the trajectory is in a small spatial range with a nearly constant orientation of the particle, indicating that the arrested diffusion takes place in translational and rotational degree of freedom. The translational and rotational mean square displacement decrease with the increase of ϕ. By power-law fitting (~tβ), it is found that β decreases from 1 to a small value at high ϕ, demonstrating that the ellipsoid experiences a transition from normal diffusion to sub-diffusion. Moreover, β for rotational motion decreases faster than that for translational motion at high ϕ, which signifies that the the rotational motion decouples from the translational motion with increasing ϕ. The results from the van Hove correlation function show that the translational displacement along the major axis of the ellipsoid is always larger than that along the minor axis, manifesting the ellipsoid prefers to diffuse along its major axis independent of ϕ. Significant non-Gaussian tail is observed in the distribution of the translational displacement along the major axis with increasing ϕ. However, the distribution of the translational displacement along the minor axis presents a nearly Gaussian behavior independent of ϕ. This indicates that the translational motion along the major axis decouples from the translational motion along the minor with increasing ϕ. For the rotational displacement, the non-Gaussian tail is only observed at the intermediate ϕ. These non-Gaussian behaviors are confirmed by calculating the non-Gaussian parameter (α2). Our experiments demonstrate that the confinements give rise to the anomalous diffusion behaviors of the anisotropic colloids, which is conducive to the understanding of transportations of anisotropic objects in complex environments.
-
Keywords:
- colloids /
- diffusion /
- ellipsoid /
- non-Gaussian distribution /
- dynamics
[1] Wang Y, Benton L A, Singh V, Pielak G J 2012 J. Phys. Chem. Lett. 3 2703
Google Scholar
[2] Grimaldo M, Lopez H, Beck C, Roosen R F, Moulin M, Devos J M, Laux V, Hartlein M, Da Vela S, Schweins R, Mariani A, Zhang F, Barrat J L, Oettel M, Forsyth V T, Seydel T, Schreiber F 2019 J. Phys. Chem. Lett. 10 1709
Google Scholar
[3] Edmond K V, Elsesser M T, Hunter G L, Pine D J, Weeks E R 2012 P. Natl. Acad. Sci. U.S.A. 109 17891
Google Scholar
[4] Sentjabrskaja T, Zaccarelli E, De Michele C, Sciortino F, Tartaglia P, Voigtmann T, Egelhaaf S U, Laurati M 2016 Nat. Commun. 7 11133
Google Scholar
[5] Xue C, Zheng X, Chen K, Tian Y, Hu G 2016 J. Phys. Chem. Lett. 7 514
Google Scholar
[6] Wang X, Chen Y, Xue L, Pothayee N, Zhang R, Riffle J S, Reineke T M, Madsen L A 2014 J. Phys. Chem. Lett. 5 3825
Google Scholar
[7] Geng Y, Dalhaimer P, Cai S, Tsai R, Tewari M, Minko T, Discher D E 2007 Nat. Nanotechnol. 2 249
Google Scholar
[8] Mitragotri S, Lahann J 2009 Nat. Mater. 8 15
Google Scholar
[9] Hnggi P, Marchesoni F 2009 Rev. Mod. Phys. 81 387
Google Scholar
[10] Zhou H X, Rivas G, Minton A P 2008 Annu. Rev. Biophys. 37 375
Google Scholar
[11] Weeks E R, Crocker J C, Levitt A C, Schofield A, Weitz D A 2000 Science 287 627
Google Scholar
[12] Chen J X, Chen Y G, Ma Y Q 2016 Soft Matter 12 1876
Google Scholar
[13] Chen J X, Zhu J X, Ma Y Q, Cao J S 2014 Epl-Europhys. Lett. 106 18003
Google Scholar
[14] Glotzer S C, Solomon M J 2007 Nat. Mater. 16 557
Google Scholar
[15] Champion J A, Katare Y K, Mitragotri S 2007 P. Natl. Acad. Sci. U.S.A. 104 11901
Google Scholar
[16] Han Y, Alsayed A M, Nobili M, Zhang J, Lubensky T C, Yodh A G 2006 Science 314 626
Google Scholar
[17] Chakrabarty A, Konya A, Wang F, Selinger J V, Sun K, Wei Q H 2013 Phys. Rev. Lett. 111 160603
Google Scholar
[18] Moreno A J, Kob W 2004 AIP Conference Proceedings 708 576
Google Scholar
[19] Kim J, Sung B J 2015 Phys. Rev. Lett. 115 158302
Google Scholar
[20] Sakha F, Fazli H 2010 J. Chem. Phys. 133 234904
Google Scholar
[21] Ho C C, Keller A, Odell J A, Ottewill R H 1993 Colloid Polym. Sci. 271 469
Google Scholar
[22] Zheng Z, Wang F, Han Y 2011 Phys. Rev. Lett. 107 065702
Google Scholar
-
图 1 (a)样品在显微镜物镜上的示意图; (b) ϕ = 0.57的样品的显微镜照片
Figure 1. (a) Schematic of the experimental sample on a microscope objective; (b) a bright-field microscope image of the colloidal suspension for ϕ = 0.57.
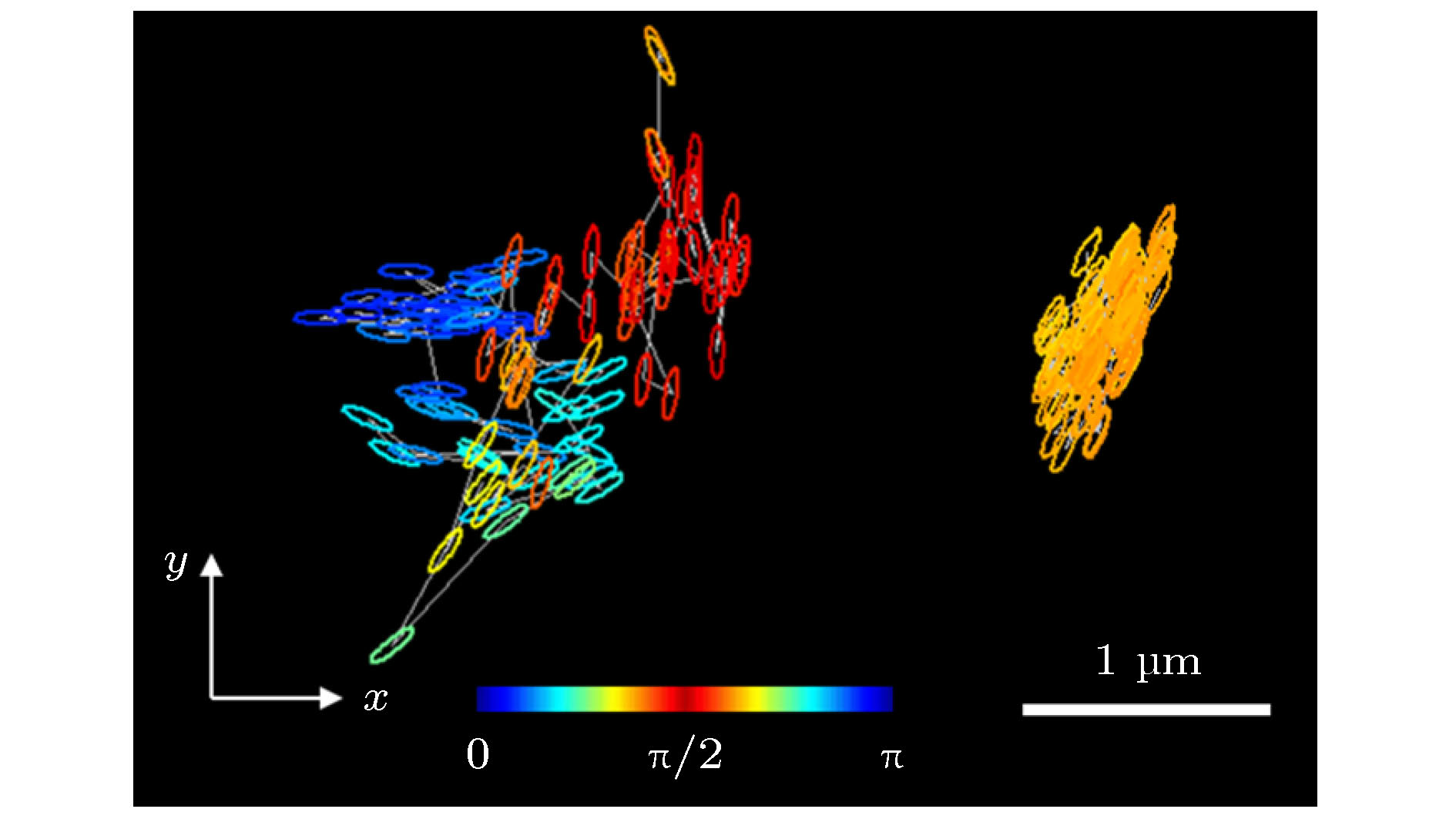
图 2 椭球在不同圆球浓度下运动100 s的轨迹: ϕ = 0.57(左)和ϕ = 0.81(右). 椭球的不同时刻位置用空心椭圆表示, 其取向是椭球长轴方向和x轴方向的夹角, 用颜色表示
Figure 2. 100 s trajectories of an ellipsoid at ϕ = 0.57 (left panel) and ϕ = 0.81 (right panel). The positions of the particle at different times are indicated by ellipses. The color indicates the orientation of the particle with respect to the x axis.
图 3 椭球在不同ϕ下的平动均方位移(a)和转动均方位移(b), 实线是时间范围为1−20 s的幂律拟合, ～tβ
Figure 3. Translational mean square displacements (a) and rotational mean square displacements (b) of ellipsoids at different ϕ. Solid lines are the power-law fits: ～tβ in the time range of 1−20 s.
图 4 平动和转动扩散指数β随浓度的变化
Figure 4. The ϕ dependent β for translational and rotational motions.
图 5 椭球在不同ϕ运动4 s的位移分布 (a)沿长轴方向平动位移; (b)沿短轴方向平动位移; (c)转动位移. 实线是高斯拟合
Figure 5. The distribution of the ellipsoid displacement for lag time of 4 s at different ϕ: (a) Translational displacement along the long axis of the ellipsoid; (b) translational displacement along the short axis, (c) rotational displacement. Solid lines are the best Gaussian fits.
图 6 椭球运动4 s沿长轴平动位移 (r//), 沿短轴平动位移 (r⊥) 和转动位移 (θ) 的非高斯参量
Figure 6. The non-Gauss parameter of the displacement of ellipsoid for lag time of 4 s: Translational displacement along the long axis of the ellipsoid (r//), translational displacement along the short axis (r⊥), and rotational displacement (θ).
-
[1] Wang Y, Benton L A, Singh V, Pielak G J 2012 J. Phys. Chem. Lett. 3 2703
Google Scholar
[2] Grimaldo M, Lopez H, Beck C, Roosen R F, Moulin M, Devos J M, Laux V, Hartlein M, Da Vela S, Schweins R, Mariani A, Zhang F, Barrat J L, Oettel M, Forsyth V T, Seydel T, Schreiber F 2019 J. Phys. Chem. Lett. 10 1709
Google Scholar
[3] Edmond K V, Elsesser M T, Hunter G L, Pine D J, Weeks E R 2012 P. Natl. Acad. Sci. U.S.A. 109 17891
Google Scholar
[4] Sentjabrskaja T, Zaccarelli E, De Michele C, Sciortino F, Tartaglia P, Voigtmann T, Egelhaaf S U, Laurati M 2016 Nat. Commun. 7 11133
Google Scholar
[5] Xue C, Zheng X, Chen K, Tian Y, Hu G 2016 J. Phys. Chem. Lett. 7 514
Google Scholar
[6] Wang X, Chen Y, Xue L, Pothayee N, Zhang R, Riffle J S, Reineke T M, Madsen L A 2014 J. Phys. Chem. Lett. 5 3825
Google Scholar
[7] Geng Y, Dalhaimer P, Cai S, Tsai R, Tewari M, Minko T, Discher D E 2007 Nat. Nanotechnol. 2 249
Google Scholar
[8] Mitragotri S, Lahann J 2009 Nat. Mater. 8 15
Google Scholar
[9] Hnggi P, Marchesoni F 2009 Rev. Mod. Phys. 81 387
Google Scholar
[10] Zhou H X, Rivas G, Minton A P 2008 Annu. Rev. Biophys. 37 375
Google Scholar
[11] Weeks E R, Crocker J C, Levitt A C, Schofield A, Weitz D A 2000 Science 287 627
Google Scholar
[12] Chen J X, Chen Y G, Ma Y Q 2016 Soft Matter 12 1876
Google Scholar
[13] Chen J X, Zhu J X, Ma Y Q, Cao J S 2014 Epl-Europhys. Lett. 106 18003
Google Scholar
[14] Glotzer S C, Solomon M J 2007 Nat. Mater. 16 557
Google Scholar
[15] Champion J A, Katare Y K, Mitragotri S 2007 P. Natl. Acad. Sci. U.S.A. 104 11901
Google Scholar
[16] Han Y, Alsayed A M, Nobili M, Zhang J, Lubensky T C, Yodh A G 2006 Science 314 626
Google Scholar
[17] Chakrabarty A, Konya A, Wang F, Selinger J V, Sun K, Wei Q H 2013 Phys. Rev. Lett. 111 160603
Google Scholar
[18] Moreno A J, Kob W 2004 AIP Conference Proceedings 708 576
Google Scholar
[19] Kim J, Sung B J 2015 Phys. Rev. Lett. 115 158302
Google Scholar
[20] Sakha F, Fazli H 2010 J. Chem. Phys. 133 234904
Google Scholar
[21] Ho C C, Keller A, Odell J A, Ottewill R H 1993 Colloid Polym. Sci. 271 469
Google Scholar
[22] Zheng Z, Wang F, Han Y 2011 Phys. Rev. Lett. 107 065702
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5525
- PDF Downloads: 90
- Cited By: 0
