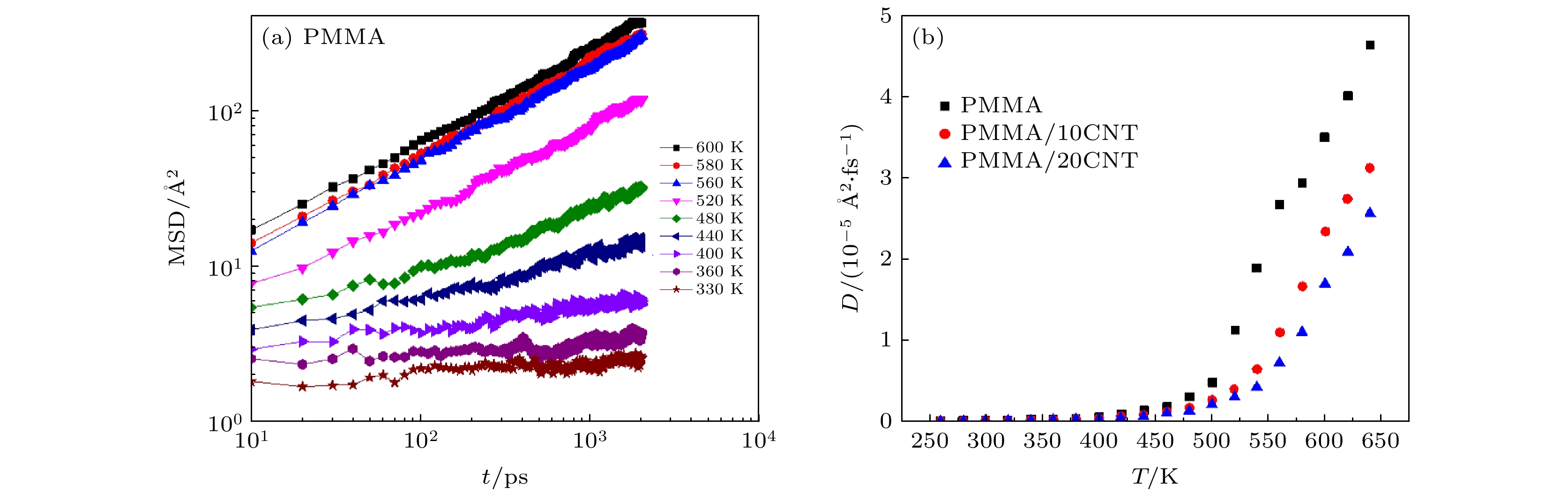
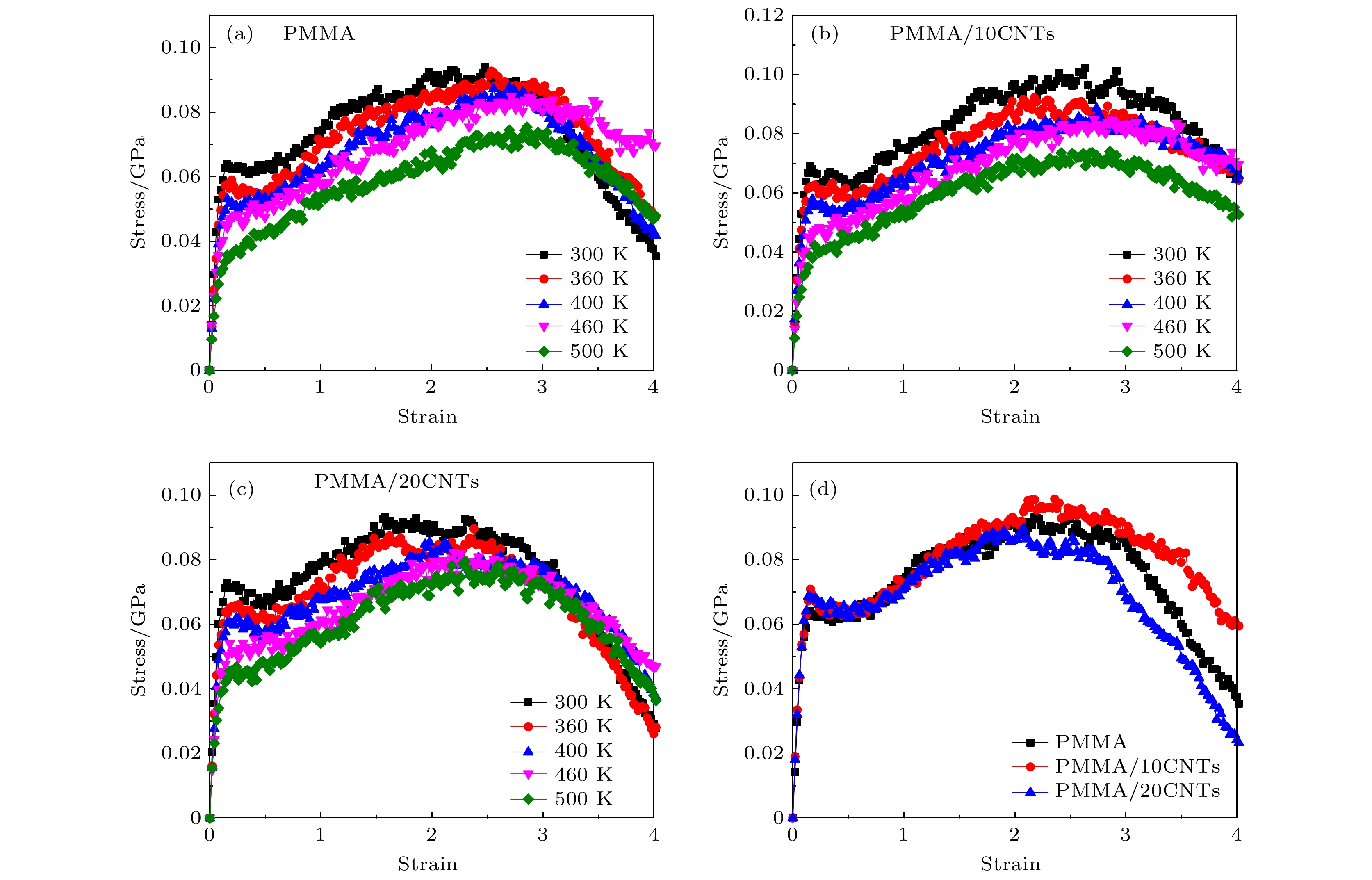
-
The glass transition temperature and nonlinear mechanics of polymer nanocomposites are strongly influenced by the short fibers. In this paper, coarse-grained molecular dynamics simulations are used to study the effects of single-walled carbon nanotube (CNT) content on the glass transition, diffusion coefficient, viscosity and nonlinear mechanical properties of poly(methyl methacrylate) (PMMA)/CNT nanocomposites. The glass transition temperature Tg is very important for the application of the materials. The Tg is related to the specific volume of the system. Generally, the location of the discontinuity on the curve of specific volume vs. temperature is the position of Tg. Our simulation results show that the Tg of PMMA/CNT composite increases with CNT content, and the result is consistent with the experimental value (434 K). This increase of Tg is evidently due to the presence of CNTs, which imposes a limit on the mobility of the molecules of PMMA. For the free volume in the liquid state, recent experiments pointed out that the molecular mutation is relatively easy to occur because the unoccupied volume is large. Further analysis of the diffusion coefficient of the PMMA/CNT indicates that the difference in diffusion characteristic occurs above the glass transition temperature, and the diffusion coefficient of PMMA system and PMMA/CNT system are the same below the glass transition temperature. Polymer materials in the service process will inevitably suffer the deformation, and the modulus and toughness of material are inversely proportional. Based on this problem, the nonlinear mechanical properties of short CNTs added PMMA composite are studied by nonequilibrium molecular dynamics. Our results show that the yield modulus increases with the CNT content increasing. However, the toughness is almost unchanged. In order to further understand the origin of stress of PMMA/CNT nanocomposites, the stretch ratio and orientation parameters of MPPA chains are also investigated in the present work. According to the stretch ratio and orientation parameters, it is not difficult to conclude that the stress-strain curve is mainly the result of the synergistic effect of molecular chain stretching and orientation. This work provides a theoretical guidance for further experiments and processing at the atomic and molecular level.
-
Keywords:
- glass transition temperature /
- nonlinear mechanics /
- nanocomposites /
- diffusion coefficient
[1] Mackay M E, Dao T T, Tuteja A, Ho D L, van Horn B, Kim H C, Hawker C J 2003 Nat. Mater. 2 762
Google Scholar
[2] Polizos G, Tuncer E, Agapov A L, Stevens D, Sokolov A P, Kidder M K, Jacobs J D, Koerner H, Vaia R A, More K L, Sauers I 2012 Polymer 53 595
Google Scholar
[3] Mammeri F, Teyssandier J, Connan C, Le Bourhis E, Chehimi M M 2012 RSC Adv. 2 2462
Google Scholar
[4] Luo J T, Wen H C, Wu W F, Chou C P 2008 Polym. Compos. 29 1285
Google Scholar
[5] De S K 1996 Short Fibre-polymer Composites (Woodhead: Woodhead Publishing) p257
[6] Salami-Kalajahi M, Haddadi-Asl V, Behboodi-Sadabad F, Rahimi-Razin S, Roghani-Mamaqani H 2012 Polym. Compos. 33 215
Google Scholar
[7] Van Loock F, Fleck N A 2018 Polymer 148 259
Google Scholar
[8] Haggenmueller R, Gommans H H, Rinzler A G, Fischer J E, Winey K I 2000 Chem. Phys. Lett. 330 219
Google Scholar
[9] Du F, Fischer J E, Winey K I 2003 J. Polym. Sci. , Part B:Polym. Phys. 41 3333
Google Scholar
[10] Wang J F, Yang J P, Tam L h, Zhang W 2021 Mech. Syst. Singal PR 153 107530
Google Scholar
[11] 杨俊升, 朱子亮, 曹启龙 2020 69 038101
Yang J S, Zhu Z L, Cao Q L 2020 Acta Phys. Sin. 69 038101
[12] 陈超, 段芳莉 2020 69 1 93102
Google Scholar
Chen C, Duan F L 2020 Acta Phys. Sin. 69 1 93102
Google Scholar
[13] Yang J S, Yang C L, Wang M S, Chen B D, Ma X G 2011 Phys. Chem. Chem. Phys. 13 15476
Google Scholar
[14] Skountzos E N, Mermigkis P G, Mavrantzas V G 2018 J. Phys. Chem. B 122 9007
Google Scholar
[15] Jiang Q, Tallury S S, Qiu Y, Pasquinelli M A 2020 Nanotechnol. Rev. 9 136
Google Scholar
[16] Mohammadi M, fazli H, karevan M, Davoodi J 2017 Eur. Polym. J. 91 121
Google Scholar
[17] Zhao J, Jiang J W, Wang L, Guo W, Rabczuk T 2014 J. Mech. Phys. Solids 71 197
Google Scholar
[18] Arash B, Park H S, Rabczuk T 2015 Compos. Struct. 134 981
Google Scholar
[19] Meng Z, Soler-Crespo R A, Xia W, Gao W, Ruiz L, Espinosa H D, Keten S 2017 Carbon 117 476
Google Scholar
[20] Mousavi A A, Arash B, Zhuang X, Rabczuk T 2016 Compos. B. Eng. 95 404
Google Scholar
[21] Ash B J, Siegel R W, Schadler L S 2004 J. Polym. Sci. , Part B:Polym. Phys. 42 4371
Google Scholar
[22] Mohammadi M, Davoodi J, Javanbakht M, Rezaei H 2018 Mater. Res. Express 6 035309
Google Scholar
-
图 1 (a) 1个PMMA聚合物链的3个单体及其由3个珠子组成的CG模型; (b) 1个(5, 5)带有10个碳原子环的CNT及其由3个珠子组成的CG模型
Figure 1. (a) Three monomers of a PMMA polymer chain and its CG model made of three beads; (b) a (5, 5) CNT with 10 rings of carbon atoms and its CG model made of three beads.
图 2 PMMA及PMMA/CNTs结构示意图
Figure 2. Schematic diagram of PMMA and PMMA/CNTs.
图 3 PMMA, PMMA/10CNTs及PMMA/20CNTs体系对应的比容随着温度的变化
Figure 3. Specific volumes of PMMA, PMMA/10CNTs and PMMA/20CNTs under the different temperatures.
图 4 (a) PMMA体系对应的MSD; (b) PMMA, PMMA/10CNTs及PMMA/20CNTs体系不同温度下对应的扩散系数
Figure 4. (a) Evolution of mean square displacement (MSD) of PMMA system; (b) the diffusion coefficient of PMMA, PMMA/ 10CNTs and PMMA/20CNTs under the different temperatures.
图 5 PMMA, PMMA/10CNTs及PMMA/20CNTs体系在300 K下对应的应力-应变(a)、拉伸比(b)和取向参数(c)变化曲线
Figure 5. Evolutions of stress-strain curves (a), stretch ratio (b) and orientational parameter (c) of PMMA, PMMA/10CNTs and PMMA/20CNTs under the temperature of 300 K.
图 6 PMMA (a), PMMA/10CNTs (b)及PMMA/20CNTs (c)结构体系在不同温度下对应的应力-应变曲线及在相同过冷度(ΔT= 135 K)下3个体系结构对应的应力-应变曲线(d)
Figure 6. E of stress-strain curves of PMMA (a), PMMA/10CNTs (b) and PMMA/20CNTs (c) under the different temperatures and the same cooling depth (ΔT = 135 K)(d).
图 7 PMMA体系维诺体积随着应变的变化过程
Figure 7. Voronoi volume of PMMA system under the different stain.
图 8 PMMA/10CNTs体系维诺体积随着应变的变化过程
Figure 8. Voronoi volume of PMMA/10CNTs system under the different stain.
表 1 PMMA及PMMA-CNT粗粒化粒子之间相互作用力场参数
Table 1. Parameters of the CG force field for PMMA and CNT beads.
Type of interaction Parameters PMMA CNT PMMA
/CNTBond kd/(kcal·mol–1·Å–2) 97.3 805.15 — d0/Å 4.05 9.45 — Angle kθ/(kcal·mol–1·Å–2) 400 32140 — θ0/(o) 84.6 180 — vdW D0/(kcal·mol–1) 0.56 5.34 1.4 r0/Å 6.53 9.45 7.7 
DownLoad: CSV
-
[1] Mackay M E, Dao T T, Tuteja A, Ho D L, van Horn B, Kim H C, Hawker C J 2003 Nat. Mater. 2 762
Google Scholar
[2] Polizos G, Tuncer E, Agapov A L, Stevens D, Sokolov A P, Kidder M K, Jacobs J D, Koerner H, Vaia R A, More K L, Sauers I 2012 Polymer 53 595
Google Scholar
[3] Mammeri F, Teyssandier J, Connan C, Le Bourhis E, Chehimi M M 2012 RSC Adv. 2 2462
Google Scholar
[4] Luo J T, Wen H C, Wu W F, Chou C P 2008 Polym. Compos. 29 1285
Google Scholar
[5] De S K 1996 Short Fibre-polymer Composites (Woodhead: Woodhead Publishing) p257
[6] Salami-Kalajahi M, Haddadi-Asl V, Behboodi-Sadabad F, Rahimi-Razin S, Roghani-Mamaqani H 2012 Polym. Compos. 33 215
Google Scholar
[7] Van Loock F, Fleck N A 2018 Polymer 148 259
Google Scholar
[8] Haggenmueller R, Gommans H H, Rinzler A G, Fischer J E, Winey K I 2000 Chem. Phys. Lett. 330 219
Google Scholar
[9] Du F, Fischer J E, Winey K I 2003 J. Polym. Sci. , Part B:Polym. Phys. 41 3333
Google Scholar
[10] Wang J F, Yang J P, Tam L h, Zhang W 2021 Mech. Syst. Singal PR 153 107530
Google Scholar
[11] 杨俊升, 朱子亮, 曹启龙 2020 69 038101
Yang J S, Zhu Z L, Cao Q L 2020 Acta Phys. Sin. 69 038101
[12] 陈超, 段芳莉 2020 69 1 93102
Google Scholar
Chen C, Duan F L 2020 Acta Phys. Sin. 69 1 93102
Google Scholar
[13] Yang J S, Yang C L, Wang M S, Chen B D, Ma X G 2011 Phys. Chem. Chem. Phys. 13 15476
Google Scholar
[14] Skountzos E N, Mermigkis P G, Mavrantzas V G 2018 J. Phys. Chem. B 122 9007
Google Scholar
[15] Jiang Q, Tallury S S, Qiu Y, Pasquinelli M A 2020 Nanotechnol. Rev. 9 136
Google Scholar
[16] Mohammadi M, fazli H, karevan M, Davoodi J 2017 Eur. Polym. J. 91 121
Google Scholar
[17] Zhao J, Jiang J W, Wang L, Guo W, Rabczuk T 2014 J. Mech. Phys. Solids 71 197
Google Scholar
[18] Arash B, Park H S, Rabczuk T 2015 Compos. Struct. 134 981
Google Scholar
[19] Meng Z, Soler-Crespo R A, Xia W, Gao W, Ruiz L, Espinosa H D, Keten S 2017 Carbon 117 476
Google Scholar
[20] Mousavi A A, Arash B, Zhuang X, Rabczuk T 2016 Compos. B. Eng. 95 404
Google Scholar
[21] Ash B J, Siegel R W, Schadler L S 2004 J. Polym. Sci. , Part B:Polym. Phys. 42 4371
Google Scholar
[22] Mohammadi M, Davoodi J, Javanbakht M, Rezaei H 2018 Mater. Res. Express 6 035309
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 5034
- PDF Downloads: 86
- Cited By: 0
