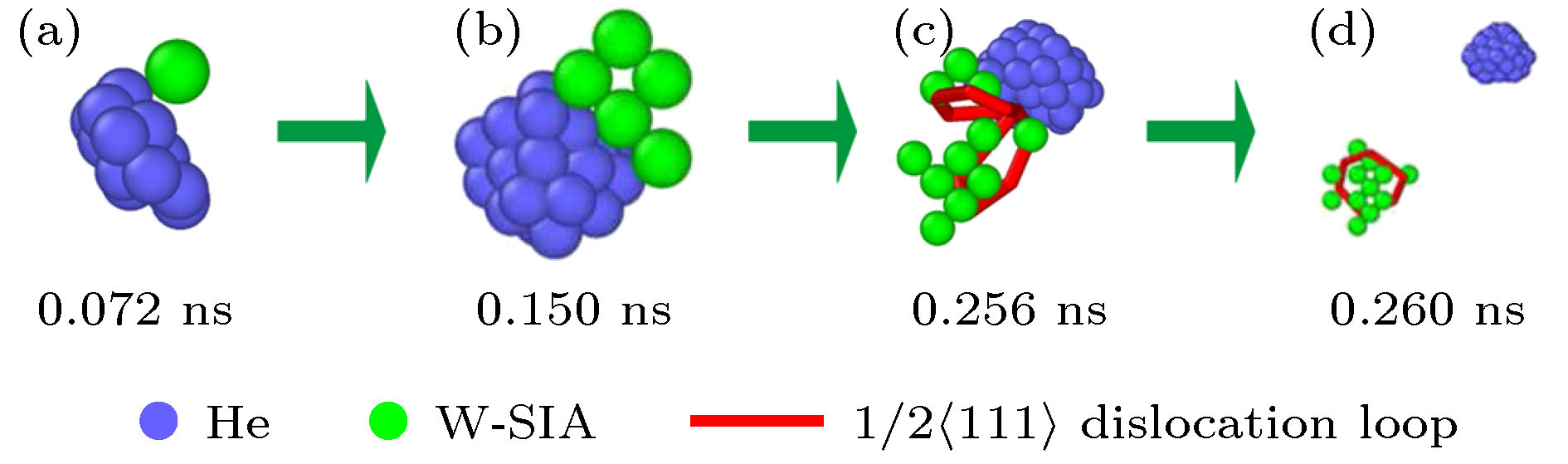
-
Tungsten (W) is a potential candidate for plasma facing materials (PFMs) of fusion reactor. The helium (He) produced in fusion reaction is insoluble and easy to gather and form to He bubbles in W, resulting in embrittlement and degradation of the performance of the W matrix. In this paper, based on molecular dynamics, the nucleation and growth of helium bubbles in the bulk and at ∑3[211](110) and ∑9[110](411) grain boundaries of W was studied. As a result, the growth mechanism of Helium bubbles at grain boundary of W was different from in bulk. Helium bubbles in bulk W grow up by extruding dislocation rings. The growth mechanism of helium bubbles at ∑3[211](110) grain boundary was as follows: Firstly, a small amount of W interstitial atoms were extruded and emitted. And then the 1/2
$\left\langle {111} \right\rangle $ dislocation line was extruded. Finally, the 1/2$\left\langle {111} \right\rangle $ dislocation line would migrate along the direction of [111] of the grain boundary interface. Moreover, the emission of W interstitial atoms and dislocation extrusion of the helium bubble were not observed in our simulated time scale at the ∑9[110](411) grain boundary. Then we used the NEB method to calculate the diffusion barrier of self-gap atoms in the bulk and at ∑3[211](110) and ∑9[110](411) grain boundaries of W, which explained the simulation results. The migration energy barrier of W self-gap atoms in the bulk and at ∑3[211](110) grain boundary was only a few to a few millielectron volts. So as long as W self-gap atoms dissociated from the surface of the He bubble in the thermal relaxation process, they can be easily migrated out. However, The migration energy of the W self-gap atom at the ∑9[110](411) grain boundary can be from a few tenths to a few electron volts. Even during the thermal relaxation process, the W self-gap atoms dissociated from the surface of the He bubble. It was difficult for the W self-gap atoms migrated out. Finally, the correlation between He bubble size and stress released was given. Either in bulk or at ∑3[211](110) and ∑9[110](411) grain boundaries of W, after the pressure of the helium bubble becomes stable with time, the radius of the helium bubble would increase rapidly whenever the pressure dropped sharply. So there was a small step on the curve of the evolution of the radius of the helium bubble with time. Thus, helium bubbles in W could promote growth by releasing pressure intermittently.-
Keywords:
- Helium bubbles /
- Tungsten /
- grain boundary /
- molecular dynamics
[1] Pintsuk G 2012 Comprehensive Nuclear Materials (Vol. 5) (Oxford: Elsevier Press) p551
[2] Hirai T, Escourbiac F, Carpentier-Chouchana S, Durocher A, Fedosov A, Ferrand L, Jokinen T, Komarov V, Merola M, Mitteau R, Pitts R A, Shu W, Sugihara M, Barabash V, Kuznetsov V, Riccardi B, Suzuki S 2014 Phys. Scr. T 159 014006
[3] Wei Q, Li N, Sun K, Wang L 2010 Scr. Mater. 63 430
Google Scholar
[4] Hetherly J, Martinez E, Di Z, Nastasi M, Caro A 2012 Scr. Mater. 66 17
Google Scholar
[5] 郭洪燕, 夏敏, 燕青芝, 郭立平, 陈济红, 葛昌纯 2016 65 077803
Google Scholar
Guo H Y, Xia M, Yan Q Z, Guo L P, Ge C C 2016 Acta Phys. Sin. 65 077803
Google Scholar
[6] Wang J, Gao X, Gao N, Wang Z G, Cui M, Wei K, Yao C, Sun J, Li B, Zhu Y, Pang L, Li Y, Wang D, Xie E 2015 J. Nucl. Mater. 457 182
Google Scholar
[7] Ding M S, Du J P, Wan L, Ogata S, Tian L, EvanMa, Han W Z, Li J, Shan Z W 2016 Nano. Lett. 16 4118
Google Scholar
[8] 马玉田, 刘俊标, 韩立, 田利丰, 王学聪, 孟祥敏, 肖善曲, 王波 2016 68 040702
Ma Y T, Liu J B, Han L, Tian L F, Wang X C, Meng X M, Xiao S Q, Wang B 2016 Acta Phys. Sin. 68 040702
[9] 王欣欣, 张颖, 周洪波, 王金龙 2014 63 046103
Google Scholar
Wang X X, Zhang Y, Zhou H B, Wang J L 2014 Acta Phys. Sin. 63 046103
Google Scholar
[10] El-Atwani O, Gonderman S, Suslov S, Efe M, Temmerman G D, Morgan T, Bystrov K, Hattar K, Allain J P 2015 Fusion Eng. Des. 93 9
Google Scholar
[11] Miyamoto M, Mikami S, Nagashima H, Iijima N, Nishijima D, Doerner R P, Yoshida N, Watanabe H, Ueda Y, Sagara A 2015 J. Nucl. Mater. 463 333
Google Scholar
[12] Wang J, Niu L-L, Shu X, Zhang Y 2015 Nucl. Fusion 55 092003
Google Scholar
[13] Kobayashi R, Hattori T, Tamura T, Ogata S 2015 J. Nucl. Mater. 463 1071
Google Scholar
[14] Sandoval L, Perez D, Uberuaga B P, Voter A F 2015 Phys. Rev. Lett. 114 105502
Google Scholar
[15] Yang S T, Hu N W, Gou X Q, Wang C L, Zhu X L 2016 RCS Advances 64 59875
[16] Yang L, Deng H Q, Gao F, Heinisch H L, Kurtz R J, Hu S Y, Li Y L, Zu X T 2013 Nucl. Instrum. Methods B 303 68
Google Scholar
[17] Xie H X, Gao N, Xu K, Lu G H, Yue T, Yin F X 2017 Acta Mater. 141 10
Google Scholar
[18] Liu X Y, Uberuaga B P, Perez D, Voter A F 2018 Mater. Res. Lett. 9 522
[19] Yang L, Gao F, Kurtz R J, Zu X T, Peng S M, Long X G, Zhou X S 2015 Acta Mater. 97 86
Google Scholar
[20] Zhao Q, Zhang Z, Li Y, Ouyang X 2017 Sci. Technol. Nucl. Ins. 2017 1
[21] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[22] Stukowski A 2010 Modell. Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[23] Ackland G J, Thetford R 1987 Philos. Mag. A 56 15
[24] Beck D E 1968 Mol. Phys. 14 311
Google Scholar
[25] Juslin N, Wirth B D 2013 J. Nucl. Mater. 432 61
Google Scholar
[26] Ziegler J F, Biersack J P, Littmark U 1985 The Stopping and Range of Ions in Matter (Vol. 1) (New York: Pergamon Press) p93
[27] Stukowski A, Albe K 2010 Modell. Simul. Mater. Sci. Eng. 18 085001
Google Scholar
[28] Guo S H, Zhu B E, Liu W C, Pan Z Y, Wang Y X 2009 Nucl. Instrum. Methods B 267 3278
Google Scholar
[29] Yang L, Zu Z Q, Peng S M, Long X G, Zhou X S, Zu X T, Heinisch H L, Kurtz R J, Gao F 2013 J. Nucl. Mater. 441 6
Google Scholar
[30] Rycroft C H, Grest G S, Landry J W, Bazant M Z 2006 Phys. Rev. E 74 021306
Google Scholar
[31] Chen L, Liu Y L, Zhou H B, Jin S, Zhang Y, Lu G H 2012 Sci. Chin. Phys. Mech. 55 614
Google Scholar
[32] He W H, Gao X, Gao N, Wang J, Wang D, Cui M H, Pang L L, Wang Z G 2018 Chin. Phys. Lett. 35 49
[33] Banisalman M J, Oda T 2019 Comput. Mater. Sci. 158 346
Google Scholar
-
图 1 单晶W中氦团簇成核长大初期的位错环发射过程
Figure 1. The punching-loop at the early stage of nucleation and growth of helium clusters in bulk W
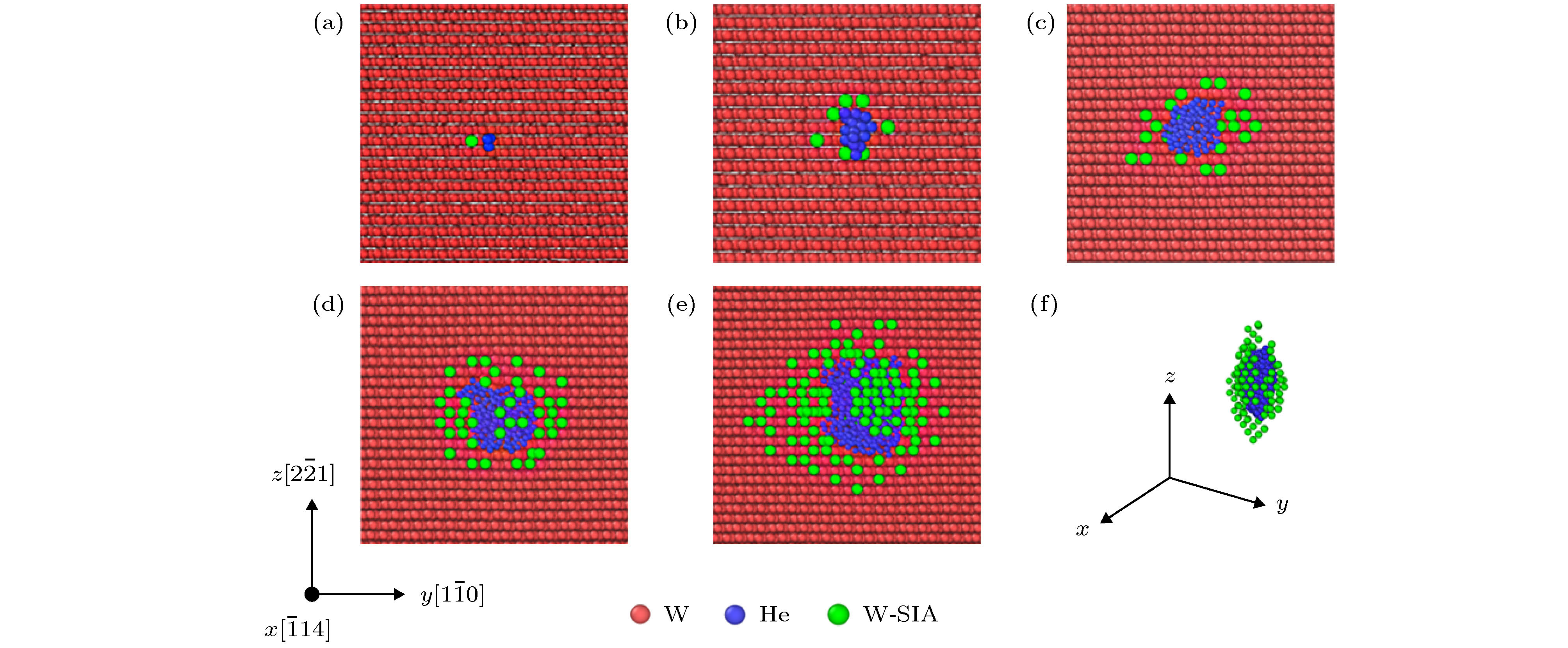
图 2 氦泡在W中∑3[211](110)晶界处的成核长大过程 (a) 0.043 ns, 8 He, 1 SIA; (b) 0.120 ns, 24 He, 6 SIAs; (c) 0.125 ns, 24 He, 6 SIAs; (d) 0.466 ns, 93 He, 21 SIAs; (e) 0.469 ns, 94 He, 22 SIAs; (f) 0.470 ns, 94 He, 22 SIAs
Figure 2. The nucleation and growth of helium clusters at grain boundary ∑3[211](110) in W: (a) 0.043 ns, 8 He, 1 SIA; (b) 0.120 ns, 24 He, 6 SIAs; (c) 0.125 ns, 24 He, 6 SIAs; (d) 0.466 ns, 93 He, 21 SIAs; (e) 0.469 ns, 94 He, 22 SIAs; (f) 0.470 ns, 94 He, 22 SIAs
图 3 氦泡在W中∑9[110](411)晶界处的成核长大过程 (a) 0.02 ns, 3 He, 1 SIA; (b) 0.1 ns, 19 He, 7 SIAs; (c) 0.5 ns, 99 He, 23 SIAs; (d) 1 ns, 199 He, 44 SIAs; (e) 2 ns, 399 He, 121 SIAs; (f) 2 ns
Figure 3. The nucleation and growth of helium clusters at grain boundary ∑9[110](411) in W: (a) 0.02 ns, 3 He, 1 SIA; (b) 0.1 ns, 19 He, 7 SIAs; (c) 0.5 ns, 99 He, 23 SIAs; (d) 1 ns, 199 He, 44 SIAs; (e) 2 ns, 399 He, 121 SIAs; (f) 2 ns
图 4 单晶W中自间隙原子的迁移能垒
Figure 4. Calculation of the migration barrier for a W crowdion defect in bulk W
图 5 W中∑3[211](110)晶界处W自间隙原子的迁移能垒
Figure 5. Calculation of the migration barrier for a W crowdion defect at grain boundary ∑3[211](110) in W
图 6 W中∑9[110](411)晶界处的自间隙原子的迁移能垒
Figure 6. Calculation of the migration barrier for a W crowdion defect at grain boundary ∑9[110](411) in W
图 7 (a)单晶W中氦泡的压强与半径随时间的变化; (b) ∑3[211](110)晶界处氦泡的压强与半径随时间的变化; (c) ∑9[110](411)晶界处氦泡的压强与半径随时间的变化
Figure 7. (a) The radius and pressure of the He bubble as a function of simulation time in bulk W; (b) the radius and pressure of the He bubble as a function of simulation time at at grain boundary ∑3[211](110); (c) the radius and pressure of the He bubble as a function of simulation time at at grain boundary ∑9[110](411)
表 1 单晶W及晶界处弗伦克尔缺陷对的形成能
Table 1. Formation energy of frenkel defect pair in bulk W and at grain boundaries.
缺陷位置 弗伦克尔缺陷对的形成能/eV 单晶W中 14.10 ∑3[211](110)晶界处 12.73 ∑9[110](411)晶界处 3.84 
DownLoad: CSV
-
[1] Pintsuk G 2012 Comprehensive Nuclear Materials (Vol. 5) (Oxford: Elsevier Press) p551
[2] Hirai T, Escourbiac F, Carpentier-Chouchana S, Durocher A, Fedosov A, Ferrand L, Jokinen T, Komarov V, Merola M, Mitteau R, Pitts R A, Shu W, Sugihara M, Barabash V, Kuznetsov V, Riccardi B, Suzuki S 2014 Phys. Scr. T 159 014006
[3] Wei Q, Li N, Sun K, Wang L 2010 Scr. Mater. 63 430
Google Scholar
[4] Hetherly J, Martinez E, Di Z, Nastasi M, Caro A 2012 Scr. Mater. 66 17
Google Scholar
[5] 郭洪燕, 夏敏, 燕青芝, 郭立平, 陈济红, 葛昌纯 2016 65 077803
Google Scholar
Guo H Y, Xia M, Yan Q Z, Guo L P, Ge C C 2016 Acta Phys. Sin. 65 077803
Google Scholar
[6] Wang J, Gao X, Gao N, Wang Z G, Cui M, Wei K, Yao C, Sun J, Li B, Zhu Y, Pang L, Li Y, Wang D, Xie E 2015 J. Nucl. Mater. 457 182
Google Scholar
[7] Ding M S, Du J P, Wan L, Ogata S, Tian L, EvanMa, Han W Z, Li J, Shan Z W 2016 Nano. Lett. 16 4118
Google Scholar
[8] 马玉田, 刘俊标, 韩立, 田利丰, 王学聪, 孟祥敏, 肖善曲, 王波 2016 68 040702
Ma Y T, Liu J B, Han L, Tian L F, Wang X C, Meng X M, Xiao S Q, Wang B 2016 Acta Phys. Sin. 68 040702
[9] 王欣欣, 张颖, 周洪波, 王金龙 2014 63 046103
Google Scholar
Wang X X, Zhang Y, Zhou H B, Wang J L 2014 Acta Phys. Sin. 63 046103
Google Scholar
[10] El-Atwani O, Gonderman S, Suslov S, Efe M, Temmerman G D, Morgan T, Bystrov K, Hattar K, Allain J P 2015 Fusion Eng. Des. 93 9
Google Scholar
[11] Miyamoto M, Mikami S, Nagashima H, Iijima N, Nishijima D, Doerner R P, Yoshida N, Watanabe H, Ueda Y, Sagara A 2015 J. Nucl. Mater. 463 333
Google Scholar
[12] Wang J, Niu L-L, Shu X, Zhang Y 2015 Nucl. Fusion 55 092003
Google Scholar
[13] Kobayashi R, Hattori T, Tamura T, Ogata S 2015 J. Nucl. Mater. 463 1071
Google Scholar
[14] Sandoval L, Perez D, Uberuaga B P, Voter A F 2015 Phys. Rev. Lett. 114 105502
Google Scholar
[15] Yang S T, Hu N W, Gou X Q, Wang C L, Zhu X L 2016 RCS Advances 64 59875
[16] Yang L, Deng H Q, Gao F, Heinisch H L, Kurtz R J, Hu S Y, Li Y L, Zu X T 2013 Nucl. Instrum. Methods B 303 68
Google Scholar
[17] Xie H X, Gao N, Xu K, Lu G H, Yue T, Yin F X 2017 Acta Mater. 141 10
Google Scholar
[18] Liu X Y, Uberuaga B P, Perez D, Voter A F 2018 Mater. Res. Lett. 9 522
[19] Yang L, Gao F, Kurtz R J, Zu X T, Peng S M, Long X G, Zhou X S 2015 Acta Mater. 97 86
Google Scholar
[20] Zhao Q, Zhang Z, Li Y, Ouyang X 2017 Sci. Technol. Nucl. Ins. 2017 1
[21] Plimpton S 1995 J. Comput. Phys. 117 1
Google Scholar
[22] Stukowski A 2010 Modell. Simul. Mater. Sci. Eng. 18 015012
Google Scholar
[23] Ackland G J, Thetford R 1987 Philos. Mag. A 56 15
[24] Beck D E 1968 Mol. Phys. 14 311
Google Scholar
[25] Juslin N, Wirth B D 2013 J. Nucl. Mater. 432 61
Google Scholar
[26] Ziegler J F, Biersack J P, Littmark U 1985 The Stopping and Range of Ions in Matter (Vol. 1) (New York: Pergamon Press) p93
[27] Stukowski A, Albe K 2010 Modell. Simul. Mater. Sci. Eng. 18 085001
Google Scholar
[28] Guo S H, Zhu B E, Liu W C, Pan Z Y, Wang Y X 2009 Nucl. Instrum. Methods B 267 3278
Google Scholar
[29] Yang L, Zu Z Q, Peng S M, Long X G, Zhou X S, Zu X T, Heinisch H L, Kurtz R J, Gao F 2013 J. Nucl. Mater. 441 6
Google Scholar
[30] Rycroft C H, Grest G S, Landry J W, Bazant M Z 2006 Phys. Rev. E 74 021306
Google Scholar
[31] Chen L, Liu Y L, Zhou H B, Jin S, Zhang Y, Lu G H 2012 Sci. Chin. Phys. Mech. 55 614
Google Scholar
[32] He W H, Gao X, Gao N, Wang J, Wang D, Cui M H, Pang L L, Wang Z G 2018 Chin. Phys. Lett. 35 49
[33] Banisalman M J, Oda T 2019 Comput. Mater. Sci. 158 346
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 9218
- PDF Downloads: 187
- Cited By: 0
