-
With the rapid development of modern technology, high-precision absolute distance measurement is playing an important role in many applications, such as scientific research, aviation and industry measurement. Among the above various measurement methods, how to realize higher-accuracy, larger-scale, and faster-speed measurement is particularly important. In the traditional technique for long-distance measurement, the emergence of optical frequency comb (OFC) provides a breakthrough technology for accurately measuring the absolute value of distance. The OFC can be considered as a multi-wavelength source,whose phase and repetition rate are locked. The OFC is a very useful light source that can provide phase-coherent link between microwave and optical domain, which has been used as a source in various distance measurement schemes that can reach an extraordinary measurement precision and accuracy. A variety of laser ranging methods such as dual-comb interferometry and dispersive interferometer based on femtosecond laser have been applied to the measuring of absolute distance. In this paper, the factors affecting the resolution and the non-ambiguous range of spectral interferometry ranging using OFC are particularly discussed. We also analyze the systematic errors and the limitations of traditional transform methods based on Fourier transform, which can conduce to the subsequent research. To address the problem caused by low resolution and unequal frequency interval, we propose a data processing method referred to as equal frequency interval resampling. The proposed method is based on cubic spline interpolation and can solve the error caused by the frequency spectrum broadening with the increase of distance. Moreover, we propose a new method based on least square fitting to calibrate the error introduced by the low resolution of interferometry spectrum obtained with fast Fourier transform (FFT). With the proposed method, the simulation results show that the systematic error is less than 0.2 μm in the non-ambiguity range and the system resolution is greatly improved. Finally, anabsolute distance measurement system based on Michelson interferometer is built to verify theproposed method. The measurement results compared with those obtained by using a high-precision commercial He-Ne laser interferometer show that the distance measurement accuracy is lower than 3 μm at any distancewithin the non-ambiguity range. The experimental results demonstrate that our data processing algorithm is able to increase the accuracy of dispersive interferometry ranging with OFC. -
Keywords:
- optical frequency comb /
- frequency domain interferometer /
- spectral interferometry /
- absolute distance measurement
[1] Trocha P, Karpov M, Ganin D, Pfeiffer M H P, Kordts A, Wolf S, Krockenberger J, Marin-Palomo P, Weimann C, Randel S, Freude W, Kippenberg T J, Koos C 2018 Science 359 887
Google Scholar
[2] 张继涛, 吴学健, 李岩, 尉昊赟 2012 61 100601
Google Scholar
Zhang J T, Wu X J, Li Y, Wei H Y 2012 Acta Phys. Sin. 61 100601
Google Scholar
[3] 邢书剑, 张福民, 曹士英, 王高文, 曲兴华 2013 62 170603
Google Scholar
Xing S J, Zhang F M, Cao S Y, Wang G W, Qu X H 2013 Acta Phys. Sin. 62 170603
Google Scholar
[4] 吴学健, 李岩, 尉昊赟, 张继涛 2012 激光与光电子学进展 49 5
Wu X J, Li Y, Wei H Y, Zhang J T 2012 Laser Optoelectron. Prog. 49 5
[5] Eckstein J N, Ferguson A I, Hänsch T W 1978 Phys. Rev. Lett. 40 847
Google Scholar
[6] Minoshima K, Matsumoto H 2000 Appl. Opt. 39 5512
Google Scholar
[7] Minoshima K, Arai K, Inaba H 2011 Opt. Express 19 26095
Google Scholar
[8] 刘亭洋, 张福民, 吴翰钟, 李建双, 石永强, 曲兴华 2016 65 020601
Google Scholar
Liu T Y, Zhang F M, Wu H Z, Li J S, Shi Y Q, Qu X H 2016 Acta Phys. Sin. 65 020601
Google Scholar
[9] Joo K N, Kim S W 2006 Opt. Express 14 5954
Google Scholar
[10] Joo K N, Kim Y, Kim S W 2008 Opt. Express 16 19799
Google Scholar
[11] 周维虎, 石俊凯, 纪荣祎, 黎尧, 刘娅 2017 仪器仪表学报 38 1859
Google Scholar
Zhou W H, Shi J K, Ji R Y, Li Y, Liu Y 2017 J. Sci. Instrum. 38 1859
Google Scholar
[12] Yang R T, Florian P, Karl M H, Michael K, Tan J B, Harald B 2015 Meas. Sci. Technol. 26 084001
Google Scholar
[13] Cui M, Zeitouny M G, Bhattacharya N, van den Berg S A, Urbach H P 2011 Opt. Express 19 6549
Google Scholar
[14] Cui M, Zeitouny M G, Bhattacharya N, van den Berg S A, Urbach H P, Braat J J M 2009 Opt. Lett. 34 1982
Google Scholar
[15] 安慰宁, 张福民, 吴翰钟, 曲兴华 2014 仪器仪表学报 35 2458
An W N, Zhang F M, Wu H Z, Qu X H 2014 J. Sci. Instrum. 35 2458
[16] 吴翰钟, 曹士英, 张福民, 曲兴华 2015 64 020601
Google Scholar
Wu H Z, Cao S Y, Zhang F M, Qu X H 2015 Acta Phys. Sin. 64 020601
Google Scholar
[17] Lee J, Kim Y J, Lee K, Lee S, Kim S W 2010 Nat. Photon. 4 716
Google Scholar
[18] Ye J 2004 Opt. Lett. 29 1153
Google Scholar
[19] 李岩 2017 仪器仪表学报 38 1841
Google Scholar
Li Y 2017 J. Sci. Instrum. 38 1841
Google Scholar
[20] 王国超, 颜树华, 杨俊, 林存宝, 杨东兴, 邹鹏飞 2013 62 070601
Google Scholar
Wang G C, Yan S H, Yang J, Lin C B, Yang D X, Zou P F 2013 Acta Phys. Sin. 62 070601
Google Scholar
[21] 朱敏昊, 吴学健, 尉昊赟, 张丽琼, 张继涛, 李岩 2013 62 070702
Google Scholar
Zhu M H, Wu X J, Wei H Y, Zhang L Q, Zhang J T, Li Y 2013 Acta Phys. Sin. 62 070702
Google Scholar
[22] Lu Z Z, Wang W Q, Zhang W F, Liu M L, Wang L R, Chu S T, Little B E, Zhao J G, Xie P, Wang X Y, Zhao W 2018 Opt. Mater. Express 8 2662
Google Scholar
-
图 1 光频梳频谱
Figure 1. Spectrum of optical frequency comb
图 2 (a)频域干涉法原理图; (b) 测距系统实物图
Figure 2. (a) Principle of frequency domain interference method; (b) the experimental setup of the measurement system
图 3 实测距离与被测距离关系
Figure 3. Relationship between actual distance and measured distance
图 4 传统FFT法脉冲包络展宽现象 (a)
$\tau = 3.3 \times {10^{ - 12}}$ ; (b)$\tau = 7.5 \times {10^{ - 11}}$ Figure 4. Broadening of the pulse envelope using original FFT: (a)
$\tau = 3.3 \times {10^{ - 12}}$ ; (b)$\tau = 7.5 \times {10^{ - 11}}$ .
图 5 等频率间隔重采样原理
Figure 5. Principle of equal frequency interval resampling
图 6 传统FFT法结果重复和跳变
Figure 6. Repetition and hopping of the measurement result
图 7 当τ = 3 × 10–11时, 仿真效果对比 (a) 等频率间隔重采样; (b) 峰值位置拟合
Figure 7. Comparison of simulation effect when τ = 3 × 10–11: (a) Equal frequency interval resampling; (b) peak position fitting
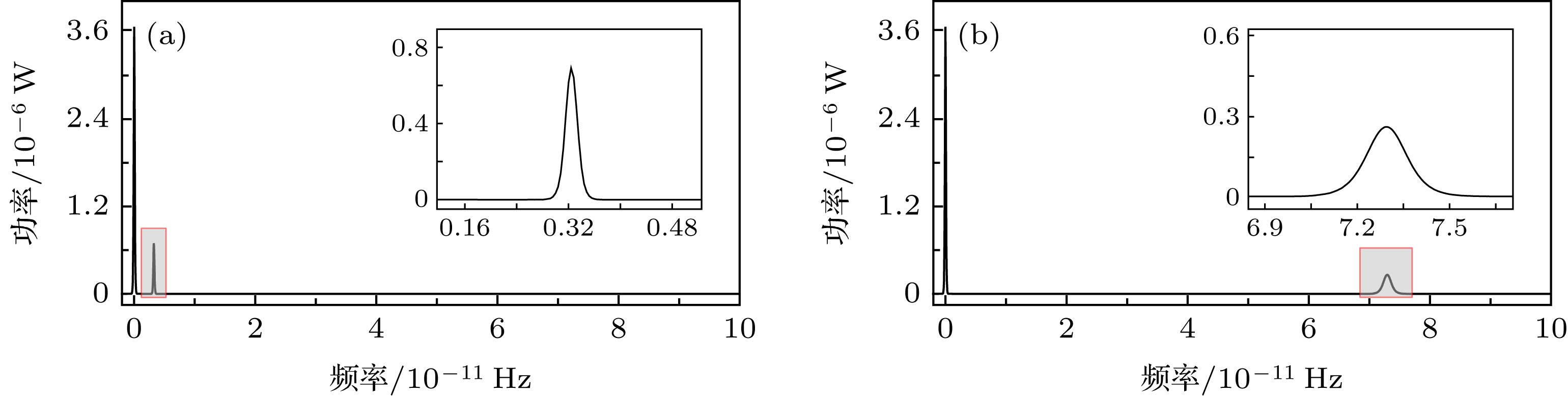
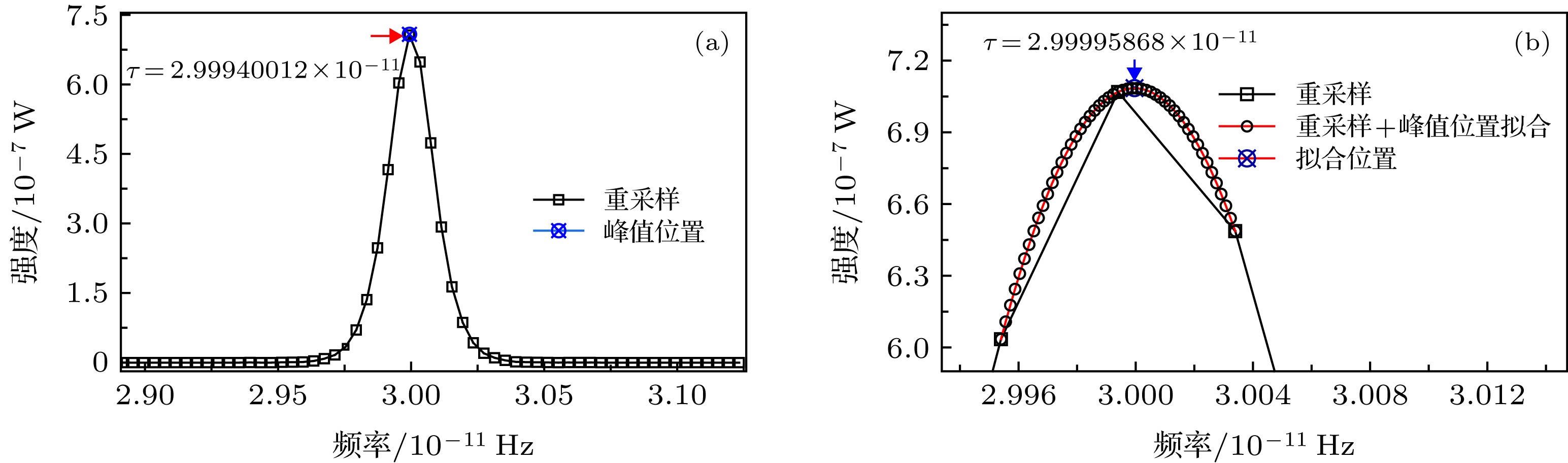
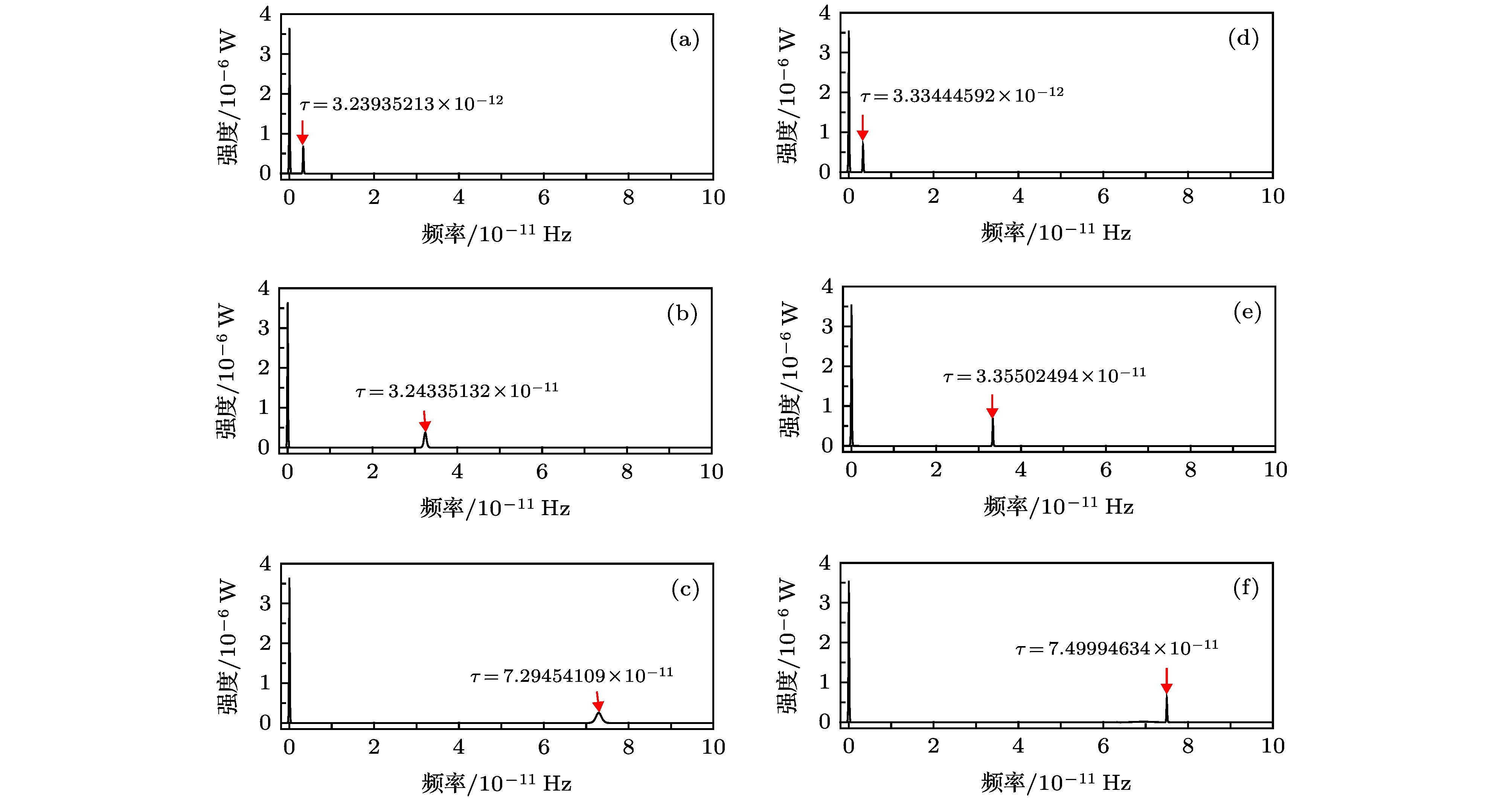
图 8 峰值定位仿真传统FFT (a) 3.335 × 10–12, (b) 3.335 × 10–11, (c) 7.500 × 10–11; 等频率间隔重采样和峰值位置拟合(d) 3.335 × 10–12, (e) 3.335 × 10–11, (f) 7.500 × 10–11
Figure 8. Peak position fitting simulation. Original FFT: (a) 3.335 × 10–12, (b) 3.335 × 10–11, (c) 7.500 × 10–11. Equal frequency interval resampling and peak position fitting (d) 3.335 × 10–12, (e) 3.335 × 10–11, (f) 7.500 × 10–11
图 9 传统FFT、等频率间隔重采样和峰值位置拟合法仿真误差比较
Figure 9. Simulation error of three methods
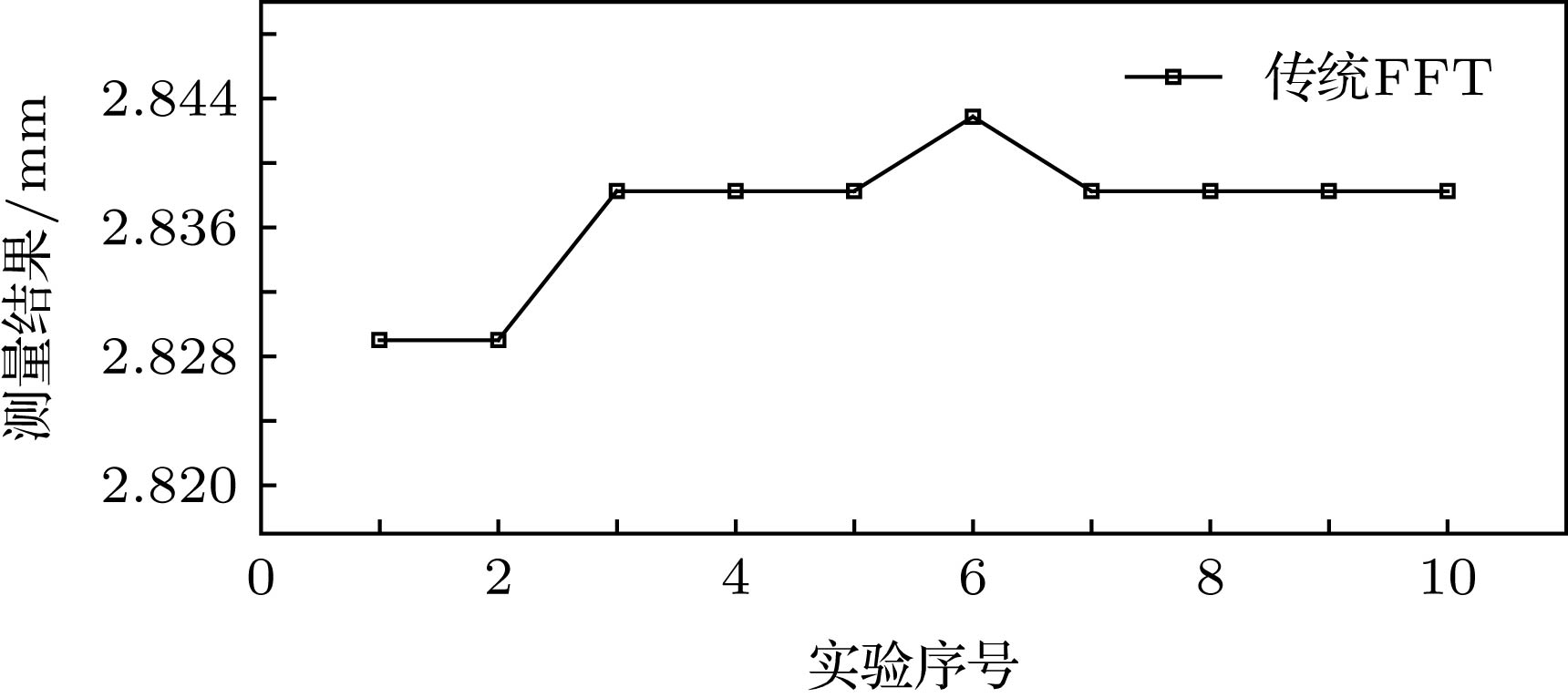
图 10 峰值定位效果对比仅传统FFT (a) L = 5.8600 mm, (b) L = 16.9850 mm, (c) L = 27.9100 mm; 等频率间隔重采样和峰值位置拟合(d) L = 5.8600 mm, (e) L = 16.9850 mm, (f) L = 27.9100 mm
Figure 10. Effect contrast of peak position fitting. Original FFT: (a) L = 5.8600 mm, (b) L = 16.9850 mm, (c) L = 27.9100 mm. Equal frequency interval resampling and peak position fitting: (d) L = 5.8600 mm, (e) L = 16.9850 mm, (f) L = 27.9100 mm
图 11 传统FFT、等频率间隔重采样和峰值位置拟合法分辨力修正效果对比 (a) 0.4997 mm; (b) 0.9998 mm; (c) 4.9980 mm
Figure 11. Correction of repetition result by three methods: (a) 0.4997 mm; (b) 0.9998 mm; (c) 4.9980 mm
表 1 传统FFT、等频率间隔重采样和峰值位置拟合法仿真结果误差比较
Table 1. Simulation error comparison of three methods.
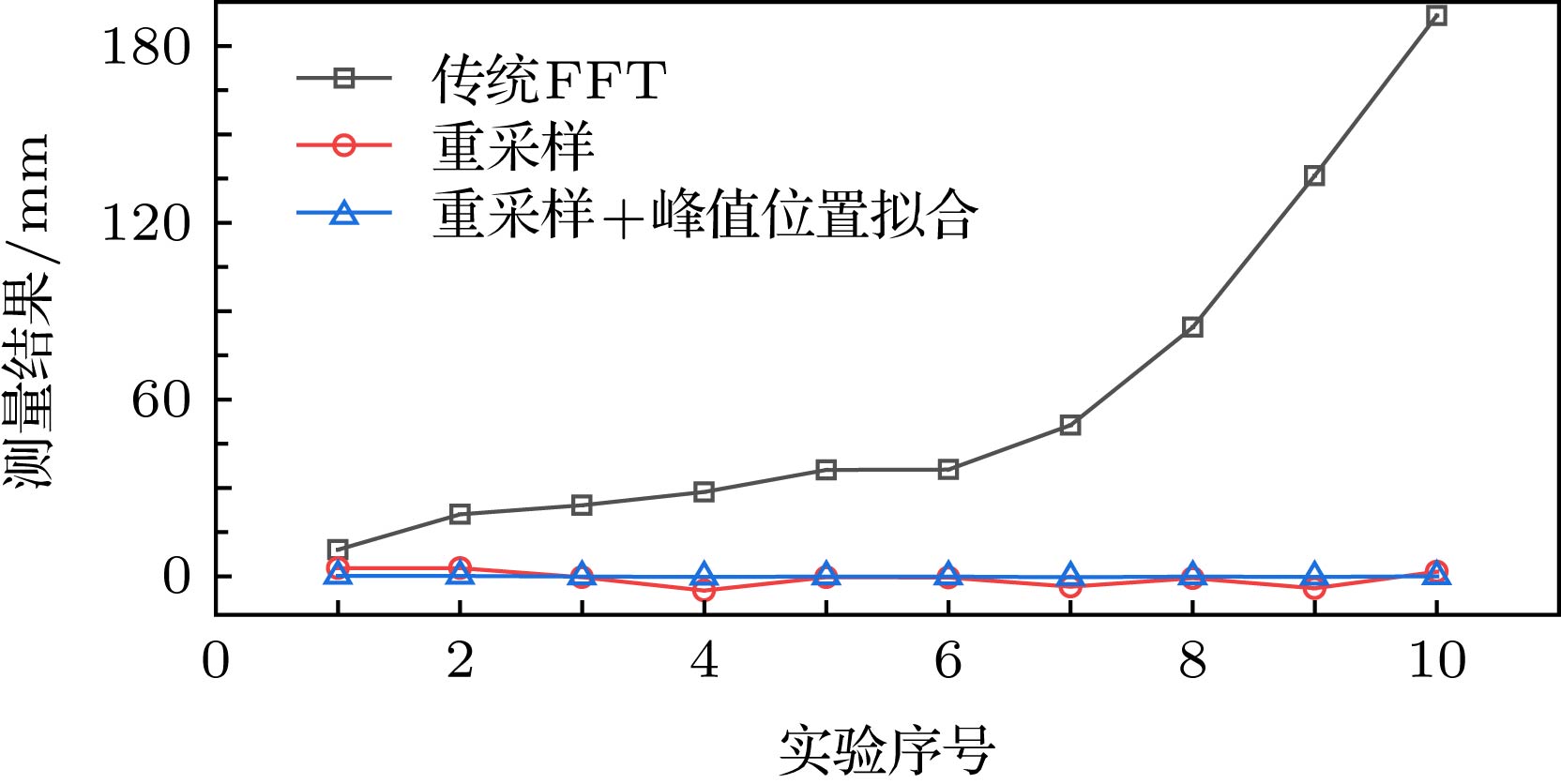
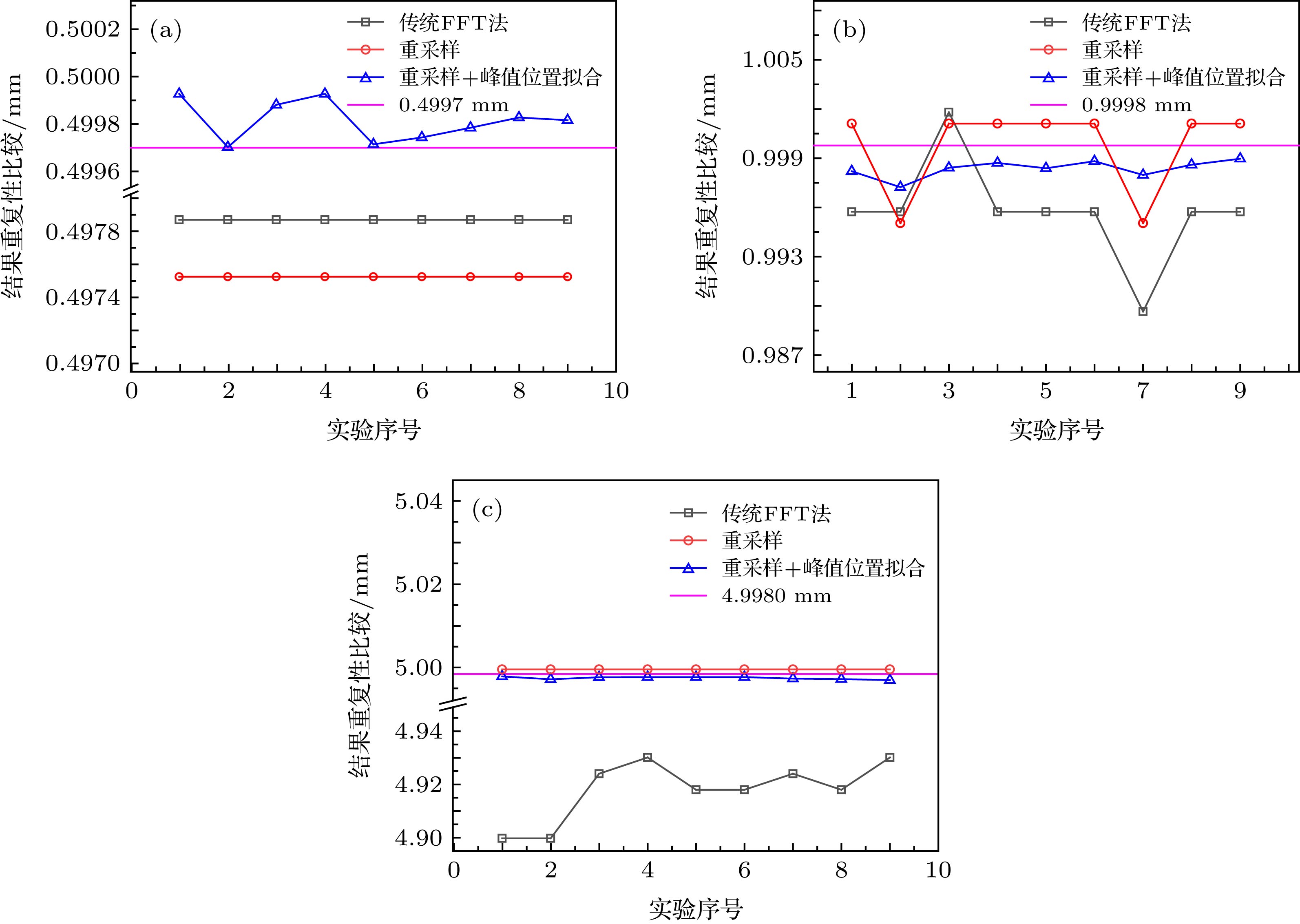
实验序号 L/mm 传统FFT法误差/μm 等频率间隔重采样误差/μm 峰值拟合误差/μm 1 0.5250 9.1031 2.8944 0.161990 2 0.8250 21.1607 2.8344 0.165549 3 0.9000 24.1751 –0.1799 0.010804 4 1.0005 28.6943 –4.6991 –0.166222 5 1.2000 36.2327 –0.239 0.000102 6 1.5000 36.2927 –0.2999 –0.008146 7 1.9950 51.3887 –3.3983 –0.183495 8 3.0000 84.5830 –0.5998 –0.038114 9 4.9950 135.9718 –3.998 –0.177303 10 7.0050 190.3629 1.5986 0.114618 
DownLoad: CSV
表 2 传统FFT、等频率间隔重采样和峰值位置拟合法误差比较
Table 2. Measurement results of different distance.
实验序号 L/mm 传统FFT法误差/μm 等频率间隔重采样误差/μm 峰值拟合误差/μm 1 0.0023 –2.3015 1.6570 0.0218 2 0.0037 –3.6001 3.0337 –0.7335 3 0.1000 3.2167 0.1031 0.8437 4 0.4997 –1.8309 0.4975 0.2267 5 0.9998 2.0097 1.0011 –0.9828 6 3.1307 –4.2793 3.1336 1.1003 7 4.9980 –13.2375 4.9995 –0.1739 8 6.2364 157.3300 6.2308 –1.9198 9 6.2511 无法定位 6.2551 –2.9791 10 9.3629 无法定位 1.5705 –2.1087
DownLoad: CSV
表 A1 文章参数表
Table A1. Parameter list
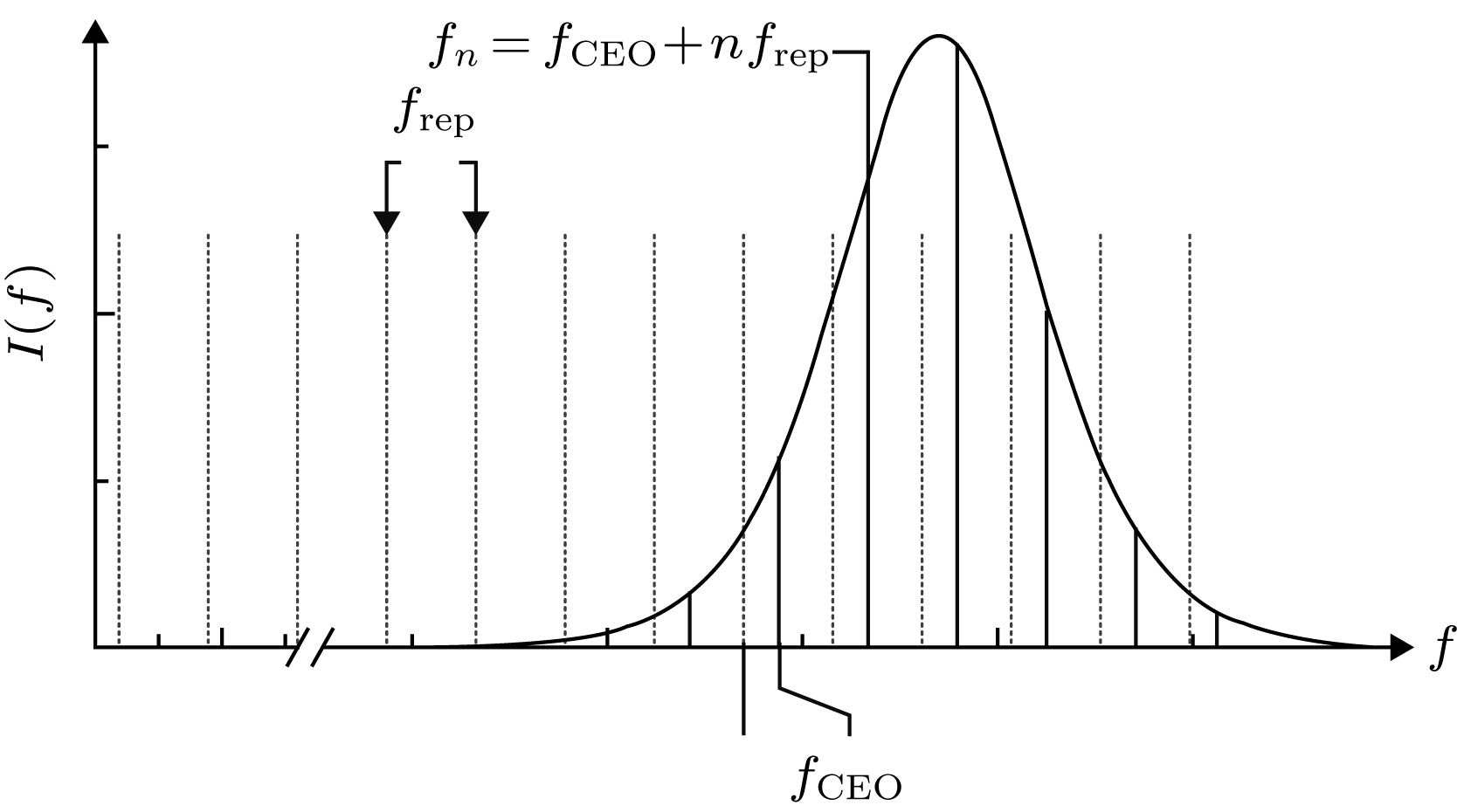
${f_{{\rm{CEO}}}}$ 光频梳偏移频率 ${f_{{\rm{rep}}}}$ 光频梳重复频率 ${T_{\rm{R}}}$ 光频梳脉冲时域间隔 $\Delta {\varphi _{{\rm{ce}}}}$ 群、相速度差异造成的相位偏移 $E\left( \upsilon \right)$ 光频梳脉冲电场信号 ${E_{{\rm{ref}}}}(\upsilon )$ 参考光电场信号 ${E_{\rm{t}}}(\upsilon )$ 测量光电场信号 a 参考光功率因数 b 测量光功率因数 $I(\upsilon )$ 光谱仪接收的频域干涉信号 ${{2ab}/ {{a^2} + {b^2}}}$ 调制深度 $I(t)$ 经FFT变换后的$I(\upsilon )$ L 测量臂和参考臂光程差/2 $\Delta t$ 2L造成时间差 τ 干涉信号振荡频率τ = L/c c 真空光速 n 折射率 ${L_{\rm{c}}}$ 相干长度 $\partial f$ 相干长度公式中的频率带宽 $\Delta \upsilon $ FFT变换的频率分辨力 $\Delta L$ FFT变换的距离分辨力=$\Delta \upsilon $*c ${L_{{\rm{NAR}}}}$ 频域干涉法的非模糊范围 f 频率 ${\rm{d}}f$ 光谱仪频率分辨力 ${\rm{d}}\lambda $ 光谱仪波长微分量 W 频谱范围 $\Delta w$ 波长范围上下限之差 B 频谱宽度 ${\lambda _{{\rm{cen}}}}$ W的中心处波长 ${f_{\rm{s}}}$ 光谱仪采样频率 $\Delta \lambda $ 光谱仪采样波长间隔 N 光谱仪采样点数 ${L_{{\rm{NAR0}}}}$ ${f_{{\rm{rep}}}} = 250{\rm{ MHz}}$理想情况下非模糊范围 ${L_{{\rm{NAR1}}}}$ 光谱仪的非模糊范围 ${L_{{\rm{NAR2}}}}$ ${f_{{\rm{rep}}}} = 40{\rm{ GHz}}$非模糊范围 $\Delta f$ 波长需转化为频率时的频率变化量 ${\lambda _1}$ 波长需转化为频率时的对应波长 $p\left( x \right)$ 二项式拟合公式 ${p_1}$ 二项式拟合二次项 ${p_2}$ 二项式拟合一次项 ${p_3}$ 二项式拟合常数项
DownLoad: CSV
-
[1] Trocha P, Karpov M, Ganin D, Pfeiffer M H P, Kordts A, Wolf S, Krockenberger J, Marin-Palomo P, Weimann C, Randel S, Freude W, Kippenberg T J, Koos C 2018 Science 359 887
Google Scholar
[2] 张继涛, 吴学健, 李岩, 尉昊赟 2012 61 100601
Google Scholar
Zhang J T, Wu X J, Li Y, Wei H Y 2012 Acta Phys. Sin. 61 100601
Google Scholar
[3] 邢书剑, 张福民, 曹士英, 王高文, 曲兴华 2013 62 170603
Google Scholar
Xing S J, Zhang F M, Cao S Y, Wang G W, Qu X H 2013 Acta Phys. Sin. 62 170603
Google Scholar
[4] 吴学健, 李岩, 尉昊赟, 张继涛 2012 激光与光电子学进展 49 5
Wu X J, Li Y, Wei H Y, Zhang J T 2012 Laser Optoelectron. Prog. 49 5
[5] Eckstein J N, Ferguson A I, Hänsch T W 1978 Phys. Rev. Lett. 40 847
Google Scholar
[6] Minoshima K, Matsumoto H 2000 Appl. Opt. 39 5512
Google Scholar
[7] Minoshima K, Arai K, Inaba H 2011 Opt. Express 19 26095
Google Scholar
[8] 刘亭洋, 张福民, 吴翰钟, 李建双, 石永强, 曲兴华 2016 65 020601
Google Scholar
Liu T Y, Zhang F M, Wu H Z, Li J S, Shi Y Q, Qu X H 2016 Acta Phys. Sin. 65 020601
Google Scholar
[9] Joo K N, Kim S W 2006 Opt. Express 14 5954
Google Scholar
[10] Joo K N, Kim Y, Kim S W 2008 Opt. Express 16 19799
Google Scholar
[11] 周维虎, 石俊凯, 纪荣祎, 黎尧, 刘娅 2017 仪器仪表学报 38 1859
Google Scholar
Zhou W H, Shi J K, Ji R Y, Li Y, Liu Y 2017 J. Sci. Instrum. 38 1859
Google Scholar
[12] Yang R T, Florian P, Karl M H, Michael K, Tan J B, Harald B 2015 Meas. Sci. Technol. 26 084001
Google Scholar
[13] Cui M, Zeitouny M G, Bhattacharya N, van den Berg S A, Urbach H P 2011 Opt. Express 19 6549
Google Scholar
[14] Cui M, Zeitouny M G, Bhattacharya N, van den Berg S A, Urbach H P, Braat J J M 2009 Opt. Lett. 34 1982
Google Scholar
[15] 安慰宁, 张福民, 吴翰钟, 曲兴华 2014 仪器仪表学报 35 2458
An W N, Zhang F M, Wu H Z, Qu X H 2014 J. Sci. Instrum. 35 2458
[16] 吴翰钟, 曹士英, 张福民, 曲兴华 2015 64 020601
Google Scholar
Wu H Z, Cao S Y, Zhang F M, Qu X H 2015 Acta Phys. Sin. 64 020601
Google Scholar
[17] Lee J, Kim Y J, Lee K, Lee S, Kim S W 2010 Nat. Photon. 4 716
Google Scholar
[18] Ye J 2004 Opt. Lett. 29 1153
Google Scholar
[19] 李岩 2017 仪器仪表学报 38 1841
Google Scholar
Li Y 2017 J. Sci. Instrum. 38 1841
Google Scholar
[20] 王国超, 颜树华, 杨俊, 林存宝, 杨东兴, 邹鹏飞 2013 62 070601
Google Scholar
Wang G C, Yan S H, Yang J, Lin C B, Yang D X, Zou P F 2013 Acta Phys. Sin. 62 070601
Google Scholar
[21] 朱敏昊, 吴学健, 尉昊赟, 张丽琼, 张继涛, 李岩 2013 62 070702
Google Scholar
Zhu M H, Wu X J, Wei H Y, Zhang L Q, Zhang J T, Li Y 2013 Acta Phys. Sin. 62 070702
Google Scholar
[22] Lu Z Z, Wang W Q, Zhang W F, Liu M L, Wang L R, Chu S T, Little B E, Zhao J G, Xie P, Wang X Y, Zhao W 2018 Opt. Mater. Express 8 2662
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 12071
- PDF Downloads: 225
- Cited By: 0
