高压下缺陷对锐钛矿相TiO<sub>2</sub>多晶电输运性能的影响: 交流阻抗测量

王月; 邵渤淮; 陈双龙; 王春杰; 高春晓

doi:10.7498/aps.72.20230020

摘要

采用高压原位阻抗谱测量技术对锐钛矿TiO₂多晶的电输运性质进行了系统研究. 在6.4, 11.5和24.6 GPa压力处发现了晶粒和晶界的电阻、参数因子和弛豫频率的反常变化行为. 研究分析表明: 6.4和11.5 GPa压力点分别对应着TiO₂由锐钛矿转变为α-PbO₂, 再转变为斜锆石的结构相变, 当压力高于24.6 GPa时, TiO₂完全转变为斜锆石相. 通过分析晶粒和晶界电阻在压力作用下的变化行为可知, 本征缺陷的存在对TiO₂高压下电输运性质的变化起着关键的作用. 在6.4 GPa压力处, 相变的发生导致缺陷的作用发生了变化, 由作为复合中心的深能级缺陷转变为向导带和价带提供载流子的浅能级缺陷, 并且作为浅能级缺陷存在至实验最高压力点38.9 GPa, 浅能级缺陷在能带中的位置也随着相变发生而改变. 晶粒和晶界的激活能随着压力升高而降低, 表明高压下载流子在晶粒和晶界的输运变得更加容易. 此外, TiO₂晶粒和晶界的弛豫频率比值随压力的升高而不断减小, 高压下的晶界效应不明显.

关键词:

高压 /
TiO₂ /
缺陷 /
晶界

Abstract

The electrical transport properties of anatase TiO₂ polycrystalline have been systematically investigated by using high pressure in-situ impedance spectroscopy measurements. The anomalous behaviors of resistance, parameter factor and relaxation frequency of grain and grain boundary can be found at 6.4, 11.5 and 24.6 GPa. The results indicate that the first two discontinuous points (6.4 and 11.5 GPa) correspond to the phase transitions of TiO₂ from anatase to α-PbO₂ and then to baddeleyite, respectively. Above 24.6 GPa, TiO₂ completely transforms into the baddeleyite phase. Based on the change of grain resistance and grain boundary resistance under pressure, intrinsic defects play a crucial effect in the electrical transport properties of TiO₂ at high pressures. At 6.4 GPa, the occurrence of phase transition gives rise to the variation of defects’ role, from a deep energy level defect (as a recombination centre) changes into a shallow energy level defect (providing carriers for the conduction and valence bands). In addition, the position of defect in energy band changes with pressure increasing. The phase transition of TiO₂ at 6.4 GPa is the rearrangement of TiO₆ octahedron, while the other one at 11.5 GPa can be attributed to the migration of oxygen Schottky defects from inner to surface. Combining the packing factor and relaxation frequency, the electrical transport properties of TiO₂ under pressure are revealed, the packing factor and the relaxation frequency are closely related to the mobility and the carrier concentration, respectively. The activation energy of grain and grain boundary decrease with the pressure elevating, indicating that the transport of carriers in grain and grain boundary become easier under pressure, and the former is smoother than the latter owing to the activation energy of grain being smaller than that of grain boundary in the same pressure range. Moreover, the relaxation frequency ratio of TiO₂ grain and TiO₂ grain boundary decreases with pressure increasing, and the grain boundary effect under high pressure is not obvious.

Keywords:

作者及机构信息

1.
渤海大学物理科学与技术学院, 锦州　121013

2.
吉林大学, 超硬材料国家重点实验室, 长春　130012

通信作者: 王春杰, cjwang@foxmail.com

基金项目: 国家自然科学基金(批准号: 12004050)和辽宁省教育厅项目(批准号: LJKMZ20221493)资助的课题.

Authors and contacts

1.
College of Physical Science and Technology, Bohai University, Jinzhou 121013, China

2.
State Key Laboratory of Superhard Materials, Jilin University, Changchun 130012, China

Corresponding author: Wang Chun-Jie, cjwang@foxmail.com

Funds: Project supported by the National Natural Science Foundation of China (Grant No. 12004050) and the Research Foundation of the Education Department of Liaoning Province, China (Grant No. LJKMZ20221493).

文章全文 : translate this paragraph

参考文献

[1]	Augustynski J 1993 Electrochim. Acta. 38 43 Google Scholar
[2]	Pezhooli N, Rahimi J, Hasti F, Maleki A 2022 Sci. Rep. 12 9885 Google Scholar
[3]	Crossland E J W, Noel N, Sivaram V, Leijtens T, Alexander-Webber J A, Snaith H J 2013 Nature 495 215 Google Scholar
[4]	Reza K M, Kurny A, Gulshan F 2017 Appl. Water. Sci. 7 1569 Google Scholar
[5]	韩迪仪, 顾阳, 胡涛政, 董雯, 倪亚贤 2021 70 038103 Google Scholar Han D Y, Gu Y, Hu T Z, Dong W, Ni Y X 2021 Acta Phys. Sin. 70 038103 Google Scholar
[6]	Liu L, Chan J, Sham T K 2010 J. Phys. Chem. C 114 21353 Google Scholar
[7]	San-Miguel A 2006 Chem. Soc. Rev. 35 876 Google Scholar
[8]	Lu X, Yang W, Quan Z, Lin T, Bai L, Wang L, Huang F, Zhao Y 2014 J. Am. Chem. Soc. 136 419 Google Scholar
[9]	Dong Z, Xiao F, Zhao A, Liu L, Sham T K, Song Y 2016 RSC Adv. 6 76142 Google Scholar
[10]	Liu F, Dong Z H, Liu L L 2019 J. Phys.: Condens. Matter 31 395403 Google Scholar
[11]	Huang Y, Chen F, Li X, Yuan Y, Dong H, Samanta S, Zhang J, Yang K 2016 J. Appl. Phys. 119 184 Google Scholar
[12]	Dong Z H, Song Y 2015 Can. J. Chem. 93 165 Google Scholar
[13]	Ji T, Gao Y, Qin T, Yue D, Gao C 2021 J. Phys. Chem. C 125 3364 Google Scholar
[14]	Li Q J, Liu B B 2016 Chin. Phys. B 25 076107 Google Scholar
[15]	Hearne G R, Zhao J, Dawe A M, Pischedda V, Maaza M, Nieuwoudt M K, Kibasomba P, Nemraoui O, Comins J D, Witcomb M J 2004 Phys. Rev. B 70 134102 Google Scholar
[16]	Haines J, Leger J M 1993 Physica B 192 233 Google Scholar
[17]	Kurita S, Ohta S, Sekiya T 2002 High Pressure Res. 22 319 Google Scholar
[18]	Ohsaka T, Yamaoka S, Shimomura O 1979 Solid State Commun. 30 34 Google Scholar
[19]	Liu L G, Mernagh T P 1992 Eur. J. Mineral. 4 45 Google Scholar
[20]	Lagarec K, Desgreniers S 1995 Solid State Commun. 94 519 Google Scholar
[21]	Sekiya T, Ohta S, Kamei S, Hanakawa M, KuritaS 2001 J. Phys. Chem. Solids 62 717 Google Scholar
[22]	Arlt T, Bermejo M, Blanco M A, Gerward L, Jiang J Z, Olsen J S, Recio J M 2000 Phys. Rev. B 61 14414 Google Scholar
[23]	Swamy V, Dubrovinsky L S, Dubrovinskaia N A, Langenhorst F, Simionovici A S, Drakopoulos M, Dmitriev V, Weber H P 2005 Solid State Commun. 134 541 Google Scholar
[24]	Swamy V, Dubrovinsky L S, Dubrovinskaia N A, Simionovici A S, Drakopoulos M, Dmitriev V, Weber H P 2003 Solid State Commun. 125 111 Google Scholar
[25]	Swamy V, Kuznetsov A, Dubrovinsky L S, McMillan P F, Prakapenka V B, Shen G, Muddle B C 2006 Phys. Rev. Lett. 96 135702 Google Scholar
[26]	Li Q, Cheng B, Yang X, Liu R, Zou B 2013 J. Phys. Chem. C 117 8516 Google Scholar
[27]	Li Q, Cheng B, Tian B, Liu R, Liu B, Wang F, Chen Z, Zou B, Cui T, Liu B 2014 RSC Adv. 4 12873 Google Scholar
[28]	Wang Q, Wang X, Li J, Qin T, Sang D, Liu J, Ke F, Wang X, Li Y, Liu C 2021 J. Mater. Chem. C 9 4764 Google Scholar
[29]	Zhang H, Zhang G, Wang J, Wang Q, Liu C 2021 J. Alloys Compd. 857 157482 Google Scholar
[30]	Su N, Sun M, Wang Q, Jin J, Sui J, Liu C, Gao C 2021 J. Phys. Chem. C 125 2713 Google Scholar
[31]	王春杰, 王月, 高春晓 2020 69 147202 Google Scholar Wang C J, Wang Y, Gao C X 2020 Acta Phys. Sin. 69 147202 Google Scholar
[32]	Wang J, Zhang G, Liu H, Wang Q, Shen W, Yan Y, Liu C, Han Y, Gao C 2017 Appl. Phys. Lett. 111 031907 Google Scholar
[33]	Duan S, Wang Q, Zou B, Jiang J, Liu K, Zhang G, Zhang, Sang D, Xu Z, Geng Y, Li J, Wang X, Li Y, Liu C 2022 Appl. Phys. Lett. 121 263904 Google Scholar
[34]	Piermarini G J, Block S, Barnett J D, Forman R A, 1975 J. Appl. Phys. 46 2774 Google Scholar
[35]	曹楚南, 张鉴清 2002 电化学阻抗谱导论 (典藏版 1) (北京: 科学出版社) 第21页 Cao C N, Zhang J Q 2002 Introduction to Electrochemical Impedance Spectroscopy (Vol. 1) (Beijing: Science Press) p21 (in Chinese)
[36]	Guo X, Yoshino T 2013 Earth Planet. Sci. Lett. 369–370 239
[37]	Guo X, Yoshino T, Katayama I 2011 Phys. Earth Planet. Inter. 188 69 Google Scholar
[38]	Rahman A U, Rafiq M A, Maaz K, Karim S, Cho S O, Hasan M M 2012 J. Appl. Phys. 112 063718 Google Scholar
[39]	Ali H, Karim S, Rafiq M A, Maaz K, Ahmad M 2014 J. Alloys Compd. 612 64 Google Scholar
[40]	Rahman A U, Rafifiq M A, Hasan M M, Maaz K, Karim S, Cho S O 2013 J. Nanopart Res. 15 1703 Google Scholar
[41]	Liu J, Yan J, Shi Q, Dong H, Zhang J, Wang Z, Huang W, Chen B, Zhang H 2019 J. Phys. Chem. C 123 4094 Google Scholar
[42]	Ma X G, Liang P, Miao L, Bie S W, Zhang C K, Xu L, Jiang J J 2009 Phys. Status. Solidi. (b) 246 2132 Google Scholar
[43]	Sekiya T, Ohta S, Kurita S 2008 Int. J. Mod. Phys. B 15 3952
[44]	Zhu T, Gao S P 2014 J. Phys. Chem. C 118 11385 Google Scholar
[45]	Liu Q J, Zhang N C, Liu F S, Liu Z T 2014 Phys. Scr. 89 075703 Google Scholar
[46]	Lin X, Wu J, Lu X, Shan Z, Wang W, Huang F 2009 Phys. Chem. Chem. Phys. 11 10047 Google Scholar
[47]	Wu J, Huang F, Shan Z, Wang Y 2011 Dalton Trans. 40 6906 Google Scholar
[48]	Keyan H U, Zian X U, Liu Y, Huang F 2020 Chem. Res. Chin. Univ. 36 1102 Google Scholar
[49]	Plata J J, Márquez A M, Sanz J F 2013 J. Phys. Chem. C 117 14502 Google Scholar
[50]	Park N G, van de Lagemaa J, Frank A J 2000 J. Phys. Chem. B 104 8989 Google Scholar
[51]	刘恩科, 朱秉升, 罗晋生 2012 半导体物理学 (第7版) (北京: 电子工业出版社) 第109页 Liu E K, Zhu B S, Luo J S 2012 The Physics of Semiconductors (Vol. 7) (Beijing: Electronic Industry Press) p109 (in Chinese)
[52]	Ohta S, Sekiya T, Kurita S 2001 Phys. Status. Solidi. (b) 223 265 Google Scholar
[53]	Muscat J, Swamy V, Harrison N M 2002 Phys. Rev. B 65 224112 Google Scholar
[54]	Wang Q, Lian G, Dickey E C 2004 Acta Mater. 52 809 Google Scholar
[55]	Bharathi K K, Markandeyulu G, Ramana C V 2011 J. Electrochem. Soc. 158 G71 Google Scholar

施引文献

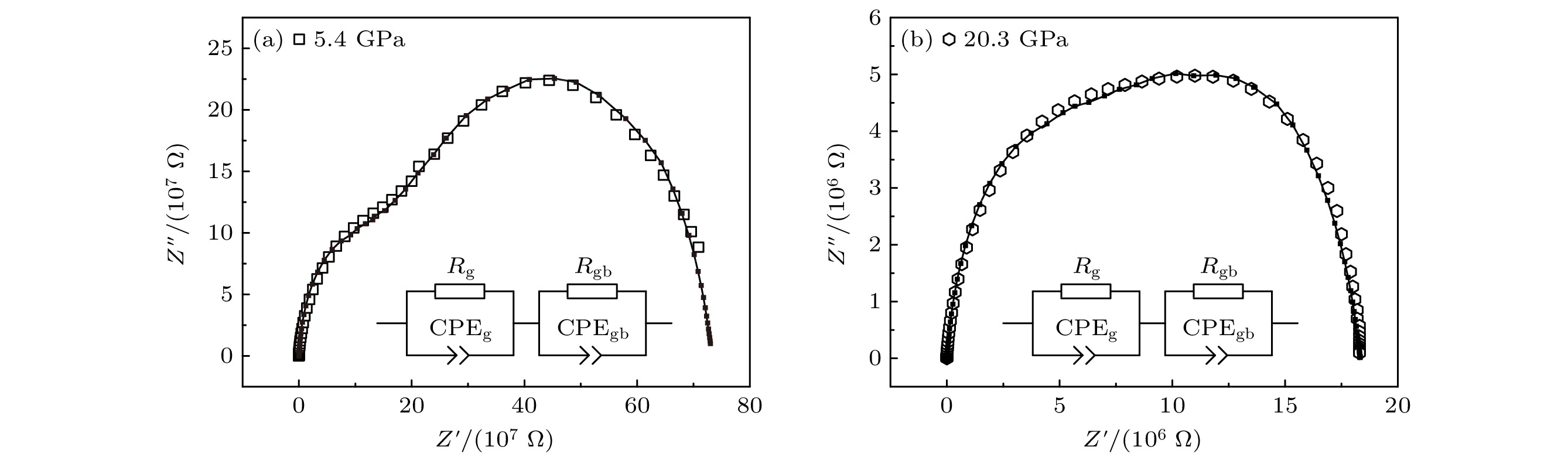
图 1 TiO₂在不同压力下的Nyquist谱图　(a) 4.9—7.6 GPa; (b) 7.6—13.0 GPa; (c) 13.0—20.3 GPa; (d) 20.3—24.6 GPa; (e) 24.6—30.1 GPa; (f) 30.1—38.9 GPa

Fig. 1. Nyquist impedance spectra of TiO₂ at different pressures: (a) 4.9–7.6 GPa; (b) 7.6–13.0 GPa; (c) 13.0–20.3 GPa; (d) 20.3–24.6 GPa; (e) 24.6–30.1 GPa; (f) 30.1–38.9 GPa.

下载: 全尺寸图片幻灯片

图 2 不同压力下TiO₂阻抗谱实验和拟合数据对比　(a) 5.4 GPa; (b) 20.3 GPa

Fig. 2. Experimental and fitting data for TiO₂ impedance spectra at different pressures: (a) 5.4 GPa; (b) 20.3 GPa.

下载: 全尺寸图片幻灯片

图 3 TiO₂参数因子随压力的变化关系

Fig. 3. Pressure dependence of parameter factor for TiO₂.

下载: 全尺寸图片幻灯片

图 4 TiO₂电阻随压力的变化关系

Fig. 4. Pressure dependence of resistance for TiO₂.

下载: 全尺寸图片幻灯片

图 5 TiO₂弛豫频率随压力的变化关系

Fig. 5. Pressure dependence of the relaxation frequency for TiO₂.

下载: 全尺寸图片幻灯片

图 6 不同压力区间TiO₂缺陷能级分布

Fig. 6. Distribution of TiO₂ defect energy levels in different pressure intervals.

下载: 全尺寸图片幻灯片

表 1 激活能随压力的变化关系

Table 1. Pressure dependence of activation energy.

Pressure region/GPa dE/dP/(meV·GPa^–1)
Grain Grain boundary

0—6.4 –11.51 –22.79
6.4—11.5 –7.59 –9.33
11.5—24.6 –6.16 –6.82
24.6—38.9 –3.54 –4.91

下载: 导出CSV

map

[1]	Augustynski J 1993 Electrochim. Acta. 38 43 Google Scholar
[2]	Pezhooli N, Rahimi J, Hasti F, Maleki A 2022 Sci. Rep. 12 9885 Google Scholar
[3]	Crossland E J W, Noel N, Sivaram V, Leijtens T, Alexander-Webber J A, Snaith H J 2013 Nature 495 215 Google Scholar
[4]	Reza K M, Kurny A, Gulshan F 2017 Appl. Water. Sci. 7 1569 Google Scholar
[5]	韩迪仪, 顾阳, 胡涛政, 董雯, 倪亚贤 2021 70 038103 Google Scholar Han D Y, Gu Y, Hu T Z, Dong W, Ni Y X 2021 Acta Phys. Sin. 70 038103 Google Scholar
[6]	Liu L, Chan J, Sham T K 2010 J. Phys. Chem. C 114 21353 Google Scholar
[7]	San-Miguel A 2006 Chem. Soc. Rev. 35 876 Google Scholar
[8]	Lu X, Yang W, Quan Z, Lin T, Bai L, Wang L, Huang F, Zhao Y 2014 J. Am. Chem. Soc. 136 419 Google Scholar
[9]	Dong Z, Xiao F, Zhao A, Liu L, Sham T K, Song Y 2016 RSC Adv. 6 76142 Google Scholar
[10]	Liu F, Dong Z H, Liu L L 2019 J. Phys.: Condens. Matter 31 395403 Google Scholar
[11]	Huang Y, Chen F, Li X, Yuan Y, Dong H, Samanta S, Zhang J, Yang K 2016 J. Appl. Phys. 119 184 Google Scholar
[12]	Dong Z H, Song Y 2015 Can. J. Chem. 93 165 Google Scholar
[13]	Ji T, Gao Y, Qin T, Yue D, Gao C 2021 J. Phys. Chem. C 125 3364 Google Scholar
[14]	Li Q J, Liu B B 2016 Chin. Phys. B 25 076107 Google Scholar
[15]	Hearne G R, Zhao J, Dawe A M, Pischedda V, Maaza M, Nieuwoudt M K, Kibasomba P, Nemraoui O, Comins J D, Witcomb M J 2004 Phys. Rev. B 70 134102 Google Scholar
[16]	Haines J, Leger J M 1993 Physica B 192 233 Google Scholar
[17]	Kurita S, Ohta S, Sekiya T 2002 High Pressure Res. 22 319 Google Scholar
[18]	Ohsaka T, Yamaoka S, Shimomura O 1979 Solid State Commun. 30 34 Google Scholar
[19]	Liu L G, Mernagh T P 1992 Eur. J. Mineral. 4 45 Google Scholar
[20]	Lagarec K, Desgreniers S 1995 Solid State Commun. 94 519 Google Scholar
[21]	Sekiya T, Ohta S, Kamei S, Hanakawa M, KuritaS 2001 J. Phys. Chem. Solids 62 717 Google Scholar
[22]	Arlt T, Bermejo M, Blanco M A, Gerward L, Jiang J Z, Olsen J S, Recio J M 2000 Phys. Rev. B 61 14414 Google Scholar
[23]	Swamy V, Dubrovinsky L S, Dubrovinskaia N A, Langenhorst F, Simionovici A S, Drakopoulos M, Dmitriev V, Weber H P 2005 Solid State Commun. 134 541 Google Scholar
[24]	Swamy V, Dubrovinsky L S, Dubrovinskaia N A, Simionovici A S, Drakopoulos M, Dmitriev V, Weber H P 2003 Solid State Commun. 125 111 Google Scholar
[25]	Swamy V, Kuznetsov A, Dubrovinsky L S, McMillan P F, Prakapenka V B, Shen G, Muddle B C 2006 Phys. Rev. Lett. 96 135702 Google Scholar
[26]	Li Q, Cheng B, Yang X, Liu R, Zou B 2013 J. Phys. Chem. C 117 8516 Google Scholar
[27]	Li Q, Cheng B, Tian B, Liu R, Liu B, Wang F, Chen Z, Zou B, Cui T, Liu B 2014 RSC Adv. 4 12873 Google Scholar
[28]	Wang Q, Wang X, Li J, Qin T, Sang D, Liu J, Ke F, Wang X, Li Y, Liu C 2021 J. Mater. Chem. C 9 4764 Google Scholar
[29]	Zhang H, Zhang G, Wang J, Wang Q, Liu C 2021 J. Alloys Compd. 857 157482 Google Scholar
[30]	Su N, Sun M, Wang Q, Jin J, Sui J, Liu C, Gao C 2021 J. Phys. Chem. C 125 2713 Google Scholar
[31]	王春杰, 王月, 高春晓 2020 69 147202 Google Scholar Wang C J, Wang Y, Gao C X 2020 Acta Phys. Sin. 69 147202 Google Scholar
[32]	Wang J, Zhang G, Liu H, Wang Q, Shen W, Yan Y, Liu C, Han Y, Gao C 2017 Appl. Phys. Lett. 111 031907 Google Scholar
[33]	Duan S, Wang Q, Zou B, Jiang J, Liu K, Zhang G, Zhang, Sang D, Xu Z, Geng Y, Li J, Wang X, Li Y, Liu C 2022 Appl. Phys. Lett. 121 263904 Google Scholar
[34]	Piermarini G J, Block S, Barnett J D, Forman R A, 1975 J. Appl. Phys. 46 2774 Google Scholar
[35]	曹楚南, 张鉴清 2002 电化学阻抗谱导论 (典藏版 1) (北京: 科学出版社) 第21页 Cao C N, Zhang J Q 2002 Introduction to Electrochemical Impedance Spectroscopy (Vol. 1) (Beijing: Science Press) p21 (in Chinese)
[36]	Guo X, Yoshino T 2013 Earth Planet. Sci. Lett. 369–370 239
[37]	Guo X, Yoshino T, Katayama I 2011 Phys. Earth Planet. Inter. 188 69 Google Scholar
[38]	Rahman A U, Rafiq M A, Maaz K, Karim S, Cho S O, Hasan M M 2012 J. Appl. Phys. 112 063718 Google Scholar
[39]	Ali H, Karim S, Rafiq M A, Maaz K, Ahmad M 2014 J. Alloys Compd. 612 64 Google Scholar
[40]	Rahman A U, Rafifiq M A, Hasan M M, Maaz K, Karim S, Cho S O 2013 J. Nanopart Res. 15 1703 Google Scholar
[41]	Liu J, Yan J, Shi Q, Dong H, Zhang J, Wang Z, Huang W, Chen B, Zhang H 2019 J. Phys. Chem. C 123 4094 Google Scholar
[42]	Ma X G, Liang P, Miao L, Bie S W, Zhang C K, Xu L, Jiang J J 2009 Phys. Status. Solidi. (b) 246 2132 Google Scholar
[43]	Sekiya T, Ohta S, Kurita S 2008 Int. J. Mod. Phys. B 15 3952
[44]	Zhu T, Gao S P 2014 J. Phys. Chem. C 118 11385 Google Scholar
[45]	Liu Q J, Zhang N C, Liu F S, Liu Z T 2014 Phys. Scr. 89 075703 Google Scholar
[46]	Lin X, Wu J, Lu X, Shan Z, Wang W, Huang F 2009 Phys. Chem. Chem. Phys. 11 10047 Google Scholar
[47]	Wu J, Huang F, Shan Z, Wang Y 2011 Dalton Trans. 40 6906 Google Scholar
[48]	Keyan H U, Zian X U, Liu Y, Huang F 2020 Chem. Res. Chin. Univ. 36 1102 Google Scholar
[49]	Plata J J, Márquez A M, Sanz J F 2013 J. Phys. Chem. C 117 14502 Google Scholar
[50]	Park N G, van de Lagemaa J, Frank A J 2000 J. Phys. Chem. B 104 8989 Google Scholar
[51]	刘恩科, 朱秉升, 罗晋生 2012 半导体物理学 (第7版) (北京: 电子工业出版社) 第109页 Liu E K, Zhu B S, Luo J S 2012 The Physics of Semiconductors (Vol. 7) (Beijing: Electronic Industry Press) p109 (in Chinese)
[52]	Ohta S, Sekiya T, Kurita S 2001 Phys. Status. Solidi. (b) 223 265 Google Scholar
[53]	Muscat J, Swamy V, Harrison N M 2002 Phys. Rev. B 65 224112 Google Scholar
[54]	Wang Q, Lian G, Dickey E C 2004 Acta Mater. 52 809 Google Scholar
[55]	Bharathi K K, Markandeyulu G, Ramana C V 2011 J. Electrochem. Soc. 158 G71 Google Scholar

[1]	陈贝, 邓永和, 祁青华, 高明, 文大东, 王小云, 彭平. 高压下快凝Pd₈₂Si₁₈非晶合金中二十面体结构分析. , 2024, 73(2): 026101. doi: 10.7498/aps.73.20231101
[2]	肖文悦, 董小硕, 买买提热夏提·买买提, 牛娜娜, 李国栋, 朱泽涛, 毕杰昊. Zn²⁺和TiO₂合金化过程中不同成分占比对薄膜结构和光催化性能的影响. , 2024, 73(18): 183301. doi: 10.7498/aps.73.20240814
[3]	王飞, 李全军, 胡阔, 刘冰冰. 高压导致纳米TiO₂形变的电子显微研究. , 2023, 72(3): 036201. doi: 10.7498/aps.72.20221656
[4]	李亚莎, 刘世冲, 刘清东, 夏宇, 胡豁然, 李光竹. 外电场下含有缔合缺陷的ZnO/${\boldsymbol{\beta }}$-Bi₂O₃界面电学性能. , 2022, 71(2): 026801. doi: 10.7498/aps.71.20210635
[5]	王月, 邵渤淮, 陈双龙, 王春杰, 高春晓. 高压下TiO₂纳米线晶粒和晶界性质及电输运行为. , 2022, 71(9): 096101. doi: 10.7498/aps.71.20212276
[6]	李亚莎, 刘世冲, 刘清东, 夏宇, 胡豁然, 李光竹. 外电场下含有缔合缺陷的ZnO/β-Bi2O3界面电学性能研究. , 2021, (): . doi: 10.7498/aps.70.20210635
[7]	高旭东, 杨得草, 魏雯静, 李公平. 电子束对ZnO和TiO₂辐照损伤的模拟计算. , 2021, 70(23): 234101. doi: 10.7498/aps.70.20211223
[8]	李鹏程, 唐重阳, 程亮, 胡永明, 肖湘衡, 陈万平. TiO₂纳米粉在水中通过摩擦还原CO₂. , 2021, 70(21): 214601. doi: 10.7498/aps.70.20210210
[9]	姚盼盼, 王玲瑞, 王家祥, 郭海中. 高压下非铅双钙钛矿Cs₂TeCl₆的结构和光学性质. , 2020, 69(21): 218801. doi: 10.7498/aps.69.20200988
[10]	王春杰, 王月, 高春晓. 高压下纳米晶ZnS晶粒和晶界性质及相变机理. , 2020, 69(14): 147202. doi: 10.7498/aps.69.20200240
[11]	王少霞, 赵旭才, 潘多桥, 庞国旺, 刘晨曦, 史蕾倩, 刘桂安, 雷博程, 黄以能, 张丽丽. 过渡金属(Cr, Mn, Fe, Co)掺杂对TiO₂磁性影响的第一性原理研究. , 2020, 69(19): 197101. doi: 10.7498/aps.69.20200644
[12]	王春杰, 王月, 高春晓. 高压下金红石相TiO₂的晶界电学性质. , 2019, 68(20): 206401. doi: 10.7498/aps.68.20190630
[13]	颜小珍, 邝小渝, 毛爱杰, 匡芳光, 王振华, 盛晓伟. 高压下ErNi2B2C弹性性质、电子结构和热力学性质的第一性原理研究. , 2013, 62(10): 107402. doi: 10.7498/aps.62.107402
[14]	张品亮, 龚自正, 姬广富, 刘崧. α-Ti2Zr高压物性的第一性原理计算研究. , 2013, 62(4): 046202. doi: 10.7498/aps.62.046202
[15]	吴迪, 赵纪军, 田华. Fe2+取代对MgSiO3钙钛矿高温高压物性的影响. , 2013, 62(4): 049101. doi: 10.7498/aps.62.049101
[16]	吕晓静, 翁春生, 李宁. 高压环境下1.58 μm波段CO2吸收光谱特性分析. , 2012, 61(23): 234205. doi: 10.7498/aps.61.234205
[17]	邓杨, 王如志, 徐利春, 房慧, 严辉. 立方(Ba0.5Sr0.5)TiO3高压诱导带隙变化的第一性原理研究. , 2011, 60(11): 117309. doi: 10.7498/aps.60.117309
[18]	冯志芳, 刘荣鹃, 李志远. 十二重准晶中的方向缺陷. , 2009, 58(3): 1948-1953. doi: 10.7498/aps.58.1948
[19]	邵光杰, 秦秀娟, 刘日平, 王文魁, 姚玉书. 氧化锌纳米晶高压下的晶粒演化和性能. , 2006, 55(1): 472-476. doi: 10.7498/aps.55.472
[20]	王海燕, 刘日平, 马明臻, 高明, 姚玉书, 王文魁. FeSi2合金在高压下的凝固. , 2004, 53(7): 2378-2383. doi: 10.7498/aps.53.2378

计量

文章访问数: 2972
PDF下载量: 101
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

搜索

留言板

高压下缺陷对锐钛矿相TiO₂多晶电输运性能的影响: 交流阻抗测量