-
理论研究了双层石墨烯薄膜体系中的四波混频特性. 计算结果表明: 通过调控声子-激子耦合强度、泵浦强度、激子-泵浦场失谐量的大小, 四波混频谱可以在两峰、三峰、四峰、五峰、六峰结构之间切换. 在弱激子-声子耦合情况下(即声子-激子耦合强度g < 激子退相速率Γ2), 四波混频谱中左/右峰的最大强度随泵浦强度的增大先增大后减弱. 在中间耦合(g = Γ2)和强耦合情况下(g >Γ2), 体系中的四波混频谱展现了呈对称性的两峰结构, 两峰的间距等于2g. 当g从1.0 THz增至4.0 THz时, 两峰的峰值都减小到原来的0.4%. 本研究不仅可以用来测量双层石墨烯体系中的声子-激子耦合强度, 而且有利于进一步探究双层石墨烯内部更深层的物理机理.Graphene thin films are often used to manufacture various optoelectronic nanodevices owing to their advantages such as light weight, small size, high quality factor, and good conductivity. So far, there have been few studies of the four-wave mixing characteristics in a bilayer graphene nanosystem, especially theoretical research. In this work, we study theoretically the four-wave mixing properties in a bilayer graphene nanosystem. Firstly, the analytical formula of the four-wave mixing signal is derived by quantum mechanical method, which is divided into three steps. 1) Total Hamiltonian of the system is written in the rotating wave approximation. 2) By using the Heisenberg equation of motion and the commutation relations between different operators, the corresponding density matrix equations are obtained. 3) To solve these density matrix equations, we make an ansatz and obtain the analytical formula of the four-wave mixing signal. Secondly, we explore the dependence of the four-wave mixing signal on the phonon-exciton coupling strength, pumping intensity and the detuning between the exciton and the pump field. The calculated results show that the lineshape of four-wave mixing spectrum can be switched among two-peaked, three-peaked, four-peaked, five-peaked and six-peaked by adjusting the phonon-exciton coupling strength, the pumping intensity, and the detuning between the exciton and the pump field. In a weak phonon-exciton coupling regime (i.e. phonon-exciton coupling strength g < dephasing rate of exciton Γ2), the intensity of the left peak and right peak of four-wave mixing signal first increase and then decrease with the increase of the pumping intensity
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ . In the intermediate and strong phonon-exciton coupling regime (i.e. g = Γ2 and g > Γ2), the four-wave mixing spectrum exhibits a two-peaked structure. The maximum values of these two peaks increase as$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ increases, and their spacing is equal to 2g. Especially, for a given pumping intensity$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ (= 10 THz2), the maximum value of the peak for g = 4 THz becomes 0.4% of that for g = 1 THz, indicating that the phonon-exciton coupling inhibits the enhancement of the four-wave mixing signal to a certain extent. Our findings can not only offer an efficient way to measure the phonon-exciton coupling strength in the bilayer graphene system, but also help one to further explore the underlying physical mechanism in such a nanosystem.-
Keywords:
- bilayer graphene /
- four-wave mixing /
- coupling
[1] Nair R R, Blake P, Grigorenko A N, Novoselov K S, Booth T J, Stauber T, Peres N M R, Geim A K 2008 Science 320 1308
Google Scholar
[2] Chen C, Rosenblatt S, Bolotin K I, Kalb W, Kim P, Kymissis I, Stormer H L, Heinz T F, Hone J 2009 Nat. Nanotechnol. 4 861
Google Scholar
[3] Jiang J W, Park H S, Rabczuk T 2012 Nanotechnology 23 475501
Google Scholar
[4] Xiang Y J, Dai X Y, Guo J, Wen S C, Tang D Y 2014 Appl. Phys. Lett. 104 051108
Google Scholar
[5] Yi Y, Chen Z, Yu X F, Zhou Z K, Li J 2019 Adv. Quantum Technol. 2 1800111
Google Scholar
[6] Zhang X J, Yuan Z H, Yang R X, He Y L, Qin Y L, Xiao S, He J 2019 J. Cent. South Univ. 26 2295
Google Scholar
[7] Tan Y, Xia X S, Liao X L, Li J B, Zhong H H, Liang S, Xiao S, Liu L H, Luo J H, He M D, Chen L Q 2020 Carbon 157 724
Google Scholar
[8] 王波, 张纪红, 李聪颖 2021 70 054207
Google Scholar
Wang B, Zhang J H, Li C Y 2021 Acta Phys. Sin. 70 054207
Google Scholar
[9] 郭晓蒙, 青芳竹, 李雪松 2021 70 098102
Google Scholar
Guo X M, Qing F Z, Li X S 2021 Acta Phys. Sin. 70 098102
Google Scholar
[10] Mayorov A S, Elias D C, Mucha-Kruczynski M, Gorbachev R V, Tudorovskiy T, Zhukov A, Morozov S V, Katsnelson M I, Fal’ko V I, Geim A K, Novoselov K S 2011 Science 333 860
Google Scholar
[11] Grigorenko A N, Polini M, Novoselov K S 2012 Nat. Photon. 6 749
Google Scholar
[12] Fei Z, Iwinski E G, Ni G X, Zhang L M, Bao W, Rodin A S, Lee Y, Wagner M, Liu M K, Dai S, Goldflam M D, Thiemens M, Keilmann F, Lau C N, Castro-Neto A H, Fogler M M, Basov D N 2015 Nano Lett. 15 4973
Google Scholar
[13] Zhao X J, Hou H, Fan X T, Wang Y, Liu Y M, Tang C, Liu S H, Ding P P, Cheng J, Lin D H, Wang C, Yang Y, Tan Y Z 2019 Nat. Commun. 10 3057
Google Scholar
[14] Xu S, Al Ezzi M M, Balakrishnan N, Garcia-Ruiz A, Tsim B, Mullan C, Barrier J, Xin N, Piot B A, Taniguchi T, Watanabe K, Carvalho A, Mishchenko A, Geim A K, Vladimir I. Fal’ko V I, Adam S, Castro Neto A H, Novoselov K S, Shi Y M 2021 Nat. Phys. 17 619
Google Scholar
[15] Malard L M, Nilsson J, Elias D C, Brant J C, Plentz F, Alves E S, Castro Neto A H, Pimenta M A 2007 Phys. Rev. B 76 201401(R
Google Scholar
[16] Tang T T, Zhang Y B, Park C H, Geng B S, Girit C, Hao Z, Martin M C, Zettl A, Crommie M F, Louie S G, Shen Y R, Wang F 2009 Nat. Nanotechnol. 5 32
Google Scholar
[17] Yang T Y, Balakrishnan J, Volmer F, Avsar A, Jaiswal M, Samm J, Ali S R, Pachoudeng A, Popinciuc M, Güntherodt G, Beschoten B 2011 Phys. Rev. Lett. 107 047206
Google Scholar
[18] Kou A, Feldman B E, Levin A J, Halperin B I, Watanabe K, Taniguchi T, Yacoby A 2014 Science 345 6192
Google Scholar
[19] Ki D K, Fal'ko V I, Abanin D A, Morpurgo A F 2014 Nano Lett. 14 2135
Google Scholar
[20] Da H X, Yan X H 2016 Opt. Lett. 41 151
Google Scholar
[21] Yu G, Wu Z, Zhan Z, Katsnelson M I, Yuan S J 2020 Phys. Rev. B 102 115123
Google Scholar
[22] Yan J, Henriksen E A, Kim P, Pinczuk A 2008 Phys. Rev. Lett. 101 136804
Google Scholar
[23] Castro Neto A H, Guinea F 2007 Phys. Rev. B 75 045404
Google Scholar
[24] Yan J A, Ruan W Y, Chou M Y 2009 Phys. Rev. B 79 115443
Google Scholar
[25] Pisana S, Lazzeri M, Casiraghi C, Novoselov K S, Geim A K, Ferrari A C, Mauri F 2007 Nat. Mater. 6 198
Google Scholar
[26] Wu W H, Zhu K D 2015 Opt. Commun. 342 199
Google Scholar
[27] Boyd R W 2008 Nonlinear Optics (San Diego: Academic Press) p278
[28] Bin W, Zhu K D 2013 Appl. Opt. 52 5816
Google Scholar
[29] Park C H, Giustino F, Cohen M L, Louie S G 2008 Nano lett. 8 4229
Google Scholar
[30] Sadeghi M, Naghdabadi R 2010 Nanotechnology 21 105705
Google Scholar
[31] Barton R A, Alden J S, Ruiz-Vargas C S, Whitney W S, Pham P H O, Park J, Parpia J M, Craighead H G, McEuen P L 2010 Nano Lett. 10 4869
Google Scholar
[32] Guo Q Q, Liang S, Gong B, Li J B, Xiao S, He M D, Chen L Q 2022 Opt. Express 30 6630
Google Scholar
[33] Li J B, He M D, Chen L Q 2014 Opt. Express 22 24734
Google Scholar
-
图 1 (a) 双层石墨烯薄膜体系与一个垂直作用于它的平面电场E的结构示意图, 该体系处于强泵浦场和弱探测场的共同作用下; (b) 双层石墨烯薄膜体系的能级示意图
Fig. 1. (a) Schematic diagram of a bilayer graphene nanosystem and an electric field E being perpendicular to the plane of graphene, where the nanosystem is simultaneously subjected to a strong pump field and a weak probe field; (b) energy level diagram of the bilayer graphene system.
图 2 (a) 四波混频信号参量α随激子-泵浦场失谐量Δex的变化关系; (b)三峰型四波混频谱的形成机理图; (c) L峰和R峰的峰值大小和位置随Δex的变化关系; 已知g = 1 THz和
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 10 THz2Fig. 2. (a) Parameter of the four-wave mixing signal α as a function of the detuning between the exciton and the pump field Δex; (b) the formation mechanism diagram of three-peaked four-wave mixing spectrum; (c) the maximum values and positions of L and R peaks as a function of Δex, the parameters used are g = 1 THz and
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 10 THz2.
图 3 (a) 四波混频信号参量α随声子-激子耦合强度g的变化关系(插图显示g = 0.2 THz时四波混频谱为五峰结构); (b) L峰和R峰的峰值大小和位置随声子-激子耦合强度g的变化关系
Fig. 3. (a) Parameter of the four-wave mixing signal α as a function of the phonon-exciton coupling strength g (inset exhibits that the four-wave mixing spectrum for g = 0.2 THz is five-peaked structure); (b) the maximum values and positions of L and R peaks as a function of the exciton-phonon coupling strength g.
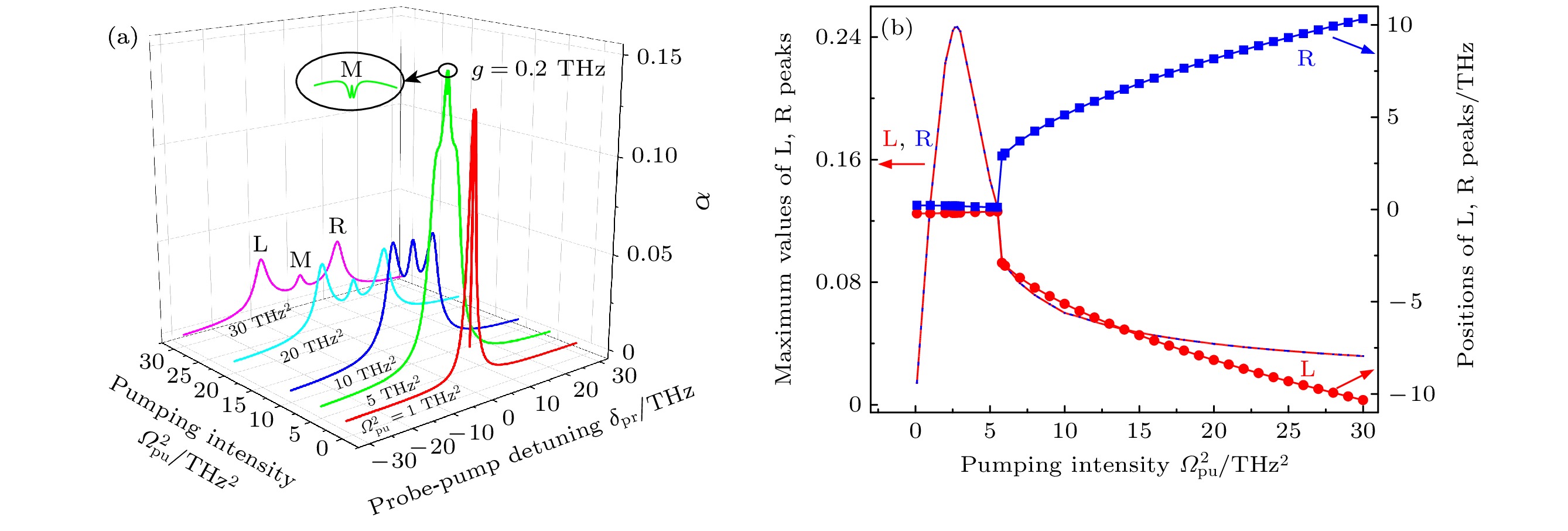
图 4 (a) 在弱耦合情况下(g = 0.2 THz), 四波混频信号参量α随泵浦强度
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ 的变化关系(插图显示$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 5 THz2时四波混频光谱为五峰结构); (b) L峰和R峰的峰值大小和位置随泵浦强度$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ 的变化关系Fig. 4. (a) In the weak phonon-exciton coupling regime (g = 0.2 THz), the dependence of the parameter of the four-wave mixing signal α on the pumping intensity
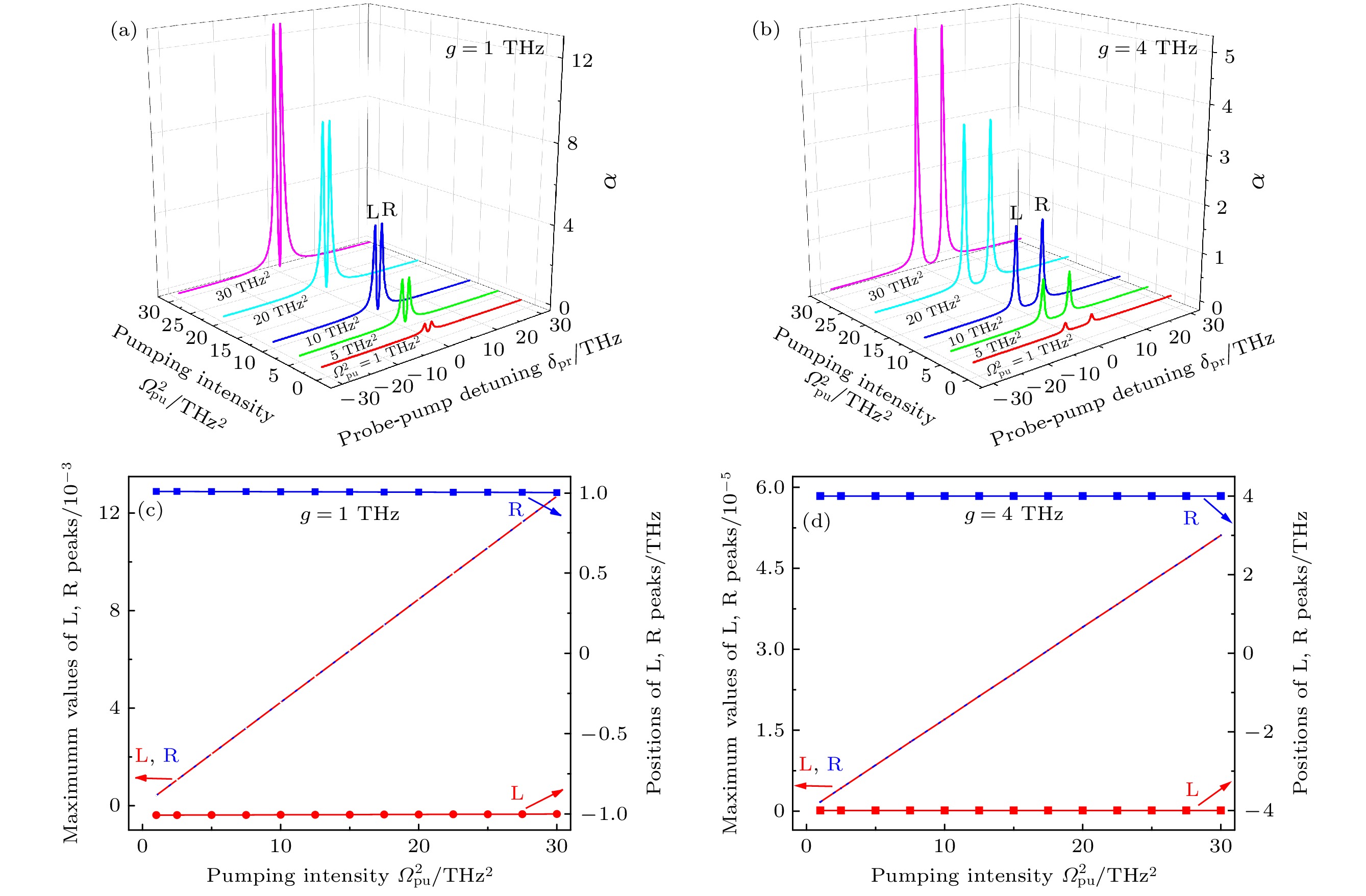
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ (the inset exhibits that the four-wave mixing spectrum for$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 5 THz2 is five-peaked structure); (b) the maximum values and positions of L and R peaks as a function of the pumping intensity$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ .
图 5 (a), (b) 在中间耦合(g = 1 THz = Γ2)和强耦合情况下(g = 4 THz >Γ2), 四波混频信号参量α随泵浦强度
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ 的变化关系; (c), (d) L峰和R峰的峰值大小和位置随泵浦强度$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ 的变化关系Fig. 5. (a), (b) In the intermediate and strong coupling regimes (g = 1 THz = Γ2 and g = 4 THz > Γ2), the parameter of the four-wave mixing signal α as a function of the pumping intensities
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ ; (c), (d) the dependence of peak values and positions for corresponding L and R peaks on the pumping intensities$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ .
图 6 (a) 当
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 10 THz2时, 比较3种不同耦合情况下四波混频信号参量α随探测-泵浦失谐量的变化关系; (b)在3种不同耦合情况下, 在δpr = 0 THz位置处的四波混频信号随泵浦强度$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ 的变化关系Fig. 6. (a) In three different coupling regimes, the parameter of the four-wave mixing signal α as a function of the probe-pump detuning δpr for
$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ = 10 THz2; (b) in three different coupling regimes, the dependence of the four-wave mixing signal positioned at δpr = 0 THz on the pumping intensity$ {\varOmega }_{{\text{pu}}}^{\text{2}} $ .表 1 四波混频谱的线型与Δex调控区间的关系
Table 1. Relation between the line-shapes of four-wave mixing spectra and the modulation region of Δex.
线型 二峰 三峰 六峰 五峰 Δex/THz $ \pm [0, 0.02) $ $ \pm [0.02, 0.12) $ $ \pm [0.12, 0.19) $ $ \pm [0.19, 10.0] $ 
下载: 导出CSV
表 2 四波混频谱的线型与g调控区间的关系
Table 2. Relation between the line-shapes of four-wave mixing spectra and the modulation region of g
线型 三峰 四峰 五峰 二峰 g/THz [0, 0.03) [0.03, 0.12) [0.12, 0.32) [0.32, 15.0]
下载: 导出CSV
表 3 四波混频谱的线型与
${\varOmega }_{{\text{pu}}}^{\text{2}}$ 调控区间的关系Table 3. Relation between the line-shapes of four-wave mixing spectra and the modulation region of
${\varOmega }_{{\text{pu}}}^{\text{2}}$ .线型 二峰 三峰 五峰 三峰 ${\varOmega }_{ {\text{pu} } }^{\text{2} }$/THz2 [0.1, 3.32) [3.32, 3.95) [3.95, 89.41] > 89.41
下载: 导出CSV
-
[1] Nair R R, Blake P, Grigorenko A N, Novoselov K S, Booth T J, Stauber T, Peres N M R, Geim A K 2008 Science 320 1308
Google Scholar
[2] Chen C, Rosenblatt S, Bolotin K I, Kalb W, Kim P, Kymissis I, Stormer H L, Heinz T F, Hone J 2009 Nat. Nanotechnol. 4 861
Google Scholar
[3] Jiang J W, Park H S, Rabczuk T 2012 Nanotechnology 23 475501
Google Scholar
[4] Xiang Y J, Dai X Y, Guo J, Wen S C, Tang D Y 2014 Appl. Phys. Lett. 104 051108
Google Scholar
[5] Yi Y, Chen Z, Yu X F, Zhou Z K, Li J 2019 Adv. Quantum Technol. 2 1800111
Google Scholar
[6] Zhang X J, Yuan Z H, Yang R X, He Y L, Qin Y L, Xiao S, He J 2019 J. Cent. South Univ. 26 2295
Google Scholar
[7] Tan Y, Xia X S, Liao X L, Li J B, Zhong H H, Liang S, Xiao S, Liu L H, Luo J H, He M D, Chen L Q 2020 Carbon 157 724
Google Scholar
[8] 王波, 张纪红, 李聪颖 2021 70 054207
Google Scholar
Wang B, Zhang J H, Li C Y 2021 Acta Phys. Sin. 70 054207
Google Scholar
[9] 郭晓蒙, 青芳竹, 李雪松 2021 70 098102
Google Scholar
Guo X M, Qing F Z, Li X S 2021 Acta Phys. Sin. 70 098102
Google Scholar
[10] Mayorov A S, Elias D C, Mucha-Kruczynski M, Gorbachev R V, Tudorovskiy T, Zhukov A, Morozov S V, Katsnelson M I, Fal’ko V I, Geim A K, Novoselov K S 2011 Science 333 860
Google Scholar
[11] Grigorenko A N, Polini M, Novoselov K S 2012 Nat. Photon. 6 749
Google Scholar
[12] Fei Z, Iwinski E G, Ni G X, Zhang L M, Bao W, Rodin A S, Lee Y, Wagner M, Liu M K, Dai S, Goldflam M D, Thiemens M, Keilmann F, Lau C N, Castro-Neto A H, Fogler M M, Basov D N 2015 Nano Lett. 15 4973
Google Scholar
[13] Zhao X J, Hou H, Fan X T, Wang Y, Liu Y M, Tang C, Liu S H, Ding P P, Cheng J, Lin D H, Wang C, Yang Y, Tan Y Z 2019 Nat. Commun. 10 3057
Google Scholar
[14] Xu S, Al Ezzi M M, Balakrishnan N, Garcia-Ruiz A, Tsim B, Mullan C, Barrier J, Xin N, Piot B A, Taniguchi T, Watanabe K, Carvalho A, Mishchenko A, Geim A K, Vladimir I. Fal’ko V I, Adam S, Castro Neto A H, Novoselov K S, Shi Y M 2021 Nat. Phys. 17 619
Google Scholar
[15] Malard L M, Nilsson J, Elias D C, Brant J C, Plentz F, Alves E S, Castro Neto A H, Pimenta M A 2007 Phys. Rev. B 76 201401(R
Google Scholar
[16] Tang T T, Zhang Y B, Park C H, Geng B S, Girit C, Hao Z, Martin M C, Zettl A, Crommie M F, Louie S G, Shen Y R, Wang F 2009 Nat. Nanotechnol. 5 32
Google Scholar
[17] Yang T Y, Balakrishnan J, Volmer F, Avsar A, Jaiswal M, Samm J, Ali S R, Pachoudeng A, Popinciuc M, Güntherodt G, Beschoten B 2011 Phys. Rev. Lett. 107 047206
Google Scholar
[18] Kou A, Feldman B E, Levin A J, Halperin B I, Watanabe K, Taniguchi T, Yacoby A 2014 Science 345 6192
Google Scholar
[19] Ki D K, Fal'ko V I, Abanin D A, Morpurgo A F 2014 Nano Lett. 14 2135
Google Scholar
[20] Da H X, Yan X H 2016 Opt. Lett. 41 151
Google Scholar
[21] Yu G, Wu Z, Zhan Z, Katsnelson M I, Yuan S J 2020 Phys. Rev. B 102 115123
Google Scholar
[22] Yan J, Henriksen E A, Kim P, Pinczuk A 2008 Phys. Rev. Lett. 101 136804
Google Scholar
[23] Castro Neto A H, Guinea F 2007 Phys. Rev. B 75 045404
Google Scholar
[24] Yan J A, Ruan W Y, Chou M Y 2009 Phys. Rev. B 79 115443
Google Scholar
[25] Pisana S, Lazzeri M, Casiraghi C, Novoselov K S, Geim A K, Ferrari A C, Mauri F 2007 Nat. Mater. 6 198
Google Scholar
[26] Wu W H, Zhu K D 2015 Opt. Commun. 342 199
Google Scholar
[27] Boyd R W 2008 Nonlinear Optics (San Diego: Academic Press) p278
[28] Bin W, Zhu K D 2013 Appl. Opt. 52 5816
Google Scholar
[29] Park C H, Giustino F, Cohen M L, Louie S G 2008 Nano lett. 8 4229
Google Scholar
[30] Sadeghi M, Naghdabadi R 2010 Nanotechnology 21 105705
Google Scholar
[31] Barton R A, Alden J S, Ruiz-Vargas C S, Whitney W S, Pham P H O, Park J, Parpia J M, Craighead H G, McEuen P L 2010 Nano Lett. 10 4869
Google Scholar
[32] Guo Q Q, Liang S, Gong B, Li J B, Xiao S, He M D, Chen L Q 2022 Opt. Express 30 6630
Google Scholar
[33] Li J B, He M D, Chen L Q 2014 Opt. Express 22 24734
Google Scholar
下载:
计量
- 文章访问数: 2799
- PDF下载量: 42
- 被引次数: 0
