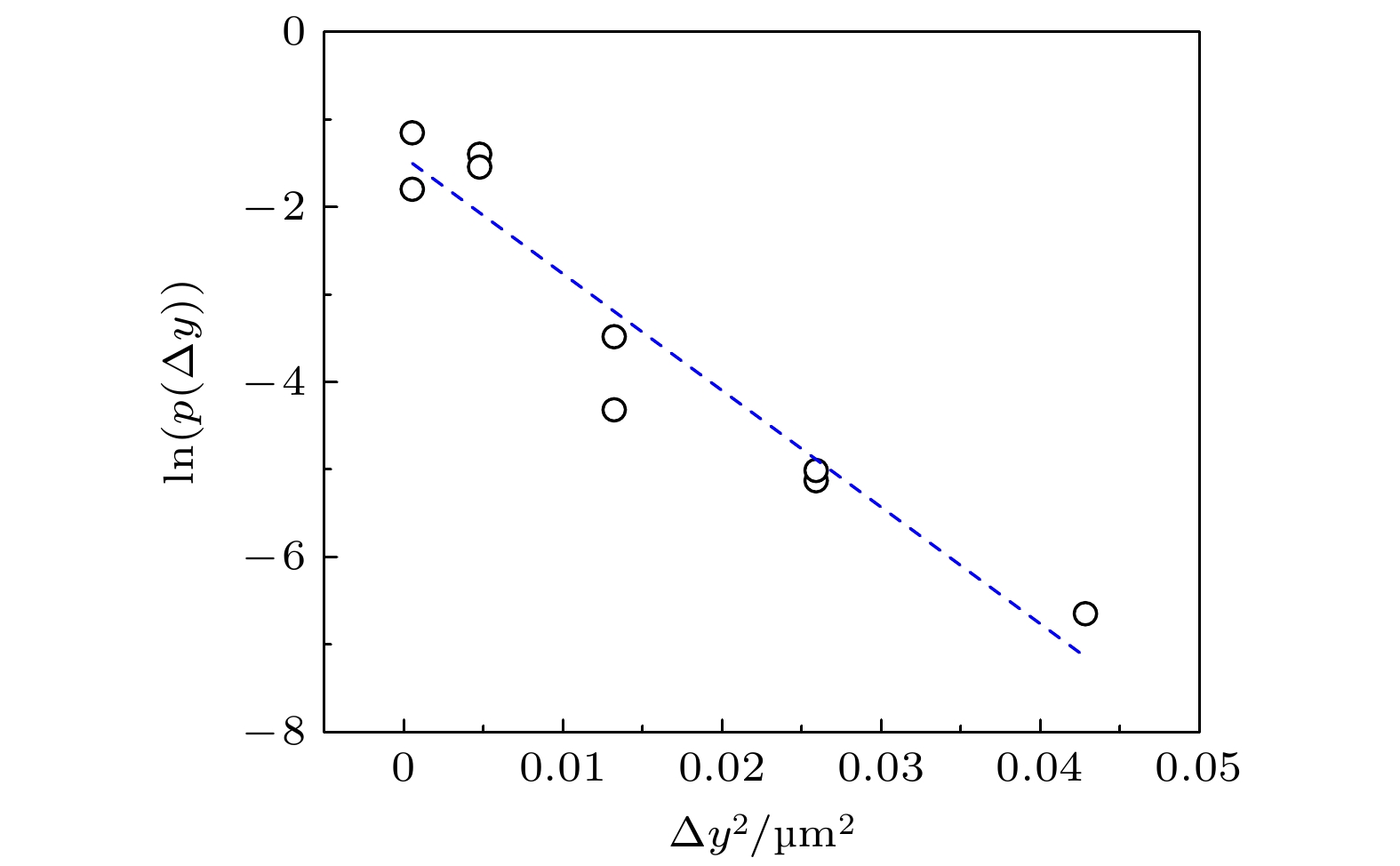
-
In active matter, the effective force between passive objects is crucial for their structure and dynamics, which is the basis for understanding the complex behaviors within active systems. Unlike equilibrium states, the factors such as the surface configuration, size, and confinement strength significantly influence the effective forces between passive particles. Previous studies have shown that the shapes of passive particles affect the aggregation of active particles, leading to different forces experienced by passive particles with different shapes. However, recently, a long-range attractive force between passive platelike particles, caused by the bacterial flow field instead of the direct bacterium-plate collisions in active bacterial suspensions, has been found. This raises an intriguing question: how does hydrodynamics affect the forces on passive particles of different shapes in different ways? In this work, we investigate the effective forces exerted on passive spherical and plate-like particles immersed in bacterial suspensions by optical-tweezers experiments. The effective force between passive particles can be calculated from the formula, $ {F_{{\text{eff}}}} = {\text{ }}k\left\langle {\Delta d} \right\rangle /2 $ , where$ \left\langle {\Delta d} \right\rangle $ represents the difference in distance between the passive particles in the bacterial bath and those in the solution without bacteria,$ k $ is the effective stiffness of optical traps. The$ {{{F}}}_{{\mathrm{e}}{\mathrm{f}}{\mathrm{f}}} > 0 $ indicates a repulsive force between passive particles, and the$ {F_{{\text{eff}}}} \lt {\text{ }}0 $ represents an effective attractive force between passive particles. Our results demonstrate that the passive spherical particles experience short-range repulsion, while plate-like particles are subjected to long-range attraction. This highlights the substantial effect of particle shape on their effective forces.The forces on passive particles are mainly attributed to two factors: direct bacterium-particle collisions and the bacterial flow field. The analysis of the bacterial concentration and orientation distribution around passive particles reveals that for spherical particles, the concentration of bacteria between particles is higher than that outside the particles, but there is almost no difference in the orientation order between bacteria inside and outside the particles. This suggests that the effective repulsion between spherical particles is mainly due to the direct bacterial collisions. Conversely, for plate-like particles, the long-range attraction is primarily influenced by the bacterial flow field rather than their direct collisions, which is evidenced by the higher bacterial density and orientation order inside the two plates compared with those outside the two plates. This study provides strong evidence that the effective force between passive particles is shape dependent in active bath, and offers new insights into controlling active-directed assembly. 
-
Keywords:
- active matter /
- optical tweezers /
- effective interaction /
- particle shape
[1] Zhang H P, Be’er A, Florin E L, Swinney H L 2010 Proc. Natl. Acad. Sci. U. S. A. 107 13626
Google Scholar
[2] Karamouzas I, Skinner B, Guy S J 2014 Phys. Rev. Lett. 113 238701
Google Scholar
[3] Palacci J, Sacanna S, Steinberg A P, Pine D J, Chaikin P M 2013 Science 339 936
Google Scholar
[4] Buttinoni I, Bialké J, Kümmel F, Löwen H, Bechinger C, Speck T 2013 Phys. Rev. Lett. 110 238301
Google Scholar
[5] Petroff A P, Wu X L, Libchaber A 2015 Phys. Rev. Lett. 114 158102
Google Scholar
[6] Bechinger C, Leonardo R Di, Löwen H, Reichhardt C, Volpe G 2016 Rev. Mod. Phys. 88 045006
Google Scholar
[7] Needleman D, Dogic Z 2017 Nat. Rev. Mater. 2 17048
Google Scholar
[8] Gonzalez-Rodriguez D, Guevorkian K, Douezan S, Brochart-Wyart F 2012 Science 338 910
Google Scholar
[9] Nelson B J, Kaliakatsos I K, Abbott J J 2010 Annu. Rev. Biomed. Eng. 12 55
Google Scholar
[10] Liu P, Ye S M, Ye F F, Chen K, Yang M C 2020 Phys. Rev. Lett. 124 158001
Google Scholar
[11] Ni R, Cohen Stuart M A, Bolhuis P G 2015 Phys. Rev. Lett. 114 018302
Google Scholar
[12] Ray D, Reichhardt C, Olson Reichhardt C J 2014 Phys. Rev. E 90 013019
Google Scholar
[13] Harder J, Mallory S A, Tung C, Valeriani C, Cacciuto A 2014 J. Chem. Phys. 141 194901
Google Scholar
[14] Leite L R, Lucena D, Potiguar F Q, Ferreira W P 2016 Phys. Rev. E 94 062602
Google Scholar
[15] Feng F, Lei T, Zhao N R 2021 Phys. Rev. E 103 022604
Google Scholar
[16] Paul S, Jayaram A, Narinder N, Speck T, Bechinger C 2022 Phys. Rev. Lett. 129 058001
Google Scholar
[17] Ning L H, Lou X, Ma Q L, Yang Y C, Luo N, Chen K, Meng F L, Zhou X, Yang M C, Peng Y 2023 Phys. Rev. Lett. 131 158301
Google Scholar
[18] Ashkin A, Dziedzic J M, Bjorkholm J E, Chu S 1986 Opt. Lett. 5 288
Google Scholar
[19] Pesce G, Jones P H, Maragò O M, Volpe G 2020 Eur. Phys. J. Plus 135 949
Google Scholar
[20] Volpe G, Maragò O M, Rubinsztein-Dunlop H, et al. 2023 J. Phys. Photonics 5 022501
Google Scholar
[21] Bustamante C, Alexander L, Maciuba K, Kaiser C M 2020 Annu. Rev. Biochem. 89 443
Google Scholar
[22] Baek Y, Solon A P, Xu X P, Nikola N, Kafri Y 2018 Phys. Rev. Lett. 120 058002
Google Scholar
[23] Walter J M, Greenfield D, Bustamante C, Liphardt J 2007 Proc. Natl. Acad. Sci. U. S. A. 104 2408
Google Scholar
[24] Peng Y, Liu Z Y, Cheng X 2021 Sci. Adv. 7 eabd1240
Google Scholar
[25] Hernandez C J, Mason T G 2007 J. Phys. Chem. C 111 4477
Google Scholar
[26] Zheng Z Y, Han Y L 2010 J. Chem. Phys. 133 124509
Google Scholar
[27] Drescher K, Dunkel J, Cisneros L H, Ganguly S, Goldstein R E 2011 Proc. Natl. Acad. Sci. U. S. A. 108 10940
Google Scholar
[28] Lauga1 E, Powers T R 2009 Rep. Prog. Phys. 72 096601
Google Scholar
-
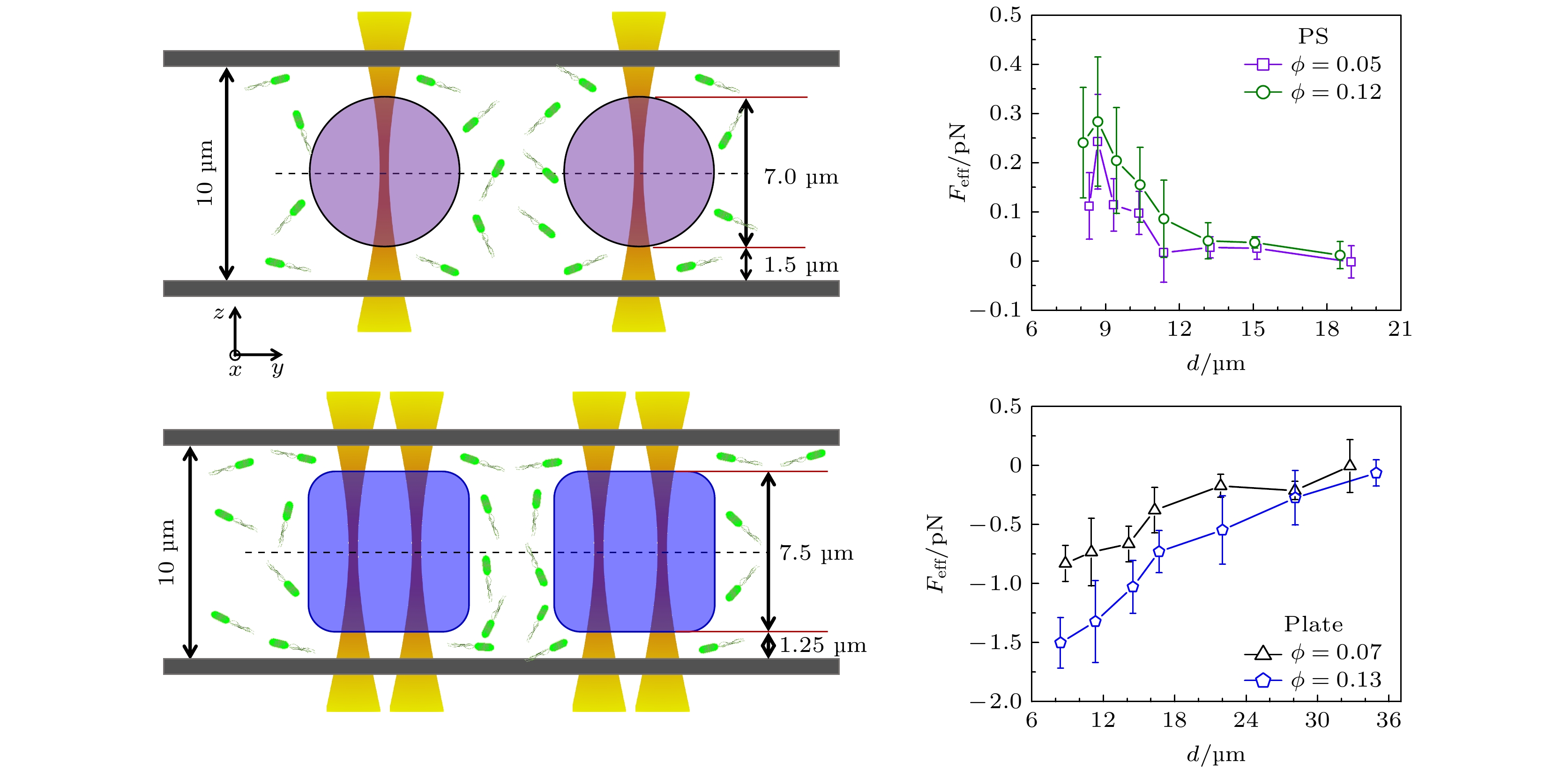
图 1 光镊实验测量惰性粒子间有效作用力的示意图 (a)使用光学势阱将两个球形聚苯乙烯胶体粒子(紫色圆)固定在两盖玻片(灰色厚实线)之间; (b)使用多个光学势阱将两平行板(蓝色方块)固定在两盖玻片之间; 实验观测平面设置在光镊的光腰位置在图中由黑色虚线标出
Figure 1. Schematic diagram of optical tweezers measuring the effective force between passive particles: (a) Two spherical PS particles (purple circles) are trapped between two cover glasses (thick grey lines); (b) two parallel plates (blue squares) are trapped between two cover slides using multiple optical potential wells. The observation plane marked by the black dashed line in (a), (b) is set at the optical waist of the optical tweezers.
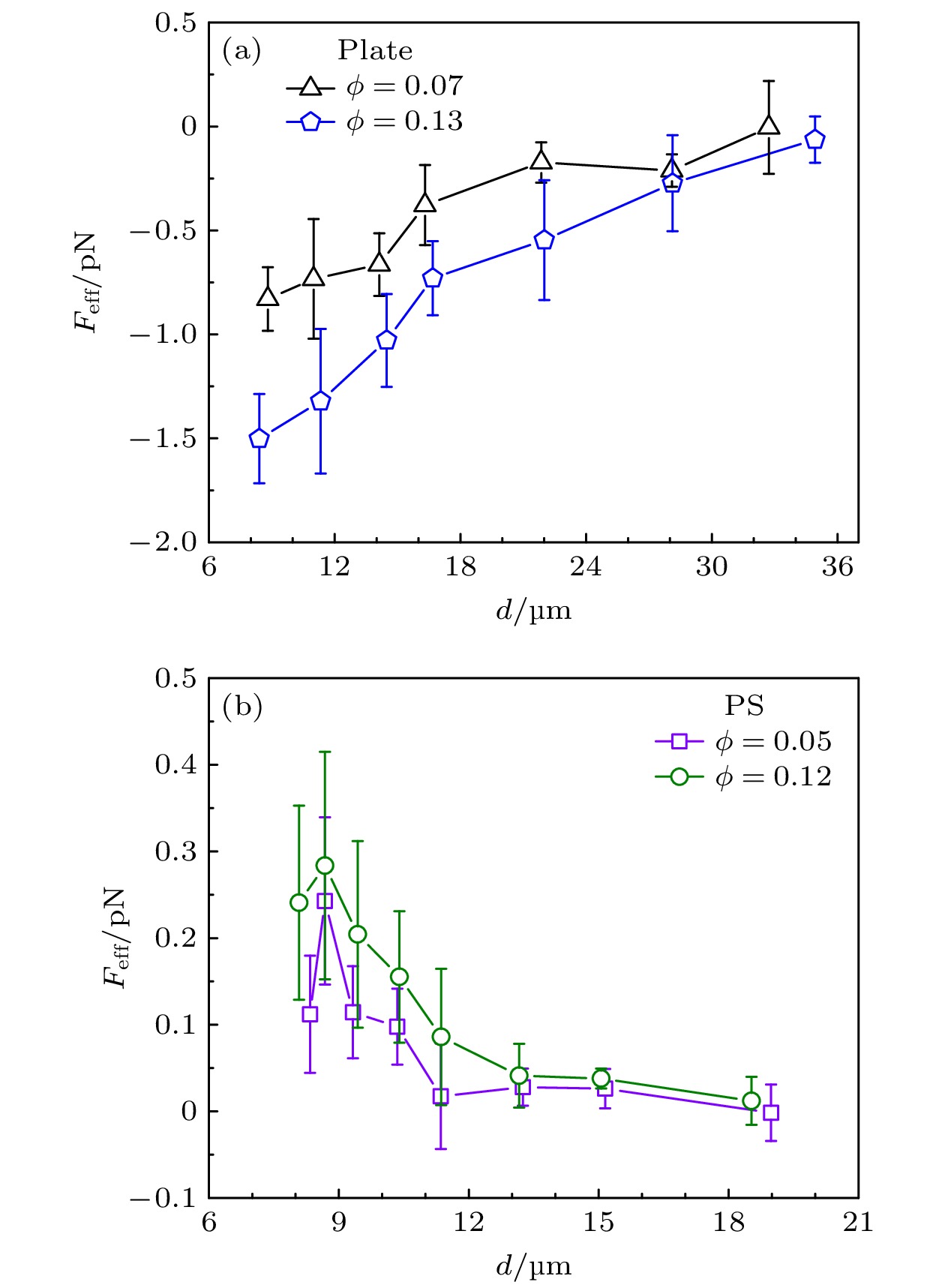
图 3 不同细菌浓度ϕ时惰性粒子间的有效相互作用力与惰性粒子间距离d的关系 (a) 板状粒子间(ϕ = 0.07, 0.13); (b)球形PS粒子间(ϕ = 0.05, 0.12); 图中的符号和误差棒分别表示由4—16次独立测量得到的平均值和标准差
Figure 3. Effective force between two passive particles as a function of the distance d of them at different bacterial concentration ϕ: (a) Two passive plates (ϕ = 0.07, 0.13); (b) two spherical PS particles (ϕ = 0.05, 0.12). The symbols and error bars represent the mean and standard deviation calculated over 4–16 independent measurements, respectively.
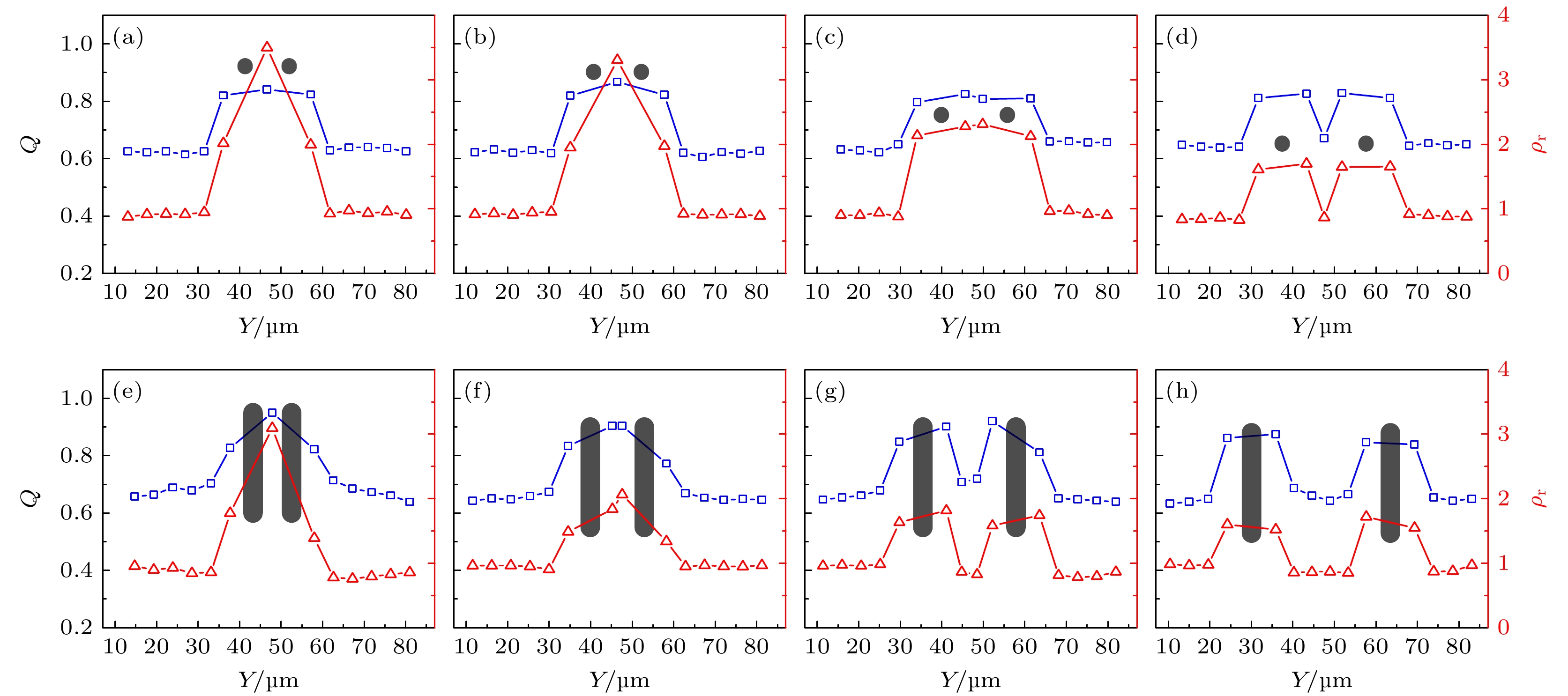
图 4 惰性粒子附近, 细菌的相对局域密度$ {\rho _{\text{r}}} $和局域取向有序性参数Q的分布, 其中红色三角形和蓝色正方形分别表示$ {\rho _{\text{r}}} $和Q, 黑色阴影圆形和板状区域分别表示球形和板状粒子的相对位置; (a)—(d)对应球形粒子, 其间距分别为8.68, 10.58, 15.47, 19.35 μm; (e)—(h)对应板状粒子, 其间距分别为8.95, 14.38, 21.89, 33.29 μm
Figure 4. Distribution of relative density of bacteria $ {\rho _{\text{r}}} $ (red triangles) and local orientation order Q (blue squares) around two passive spherical particles (black shaded circles) and plate-like particles (black plate-like area): (a)–(d) Correspond to spherical particles with the distances of 8.68, 10.58, 15.47, and 19.35 μm, respectively; (e)–(h) correspond to plates with the distances of 8.95, 14.38, 21.89, and 33.29 μm, respectively.
-
[1] Zhang H P, Be’er A, Florin E L, Swinney H L 2010 Proc. Natl. Acad. Sci. U. S. A. 107 13626
Google Scholar
[2] Karamouzas I, Skinner B, Guy S J 2014 Phys. Rev. Lett. 113 238701
Google Scholar
[3] Palacci J, Sacanna S, Steinberg A P, Pine D J, Chaikin P M 2013 Science 339 936
Google Scholar
[4] Buttinoni I, Bialké J, Kümmel F, Löwen H, Bechinger C, Speck T 2013 Phys. Rev. Lett. 110 238301
Google Scholar
[5] Petroff A P, Wu X L, Libchaber A 2015 Phys. Rev. Lett. 114 158102
Google Scholar
[6] Bechinger C, Leonardo R Di, Löwen H, Reichhardt C, Volpe G 2016 Rev. Mod. Phys. 88 045006
Google Scholar
[7] Needleman D, Dogic Z 2017 Nat. Rev. Mater. 2 17048
Google Scholar
[8] Gonzalez-Rodriguez D, Guevorkian K, Douezan S, Brochart-Wyart F 2012 Science 338 910
Google Scholar
[9] Nelson B J, Kaliakatsos I K, Abbott J J 2010 Annu. Rev. Biomed. Eng. 12 55
Google Scholar
[10] Liu P, Ye S M, Ye F F, Chen K, Yang M C 2020 Phys. Rev. Lett. 124 158001
Google Scholar
[11] Ni R, Cohen Stuart M A, Bolhuis P G 2015 Phys. Rev. Lett. 114 018302
Google Scholar
[12] Ray D, Reichhardt C, Olson Reichhardt C J 2014 Phys. Rev. E 90 013019
Google Scholar
[13] Harder J, Mallory S A, Tung C, Valeriani C, Cacciuto A 2014 J. Chem. Phys. 141 194901
Google Scholar
[14] Leite L R, Lucena D, Potiguar F Q, Ferreira W P 2016 Phys. Rev. E 94 062602
Google Scholar
[15] Feng F, Lei T, Zhao N R 2021 Phys. Rev. E 103 022604
Google Scholar
[16] Paul S, Jayaram A, Narinder N, Speck T, Bechinger C 2022 Phys. Rev. Lett. 129 058001
Google Scholar
[17] Ning L H, Lou X, Ma Q L, Yang Y C, Luo N, Chen K, Meng F L, Zhou X, Yang M C, Peng Y 2023 Phys. Rev. Lett. 131 158301
Google Scholar
[18] Ashkin A, Dziedzic J M, Bjorkholm J E, Chu S 1986 Opt. Lett. 5 288
Google Scholar
[19] Pesce G, Jones P H, Maragò O M, Volpe G 2020 Eur. Phys. J. Plus 135 949
Google Scholar
[20] Volpe G, Maragò O M, Rubinsztein-Dunlop H, et al. 2023 J. Phys. Photonics 5 022501
Google Scholar
[21] Bustamante C, Alexander L, Maciuba K, Kaiser C M 2020 Annu. Rev. Biochem. 89 443
Google Scholar
[22] Baek Y, Solon A P, Xu X P, Nikola N, Kafri Y 2018 Phys. Rev. Lett. 120 058002
Google Scholar
[23] Walter J M, Greenfield D, Bustamante C, Liphardt J 2007 Proc. Natl. Acad. Sci. U. S. A. 104 2408
Google Scholar
[24] Peng Y, Liu Z Y, Cheng X 2021 Sci. Adv. 7 eabd1240
Google Scholar
[25] Hernandez C J, Mason T G 2007 J. Phys. Chem. C 111 4477
Google Scholar
[26] Zheng Z Y, Han Y L 2010 J. Chem. Phys. 133 124509
Google Scholar
[27] Drescher K, Dunkel J, Cisneros L H, Ganguly S, Goldstein R E 2011 Proc. Natl. Acad. Sci. U. S. A. 108 10940
Google Scholar
[28] Lauga1 E, Powers T R 2009 Rep. Prog. Phys. 72 096601
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 1298
- PDF Downloads: 58
- Cited By: 0
