-
Doping and defect control in semiconductors are essential prerequisites for their practical applications. First-principles calculations of defects based on density functional theory offer crucial guidance for doping and defect control. In this paper, the developments in the theoretical methods of first-principles semiconductor defect calculations are introduced. Firstly, we introduce the method of calculating the defect formation energy and finite-size errors to the formation energy caused by the supercell method. Then, we present corresponding image charge correction schemes, which include the widely used post-hoc corrections (such as Makov-Payne, Lany-Zunger, Freysoldt-Neugebauer-van de Walle schemes), the recently developed self-consistent potential correction which performs the image charge correction in the self-consistent loop for solving Kohn-Sham equations, and the self-consistent charge correction scheme which does not require an input of macroscopic dielectric constants. Further, we extend our discussion to charged defect calculations in low-dimensional semiconductors, elucidate the issue of charged defect formation energy divergence with the increase of vacuum thickness within the jellium model and introduce our theoretical model which solves this energy divergence issue by placing the ionized electrons or holes in the realistic host band-edge states instead of the virtual jellium state. Furthermore, we provide a brief overview of defect calculation correction methods due to the DFT band gap error, including the scissors operator, LDA+U and hybrid functionals. Finally, in order to describe the calculation of defect formation energy under illumination, we present our self-consistent two-Fermi-reservoir model, which can well predict the defect concentration and carrier concentration in the Mg doped GaN system under illumination. This work summarizes the recent developments regarding first-principles calculations of defects in semiconducting materials and low-dimensional semiconductors, under whether equilibrium conditions or non-equilibrium conditions, thus promoting further developments of doping and defect control within semiconductors.
-
Keywords:
- defect calculation in semiconductors /
- image charge correction /
- defect physics in low-dimensional semiconductors /
- non-equilibrium doping
[1] Grasser T 2012 Microelectron. Reliab. 52 39
Google Scholar
[2] Grasser T, Kaczer B, Goes W, Reisinger H, Aichinger T, Hehenberger P, Wagner P J, Schanovsky F, Franco J, Luque M T T, Nelhiebel M 2011 IEEE T. Electron Dev. 58 3652
Google Scholar
[3] Krishnan A T, Chancellor C, Chakravarthi S, Nicollian P E, Reddy V, Varghese A, Khamankar R B, Krishnan S 2005 IEEE International Electron Devices Meeting, 2005. IEDM Technical Digest Arazon, USA, December 5, 2005 p4
[4] Breitenstein O, Bauer J, Altermatt P P, Ramspeck K 2009 Solid State Phenom. 156-158 1
Google Scholar
[5] Aydin E, De Bastiani M, De Wolf S 2019 Adv. Mater. 31 1900428
Google Scholar
[6] Park J S, Kim S, Xie Z, Walsh A 2018 Nat. Rev. Mater. 3 194
Google Scholar
[7] Bai S, Zhang N, Gao C, Xiong Y 2018 Nano Energy 53 296
Google Scholar
[8] Jia J, Qian C, Dong Y, Li Y F, Wang H, Ghoussoub M, Butler K T, Walsh A, Ozin G A 2017 Chem. Soc. Rev. 46 4631
Google Scholar
[9] Ulbricht R, Hendry E, Shan J, Heinz T F, Bonn M 2011 Rev. Mod. Phys. 83 543
Google Scholar
[10] deQuilettes D W, Frohna K, Emin D, Kirchartz T, Bulovic V, Ginger D S, Stranks S D 2019 Chem. Rev. 119 11007
Google Scholar
[11] Reshchikov M A, McNamara J D, Toporkov M, Avrutin V, Morkoç H, Usikov A S, Helava H I, Makarov Y N 2016 Sci. Rep. 6 37511
Google Scholar
[12] Vines L, Monakhov E, Kuznetsov A 2022 J. Appl. Phys. 132 150401
Google Scholar
[13] McCluskey M D 2020 J. Appl. Phys. 127 101101
Google Scholar
[14] Tuomisto F 2019 Characterisation and Control of Defects in Semiconductors (London: Institution of Engineering and Technology
[15] Ingebrigtsen M E, Varley J B, Kuznetsov A Y, Svensson B G, Alfieri G, Mihaila A, Badstübner U, Vines L 2018 Appl. Phys. Lett. 112 042104
Google Scholar
[16] Venzie A, Portoff A, Valenzuela E C P, Stavola M, Fowler W B, Pearton S J, Glaser E R 2022 J. Appl. Phys. 131 035706
Google Scholar
[17] Huang H-L, Chae C, Hwang J 2022 J. Appl. Phys. 131 190901
Google Scholar
[18] Watkins G D 1999 Phys. Solid State 41 746
Google Scholar
[19] Willardson R K, Weber E R, Stavola M 1998 Identification of Defects in Semiconductors (Academic Press
[20] Walsh A, Butler K T 2013 Acc. Chem. Res. 47 364
Google Scholar
[21] Le Bahers T, Rérat M, Sautet P 2014 J. Phys. Chem. C 118 5997
Google Scholar
[22] Jain A, Shin Y, Persson K A 2016 Nat. Rev. Mater. 1 15004
Google Scholar
[23] Freysoldt C, Grabowski B, Hickel T, Neugebauer J, Kresse G, Janotti A, Van de Walle C G 2014 Rev. Mod. Phys. 86 253
Google Scholar
[24] Wang L 2019 J. Semicond. 40 091101
Google Scholar
[25] Suo Z, Wang L, Li S, Luo J 2022 J. Semicond. 43 112801
Google Scholar
[26] Cao R, Deng H X, Luo J W, Wei S H 2019 J. Semicond. 40 042102
Google Scholar
[27] Makov G, Payne M C 1995 Phys. Rev. B 51 4014
Google Scholar
[28] Lany S, Zunger A 2008 Phys. Rev. B 78 235104
Google Scholar
[29] Lany S, Zunger A 2009 Modell. Simul. Mater. Sci. Eng. 17 084002
Google Scholar
[30] Freysoldt C, Neugebauer J, Van de Walle C G 2009 Phys. Rev. Lett. 102 016402
Google Scholar
[31] da Silva M C, Lorke M, Aradi B, Farzalipour Tabriz M, Frauenheim T, Rubio A, Rocca D, Deák P 2021 Phys. Rev. Lett. 126 076401
Google Scholar
[32] Suo Z J, Luo J W, Li S S, Wang L W 2020 Phys. Rev. B 102 174110
Google Scholar
[33] Baraff G A, Schlüter M 1984 Phys. Rev. B 30 3460
Google Scholar
[34] Anisimov V I, Zaanen J, Andersen O K 1991 Phys. Rev. B 44 943
Google Scholar
[35] Heyd J, Scuseria G E, Ernzerhof M 2006 J. Chem. Phys. 124 219906
Google Scholar
[36] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
[37] Fuchs F, Furthmüller J, Bechstedt F, Shishkin M, Kresse G 2007 Phys. Rev. B 76 115109
Google Scholar
[38] Komsa H P, Rantala T T, Pasquarello A 2012 Phys. Rev. B 86 045112
Google Scholar
[39] Leslie M, Gillan N J 1985 J. Phys. C: Solid State Phys. 18 973
Google Scholar
[40] Castleton C W M, Höglund A, Mirbt S 2006 Phys. Rev. B 73 035215
Google Scholar
[41] Hine N D M, Frensch K, Foulkes W M C, Finnis M W 2009 Phys. Rev. B 79 024112
Google Scholar
[42] Murphy S T, Hine N D M 2013 Phys. Rev. B 87 094111
Google Scholar
[43] Kumagai Y, Oba F 2014 Phys. Rev. B 89 195205
Google Scholar
[44] Freysoldt C, Neugebauer J 2018 Phys. Rev. B 97 205425
Google Scholar
[45] Wang D, Han D, Li X B, Xie S Y, Chen N K, Tian W Q, West D, Sun H-B, Zhang S B 2015 Phys. Rev. Lett. 114 196801
Google Scholar
[46] Wang D, Han D, Li X B, Chen N K, West D, Meunier V, Zhang S, Sun H B 2017 Phys. Rev. B 96 155424
Google Scholar
[47] Richter N A, Sicolo S, Levchenko S V, Sauer J, Scheffler M 2013 Phys. Rev. Lett. 111 045502
Google Scholar
[48] Xiao J, Yang K, Guo D, Shen T, Deng H X, Li S S, Luo J W, Wei S H 2020 Phys. Rev. B 101 165306
Google Scholar
[49] Godby R W, Schlüter M, Sham L J 1986 Phys. Rev. Lett. 56 2415
Google Scholar
[50] Mori-Sánchez P, Cohen A J, Yang W 2008 Phys. Rev. Lett. 100 146401
Google Scholar
[51] Janotti A, Van de Walle C G 2007 Phys. Rev. B 76 165202
Google Scholar
[52] Clark S J, Robertson J 2010 Phys. Status 248 537
Google Scholar
[53] Zanolli Z, Fuchs F, Furthmüller J, von Barth U, Bechstedt F 2007 Phys. Rev. B 75 245121
Google Scholar
[54] Perdew J P 2009 Int. J. Quantum Chem 28 497
Google Scholar
[55] Perdew J P, Levy M 1983 Phys. Rev. Lett. 51 1884
Google Scholar
[56] Sham L J, Schlüter M 1983 Phys. Rev. Lett. 51 1888
Google Scholar
[57] Wang L W 2001 Appl. Phys. Lett. 78 1565
Google Scholar
[58] Zhang G, Canning A, Grønbech-Jensen N, Derenzo S, Wang L W 2013 Phys. Rev. Lett. 110 166404
Google Scholar
[59] Kang J, Wang L W 2022 Phys. Rev. Appl. 18 064001
Google Scholar
[60] Zhang S B, Wei S H, Zunger A 2001 Phys. Rev. B 63 075205
Google Scholar
[61] Zhang S B 2002 J. Phys. : Condens. Matter 14 R881
Google Scholar
[62] Van de Walle C G, Neugebauer J 2004 J. Appl. Phys. 95 3851
Google Scholar
[63] Wei S H, Zunger A 1988 Phys. Rev. B 37 8958
Google Scholar
[64] Janotti A, Van de Walle C G 2010 Phys. Status 248 799
Google Scholar
[65] Cococcioni M, de Gironcoli S 2005 Phys. Rev. B 71 035105
Google Scholar
[66] Boonchun A, Lambrecht W R L 2010 Phys. Status 248 1043
Google Scholar
[67] Becke A D 1993 J. Chem. Phys. 98 1372
Google Scholar
[68] Muscat J, Wander A, Harrison N M 2001 Chem. Phys. Lett. 342 397
Google Scholar
[69] Perdew J P, Ernzerhof M, Burke K 1996 J. Chem. Phys. 105 9982
Google Scholar
[70] Cai X, Zhang P, Wei S H 2019 J. Semicond. 40 092101
Google Scholar
[71] Deák P, Aradi B, Frauenheim T, Janzén E, Gali A 2010 Phys. Rev. B 81 153203
Google Scholar
[72] Davies G, Hamer M F, Price W C 1997 Proceed. of the Royal Soc. London. A. Math. and Phys. Sci. 348 285
Google Scholar
[73] Komsa H P, Pasquarello A 2011 Phys. Rev. B 84 075207
Google Scholar
[74] Blakemore J S 1982 J. Appl. Phys. 53 R123
Google Scholar
[75] Lin L 2016 J. Chem. Theory Comput. 12 2242
Google Scholar
[76] Bang J, Sun Y Y, Abtew T A, Samanta A, Zhang P, Zhang S B 2013 Phys. Rev. B 88 035134
Google Scholar
[77] Bryan Z, Bryan I, Gaddy B E, Reddy P, Hussey L, Bobea M, Guo W, Hoffmann M, Kirste R, Tweedie J, Gerhold M, Irving D L, Sitar Z, Collazo R 2014 Appl. Phys. Lett. 105 222101
Google Scholar
[78] Male J, Agne M T, Goyal A, Anand S, Witting I T, Stevanović V, Snyder G J 2019 Mater. Horiz. 6 1444
Google Scholar
[79] Cai X, Luo J, Li S, Wei S, Deng H 2022 Phys. Rev. B 106 214102
Google Scholar
[80] Harper R L, Hwang S, Giles N C, Schetzina J F, Dreifus D L, Myers T H 1989 Appl. Phys. Lett. 54 170
Google Scholar
[81] Fujita S, Fujita S 1992 J. Cryst. Growth 117 67
Google Scholar
[82] Simpson J, Hauksson I, Wang S Y, Stewart H, Prior K A, Cavenett B C 1993 Physica B 185 164
Google Scholar
[83] Li X, Zhang B, Zhu H, Dong X, Xia X, Cui Y, Huang K, Du G 2008 Appl. Surf. Sci. 254 2081
Google Scholar
[84] Bryan Z, Hoffmann M, Tweedie J, Kirste R, Callsen G, Bryan I, Rice A, Bobea M, Mita S, Xie J, Sitar Z, Collazo R 2012 J. Electron. Mater. 42 815
Google Scholar
[85] Klump A, Hoffmann M P, Kaess F, Tweedie J, Reddy P, Kirste R, Sitar Z, Collazo R 2020 J. Appl. Phys. 127 045702
Google Scholar
[86] Ning F, Huang J, Kang J 2023 Phys. Rev. Appl. 19 054046
Google Scholar
[87] Yang J, Wei S H 2019 Chin. Phys. B 28 086106
Google Scholar
-
图 1 周期性边界条件下的缺陷及其镜像(左)和体材料环境中的孤立缺陷(右)
Figure 1. Schematic illustration of a charged defect in a finite supercell under periodic boundary conditions (left) or in an infinite large supercell (right).
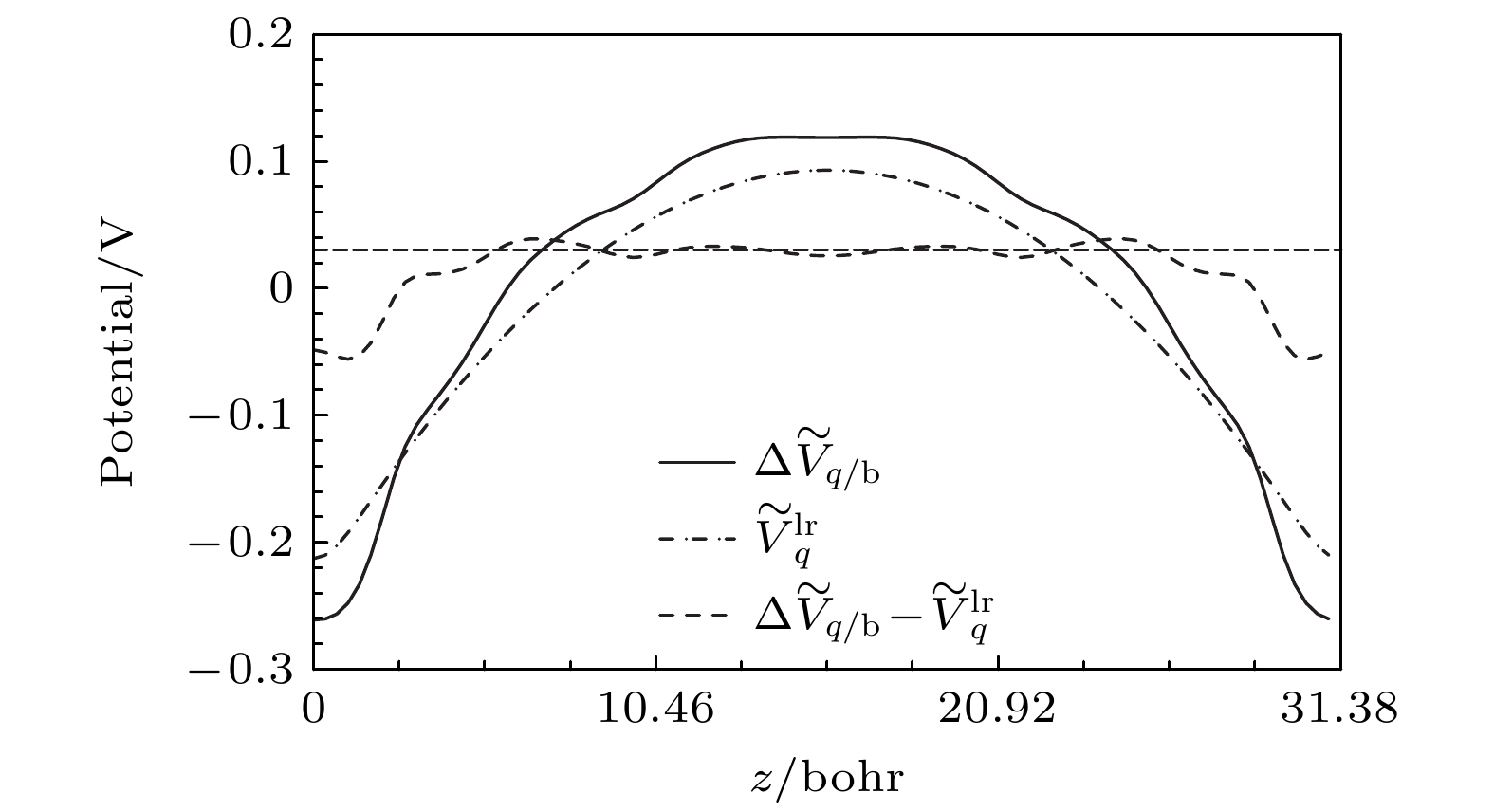
图 2 立方GaAs超胞(3×3×3)中 $ {{{\mathrm{V}}}}_{{{\mathrm{Ga}}}}^{{3-}} $的电势分布, 其中 $ {\Delta \widetilde{{V}}}_{{q}{/b}} $, $ {\widetilde{{V}}}_{{q}}^{{\mathrm{lr}}} $及 $ {\Delta \widetilde{{V}}}_{{q}{/b}}-{\widetilde{{V}}}_{{q}}^{{\mathrm{lr}}} $分别为缺陷的总势场、长程势和短程势, 水平虚线指示潜在的电势对齐项, 缺陷和镜像分别位于z = 0和z = 31.38 Bohr处, 图片引用自参考文献[30] (版权属于美国物理学会)
Figure 2. Potentials for a $ {{{\mathrm{V}}}}_{{{\mathrm{Ga}}}}^{{3-}} $ in a 3×3×3 cubic GaAs supercell. The $ {\Delta \widetilde{{V}}}_{{q}{/b}} $, $ {\widetilde{{V}}}_{{q}}^{{\mathrm{lr}}} $ and $ {\Delta \widetilde{{V}}}_{{q}{/b}}-{\widetilde{{V}}}_{{q}}^{{\mathrm{lr}}} $ are respectively the total, long-range and short-range part, of the defect electrostatic potential. The potential alignment term is indicated by the dashed line. The defect is located at z = 0 Bohr with a periodic image at z = 31.38 Bohr. Reprinted with permission from Ref. [30], copyright 2009 by the American Physical Society.
图 3 锐钛矿相TiO2(001)片层表面 $ {{{\mathrm{O}}}}_{2}^{{-1}} $沿表面法向的面平均电荷和平均静电势分布 (a)未经过SCPC修正; (b)经过SCPC修正, 红色和玫红色曲线分别代表修正前后离子与电子的总势场, 绿色曲线代表修正势, 经过SCPC修正后, 真空内的势场升高, 电荷分布消失; 图片引用自参考文献[31] (版权属于美国物理学会)
Figure 3. Planar average of the extra charge and the electrostatic potential along the surface normal for an $ {{{\mathrm{O}}}}_{2}^{{-1}} $ molecule on the surface of an anatase-TiO2 (001) slab, without (a) and with (b) the SCPC correction. Reprinted with permission from Ref. [31]. Copyright 2021 by the American Physical Society.
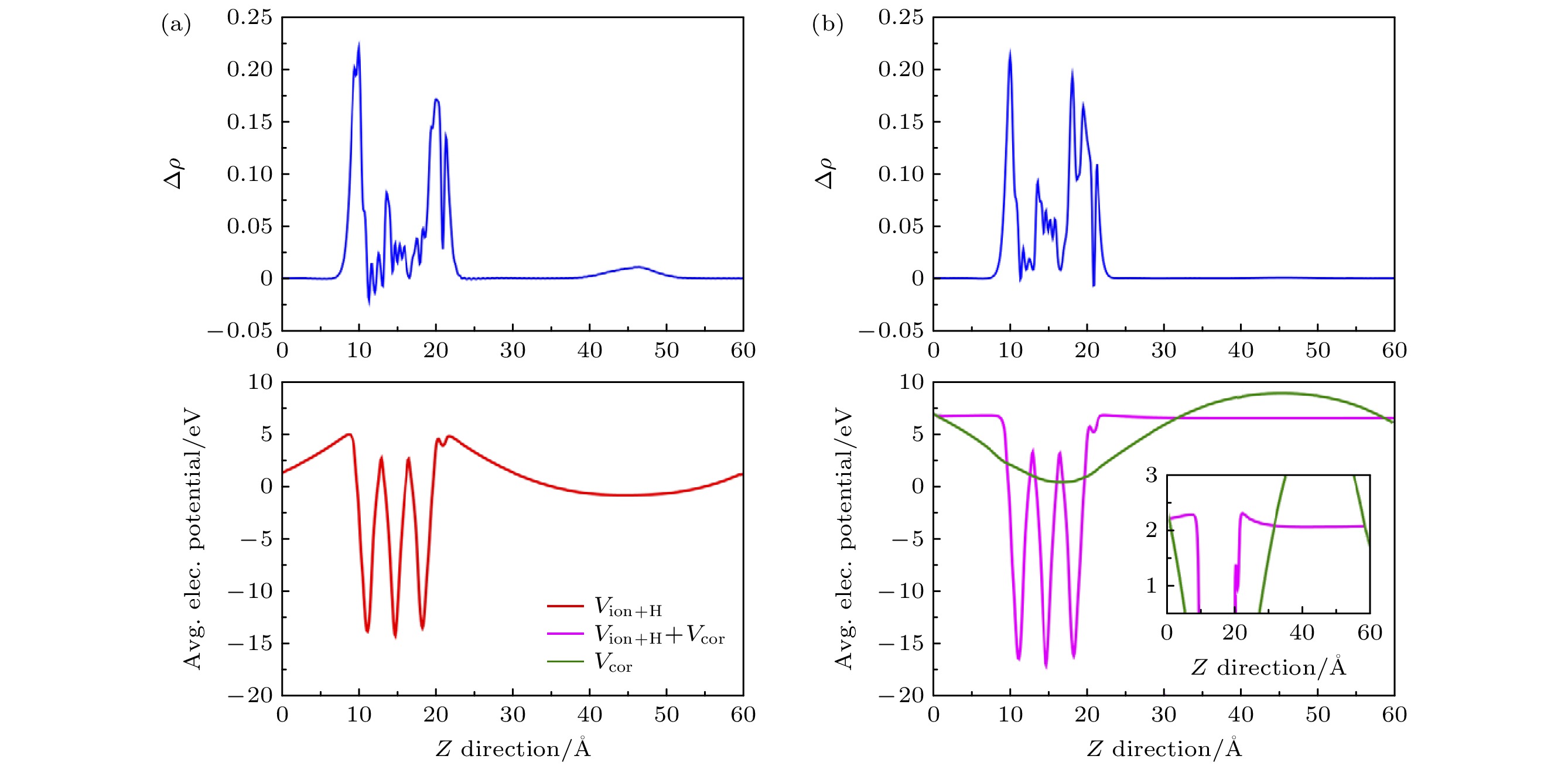
图 4 金刚石超胞(4×4×4)中的 $ {{{\mathrm{V}}}}_{{{\mathrm{C}}}}^{{+1}} $的缺陷电荷密度 (a)屏蔽缺陷电荷密度 $ {{\rho}}_{{{\mathrm{d, sc}}}}^{{{\mathrm{N}}}} $; (b)屏蔽缺陷电荷的核心电荷密度 $ {{\rho}}_{{{\mathrm{d, sc}}}}^{{{\mathrm{N, core}}}} $; (c)无屏蔽的缺陷电荷密度 $ {{\rho}}_{{{\mathrm{d}}}}{}={}{\left|{{\varphi}}_{{{\mathrm{d}}}}\right|}^{2} $, 其中 $ {{\varphi}}_{{{\mathrm{d}}}} $为缺陷态的波函数; (d) $ {{\rho}}_{{{\mathrm{d}}}}{(}{r}{)/}{{ \varepsilon }}_{{\infty}} $ ( $ {{ \varepsilon }}_{{\infty}} $ = 5.62), 图片引用自参考文献[32] (版权属于美国物理学会)
Figure 4. Defect charge distribution for $ {{{\mathrm{V}}}}_{{{\mathrm{C}}}}^{{+1}} $ in a cubic 512-atom diamond supercell (a = 14.13 Å): (a) The screened defect charge density $ {{\rho}}_{{{\mathrm{d, sc}}}}^{{{\mathrm{N}}}} $; (b) core defect charge density $ {{\rho}}_{{{\mathrm{d, sc}}}}^{{{\mathrm{N, core}}}} $; (c) unscreened defect charge density $ {{\rho}}_{{{\mathrm{d}}}}{}={}{\left|{{\varphi}}_{{{\mathrm{d}}}}\right|}^{2} $, where $ {{\varphi}}_{{{\mathrm{d}}}} $ is the wave function of the defect state, and (d) $ {{\rho}}_{{{\mathrm{d}}}}{(}{r}{)/}{{ \varepsilon }}_{{\infty}} $ ( $ {{ \varepsilon }}_{{\infty}} $ = 5.62). Reprinted with permission from Ref. [32], copyright 2020 by the American Physical Society.
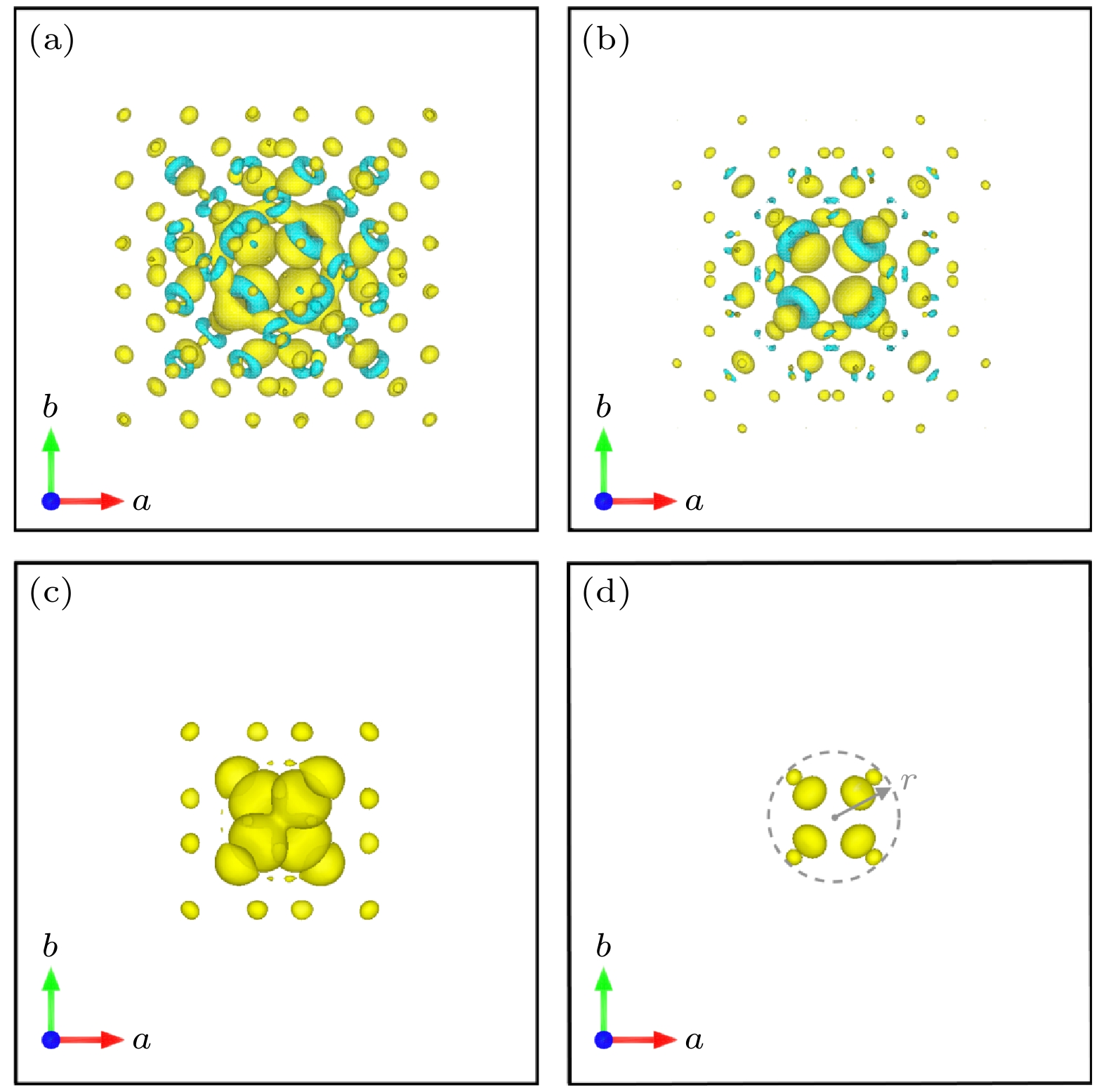
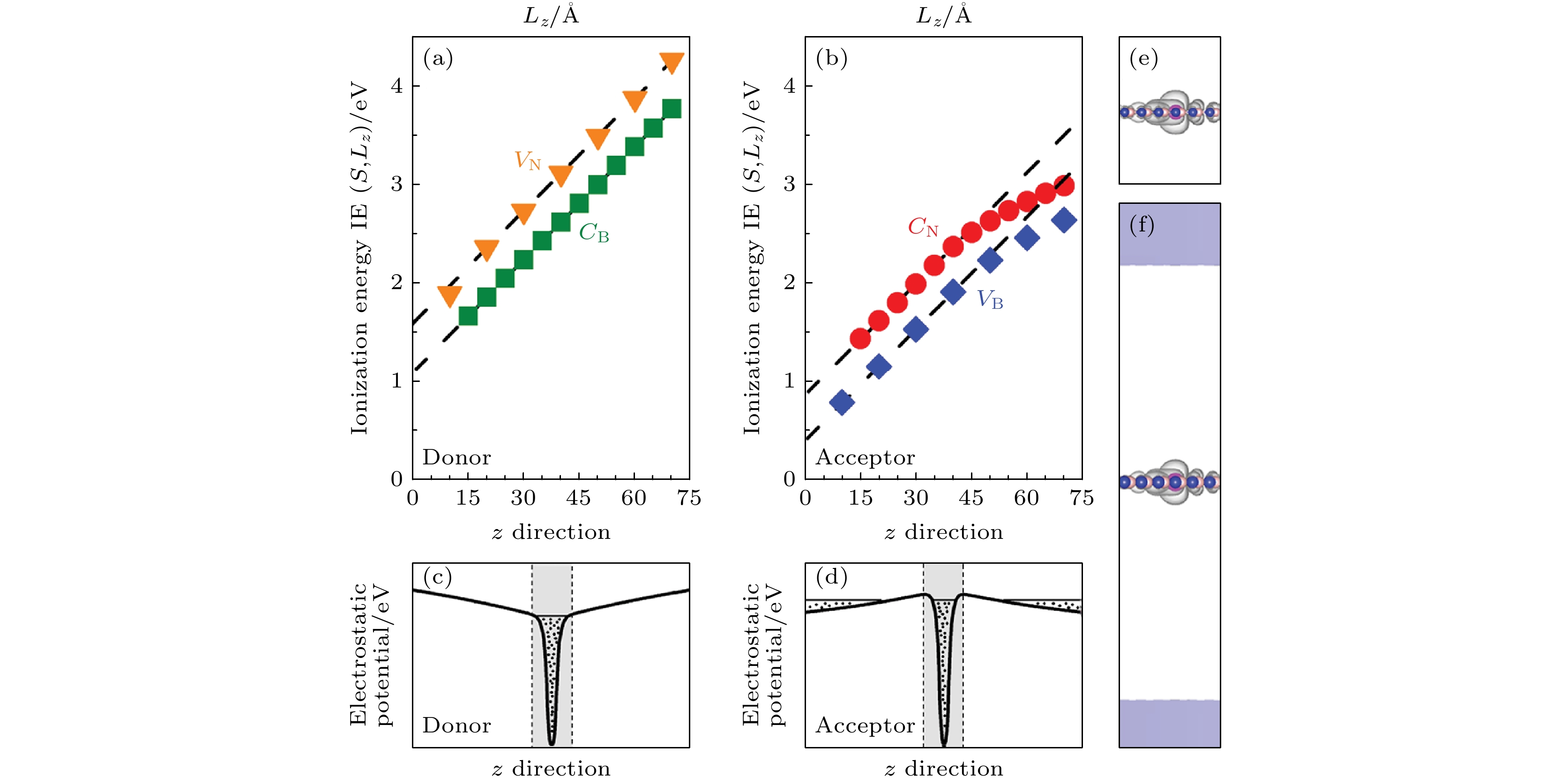
图 5 二维BN的(a)施主(CB和VN)及(b)受主缺陷(CN和VB)的离化能与超胞z方向长度的关系; (c)和(d)分别是施主和受主缺陷在z方向的静电势分布, 水平直线代表费米能级的位置; (e), (f)分别是采用Lz = 20和70 Å的超胞计算的 $ {{{\mathrm{C}}}}_{{{\mathrm{N}}}}^{{-1}} $缺陷的电荷密度分布, (f)中的阴影部分表示电子在虚假真空态的占据. 图片引用自参考文献[45] (版权属于美国物理学会)
Figure 5. Calculated ionization energies of (a) donors, CB and VN , and (b) acceptors, CN and VB in 2D BN, as a function of cell length in z direction (Lz); (c), (d): schematic illustration of the corresponding electrostatic potentials; (e), (f) acceptor state in $ {{{\mathrm{C}}}}_{{{\mathrm{N}}}}^{{-1}} $ at different Lz = 20 and 70 Å, respectively. The shade areas at the top and bottom of panel (f) are the calculated defect states unphysically delocalized into the vacuum. Reprinted with permission from Ref. [45] . Copyright 2015 by the American Physical Society
图 6 (a) 转移真实态模型示意图, 电子从缺陷电离到导带, 导带的电子占据服从费米-狄拉克分布; (b)二维结构缺陷模型在凝胶模型和转移真实态模型电离出的电荷密度分布示意图, 绿色阴影代表凝胶电荷分布 (jellium-CD), 橙色区域点状标记代表真实态的电荷分布 (real-CD); (c) 凝胶模型(JM)和TRSM修正的二维BN中 $ {{{\mathrm{C}}}}_{{{\mathrm{N}}}}^{{1-}} $的缺陷形成能与层间距Lz的关系. 图片引用自参考文献[48] (版权属于美国物理学会)
Figure 6. (a) Schematic plot of the TRSM model, where the electrons excited from the defect state to the conduction band minimum (CBM) follow the Fermi-Dirac distribution; (b) schematic plots of the jellium charge distribution (jellium-CD) and real state charge distributions (real-CD) in a model with a 2D layer (in orange). The jellium and real charges are represented by the green and dotted orange area respectively; (c) formation energies of $ {{{\mathrm{C}}}}_{{{\mathrm{N}}}}^{{1-}} $ in BN monolayer corrected by the jellium model (JM) and TRSM respectively, as functions of layer separation Lz . Reprinted with permission from Ref. [48], copyright 2020 by the American Physical Society.
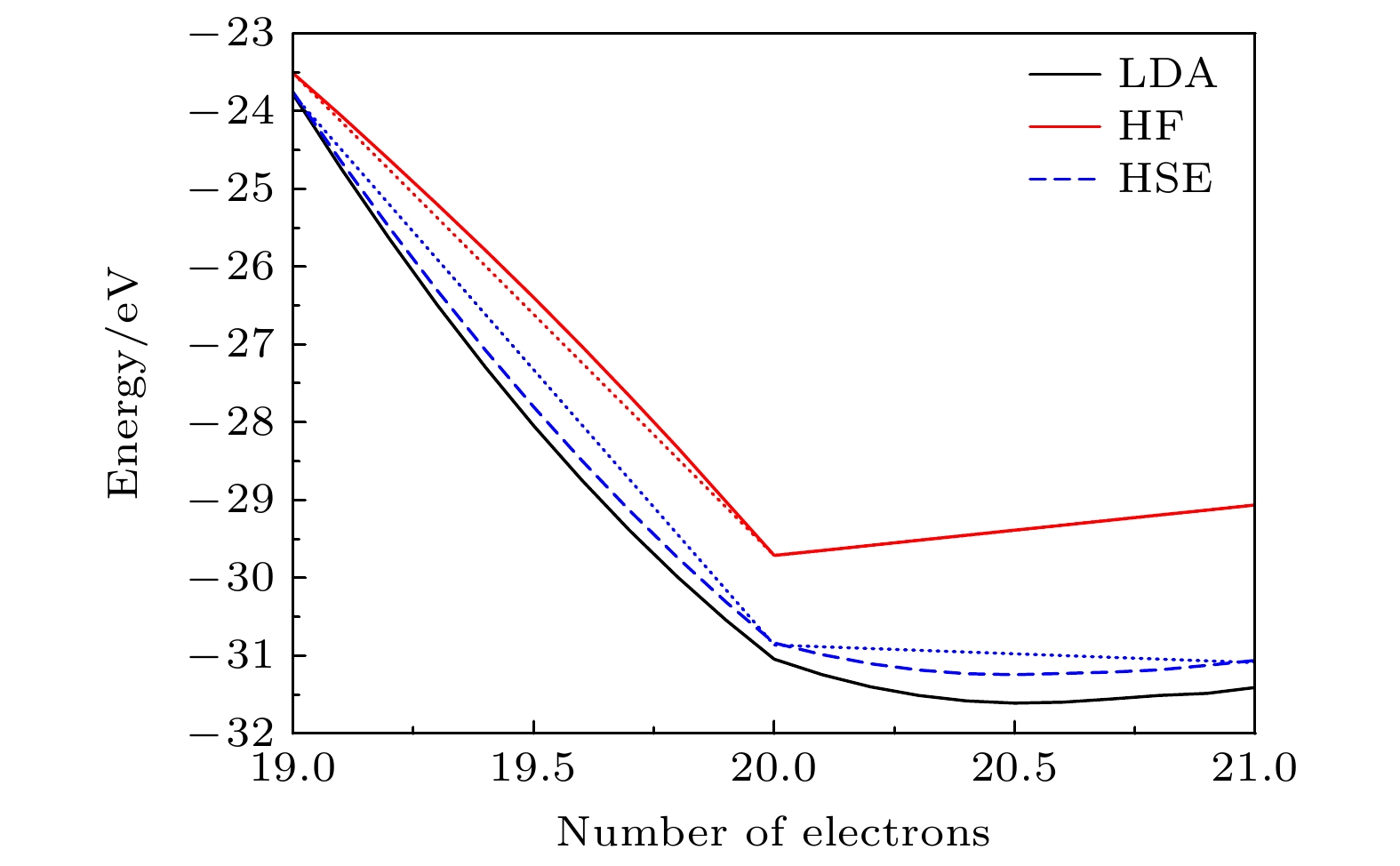
图 7 由LDA、Hartree-Fock理论和HSE混合泛函得到的Si4H4团簇的总能量与电子数量的关系, 点线表示精确解. 图片引用自参考文献[23] (版权属于美国物理学会)
Figure 7. Total energy of a Si4H4 cluster with respect to the number of electrons, for the LDA, Hartree-Fock theory, and hybrid functional (HSE). The dotted straight lines denote the ideal exact solutions. Reprinted with permission from Ref. [23] , copyright 2014 by the American Physical Society.
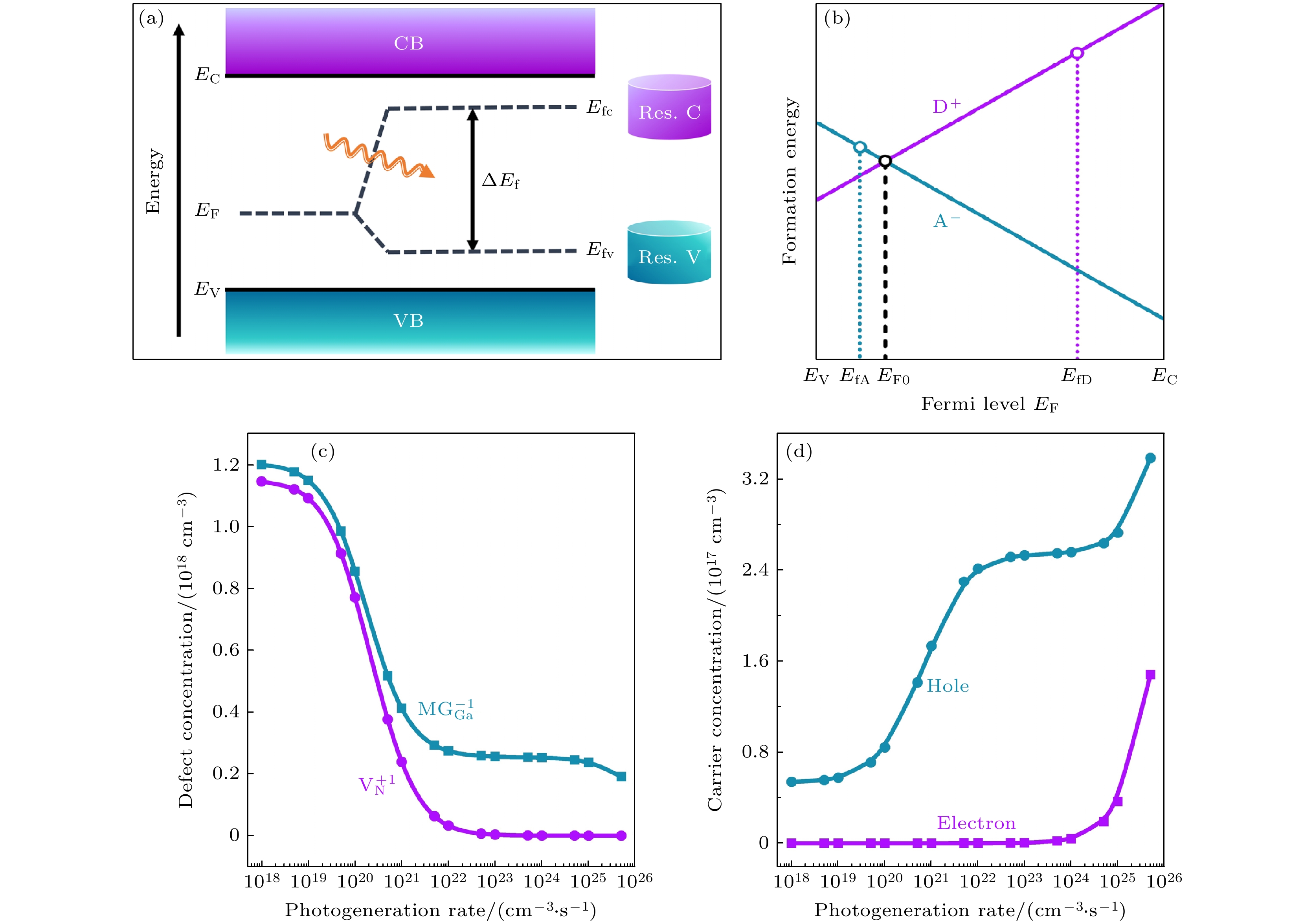
图 8 “双费米库”非平衡缺陷模型及其在GaN体系中的应用 (a)费米能级(EF)在光照条件下分裂成两个准费米能级(Efc及Efv)的示意图; (b) p 型半导体受主A和施主D电离后的缺陷形成能与费米能级的关系示意图; (c) GaN中 $ {{{\mathrm{Mg}}}}_{{{\mathrm{Ga}}}}^{{-1}} $和 $ {{{\mathrm{V}}}}_{{{\mathrm{N}}}}^{{+1}} $带电缺陷的浓度及(d)载流子浓度, 与光生速率G的函数关系, 图片引用自参考文献[79] (版权属于美国物理学会)
Figure 8. Two Fermi reservoir model for the description of nonequilibrium defect formation under illumination. (a) Energy diagram of a semiconductor going from equilibrium to nonequilibrium steady state condition under illumination. In this case, the equilibrium Fermi level (EF) is split into two quasi-Fermi levels (Efc and Efv), which could be treated as two Fermi reservoirs. (b) Schematic plot of the dependence of defect formation energy on the Fermi level for a p-type semiconductor with a hole-producing acceptor A and a hole-killing donor D. (c) Concentrations of charged defects $ {{{\mathrm{Mg}}}}_{{{\mathrm{Ga}}}}^{{-1}} $ and $ {{{\mathrm{V}}}}_{{{\mathrm{N}}}}^{{+1}} $, and (d) carrier concentrations in GaN as a function of the photogeneration rate G. Reprinted with permission from Ref. [79] , copyright 2022 by the American Physical Society
-
[1] Grasser T 2012 Microelectron. Reliab. 52 39
Google Scholar
[2] Grasser T, Kaczer B, Goes W, Reisinger H, Aichinger T, Hehenberger P, Wagner P J, Schanovsky F, Franco J, Luque M T T, Nelhiebel M 2011 IEEE T. Electron Dev. 58 3652
Google Scholar
[3] Krishnan A T, Chancellor C, Chakravarthi S, Nicollian P E, Reddy V, Varghese A, Khamankar R B, Krishnan S 2005 IEEE International Electron Devices Meeting, 2005. IEDM Technical Digest Arazon, USA, December 5, 2005 p4
[4] Breitenstein O, Bauer J, Altermatt P P, Ramspeck K 2009 Solid State Phenom. 156-158 1
Google Scholar
[5] Aydin E, De Bastiani M, De Wolf S 2019 Adv. Mater. 31 1900428
Google Scholar
[6] Park J S, Kim S, Xie Z, Walsh A 2018 Nat. Rev. Mater. 3 194
Google Scholar
[7] Bai S, Zhang N, Gao C, Xiong Y 2018 Nano Energy 53 296
Google Scholar
[8] Jia J, Qian C, Dong Y, Li Y F, Wang H, Ghoussoub M, Butler K T, Walsh A, Ozin G A 2017 Chem. Soc. Rev. 46 4631
Google Scholar
[9] Ulbricht R, Hendry E, Shan J, Heinz T F, Bonn M 2011 Rev. Mod. Phys. 83 543
Google Scholar
[10] deQuilettes D W, Frohna K, Emin D, Kirchartz T, Bulovic V, Ginger D S, Stranks S D 2019 Chem. Rev. 119 11007
Google Scholar
[11] Reshchikov M A, McNamara J D, Toporkov M, Avrutin V, Morkoç H, Usikov A S, Helava H I, Makarov Y N 2016 Sci. Rep. 6 37511
Google Scholar
[12] Vines L, Monakhov E, Kuznetsov A 2022 J. Appl. Phys. 132 150401
Google Scholar
[13] McCluskey M D 2020 J. Appl. Phys. 127 101101
Google Scholar
[14] Tuomisto F 2019 Characterisation and Control of Defects in Semiconductors (London: Institution of Engineering and Technology
[15] Ingebrigtsen M E, Varley J B, Kuznetsov A Y, Svensson B G, Alfieri G, Mihaila A, Badstübner U, Vines L 2018 Appl. Phys. Lett. 112 042104
Google Scholar
[16] Venzie A, Portoff A, Valenzuela E C P, Stavola M, Fowler W B, Pearton S J, Glaser E R 2022 J. Appl. Phys. 131 035706
Google Scholar
[17] Huang H-L, Chae C, Hwang J 2022 J. Appl. Phys. 131 190901
Google Scholar
[18] Watkins G D 1999 Phys. Solid State 41 746
Google Scholar
[19] Willardson R K, Weber E R, Stavola M 1998 Identification of Defects in Semiconductors (Academic Press
[20] Walsh A, Butler K T 2013 Acc. Chem. Res. 47 364
Google Scholar
[21] Le Bahers T, Rérat M, Sautet P 2014 J. Phys. Chem. C 118 5997
Google Scholar
[22] Jain A, Shin Y, Persson K A 2016 Nat. Rev. Mater. 1 15004
Google Scholar
[23] Freysoldt C, Grabowski B, Hickel T, Neugebauer J, Kresse G, Janotti A, Van de Walle C G 2014 Rev. Mod. Phys. 86 253
Google Scholar
[24] Wang L 2019 J. Semicond. 40 091101
Google Scholar
[25] Suo Z, Wang L, Li S, Luo J 2022 J. Semicond. 43 112801
Google Scholar
[26] Cao R, Deng H X, Luo J W, Wei S H 2019 J. Semicond. 40 042102
Google Scholar
[27] Makov G, Payne M C 1995 Phys. Rev. B 51 4014
Google Scholar
[28] Lany S, Zunger A 2008 Phys. Rev. B 78 235104
Google Scholar
[29] Lany S, Zunger A 2009 Modell. Simul. Mater. Sci. Eng. 17 084002
Google Scholar
[30] Freysoldt C, Neugebauer J, Van de Walle C G 2009 Phys. Rev. Lett. 102 016402
Google Scholar
[31] da Silva M C, Lorke M, Aradi B, Farzalipour Tabriz M, Frauenheim T, Rubio A, Rocca D, Deák P 2021 Phys. Rev. Lett. 126 076401
Google Scholar
[32] Suo Z J, Luo J W, Li S S, Wang L W 2020 Phys. Rev. B 102 174110
Google Scholar
[33] Baraff G A, Schlüter M 1984 Phys. Rev. B 30 3460
Google Scholar
[34] Anisimov V I, Zaanen J, Andersen O K 1991 Phys. Rev. B 44 943
Google Scholar
[35] Heyd J, Scuseria G E, Ernzerhof M 2006 J. Chem. Phys. 124 219906
Google Scholar
[36] Heyd J, Scuseria G E, Ernzerhof M 2003 J. Chem. Phys. 118 8207
Google Scholar
[37] Fuchs F, Furthmüller J, Bechstedt F, Shishkin M, Kresse G 2007 Phys. Rev. B 76 115109
Google Scholar
[38] Komsa H P, Rantala T T, Pasquarello A 2012 Phys. Rev. B 86 045112
Google Scholar
[39] Leslie M, Gillan N J 1985 J. Phys. C: Solid State Phys. 18 973
Google Scholar
[40] Castleton C W M, Höglund A, Mirbt S 2006 Phys. Rev. B 73 035215
Google Scholar
[41] Hine N D M, Frensch K, Foulkes W M C, Finnis M W 2009 Phys. Rev. B 79 024112
Google Scholar
[42] Murphy S T, Hine N D M 2013 Phys. Rev. B 87 094111
Google Scholar
[43] Kumagai Y, Oba F 2014 Phys. Rev. B 89 195205
Google Scholar
[44] Freysoldt C, Neugebauer J 2018 Phys. Rev. B 97 205425
Google Scholar
[45] Wang D, Han D, Li X B, Xie S Y, Chen N K, Tian W Q, West D, Sun H-B, Zhang S B 2015 Phys. Rev. Lett. 114 196801
Google Scholar
[46] Wang D, Han D, Li X B, Chen N K, West D, Meunier V, Zhang S, Sun H B 2017 Phys. Rev. B 96 155424
Google Scholar
[47] Richter N A, Sicolo S, Levchenko S V, Sauer J, Scheffler M 2013 Phys. Rev. Lett. 111 045502
Google Scholar
[48] Xiao J, Yang K, Guo D, Shen T, Deng H X, Li S S, Luo J W, Wei S H 2020 Phys. Rev. B 101 165306
Google Scholar
[49] Godby R W, Schlüter M, Sham L J 1986 Phys. Rev. Lett. 56 2415
Google Scholar
[50] Mori-Sánchez P, Cohen A J, Yang W 2008 Phys. Rev. Lett. 100 146401
Google Scholar
[51] Janotti A, Van de Walle C G 2007 Phys. Rev. B 76 165202
Google Scholar
[52] Clark S J, Robertson J 2010 Phys. Status 248 537
Google Scholar
[53] Zanolli Z, Fuchs F, Furthmüller J, von Barth U, Bechstedt F 2007 Phys. Rev. B 75 245121
Google Scholar
[54] Perdew J P 2009 Int. J. Quantum Chem 28 497
Google Scholar
[55] Perdew J P, Levy M 1983 Phys. Rev. Lett. 51 1884
Google Scholar
[56] Sham L J, Schlüter M 1983 Phys. Rev. Lett. 51 1888
Google Scholar
[57] Wang L W 2001 Appl. Phys. Lett. 78 1565
Google Scholar
[58] Zhang G, Canning A, Grønbech-Jensen N, Derenzo S, Wang L W 2013 Phys. Rev. Lett. 110 166404
Google Scholar
[59] Kang J, Wang L W 2022 Phys. Rev. Appl. 18 064001
Google Scholar
[60] Zhang S B, Wei S H, Zunger A 2001 Phys. Rev. B 63 075205
Google Scholar
[61] Zhang S B 2002 J. Phys. : Condens. Matter 14 R881
Google Scholar
[62] Van de Walle C G, Neugebauer J 2004 J. Appl. Phys. 95 3851
Google Scholar
[63] Wei S H, Zunger A 1988 Phys. Rev. B 37 8958
Google Scholar
[64] Janotti A, Van de Walle C G 2010 Phys. Status 248 799
Google Scholar
[65] Cococcioni M, de Gironcoli S 2005 Phys. Rev. B 71 035105
Google Scholar
[66] Boonchun A, Lambrecht W R L 2010 Phys. Status 248 1043
Google Scholar
[67] Becke A D 1993 J. Chem. Phys. 98 1372
Google Scholar
[68] Muscat J, Wander A, Harrison N M 2001 Chem. Phys. Lett. 342 397
Google Scholar
[69] Perdew J P, Ernzerhof M, Burke K 1996 J. Chem. Phys. 105 9982
Google Scholar
[70] Cai X, Zhang P, Wei S H 2019 J. Semicond. 40 092101
Google Scholar
[71] Deák P, Aradi B, Frauenheim T, Janzén E, Gali A 2010 Phys. Rev. B 81 153203
Google Scholar
[72] Davies G, Hamer M F, Price W C 1997 Proceed. of the Royal Soc. London. A. Math. and Phys. Sci. 348 285
Google Scholar
[73] Komsa H P, Pasquarello A 2011 Phys. Rev. B 84 075207
Google Scholar
[74] Blakemore J S 1982 J. Appl. Phys. 53 R123
Google Scholar
[75] Lin L 2016 J. Chem. Theory Comput. 12 2242
Google Scholar
[76] Bang J, Sun Y Y, Abtew T A, Samanta A, Zhang P, Zhang S B 2013 Phys. Rev. B 88 035134
Google Scholar
[77] Bryan Z, Bryan I, Gaddy B E, Reddy P, Hussey L, Bobea M, Guo W, Hoffmann M, Kirste R, Tweedie J, Gerhold M, Irving D L, Sitar Z, Collazo R 2014 Appl. Phys. Lett. 105 222101
Google Scholar
[78] Male J, Agne M T, Goyal A, Anand S, Witting I T, Stevanović V, Snyder G J 2019 Mater. Horiz. 6 1444
Google Scholar
[79] Cai X, Luo J, Li S, Wei S, Deng H 2022 Phys. Rev. B 106 214102
Google Scholar
[80] Harper R L, Hwang S, Giles N C, Schetzina J F, Dreifus D L, Myers T H 1989 Appl. Phys. Lett. 54 170
Google Scholar
[81] Fujita S, Fujita S 1992 J. Cryst. Growth 117 67
Google Scholar
[82] Simpson J, Hauksson I, Wang S Y, Stewart H, Prior K A, Cavenett B C 1993 Physica B 185 164
Google Scholar
[83] Li X, Zhang B, Zhu H, Dong X, Xia X, Cui Y, Huang K, Du G 2008 Appl. Surf. Sci. 254 2081
Google Scholar
[84] Bryan Z, Hoffmann M, Tweedie J, Kirste R, Callsen G, Bryan I, Rice A, Bobea M, Mita S, Xie J, Sitar Z, Collazo R 2012 J. Electron. Mater. 42 815
Google Scholar
[85] Klump A, Hoffmann M P, Kaess F, Tweedie J, Reddy P, Kirste R, Sitar Z, Collazo R 2020 J. Appl. Phys. 127 045702
Google Scholar
[86] Ning F, Huang J, Kang J 2023 Phys. Rev. Appl. 19 054046
Google Scholar
[87] Yang J, Wei S H 2019 Chin. Phys. B 28 086106
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4249
- PDF Downloads: 268
- Cited By: 0
