-
研究了在二维自旋-轨道耦合的相互作用超冷玻色气体中存在一维光晶格时, 超流条纹相到超固相的非平衡动力学. 通过研究这一动力学过程中的缺陷(位相空间中的涡旋)及波函数的变化行为, 利用涡旋数及波函数的交叠等描述方法, 确定了考虑光晶格深度随时间线性变化的量子淬火动力学过程的转变时间. 发现在转变时间之前, 体系对于淬火过程没有响应. 当演化时间超过转变时间后, 系统开始迅速响应, 涡旋数及体系的波函数开始迅速变化. 当演化时间足够长时, 系统将达到稳态. 另外还发现, 在上述动力学过程中, 由于体系中自旋-轨道耦合的存在, 系统在空间中的密度分布与自旋在空间中的结构始终相伴生, 即具有拓扑结构的磁斯格明子(反斯格明子)的中心位置始终与体系密度分布的极小值位置相对应.
-
关键词:
- 自旋-轨道耦合 /
- 量子淬火 /
- 超固体 /
- 斯格明子与反斯格明子
In this work, we study the non-equilibrium quench dynamics from the superfluid stripe phase to the supersolid phase of a two dimensional spin-orbital coupled interacting Bose-Einstein condensate in the presence of a one dimensional optical lattice. The quench protocol here is constructed through varying the lattice depth linearly with the evolution time. By using the time-dependent Gutzwiller method, various physical quantities, such as the vortex number and the overlap of wave-function, have been investigated with respect to the quench time. Through analyzing the dynamical behavior of the above physical quantities, we find out the transition time of the quench procedure, which captures the freeze out time indicating the moment that the system catches the quench speed beginning to evolve quickly. Before the transition time, the dynamics is frozen and the state of the system cannot follow the changes in the Hamiltonian. While passing the transition time, we find that there are significant alterations to both the vortex number and the wave-function. At the transition time, on one hand the vortex number abruptly increases from zero; on the other hand the overlap of wave-function departures from 1 shortly. These signatures indicate that the system evolves rapidly when passing the transition time. Furthermore, we also find that due to the presence of spin-orbital coupling, the spin texture represents a periodic magnetic structure accompanying with the emergence of the supersolid dynamically. It is shown that during the quench procedure, the density distribution of the system are always accompanied with the spatial structure of spin texture, i.e., the central position of topological spin skyrmion (antiskyrmion) corresponding to the minimum position of the density distribution. The topological charge of the above spin structures also shows interesting dynamical properties. We find that the quantized topological charge appears with the emergence of the supersolid dynamically.-
Keywords:
- spin-orbital coupling /
- quantum quench /
- supersolid /
- skyrmion and antiskyrmion
[1] Xiao D, Chang M C, Niu Q 2010 Rev. Mod. Phys. 82 1959
Google Scholar
[2] Nagaosa N, Sinova J, Onoda S, MacDonald A H, Ong N P 2010 Rev. Mod. Phys. 82 1539
Google Scholar
[3] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[4] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[5] Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
Google Scholar
[6] Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301
Google Scholar
[7] Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302
Google Scholar
[8] Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301
Google Scholar
[9] Wu Z, Zhang L, Sun W. Xu X T, Wang B Z, Ji S C, Deng Y J, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[10] Léonaed J, Morales A, Zupancic P, Esslinger T, Donner T 2017 Nature 543 87
Google Scholar
[11] Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F C, Jamison A O, Ketterle W 2017 Nature 543 91
Google Scholar
[12] Gross E P 1957 Phys. Rev. 106 161
Google Scholar
[13] Gross E P 1958 Ann. Phys. 4 57
Google Scholar
[14] Boninsegni M 2009 Phys. Rev. B 79 174203
Google Scholar
[15] Leggett A J 1970 Phys. Rev. Lett. 25 1543
Google Scholar
[16] Clark B K, Ceperley D M 2006 Phys. Rev. Lett. 96 105302
Google Scholar
[17] Ray M W, Hallock R B 2008 Phys. Rev. Lett. 100 235301
Google Scholar
[18] Henkel N, Nath R, Pohl T 2010 Phys. Rev. Lett. 104 195302
Google Scholar
[19] Cinti F, Jain P, Boninsegni M, Micheli A, Zoller P, Pupillo G 2010 Phys. Rev. Lett. 105 135301
Google Scholar
[20] Henkel N, Cinti F, Jain P, Pupillo G, Pohl T 2012 Phys. Rev. Lett. 108 265301
Google Scholar
[21] Wessel S, Troyer M 2005 Phys. Rev. Lett. 95 127205
Google Scholar
[22] Danshita I, Sá de Melo C A R 2009 Phys. Rev. Lett. 103 225301
Google Scholar
[23] Tieleman O, Lazarides A, Smith C M 2011 Phys. Rev. A 83 013627
Google Scholar
[24] Wang H, Li S, Arzamasovs M, Liu W V, Liu B 2022 Phys. Rev. A 105 063301
Google Scholar
[25] Norcia M A, Politi C, Klaus L, Poli E, Sohmen M, Mark M J, Bisset R N, Santos L, Ferlaino F 2021 Nature 596 357
Google Scholar
[26] Bandyopadhyay S, Bai R, Pal S, Suthar K, Nath R, Angom D 2019 Phys. Rev. A 100 053623
Google Scholar
[27] Sable H, Gaur D, Bandyopadhyay S, Nath R, Angom D 2021 arXiv: 2106.01725 [cond-mat.quant-gas]
[28] Shimizu K, Hirano T, Park J, Kuno Y, Ichinose I 2018 Phys. Rev. A 98 063603
Google Scholar
[29] Wang H, He X Y, Li S, Li H R, Liu B 2022 arXiv: 2209.12408 [cond-mat.quant-gas]
[30] Titum P, Iosue J T, Garrison J R, Gorshkov A V, Gong Z X 2019 Phys. Rev. Lett. 123 115701
Google Scholar
[31] Chen Z, Tang T, Austin J, Shaw Z, Zhao L, Liu Y 2019 Phys. Rev. Lett. 123 113002
Google Scholar
[32] Bloch I, Dalibard J, Zwerger W 2008 Rev. Mod. Phys. 80 885
Google Scholar
[33] Polkovnikov A, Sengupta K, Silva A, Vengalattore M 2011 Rev. Mod. Phys. 83 863
Google Scholar
[34] Goo J, Lim Y, Shin Y 2021 Phys. Rev. Lett. 127 115701
Google Scholar
[35] Huang Q, Yao R X, Liang L B, Wang S, Zheng Q P, Li D P, Xiong W, Zhou X J, Chen W L, Chen X Z, Hu J Z 2021 Phys. Rev. Lett. 127 200601
Google Scholar
[36] Shimizu K, Kuno Y, Hirano T, Ichinose I 2018 Phys. Rev. A 97 033626
Google Scholar
[37] Shimizu K, Hirano T, Park J, Kuno Y, Ichinose I 2018 New J. Phys. 20 083006
Google Scholar
[38] Goffe W L, Ferrier G D, Rogers J 1994 J. Econometrics 60 65
Google Scholar
[39] Kirkpatrick S, Gelatt C D, Vecchi M P 1983 Science 220 4598
Google Scholar
[40] Li S, Wang H, Li F L, Cui X L, Liu B 2020 Phys. Rev. A 102 033328
Google Scholar
[41] Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402
Google Scholar
[42] Choi S, Morgan S A, Burnett K 1998 Phys. Rev. A 57 4057
Google Scholar
[43] Tsubota M, Kasamatsu K, Ueda M 2002 Phys. Rev. A 65 023603
Google Scholar
[44] Antoine X, Duboscq R 2015 Comput. Phys. Commun. 193 95
Google Scholar
[45] Einkemmer L, Ostermann A 2014 SIAM J. Numer. Anal. 52 140
Google Scholar
[46] Kasamatsu K, Tsubota M, Ueda M 2004 Phys. Rev. Lett. 93 250406
Google Scholar
[47] Aftalion A, Mason P 2013 Phys. Rev. A 88 023610
Google Scholar
[48] Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B 50 155301
Google Scholar
[49] Liu C F, Fan H, Zhang Y C, Wang D S, Liu W M 2012 Phys. Rev. A 86 053616
Google Scholar
[50] Girvin S M 2000 Phys. Today 53 39
Google Scholar
[51] Kasamatsu K, Tsubota M, Ueda M 2005 Phys. Rev. A 71 043611
Google Scholar
-
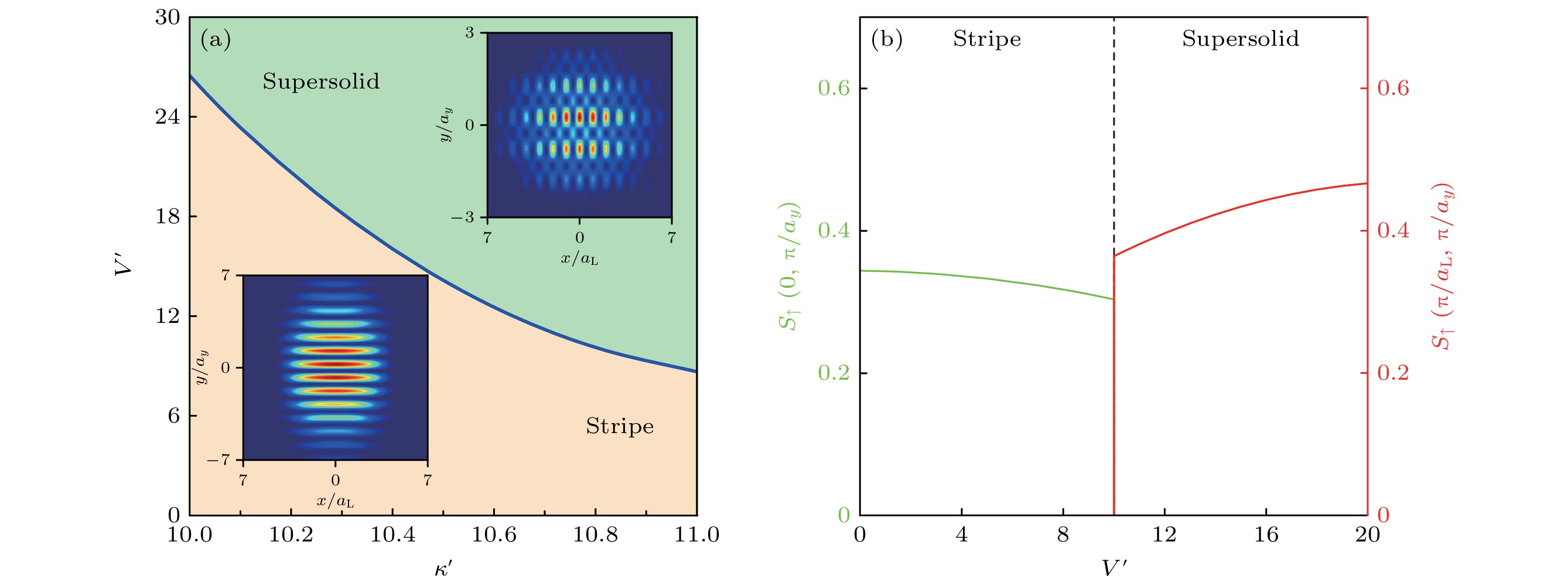
图 1 (a)存在一维光晶格束缚的二维SOC相互作用超冷玻色气体的零温平衡态相图. 相图中包含两种平衡态物相分别为超流条纹相和超固相. 左下角和右上角的插图分别代表超流条纹相和超固相中自旋向上组分的密度分布, 其中超流条纹相的密度涨落只在空间中的一个方向即y方向存在, 而超固相是在整个二维空间形成周期分布. 插图中的超流条纹相是在
$ V^{\prime}=0 $ ,$ \kappa^{\prime}=10.8 $ 的情况下, 而超固相则是在$ V^{\prime}=20 $ ,$ \kappa^{\prime}=10.8 $ 的情况下. 另外, 哈密顿量中的其他参数取为$ c_{0}^{\prime}=10 $ ,$ c_{2}^{\prime}=-0.8 c_{0}^{\prime} $ 和$ k_{{\rm{L}}}^{\prime}=3.5 \pi $ . (b)结构因子$ S_{\sigma }({\boldsymbol{k}}) $ 随光晶格深度的变化, 这里取$ \kappa^{\prime}=10.8 $ , 其他参数与图(a)一致Fig. 1. (a) Equilibrium zero-temperature phase diagram as a function of the SOC strength and lattice depth. The phase diagram consists of two different phases, which are the superfluid stripe phase and supersolid phase. The insets show the density distribution of spin-up atoms for the superfluid stripe phase and supersolid phase, respectively. Here, we choose
$ V^{\prime}=0 $ ,$\kappa^{\prime}=10.8 $ for the superfluid stripe phase and$V^{\prime}=20, \kappa^{\prime}=10.8 $ for the supersolid phase. Other parameters are chosen as$c_{0}^{\prime}=10, c_{2}^{\prime}=-0.8c_{0}^{\prime} $ and$ k_{\mathrm{L}}^{\prime}=3.5 \pi $ . (b) Structure factor as a function of the lattice depth. Here,$\kappa^{\prime}=10.8 $ , other parameters are the same as in panel (a).
图 2 (a) 涡旋数Nv随演化时间的变化行为, 虚线的位置对应于转变时间
$ {\hat{t}} $ . 这里选取$ \tau_{{\rm{Q}}}=20 $ ,$ V_{{\rm{i}}}^{\prime}=0 $ ,$ V_{{\rm{f}}}^{\prime}=20 $ ,$ \gamma=0.03 $ . (b), (c) 分别为$ t'=-20 $ 和$ t'=260 $ 时刻自旋向上组分的波函数的相位角分布, 其中$ t'=-20=-\tau_{{\rm{Q}}} $ 代表系统演化的初始时刻. 哈密顿量中的其他参数与图1(a)一致Fig. 2. (a) Vortex number Nv as a function of the evolution time. The dashed line corresponds to the transition time
${\hat{t}} $ . Here, we choose τQ = 20,$V_{{\rm{i}}}^{\prime}=0, V_{{\rm{f}}}^{\prime}=20 $ and γ = 0.03. (b), (c) Snapshots of phase of the spin-up wave-function at$ t'=-20 $ and$ t'=260 $ , respectively, where t′ = −20 = −τQ represents the initial time. Other parameters are the same as in Fig. 1(a)
图 3 (a) 波函数交叠
$ O(t') $ 随转变时间$ {\hat{t}} $ 的变化行为, 当$ O(t') $ 开始偏离1时, 所对应的$ {\hat{t}} $ 与图2(a) 中的相同. (b) 系统的转变时间$ {\hat{t}} $ 随γ的变化行为. 哈密顿量中的其他参数与图2一致Fig. 3. (a) Overlap of the wave-function
$ O(t') $ as a function of the transition time. When$O(t') $ begins to deviate from 1, the corresponding$ {\hat{t}}$ is the same as in Fig. 2(a). (b) Transition time$ {\hat{t}} $ as a function of γ. Other parameters are the same as in Fig. 2
图 4 (a)—(c)自旋纹理的动力学演化行为, 对应的演化时间分别为
$ t'=4,\; 80,\; 260 $ .$ xy $ 平面内的矢量$ {\boldsymbol S}_{{\rm{in-plane}}} $ 用来描述自旋密度矢量在$ xy $ 平面内的分量, 颜色表示z方向的分量$ {\boldsymbol S}_{z} $ . (d)—(f)系统沿x方向上的密度分布, 实线和虚线分别对应自旋两组分沿x方向的密度分布, y方向的位置分别由(a)—(c)中的实线和虚线标定. (g)—(i)拓扑荷密度分布. (a)—(i)中的‘*’和方块标记空间中的相同位置. 哈密顿量中的其他参数与图2一致Fig. 4. (a)–(c) Spin texture as a function of the evolution time for t′ = 4, 80, 260, respectively. Here, the vector Sin−plane describes the xy-plane component of the spin density vector and the color represents the z-direction component. (d)–(f) Density distribution along x-direction, where the solid line and dashed line correspond to the density distribution of the two components along the x-direction, respectively. (g)–(i) Topological charge density. Here, the ‘*’ and square mark the same spatial position as shown in panel (a)–(i). Other parameters are the same as in Fig. 2
-
[1] Xiao D, Chang M C, Niu Q 2010 Rev. Mod. Phys. 82 1959
Google Scholar
[2] Nagaosa N, Sinova J, Onoda S, MacDonald A H, Ong N P 2010 Rev. Mod. Phys. 82 1539
Google Scholar
[3] Qi X L, Zhang S C 2011 Rev. Mod. Phys. 83 1057
Google Scholar
[4] Hasan M Z, Kane C L 2010 Rev. Mod. Phys. 82 3045
Google Scholar
[5] Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83
Google Scholar
[6] Wang P J, Yu Z Q, Fu Z K, Miao J, Huang L H, Chai S J, Zhai H, Zhang J 2012 Phys. Rev. Lett. 109 095301
Google Scholar
[7] Cheuk L W, Sommer A T, Hadzibabic Z, Yefsah T, Bakr W S, Zwierlein M W 2012 Phys. Rev. Lett. 109 095302
Google Scholar
[8] Zhang J Y, Ji S C, Chen Z, Zhang L, Du Z D, Yan B, Pan G S, Zhao B, Deng Y J, Zhai H, Chen S, Pan J W 2012 Phys. Rev. Lett. 109 115301
Google Scholar
[9] Wu Z, Zhang L, Sun W. Xu X T, Wang B Z, Ji S C, Deng Y J, Chen S, Liu X J, Pan J W 2016 Science 354 83
Google Scholar
[10] Léonaed J, Morales A, Zupancic P, Esslinger T, Donner T 2017 Nature 543 87
Google Scholar
[11] Li J R, Lee J, Huang W, Burchesky S, Shteynas B, Top F C, Jamison A O, Ketterle W 2017 Nature 543 91
Google Scholar
[12] Gross E P 1957 Phys. Rev. 106 161
Google Scholar
[13] Gross E P 1958 Ann. Phys. 4 57
Google Scholar
[14] Boninsegni M 2009 Phys. Rev. B 79 174203
Google Scholar
[15] Leggett A J 1970 Phys. Rev. Lett. 25 1543
Google Scholar
[16] Clark B K, Ceperley D M 2006 Phys. Rev. Lett. 96 105302
Google Scholar
[17] Ray M W, Hallock R B 2008 Phys. Rev. Lett. 100 235301
Google Scholar
[18] Henkel N, Nath R, Pohl T 2010 Phys. Rev. Lett. 104 195302
Google Scholar
[19] Cinti F, Jain P, Boninsegni M, Micheli A, Zoller P, Pupillo G 2010 Phys. Rev. Lett. 105 135301
Google Scholar
[20] Henkel N, Cinti F, Jain P, Pupillo G, Pohl T 2012 Phys. Rev. Lett. 108 265301
Google Scholar
[21] Wessel S, Troyer M 2005 Phys. Rev. Lett. 95 127205
Google Scholar
[22] Danshita I, Sá de Melo C A R 2009 Phys. Rev. Lett. 103 225301
Google Scholar
[23] Tieleman O, Lazarides A, Smith C M 2011 Phys. Rev. A 83 013627
Google Scholar
[24] Wang H, Li S, Arzamasovs M, Liu W V, Liu B 2022 Phys. Rev. A 105 063301
Google Scholar
[25] Norcia M A, Politi C, Klaus L, Poli E, Sohmen M, Mark M J, Bisset R N, Santos L, Ferlaino F 2021 Nature 596 357
Google Scholar
[26] Bandyopadhyay S, Bai R, Pal S, Suthar K, Nath R, Angom D 2019 Phys. Rev. A 100 053623
Google Scholar
[27] Sable H, Gaur D, Bandyopadhyay S, Nath R, Angom D 2021 arXiv: 2106.01725 [cond-mat.quant-gas]
[28] Shimizu K, Hirano T, Park J, Kuno Y, Ichinose I 2018 Phys. Rev. A 98 063603
Google Scholar
[29] Wang H, He X Y, Li S, Li H R, Liu B 2022 arXiv: 2209.12408 [cond-mat.quant-gas]
[30] Titum P, Iosue J T, Garrison J R, Gorshkov A V, Gong Z X 2019 Phys. Rev. Lett. 123 115701
Google Scholar
[31] Chen Z, Tang T, Austin J, Shaw Z, Zhao L, Liu Y 2019 Phys. Rev. Lett. 123 113002
Google Scholar
[32] Bloch I, Dalibard J, Zwerger W 2008 Rev. Mod. Phys. 80 885
Google Scholar
[33] Polkovnikov A, Sengupta K, Silva A, Vengalattore M 2011 Rev. Mod. Phys. 83 863
Google Scholar
[34] Goo J, Lim Y, Shin Y 2021 Phys. Rev. Lett. 127 115701
Google Scholar
[35] Huang Q, Yao R X, Liang L B, Wang S, Zheng Q P, Li D P, Xiong W, Zhou X J, Chen W L, Chen X Z, Hu J Z 2021 Phys. Rev. Lett. 127 200601
Google Scholar
[36] Shimizu K, Kuno Y, Hirano T, Ichinose I 2018 Phys. Rev. A 97 033626
Google Scholar
[37] Shimizu K, Hirano T, Park J, Kuno Y, Ichinose I 2018 New J. Phys. 20 083006
Google Scholar
[38] Goffe W L, Ferrier G D, Rogers J 1994 J. Econometrics 60 65
Google Scholar
[39] Kirkpatrick S, Gelatt C D, Vecchi M P 1983 Science 220 4598
Google Scholar
[40] Li S, Wang H, Li F L, Cui X L, Liu B 2020 Phys. Rev. A 102 033328
Google Scholar
[41] Ji A C, Liu W M, Song J L, Zhou F 2008 Phys. Rev. Lett. 101 010402
Google Scholar
[42] Choi S, Morgan S A, Burnett K 1998 Phys. Rev. A 57 4057
Google Scholar
[43] Tsubota M, Kasamatsu K, Ueda M 2002 Phys. Rev. A 65 023603
Google Scholar
[44] Antoine X, Duboscq R 2015 Comput. Phys. Commun. 193 95
Google Scholar
[45] Einkemmer L, Ostermann A 2014 SIAM J. Numer. Anal. 52 140
Google Scholar
[46] Kasamatsu K, Tsubota M, Ueda M 2004 Phys. Rev. Lett. 93 250406
Google Scholar
[47] Aftalion A, Mason P 2013 Phys. Rev. A 88 023610
Google Scholar
[48] Wang H, Wen L H, Yang H, Shi C X, Li J H 2017 J. Phys. B 50 155301
Google Scholar
[49] Liu C F, Fan H, Zhang Y C, Wang D S, Liu W M 2012 Phys. Rev. A 86 053616
Google Scholar
[50] Girvin S M 2000 Phys. Today 53 39
Google Scholar
[51] Kasamatsu K, Tsubota M, Ueda M 2005 Phys. Rev. A 71 043611
Google Scholar
下载:
计量
- 文章访问数: 3907
- PDF下载量: 175
- 被引次数: 0
