-
The superconducting solenoid with constant large current is exposed to an alternating magnetic field during the acceleration of the superconducting maglev train, which will cause flux jump of the superconducting solenoid. It can reduce the current-carrying capacity of the solenoid, and generate a lot of heat and make the temperature of the superconducting solenoid rise sharply, which will make the whole superconducting coils quenched. Thus the research of flux jump has very important scientific significance. Nb3Sn superconducting wire is a composite structure composed of multiple superconducting filaments、copper and epoxy resin. In this paper, the magneto-thermal instability behavior of a three-dimensional superconducting wires under alternating magnetic fields and constant current is studied by using a two-dimensional model in which the net current of each filament is constrained to zero. By analyzing the effect of amplitude and frequency of alternating magnetic field on flux jump of a Nb3Sn superconducting wire, we find that when the magnetic field amplitude keeps unchanged, the magnetic field threshold of the initial flux jump changes non-monotonically with the frequency. While the frequency keeps unchanged, the threshold of the initial flux jump changes monotonously with the amplitude of the alternating magnetic field. In addition, with the decreasing applied field, the frequency range for flux jump first increases then decreases to certain critical frequency when the superconducting wire does not have flux jumps. The results of this paper can provide a theoretical basis for regulating the magneto-thermal instability of superconducting wires.
-
Keywords:
- Nb3Sn multifilamentary composite superconducting wire /
- flux jump /
- alternating magnetic field /
- maglev system
[1] 杨晶, 朴明伟, 高文斌, 傅凯, 高辉 2019 计算机集成制造系统 25 1908
 Google Scholar
Google Scholar
Yang J, Piao M W, Gao W B, Fu K, Gao H 2019 Comput. Integr. Manuf. Syst. 25 1908
 Google Scholar
Google Scholar
[2] 熊嘉阳, 邓自刚 2021 交通运输工程学报 21 177
 Google Scholar
Google Scholar
Xiong J Y, Deng Z G 2021 J. Traffic Transp. Eng. 21 177
 Google Scholar
Google Scholar
[3] Saijo T, Koike S, Tadakuma S 1981 IEEE Trans. Ind. Appl. 17 533
 Google Scholar
Google Scholar
[4] Kyotani Y 1988 IEEE Trans. Magn. 24 804
 Google Scholar
Google Scholar
[5] Meins J, Miller L, Mayer W 1988 IEEE Trans. Magn. 24 808
 Google Scholar
Google Scholar
[6] Schultz L, de Haas O, Verges P, Beyer C, Rohlig S, Olsen H, Kuhn L, Berger D, Noteboom U, Funk U 2005 IEEE Trans. Appl. Supercond. 15 2301
 Google Scholar
Google Scholar
[7] Okano M, Iwamoto T, Furuse M, Fuchino S, Ishii I 2006 J. Phys.: Conf. Ser. 43 999
 Google Scholar
Google Scholar
[8] Kusada S, Igarashi M, Nemoto K, Okutomi T, Hirano S, Kuwano K, Tominaga T, Terai M, Kuriyama T, Tasaki K 2007 IEEE Trans. Appl. Supercond. 17 2111
 Google Scholar
Google Scholar
[9] Wang J, Wang S, Zeng Y, Huang H, Luo F, Xu Z, Tang Q, Lin G, Zhang C, Ren Z 2002 Physica C 378 809
 Google Scholar
Google Scholar
[10] Stumberger G, Aydemir M T, Zarko D, Lipo T A 2004 IEEE Trans. Appl. Supercond. 14 54
 Google Scholar
Google Scholar
[11] 胡赣娟 2005 硕士 (浙江: 浙江大学)
Hu G J 2005 M. S. Thesis (Zhejiang: Zhejiang University) (in Chinese)
[12] Turman B, Marder B, Rohwein G, Aeschliman D, KelIey J, Cowan M, Zimmerman R 1995 Sandia National Laboratories, the United States Department of Energy
[13] 闻海虎 2021 70 143
 Google Scholar
Google Scholar
Wen H H 2021 Acta Phys. Sin. 70 143
 Google Scholar
Google Scholar
[14] Mints R 1996 Phys. Rev. B 53 12311
 Google Scholar
Google Scholar
[15] Kim Y, Hempstead C, Strnad A 1963 Phys. Rev. 129 528
 Google Scholar
Google Scholar
[16] Neuringer L, Shapira Y 1966 Phys. Rev. 148 231
 Google Scholar
Google Scholar
[17] Wertheimer M R, Gilchrist J le G 1967 J. Phys. Chem. Solids 28 2509
 Google Scholar
Google Scholar
[18] Mints R, Rakhmanov A 1981 Rev. Mod. Phys. 53 551
 Google Scholar
Google Scholar
[19] Wipf S L 1991 Cryog. 31 936
 Google Scholar
Google Scholar
[20] Nabialek A, Niewczas M, Dabkowska H, Dabkowski A, Castellan J, Gaulin B 2003 Phys. Rev. B 67 024518
 Google Scholar
Google Scholar
[21] Hirano T, Fujishiro H, Naito T, Ainslie M D 2020 Supercond. Sci. Technol. 33 044003
 Google Scholar
Google Scholar
[22] Zhou Y H, Yang X B 2006 Phys. Rev. B 74 054507
 Google Scholar
Google Scholar
[23] Yang X B, Zhou Y H, Tu S D 2010 Physica C 470 109
 Google Scholar
Google Scholar
[24] Swartz P, Bean C 1968 J. Appl. Phys. 39 4991
 Google Scholar
Google Scholar
[25] Bean C P 1962 Phys. Rev. Lett. 8 250
 Google Scholar
Google Scholar
[26] Müller K H, Andrikidis C 1994 Phys. Rev. B 49 1294
 Google Scholar
Google Scholar
[27] Gou X F, Zheng X J, Zhou Y H 2007 IEEE Trans. Appl. Supercond. 17 3795
 Google Scholar
Google Scholar
[28] Kashikhin V V, Zlobin A V 2005 IEEE Trans. Appl. Supercond. 15 1621
 Google Scholar
Google Scholar
[29] Zhang W W, Xia J, Yong H D, Zhou Y H 2020 AIP Adv. 10 025021
 Google Scholar
Google Scholar
[30] Zhao Y F, Xia T D, He T H, Feng W J 2010 J. Low Temp. Phys. 160 201
 Google Scholar
Google Scholar
[31] Wang Q Y, Xue C, Chen Y Q, Ou X J, Wu W, Liu W, Ma P, Sun L T, Zhao H W, Zhou Y H 2022 Physica C 593 1354002
 Google Scholar
Google Scholar
[32] Lahtinen V, Stenvall A 2013 IEEE Trans. Appl. Supercond. 24 1
 Google Scholar
Google Scholar
[33] Barzi E, Limon P J, Yamada R, Zlobin A V 2001 IEEE Trans. Appl. Supercond. 11 3595
 Google Scholar
Google Scholar
-
图 1 (a)单根
$ {\text{Nb}}_3{\text{Sn}} $ 超导线横截面几何形状及相关尺寸(单位: mm)示意图, 选取了超导线横截面中间的线段$ A-A $ 来展示超导线的电流密度和磁通密度分布. 超导线置于沿着y方向的垂直交变磁场中, 交变磁场形式如图(b)所示Figure 1. (a) Schematic diagram of the section cross of single
$ {\text{Nb}}_3{\text{Sn}} $ superconducting wire (unit: mm). We select a line$ A-A $ in the middle of the cross section to show the current density and flux density distribution of the superconducting wire. The superconducting wire is exposed to a perpendicular magnetic field along y axis. The form of the alternating magnetic field is shown in panel (b)图 3 为了验证本文采用的H法的准确性, 分别使用H法和
$H\text-\varphi$ 法模拟三根超导芯丝在$ 2.5 $ 和$ 5\; {\text{s}} $ 时的(a)电流密度$ J_z $ 、(b)磁通密度$ B_y $ , 以及(c)三根芯丝的发热功率随时间的变化Figure 3. In order to verify the H formula adopted by this paper, (a) the current density
$ J_z $ , (b) flux density$ B_y $ at$ 2.5 $ and$5\; {\rm{s}}$ , and (c) the variations of total thermal power of the three filaments versus time were conducted both by H and$H \text-\varphi$ formulas图 5 超导线发生跳跃时的(a)平均电场
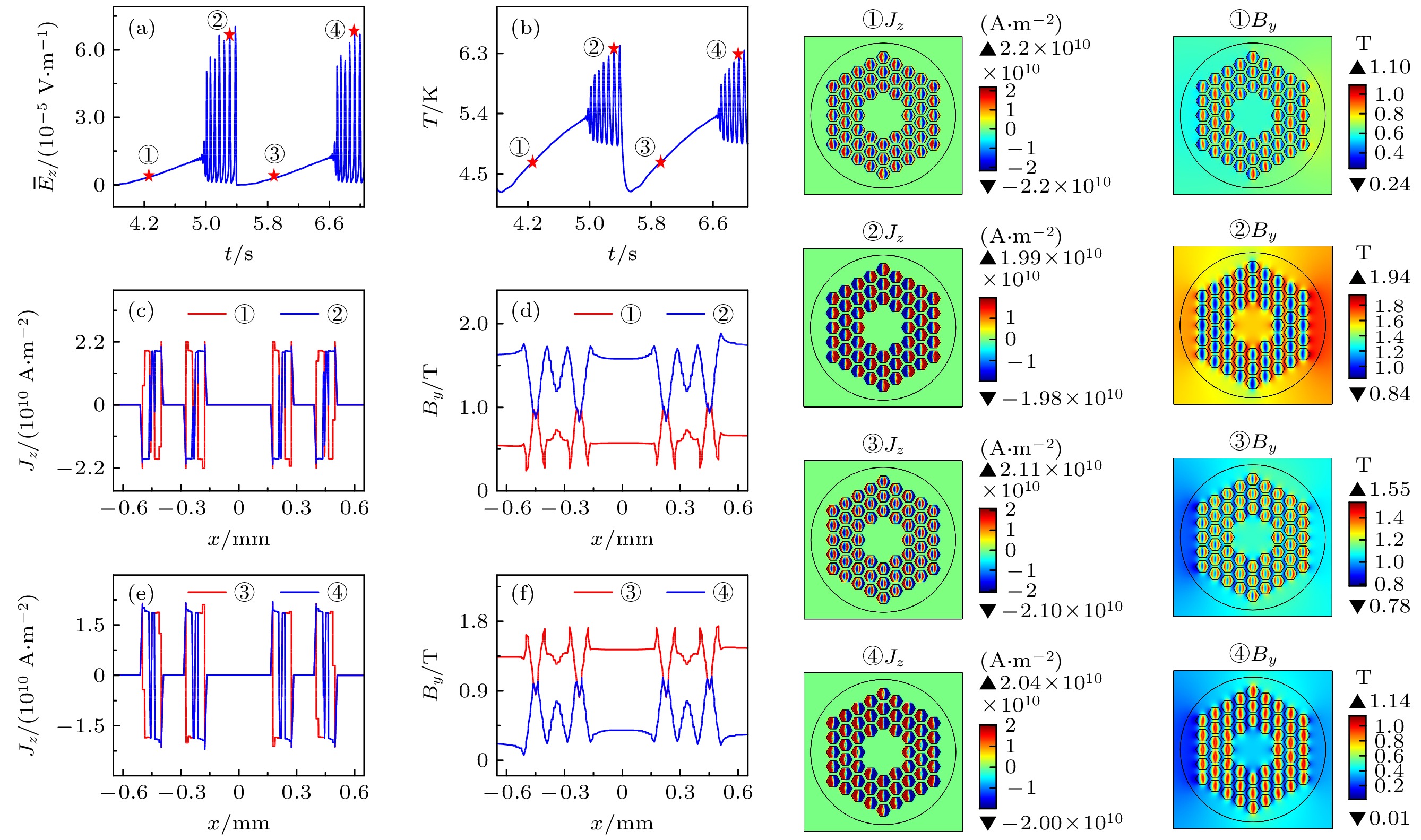
$ \bar{E_z} $ 和(b)平均温度T随时间的变化曲线; (c)和(d)分别展示了上升阶段$\bar{E_z}\text-t$ 或者$T\text-t$ 曲线对应时刻超导线中心$ A-A $ 线的电流密度$ J_z $ 和磁通密度$ B_y $ 分布; (e)和(d)分别展示了下降段电流密度$ J_z $ 和磁通密度$ B_y $ 分布, 插图①—④分别展示了在$T\text-t$ 曲线上对应时刻单根芯丝的电流密度和磁通密度图Figure 5. The variations of (a) average electric field
$ \bar{E_z} $ and (b) average temperature T as a function of time when flux jump occurs in the superconducting wire. (c) and (d) show the distributions of current density$ J_z $ and flux density$ B_y $ at the center of superconducting wire in ascending branch at oscillating times indicated in$\bar{E_z}\text-t$ or$T\text-t$ curve. (e) and (d) show the current density$ J_z $ and flux density$ B_y $ distributions in the descending branch. The insets ①–④ show the contours of current density and flux density of a single filament at times indicated in the$T\text-t$ curve图 6 超导线发生跳跃时的(a)平均电场
$ \bar{E_z} $ 和(b)平均温度T随时间的变化曲线, (c)和(d)分别展示了上升段阶段$\bar{E_z}\text-t$ 或者$T\text-t$ 曲线上未震荡和发生震荡对应时刻电流密度$ J_z $ 和磁通密度$ B_y $ 分布; (e)和(d)分别展示了下降段未震荡和发生震荡时的电流密度$ J_z $ 和磁通密度$ B_y $ 分布, 插图①—④分别展示了在$T\text-t$ 曲线上对应时刻整根超导线的电流密度和磁通密度图Figure 6. The variations of (a) average electric field
$ \bar{E_z} $ and (b) average temperature T as a function of time when flux jump occurs in the superconducting wire. (c) and (d) show the distributions of current density$ J_z $ and flux density$ B_y $ at the center of superconducting wire in ascending branch at no oscillating and oscillating times in$\bar{E_z}\text-t$ or$T\text-t$ curve. (e) and (d) show the current density$ J_z $ and flux density$ B_y $ distributions in the descending branch. The insets ①–④ show the contours of current density and flux density of the whole superconducting wire at times indicated in the$T\text-t$ curve.图 8 在
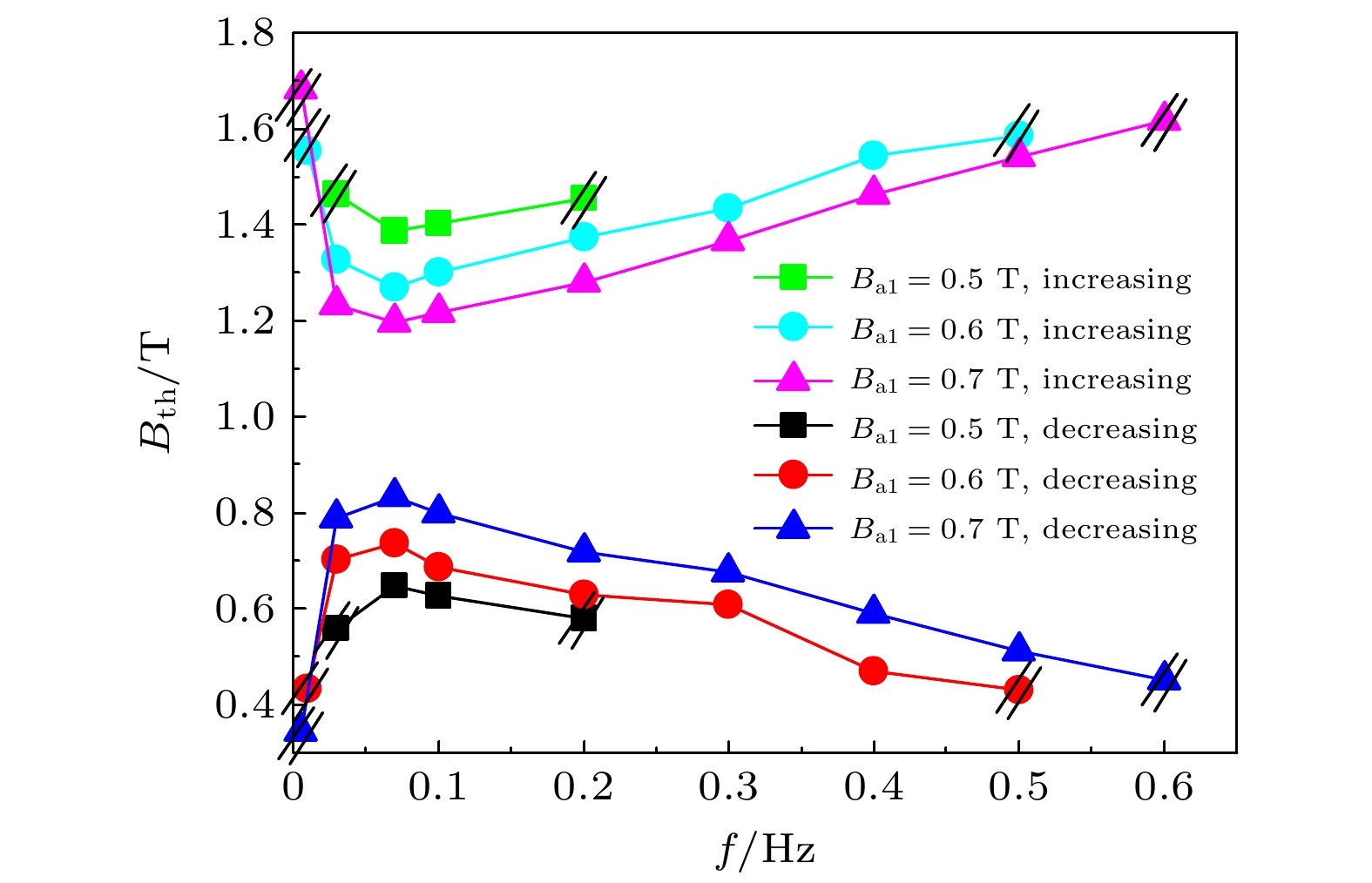
$ T_0 = 4.2\ {\text{K}} $ 下, 不同幅值下超导线发生初次磁通跳跃的磁场阈值$B_{{\rm{th}}}$ 随频率f的变化(图中双斜线表示不发生磁通跳跃的临界点)Figure 8. The variations of magnetic field threshold of the initial flux jump
$B_{{\rm{th}}}$ versus frequency f at different amplitudes at$ T_0 = 4.2\ {\text{K}} $ (The double slash lines in the figure indicate critical points where flux jump does not occur)图 9 在
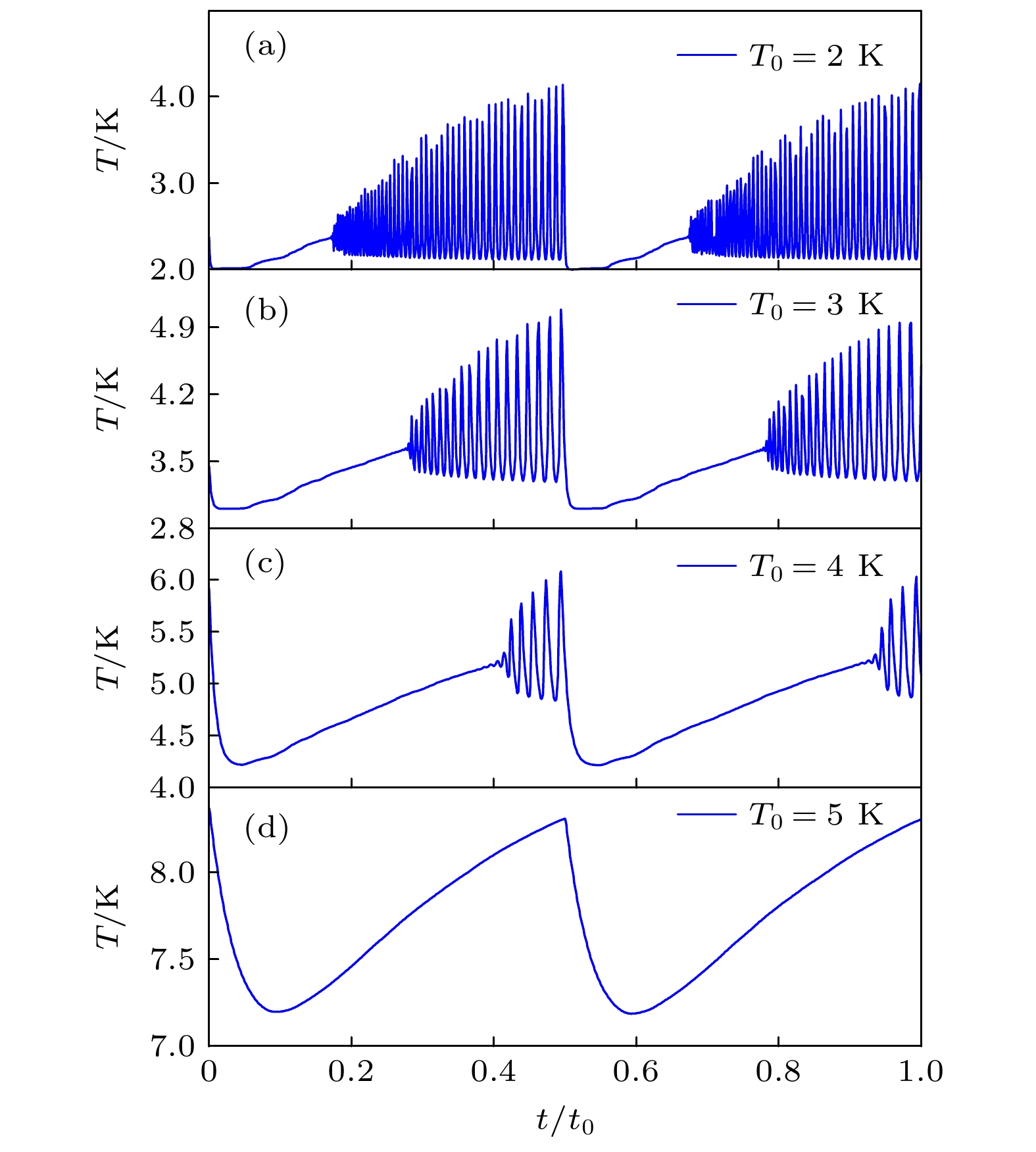
$ T_0 = 4.2\ {\text{K}} $ 和频率为$ 0.3\ {\text{Hz}} $ 时, 不同幅值下超导线的平均温度随时间的变化, 插图①—④分别展示了在$T\text-t/t_{0}$ 曲线上对应时刻整根超导线的电流密度图Figure 9. The developments of average temperature of a superconducting wire as a function of time for different amplitudes at the frequency of 0.3 Hz and
$ T_0 = 4.2\ {\text{K}} $ .The insets ①–④ show the contours of current density of the whole superconducting wire at times indicated in the$T\text-t/t_{0}$ curve图 10 在
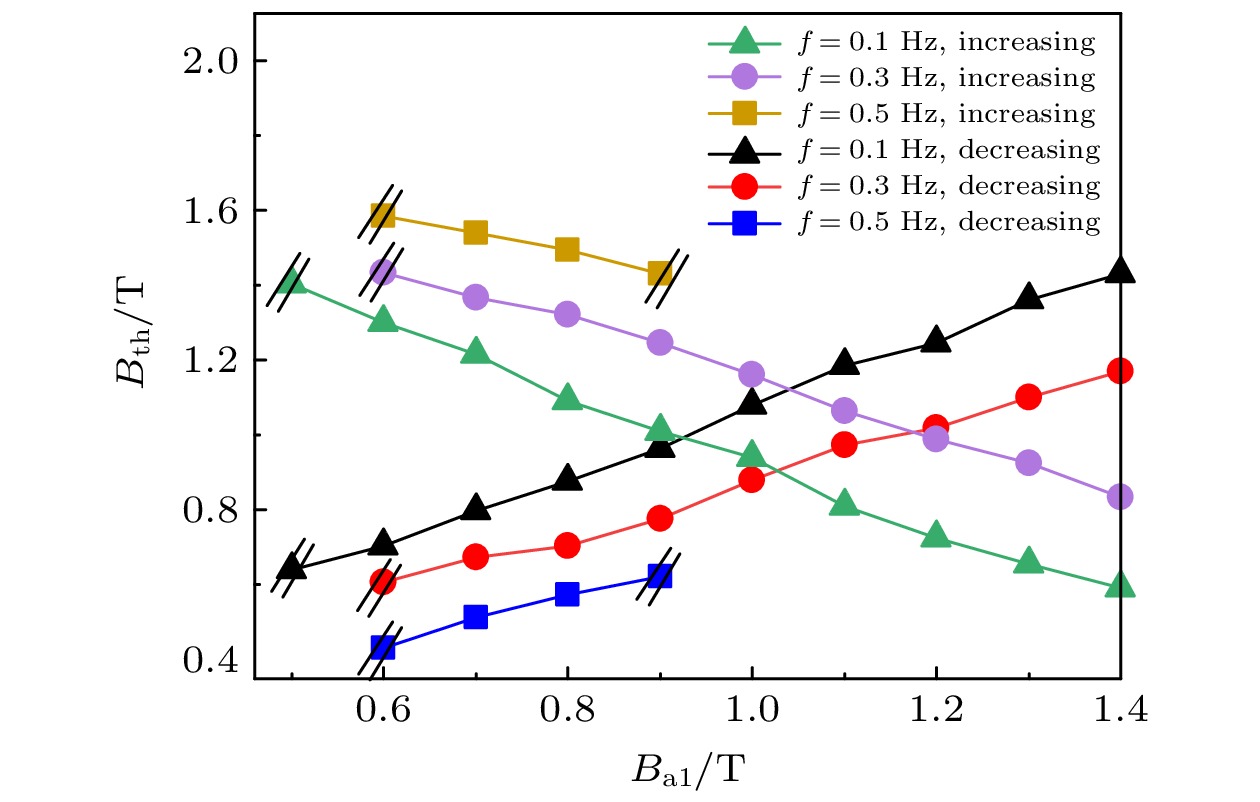
$ T_0 = 4.2\ {\text{K}} $ 下, 不同频率时初次发生磁通跳跃的磁场阈值$B_{{\rm{th}}}$ 随幅值$B_{{\rm{a}}1}$ 的变化(图中双斜线表示不发生磁通跳跃的临界点)Figure 10. At
$ T_0 = 4.2\ {\text{K}} $ , the variations of magnetic field threshold of the initial flux jump$B_{{\rm{th}}}$ with the amplitude$B_{{\rm{a}}1}$ at different frequencies (The double slash lines in the figure indicate critical points where flux jumping does not occur)图 11 (a)在
$ T_0 = 4.2\ {\text{K}} $ 时超导线发生磁通跳跃的频率和幅值的临界阈值曲线, 其中黑色实线所包围的蓝色区域为超导线发生磁通跳跃的区域, 其余灰色区域为磁通平稳穿透的区域. (b)—(g)分别展示了图(a)中6个点对应情况下超导线的平均电场和平均温度随时间的变化, 插图$ a_1 $ —$ c_2 $ 表示$ \bar{E_z}-t $ 曲线的最高点(黑色实心圆点)对应时刻超导线的电流密度Figure 11. At
$ T_0 = 4.2\ {\text{K}} $ , (a)the critical threshold of frequency and amplitude(black curve)when flux jump will happen in a superconducting wire. The blue region is the region where flux jump occurs, and the rest gray region is the region where smooth penetration occurs; (b)–(g) show the variations of the average electric field and temperature of the superconducting wire with time under the corresponding conditions of the 6 points in (a); Panels$ a_1 $ –$ c_2 $ show the current density of the superconducting wire at times (black solid dots) corresponding to the highest points of the$ \bar{E_z}-t $ curves图 13 不同频率和幅值下初次发生磁通跳跃的磁场阈值
$ B_{{\rm{th}}} $ 随背景温度$ T_0 $ 的变化(图中双斜线表示不发生磁通跳跃的临界点)Figure 13. The variations of magnetic field threshold of the initial flux jump
$ B_{{\rm{th}}} $ with the background temperature$ T_0 $ at different frequencies and amplitudes (The double slash lines in the figure indicate critical points where flux jump does not occur) -
[1] 杨晶, 朴明伟, 高文斌, 傅凯, 高辉 2019 计算机集成制造系统 25 1908
 Google Scholar
Google Scholar
Yang J, Piao M W, Gao W B, Fu K, Gao H 2019 Comput. Integr. Manuf. Syst. 25 1908
 Google Scholar
Google Scholar
[2] 熊嘉阳, 邓自刚 2021 交通运输工程学报 21 177
 Google Scholar
Google Scholar
Xiong J Y, Deng Z G 2021 J. Traffic Transp. Eng. 21 177
 Google Scholar
Google Scholar
[3] Saijo T, Koike S, Tadakuma S 1981 IEEE Trans. Ind. Appl. 17 533
 Google Scholar
Google Scholar
[4] Kyotani Y 1988 IEEE Trans. Magn. 24 804
 Google Scholar
Google Scholar
[5] Meins J, Miller L, Mayer W 1988 IEEE Trans. Magn. 24 808
 Google Scholar
Google Scholar
[6] Schultz L, de Haas O, Verges P, Beyer C, Rohlig S, Olsen H, Kuhn L, Berger D, Noteboom U, Funk U 2005 IEEE Trans. Appl. Supercond. 15 2301
 Google Scholar
Google Scholar
[7] Okano M, Iwamoto T, Furuse M, Fuchino S, Ishii I 2006 J. Phys.: Conf. Ser. 43 999
 Google Scholar
Google Scholar
[8] Kusada S, Igarashi M, Nemoto K, Okutomi T, Hirano S, Kuwano K, Tominaga T, Terai M, Kuriyama T, Tasaki K 2007 IEEE Trans. Appl. Supercond. 17 2111
 Google Scholar
Google Scholar
[9] Wang J, Wang S, Zeng Y, Huang H, Luo F, Xu Z, Tang Q, Lin G, Zhang C, Ren Z 2002 Physica C 378 809
 Google Scholar
Google Scholar
[10] Stumberger G, Aydemir M T, Zarko D, Lipo T A 2004 IEEE Trans. Appl. Supercond. 14 54
 Google Scholar
Google Scholar
[11] 胡赣娟 2005 硕士 (浙江: 浙江大学)
Hu G J 2005 M. S. Thesis (Zhejiang: Zhejiang University) (in Chinese)
[12] Turman B, Marder B, Rohwein G, Aeschliman D, KelIey J, Cowan M, Zimmerman R 1995 Sandia National Laboratories, the United States Department of Energy
[13] 闻海虎 2021 70 143
 Google Scholar
Google Scholar
Wen H H 2021 Acta Phys. Sin. 70 143
 Google Scholar
Google Scholar
[14] Mints R 1996 Phys. Rev. B 53 12311
 Google Scholar
Google Scholar
[15] Kim Y, Hempstead C, Strnad A 1963 Phys. Rev. 129 528
 Google Scholar
Google Scholar
[16] Neuringer L, Shapira Y 1966 Phys. Rev. 148 231
 Google Scholar
Google Scholar
[17] Wertheimer M R, Gilchrist J le G 1967 J. Phys. Chem. Solids 28 2509
 Google Scholar
Google Scholar
[18] Mints R, Rakhmanov A 1981 Rev. Mod. Phys. 53 551
 Google Scholar
Google Scholar
[19] Wipf S L 1991 Cryog. 31 936
 Google Scholar
Google Scholar
[20] Nabialek A, Niewczas M, Dabkowska H, Dabkowski A, Castellan J, Gaulin B 2003 Phys. Rev. B 67 024518
 Google Scholar
Google Scholar
[21] Hirano T, Fujishiro H, Naito T, Ainslie M D 2020 Supercond. Sci. Technol. 33 044003
 Google Scholar
Google Scholar
[22] Zhou Y H, Yang X B 2006 Phys. Rev. B 74 054507
 Google Scholar
Google Scholar
[23] Yang X B, Zhou Y H, Tu S D 2010 Physica C 470 109
 Google Scholar
Google Scholar
[24] Swartz P, Bean C 1968 J. Appl. Phys. 39 4991
 Google Scholar
Google Scholar
[25] Bean C P 1962 Phys. Rev. Lett. 8 250
 Google Scholar
Google Scholar
[26] Müller K H, Andrikidis C 1994 Phys. Rev. B 49 1294
 Google Scholar
Google Scholar
[27] Gou X F, Zheng X J, Zhou Y H 2007 IEEE Trans. Appl. Supercond. 17 3795
 Google Scholar
Google Scholar
[28] Kashikhin V V, Zlobin A V 2005 IEEE Trans. Appl. Supercond. 15 1621
 Google Scholar
Google Scholar
[29] Zhang W W, Xia J, Yong H D, Zhou Y H 2020 AIP Adv. 10 025021
 Google Scholar
Google Scholar
[30] Zhao Y F, Xia T D, He T H, Feng W J 2010 J. Low Temp. Phys. 160 201
 Google Scholar
Google Scholar
[31] Wang Q Y, Xue C, Chen Y Q, Ou X J, Wu W, Liu W, Ma P, Sun L T, Zhao H W, Zhou Y H 2022 Physica C 593 1354002
 Google Scholar
Google Scholar
[32] Lahtinen V, Stenvall A 2013 IEEE Trans. Appl. Supercond. 24 1
 Google Scholar
Google Scholar
[33] Barzi E, Limon P J, Yamada R, Zlobin A V 2001 IEEE Trans. Appl. Supercond. 11 3595
 Google Scholar
Google Scholar
Catalog
Metrics
- Abstract views: 5633
- PDF Downloads: 83
- Cited By: 0



















 DownLoad:
DownLoad: