-
At a half-filled Landau level, composite fermions with chiral p-wave pairing will form a Moore-Read state which hosts charge-e/4 fractional excitation. This excitation supports non-Abelian statistics and has potential to enable topological quantum computation. Owing to the SU(4) symmetry of electron and electric-field tunability, the bilayer graphene becomes an ideal platform for exploring physics of multi-component quantum Hall state and is candidate for realizing non-Abelian statistics. In this work, high-quality bilayer graphene/hBN heterostructure is fabricated by using dry-transfer technique, and electric transport measurement is performed to study quantum Hall state behavior in bilayer graphene under electric field and magnetic field. Under strong magnetic field, the sequences of incompressible state with quantized Hall conductivity are revealed at –5/2, –1/2, 3/2 filling of Landau level. The feature of even-denominator quantum Hall state is more visible then weaker with increasing magnetic field, and this corresponds to the polarization of Landau level wave function. The experimental results indicate that the observed even-denominator fractional quantum Hall state belongs to the topological phase described by Pfaffian wavefunction.
-
Keywords:
- graphene /
- fractional quantum Hall effect /
- topological phase
[1] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[2] Jain J K 1989 Phys. Rev. Lett. 63 199
Google Scholar
[3] Lopez A, Fradkin E 1991 Phys. Rev. B 44 5246
Google Scholar
[4] Halperin B I, Lee P A, Read N 1993 Phys. Rev. B Condens. Matter. 47 7312
Google Scholar
[5] Moore G, Read N 1991 Nuclear Phys. B 360 362
Google Scholar
[6] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Modern Phys. 80 1083
Google Scholar
[7] Willett R, Eisenstein J P, Stormer H L, Tsui D C, Gossard A C, English J H 1987 Phys. Rev. Lett. 59 1776
Google Scholar
[8] Pan W, Xia J S, Shvarts V, et al. 1999 Phys. Rev. Lett. 83 3530
Google Scholar
[9] Banerjee M, Heiblum M, Umansky V, Feldman D E, Oreg Y, Stern A 2018 Nature 559 205
Google Scholar
[10] Lin X, Dillard C, Kastner M A, Pfeiffer L N, West K W 2012 Phys. Rev. B 85 165321
[11] Baer S, Rössler C, Ihn T, Ensslin K, Reichl C, Wegscheider W 2014 Phys. Rev. B 90 075403
[12] Zibrov A A, Spanton E M, Zhou H, Kometter C, Taniguchi T, Watanabe K, Young A F 2018 Nature Phys. 14 930
Google Scholar
[13] Kim Y, Balram A C, Taniguchi T, Watanabe K, Jain J K, Smet J H 2018 Nature Phys. 15 154
[14] Goerbig M O 2011 Rev. Mod. Phys. 83 1193
Google Scholar
[15] Young A F, Dean C R, Wang L, et al. 2012 Nature Phys. 8 550
Google Scholar
[16] Lee K, Fallahazad B, Xue J, Dillen D C, Kim K, Taniguchi T, Watanabe K, Tutuc E 2014 Science 345 58
Google Scholar
[17] Kou A, Feldman B E, Levin A J, Halperin B I, Watanabe K, Taniguchi T, Yacoby A 2014 Science 345 55
Google Scholar
[18] Maher P, Wang L, Gao Y, et al. 2014 Science 345 61
Google Scholar
[19] Apalkov V M, Chakraborty T 2011 Phys. Rev. Lett. 107 186803
Google Scholar
[20] Papic Z, Abanin D A 2014 Phys. Rev. Lett. 112 046602
Google Scholar
[21] Zhu Z, Sheng D N, Sodemann I 2020 Phys. Rev. Lett. 124 097604
Google Scholar
[22] Zibrov A A, Kometter C, Zhou H, et al. 2017 Nature 549 360
Google Scholar
[23] Li J I A, Tan C, Chen S, et al. 2017 Science 358 648
Google Scholar
[24] Wang L, Meric I, Huang P Y, et al. 2013 Science 342 614
Google Scholar
[25] Hunt B M, Li J I A, Zibrov A A, et al. 2017 Nat. Commun. 8 948
Google Scholar
[26] McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503
Google Scholar
[27] Rezayi E H, Simon S H 2011 Phys. Rev. Lett. 106 116801
Google Scholar
[28] Wu Y H, Shi T, Jain J K 2017 Nano Lett. 17 4643
Google Scholar
[29] Rezayi E H 2017 Phys. Rev. Lett. 119 026801
Google Scholar
[30] Wang Y, Ponomarenko V, Wan Z, West K W, Baldwin K W, Pfeiffer L N, Lyanda-Geller Y, Rokhinson L P 2021 Nat. Commun. 12 5312
Google Scholar
[31] Wei D S, van der Sar T, Lee S H, Watanabe K, Taniguchi T, Halperin B I, Yacoby A 2018 Science 362 229
Google Scholar
[32] Nakamura J, Liang S, Gardner G C, Manfra M J 2020 Nature Phys. 16 931
Google Scholar
[33] Bartolomei H, Kumar M, Bisognin R, et al. 2020 Science 368 173
Google Scholar
-
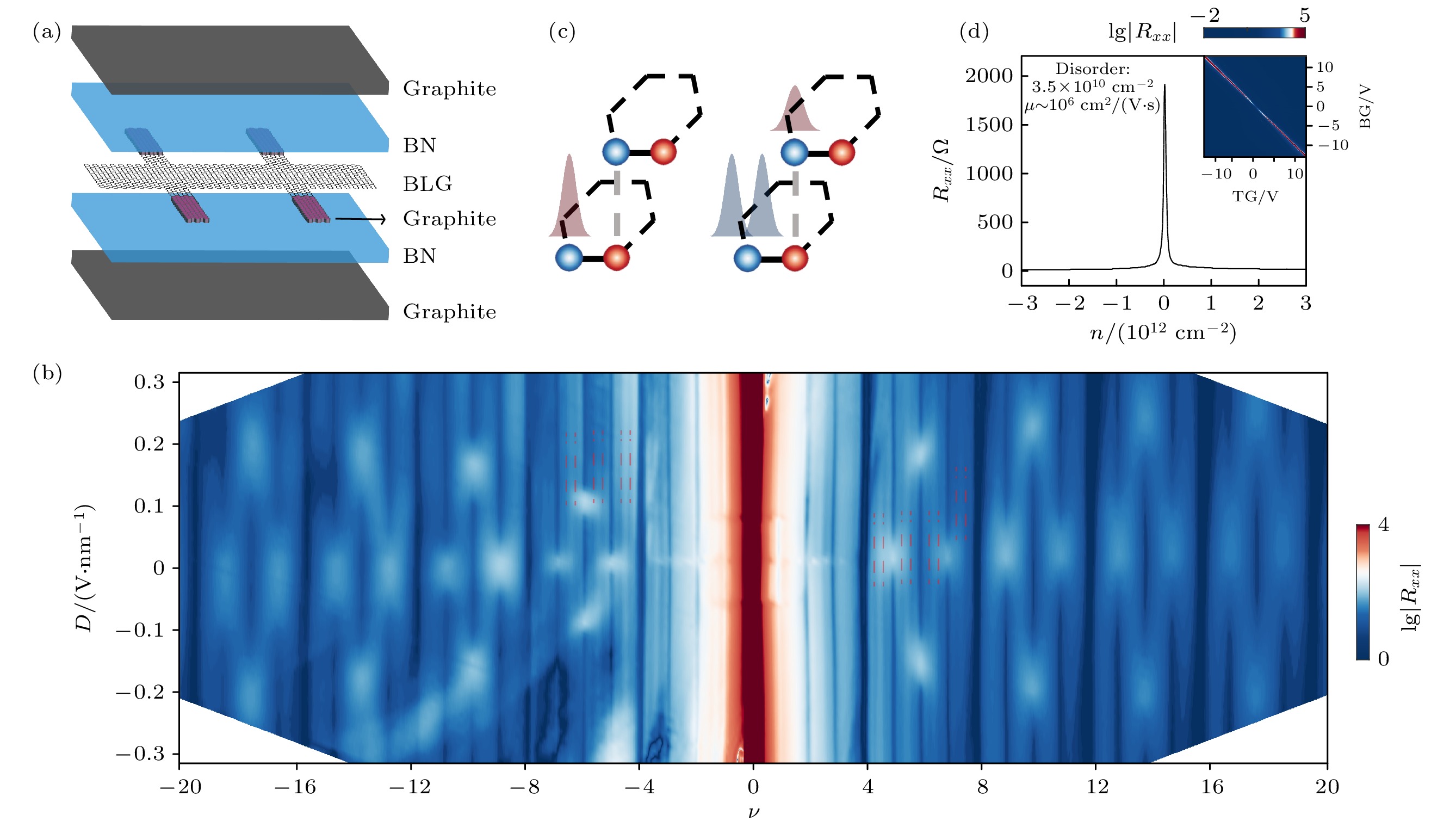
图 1 器件结构和双层石墨烯里的分数量子霍尔态 (a) 器件结构图; (b) 温度T = 1.5 K与磁场B = 8 T时, 纵向电阻Rxx随电位移场D和填充因子ν的变化图(红色虚线表示以3为分母的分数态) (c) 双层石墨烯N = 0, 1朗道能级中四原子上波函数分布图; (d) 温度T = 1.5 K, 电位移场D = 0 V/nm与磁场B = 0 T时, 纵向电阻Rxx随载流子浓度n的变化
Figure 1. Device structure and FQH states for in bilayer graphene (BLG): (a) Device schematic; (b) Rxx versus ν and D at B = 8 T and T = 1.5 K (The red dotted lines represent the fractional state with 3 as the denominator); (c) the schematic of wave function distribution on the four atomic sites of BLG for the N = 0, 1 Landau orbital states; (d) Rxx versus n at D = 0 V/nm, B = 0 T, and T = 1.5 K.
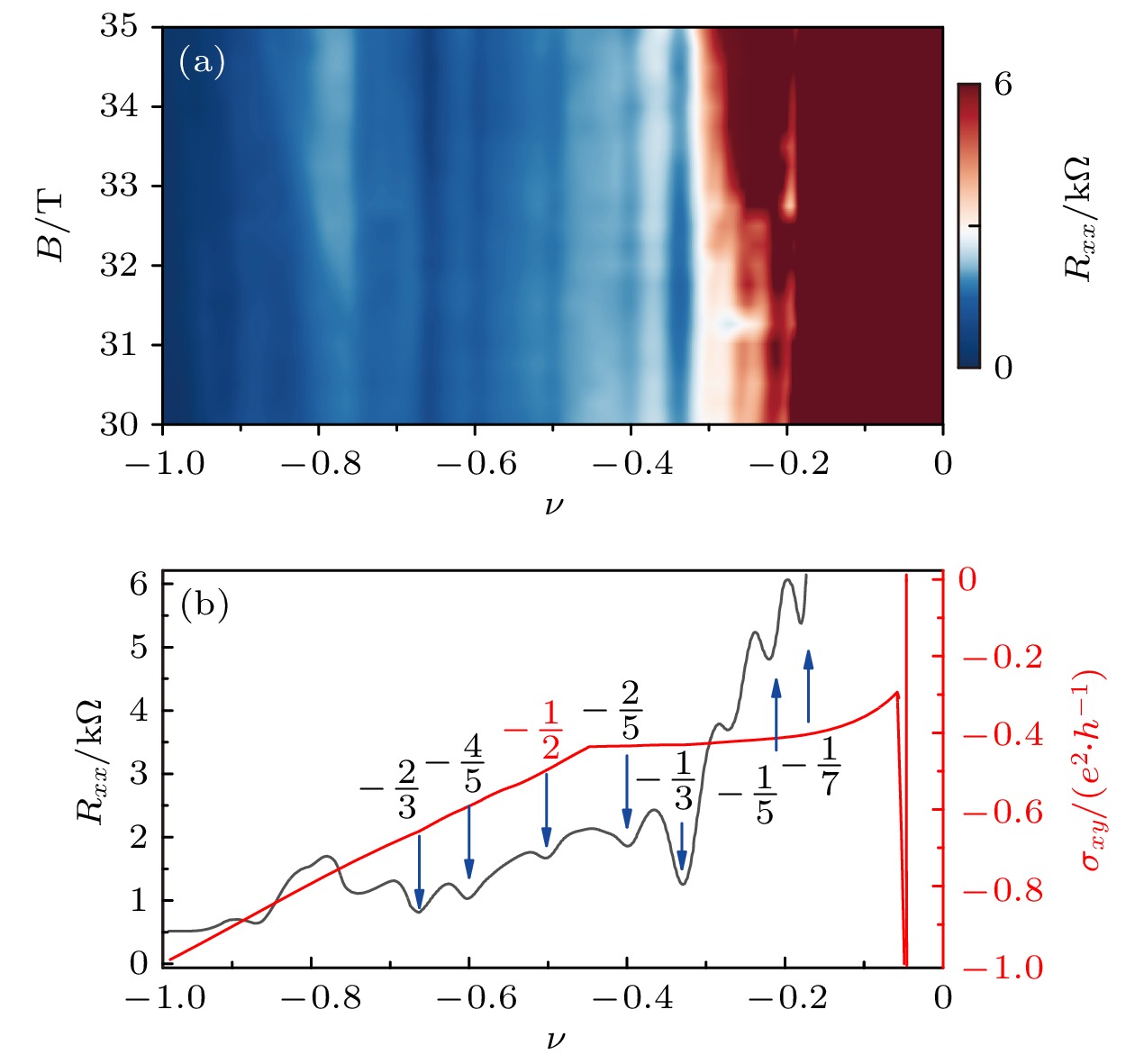
图 2 1 < ν < 2时的分数量子霍尔态 (a) 温度T = 0.3 K与电位移场D = –0.12 V/nm时, 纵向电阻Rxx随填充因子ν和磁场B变化的二维图; (b) 固定温度T = 0.3 K、电位移场D = –0.12 V/nm与磁场B = 33.75 T时, 纵向电阻Rxx (黑色)和横向电导σxy (红色)随ν的变化
Figure 2. FQH states for 1 < ν < 2: (a) Rxx as a function of ν and B at T = 0.3 K and D = –0.12 V/nm; (b) Rxx (black) and σxy (red) versus ν at B = 33.75 T, D = –0.12 V/nm and T = 0.3 K.
图 4 –2
< ν < –3时的分数量子霍尔态 (a) 温度T = 0.3 K与电位移场D = –0.12 V/nm时, 纵向电阻Rxx随填充因子ν和磁场B变化的二维图; (b) 固定温度T = 0.3 K、电位移场D = –0.12 V/nm与磁场B = 28 T时, 纵向电阻Rxx和横向电导σxy随ν的变化 Figure 4. FQH states for –2
< ν < –3: (a) Rxx as a function of filling factor ν and magnetic field B at T = 0.3 K and D = –0.12 V/nm; (b) Rxx (black) and σxy (red) versus ν at B = 28 T, D = –0.12 V/nm and T = 0.3 K. 
图 3 –1 < ν < 0时的分数量子霍尔态 (a) 温度T = 0.3 K与电位移场D = –0.12 V/nm时, 纵向电阻Rxx随填充因子ν和磁场B变化的二维图; (b) 固定温度T = 0.3 K、电位移场D = –0.12 V/nm与磁场B = 31.58 T时, 纵向电阻Rxx (黑色)和横向电导σxy (红色)随填充因子ν的变化
Figure 3. FQH states for –1 < ν < 0: (a) Rxx as a function of ν and magnetic field B at T = 0.3 K and D = –0.12 V/nm; (b) Rxx (black) and σxy (red) versus ν at B = 31.58 T, D = –0.12 V/nm and T = 0.3 K.
图 5 不同磁场下分数量子霍尔态的演化 (a)—(c) 分别描述了温度T = 0.3 K与电位移场D = –0.12 V/nm时, 不同磁场下纵向电阻Rxx随填充因子ν的变化 (为了能在不同磁场下显示更清楚, 每条曲线都加入了偏移量), 其中(a) –3 < ν <
–2, (b) –1 < ν < 0和(c) 1 < ν < 2 Figure 5. Evolution of FQH states under different magnetic fields: (a)–(c) Rxx as a function of filling factor ν at T = 0.3 K and D = –0.12 V/nm for different magnetic field, corresponding to filling fractions spanning (a) –3 < ν < –2, (b) –1 < ν < 0, and (c) 1 < ν < 2.
-
[1] Tsui D C, Stormer H L, Gossard A C 1982 Phys. Rev. Lett. 48 1559
Google Scholar
[2] Jain J K 1989 Phys. Rev. Lett. 63 199
Google Scholar
[3] Lopez A, Fradkin E 1991 Phys. Rev. B 44 5246
Google Scholar
[4] Halperin B I, Lee P A, Read N 1993 Phys. Rev. B Condens. Matter. 47 7312
Google Scholar
[5] Moore G, Read N 1991 Nuclear Phys. B 360 362
Google Scholar
[6] Nayak C, Simon S H, Stern A, Freedman M, Das Sarma S 2008 Rev. Modern Phys. 80 1083
Google Scholar
[7] Willett R, Eisenstein J P, Stormer H L, Tsui D C, Gossard A C, English J H 1987 Phys. Rev. Lett. 59 1776
Google Scholar
[8] Pan W, Xia J S, Shvarts V, et al. 1999 Phys. Rev. Lett. 83 3530
Google Scholar
[9] Banerjee M, Heiblum M, Umansky V, Feldman D E, Oreg Y, Stern A 2018 Nature 559 205
Google Scholar
[10] Lin X, Dillard C, Kastner M A, Pfeiffer L N, West K W 2012 Phys. Rev. B 85 165321
[11] Baer S, Rössler C, Ihn T, Ensslin K, Reichl C, Wegscheider W 2014 Phys. Rev. B 90 075403
[12] Zibrov A A, Spanton E M, Zhou H, Kometter C, Taniguchi T, Watanabe K, Young A F 2018 Nature Phys. 14 930
Google Scholar
[13] Kim Y, Balram A C, Taniguchi T, Watanabe K, Jain J K, Smet J H 2018 Nature Phys. 15 154
[14] Goerbig M O 2011 Rev. Mod. Phys. 83 1193
Google Scholar
[15] Young A F, Dean C R, Wang L, et al. 2012 Nature Phys. 8 550
Google Scholar
[16] Lee K, Fallahazad B, Xue J, Dillen D C, Kim K, Taniguchi T, Watanabe K, Tutuc E 2014 Science 345 58
Google Scholar
[17] Kou A, Feldman B E, Levin A J, Halperin B I, Watanabe K, Taniguchi T, Yacoby A 2014 Science 345 55
Google Scholar
[18] Maher P, Wang L, Gao Y, et al. 2014 Science 345 61
Google Scholar
[19] Apalkov V M, Chakraborty T 2011 Phys. Rev. Lett. 107 186803
Google Scholar
[20] Papic Z, Abanin D A 2014 Phys. Rev. Lett. 112 046602
Google Scholar
[21] Zhu Z, Sheng D N, Sodemann I 2020 Phys. Rev. Lett. 124 097604
Google Scholar
[22] Zibrov A A, Kometter C, Zhou H, et al. 2017 Nature 549 360
Google Scholar
[23] Li J I A, Tan C, Chen S, et al. 2017 Science 358 648
Google Scholar
[24] Wang L, Meric I, Huang P Y, et al. 2013 Science 342 614
Google Scholar
[25] Hunt B M, Li J I A, Zibrov A A, et al. 2017 Nat. Commun. 8 948
Google Scholar
[26] McCann E, Koshino M 2013 Rep. Prog. Phys. 76 056503
Google Scholar
[27] Rezayi E H, Simon S H 2011 Phys. Rev. Lett. 106 116801
Google Scholar
[28] Wu Y H, Shi T, Jain J K 2017 Nano Lett. 17 4643
Google Scholar
[29] Rezayi E H 2017 Phys. Rev. Lett. 119 026801
Google Scholar
[30] Wang Y, Ponomarenko V, Wan Z, West K W, Baldwin K W, Pfeiffer L N, Lyanda-Geller Y, Rokhinson L P 2021 Nat. Commun. 12 5312
Google Scholar
[31] Wei D S, van der Sar T, Lee S H, Watanabe K, Taniguchi T, Halperin B I, Yacoby A 2018 Science 362 229
Google Scholar
[32] Nakamura J, Liang S, Gardner G C, Manfra M J 2020 Nature Phys. 16 931
Google Scholar
[33] Bartolomei H, Kumar M, Bisognin R, et al. 2020 Science 368 173
Google Scholar
DownLoad:
Catalog
Metrics
- Abstract views: 4868
- PDF Downloads: 238
- Cited By: 0
